Hostname: page-component-cd9895bd7-8ctnn Total loading time: 0 Render date: 2024-12-24T13:15:27.511Z Has data issue: false hasContentIssue false
  • English
  • Français

Trajectory of a synthetic jet issuing into high-Reynolds-number turbulent boundary layers

Published online by Cambridge University Press:  05 October 2018

Tim Berk Open the ORCID record for Tim Berk [Opens in a new window] ,
Nicholas Hutchins ,
Ivan Marusic  and
Bharathram Ganapathisubramani
Tim Berk
Affiliation:
Aerodynamics and Flight Mechanics Research Group, University of Southampton, Southampton SO17 1BJ, UK
Nicholas Hutchins
Affiliation:
Department of Mechanical Engineering, The University of Melbourne, Victoria 3010, Australia
Ivan Marusic
Affiliation:
Department of Mechanical Engineering, The University of Melbourne, Victoria 3010, Australia
Bharathram Ganapathisubramani*
Affiliation:
Aerodynamics and Flight Mechanics Research Group, University of Southampton, Southampton SO17 1BJ, UK
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Synthetic jets are zero-net-mass-flux actuators that can be used in a range of flow control applications. For some applications, the scaling of the trajectory of the jet with actuation and cross-flow parameters is important. This scaling is investigated for changes in the friction Reynolds number, changes in the velocity ratio (defined as the ratio between the mean jet blowing velocity and the free-stream velocity) and changes in the actuation frequency of the jet. 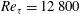A distinctive aspect of this study is the high-Reynolds-number turbulent boundary layers (up to $Re_{\unicode[STIX]{x1D70F}}=12\,800$) of the cross-flow. To our knowledge, this is the first study to investigate the effect of the friction Reynolds number of the cross-flow on the trajectory of an (unsteady) jet, as well as the first study to systematically investigate the scaling of the trajectory with actuation frequency. A broad range of parameters is varied (rather than an in-depth investigation of a single parameter) and the results of this study are meant to indicate the relative importance of each parameter rather than the exact influence on the trajectory. Within the range of parameters explored, the critical ones are found to be the velocity ratio as well as a non-dimensional frequency based on the jet actuation frequency, the cross-flow velocity and the jet dimensions. The Reynolds number of the boundary layer is shown to have only a small effect on the trajectory. An expression for the trajectory of the jet is derived from the data, which (in the limit) is consistent with known expressions for the trajectory of a steady jet in a cross-flow.

JFM classification

Wakes/Jets: Jets Turbulent Flows: Turbulent boundary layers
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 856 , 10 December 2018 , pp. 531 - 551
Check for updates
Copyright
© 2018 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Abbassi, M. R., Baars, W., Hutchins, N. & Marusic, I. 2017 Skin-friction drag reduction in a high-Reynolds-number turbulent boundary layer via real-time control of large-scale structures. Intl J. Heat Fluid Flow 67 (B), 30–41.Google Scholar
Broadwell, J. & Breidenthal, R. 1984 Structure and mixing of a transverse jet in incompressible flow. J. Fluid Mech. 148, 405–412.Google Scholar
Cater, J. E. & Soria, J. 2002 The evolution of round zero-net-mass-flux jets. J. Fluid Mech. 472, 167–200.Google Scholar
Chauhan, K. A., Monkewitz, P. A. & Nagib, H. M. 2009 Criteria for assessing experiments in zero pressure gradient boundary layers. Fluid Dyn. Res. 41, 021404.Google Scholar
Dandois, J., Garnier, E. & Sagaut, P. 2007 Numerical simulation of active separation control by a synthetic jet. J. Fluid Mech. 574, 25–58.Google Scholar
Davitian, J., Hendrickson, C., M’Closkey, R. T. & Karagozian, A. R. 2010 Strategic control of transverse jet shear layer instabilities. AIAA J. 48 (9), 2145–2156.Google Scholar
Eroglu, A. & Breidenthal, R. E. 2001 Structure, penetration, and mixing of pulsed jets in crossflow. AIAA J. 39 (3), 417–423.Google Scholar
Fric, T. & Roshko, A. 1994 Vortical structure in the wake of a transverse jet. J. Fluid Mech. 279, 1–47.Google Scholar
Gharib, M., Rambod, E. & Shariff, K. 1998 A universal time scale for vortex ring formation. J. Fluid Mech. 360, 121–140.Google Scholar
Hussain, A. & Reynolds, W. 1970 The mechanics of an organized wave in turbulent shear flow. J. Fluid Mech. 41, 241–258.Google Scholar
Hutchins, N., Nickels, T. B., Marusic, I. & Chong, M. S. 2009 Hot-wire spatial resolution issues in wall-bounded turbulence. J. Fluid Mech. 635, 103–136.Google Scholar
Jabbal, M. & Zhong, S. 2008 The near wall effect of synthetic jets in a boundary layer. Intl J. Heat Fluid Flow 29 (1), 119–130.Google Scholar
Jabbal, M. & Zhong, S. 2010 Particle image velocimetry measurements of the interaction of synthetic jets with a zero-pressure gradient laminar boundary layer. Phys. Fluids 22 (6), 063603.Google Scholar
Johari, H. 2006 Scaling of fully pulsed jets in crossflow. AIAA J. 44 (11), 2719–2725.Google Scholar
Johari, H., Pacheco-Tougas, M. & Hermanson, J. C. 1999 Penetration and mixing of fully modulated turbulent jets in crossflow. AIAA J. 37 (7), 842–850.Google Scholar
Karagozian, A. R. 1986 An analytical model for the vorticity associated with a transverse jet. AIAA J. 24 (3), 429–436.Google Scholar
Karagozian, A. R. 2014 The jet in crossflow. Phys. Fluids 26, 101303.Google Scholar
Keffer, J. F. & Baines, W. D. 1962 The round turbulent jet in a cross-wind. J. Fluid Mech. 15 (4), 481–496.Google Scholar
Kim, W., Kim, C. & Jung, K. J. 2012 Separation control characteristics of synthetic jets depending on exit configuration. AIAA J. 50 (3), 559–570.Google Scholar
Klewicki, J. 2010 Reynolds number dependence, scaling, and dynamics of turbulent boundary layers. Trans. ASME J. Fluids Engng 132 (9), 094001.Google Scholar
Lim, T. T., Lua, K. B. & Thet, K. 2008 Does Kutta lift exist on a vortex ring in a uniform cross flow? Phys. Fluids 20 (5), 051701.Google Scholar
Mahesh, K. 2013 The interaction of jets with crossflow. Annu. Rev. Fluid Mech. 45 (1), 379–407.Google Scholar
Margason, R. J. 1993 Fifty years of jet in cross flow research. In AGARD, Computational and Experimental Assessment of Jets in Cross Flow, AGARD–CP–534, Advisory Group for Aerospace Research and Development.Google Scholar
Marusic, I., Mathis, R. & Hutchins, N. 2010 Predictive model for wall-bounded turbulent flow. Science 329 (5988), 193–196.Google Scholar
M’Closkey, R. T., King, J. M., Cortelezzi, L. & Karagozian, A. R. 2002 The actively controlled jet in crossflow. J. Fluid Mech. 452, 325–335.Google Scholar
Megerian, S., Davitian, J., Alves, B. & Karagozian, A. R. 2007 Transverse-jet shear-layer instabilities. Part 1. Experimental studies. J. Fluid Mech. 593, 93–129.Google Scholar
Miller, D. N., Yagle, P. J., Bender, E. E. & Smith, B. R. 2001 A computational investigation of pulsed injection into a confined expanding crossflow. In 15th AIAA Computational Fluid Dynamics Conference, Anaheim, CA, pp. 2001–3026. American Institute of Aeronautics and Astronautics.Google Scholar
Muldoon, F. & Acharya, S. 2009 DNS study of pulsed film cooling for enhanced cooling effectiveness. Intl J. Heat Mass Transfer 52, 3118–3127.Google Scholar
Muppidi, S. & Mahesh, K. 2005 Study of trajectories of jets in crossflow using direct numerical simulations. J. Fluid Mech. 530, 81–100.Google Scholar
Narayanan, S., Barooah, P. & Cohen, J. M. 2003 Dynamics and control of an isolated jet in crossflow. AIAA J. 41 (12), 2316–2330.Google Scholar
Nickels, T. B., Marusic, I., Hafez, S. & Chong, M. S. 2005 Evidence of the k 1 -1 law in a high-Reynolds-number turbulent boundary layer. Phys. Rev. Lett. 95 (7), 074501.Google Scholar
O’Farrell, C. & Dabiri, J. O. 2014 Pinch-off of non-axisymmetric vortex rings. J. Fluid Mech. 740, 61–96.Google Scholar
Rathnasingham, R. & Breuer, K. S. 2003 Active control of turbulent boundary layers. J. Fluid Mech. 495, 209–233.Google Scholar
Sau, R. & Mahesh, K. 2008 Dynamics and mixing of vortex rings in crossflow. J. Fluid Mech. 604, 389–409.Google Scholar
Shapiro, S. R., King, J. M., M’Closkey, R. T. & Karagozian, A. R. 2006 Optimization of controlled jets in crossflow. AIAA J. 44 (6), 1292–1298.Google Scholar
Shuster, J., Pink, R., McEligot, D. & Smith, D. 2005 The interaction of a circular synthetic jet with a cross-flow boundary layer. In 35th AIAA Fluid Dynamics Conference and Exhibit, Toronto, ON. American Institute of Aeronautics and Astronautics.Google Scholar
Smith, B. L. & Glezer, A. 1998 The formation and evolution of synthetic jets. Phys. Fluids 10 (9), 2281–2297.Google Scholar
Smith, D. R. 2002 Interaction of a synthetic jet with a crossflow boundary layer. AIAA J. 40 (11), 2277–2288.Google Scholar
Smith, S. H. & Mungal, M. G. 1998 Mixing, structure and scaling of the jet in crossflow. J. Fluid Mech. 357, 83–122.Google Scholar
Smits, A. J., McKeon, B. J. & Marusic, I. 2011 High Reynolds number wall turbulence. Annu. Rev. Fluid Mech. 43 (1), 353–375.Google Scholar
Van Buren, T. & Amitay, M. 2016 Comparison between finite-span steady and synthetic jets issued into a quiescent fluid. Exp. Therm. Fluid Sci. 75, 16–24.Google Scholar
Van Buren, T., Whalen, E. & Amitay, M. 2014 Vortex formation of a finite-span synthetic jet: effect of rectangular orifice geometry. J. Fluid Mech. 745, 180–207.Google Scholar
Van Buren, T., Leong, C. M., Whalen, E. & Amitay, M. 2016 Impact of orifice orientation on a finite-span synthetic jet interaction with a crossflow. Phys. Fluids 28, 037106.Google Scholar
Vermeulen, P. J., Chin, C. & Yu, W. K. 1990 Mixing of an acoustically pulsed air jet with a confined crossflow. J. Propul. 6 (6), 777–783.Google Scholar
Wu, J. M., Vakili, A. D. & Yu, F. M. 1988 Investigation of the interacting flow of nonsymmetric jets in crossflow. AIAA J. 26 (8), 940–947.Google Scholar