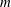Hostname: page-component-586b7cd67f-dsjbd Total loading time: 0 Render date: 2024-11-23T12:45:16.265Z Has data issue: false hasContentIssue false
  • English
  • Français

Three-dimensional quasi-geostrophic vortex equilibria with $m$-fold symmetry

Published online by Cambridge University Press:  22 January 2019

Jean N. Reinaud Open the ORCID record for Jean N. Reinaud [Opens in a new window]
Jean N. Reinaud*
Affiliation:
Mathematical Institute, University of St Andrews, North Haugh, St Andrews KY16 9SS, UK
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We investigate arrays of 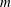$m$ three-dimensional, unit-Burger-number, quasi-geostrophic vortices in mutual equilibrium whose centroids lie on a horizontal circular ring; or$m+1$ vortices where the additional vortex lies on the vertical ‘central’ axis passing through the centre of the array. We first analyse the linear stability of circular point vortex arrays. Three distinct categories of vortex arrays are considered. In the first category, the 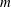$m$ identical point vortices are equally spaced on a circular ring and no vortex is located on the vertical central axis. In the other two categories, a ‘central’ vortex is added. The latter two categories differ by the sign of the central vortex. We next turn our attention to finite-volume vortices for the same three categories. The vortices consist of finite volumes of uniform potential vorticity, and the equilibrium vortex arrays have an (imposed) 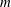$m$-fold symmetry. For simplicity, all vortices have the same volume and the same potential vorticity, in absolute value. For such finite-volume vortex arrays, we determine families of equilibria which are spanned by the ratio of a distance separating the vortices and the array centre to the vortices’ mean radius. We determine numerically the shape of the equilibria for 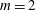$m=2$ up to 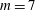$m=7$, for each three categories, and we address their linear stability. For the $m$-vortex circular arrays, all configurations with $m\geqslant 6$ are unstable. Point vortex arrays are linearly stable for 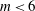$m<6$. Finite-volume vortices may, however, be sensitive to instabilities deforming the vortices for 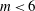$m<6$ if the ratio of the distance separating the vortices to their mean radius is smaller than a threshold depending on 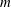$m$. Adding a vortex on the central axis modifies the overall stability properties of the vortex arrays. For 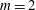$m=2$, a central vortex tends to destabilise the vortex array unless the central vortex has opposite sign and is intense. For 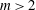$m>2$, the unstable regime can be obtained if the strength of the central vortex is larger in magnitude than a threshold depending on the number of vortices. This is true whether the central vortex has the same sign as or the opposite sign to the peripheral vortices. A moderate-strength like-signed central vortex tends, however, to stabilise the vortex array when located near the plane containing the array. On the contrary, most of the vortex arrays with an opposite-signed central vortex are unstable.

JFM classification

Geophysical and Geological Flows: Quasi-geostrophic flows Vortex Flows: Vortex instability Vortex Flows: Vortex interactions
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 863 , 25 March 2019 , pp. 32 - 59
Copyright
© 2019 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Adriani, A., Mura, A., Orton, G., Hansen, C., Altieri, F., Moriconi, M. L., Rogers, J., Eichstdt, G., Momary, T., Ingersoll, A. P. et al. 2018 Clusters of cyclones encircling Jupiter’s poles. Nature 555, 216–219.10.1038/nature25491Google Scholar
Aref, H. 2009 Stability of relative equilibria of three vortices. Phys. Fluids 21, 094101.10.1063/1.3216063Google Scholar
Burbea, J. 1982 On patches of uniform vorticity in a plane of irrotational flow. Arch. Rat. Mech. Anal. 77, 349–358.10.1007/BF00280642Google Scholar
Carnevale, G. F. & Kloosterziel, R. .C. 1994 Emergence and evolution of triangular vortices. J. Fluid Mech. 259, 305–331.10.1017/S0022112094000157Google Scholar
Chelton, D. B., Schlax, M. G. & Samelson, R. M. 2011 Global observations of nonlinear mesoscale eddies. Prog. Oceanogr. 91, 161–216.10.1016/j.pocean.2011.01.002Google Scholar
Crowdy, D. G. 2002 Exact solutions for rotating vortex arrays with finite-area cores. J. Fluid Mech. 469, 209–235.10.1017/S0022112002001817Google Scholar
Crowdy, D. G. 2003 Polygonal n-vortex arrays: a Stuart model. Phys. Fluids 15 (12), 3710–3717.10.1063/1.1623766Google Scholar
Dijkstra, H. A. 2008 Dynamical Oceanography. Springer.Google Scholar
Dritschel, D. G. 1985 The stability and energetics of corotating uniform vortices. J. Fluid Mech. 157, 95–134.10.1017/S0022112085002324Google Scholar
Dritschel, D. G. 1988 Contour surgery: a topological reconnection scheme for extended integrations using contour dynamics. J. Comput. Phys. 77, 240–266.10.1016/0021-9991(88)90165-9Google Scholar
Dritschel, D. G. 1995 A general theory for two-dimensional vortex interactions. J. Fluid Mech. 293, 269–303.10.1017/S0022112095001716Google Scholar
Dritschel, D. G. 2002 Vortex merger in rotating stratified flows. J. Fluid Mech. 455, 83–101.10.1017/S0022112001007364Google Scholar
Dritschel, D. G. & Saravanan, R. 1994 Three-dimensional quasi-geostrophic contour dynamics, with an application to stratospheric vortex dynamics. Q. J. R. Meteorol. Soc. 120, 1267–1297.10.1002/qj.49712051908Google Scholar
Ebbesmeyer, C. C., Taft, B. A., McWilliams, J. C., Shen, C. Y., Riser, S. C., Rossby, H. T., Biscaye, P. E. & Östlund, H. G. 1986 Detection, structure and origin of extreme anomalies in a western Atlantic oceanographic section. J. Phys. Oceanogr. 16, 591–612.10.1175/1520-0485(1986)016<0591:DSAOOE>2.0.CO;22.0.CO;2>Google Scholar
Gryanik, V. M. 1983 Dynamics of localized vortex perturbations on vortex charges in a baroclinic fluid. Izv. Atmos. Acean. Phys. 19, 347–352.Google Scholar
Kizner, Z. 2011 Stability of point-vortex multipoles revisited. Phys. Fluids 23, 064104.10.1063/1.3596270Google Scholar
Kizner, Z. 2014 On the stability of two-layer geostrophic point-vortex multipoles. Phys. Fluids 26, 046602.10.1063/1.4870239Google Scholar
Kizner, Z. & Khvoles, R. 2004a The tripole vortex: experimental evidence and explicit solutions. Phys. Rev. E 70 (1), 016307.Google Scholar
Kizner, Z. & Khvoles, R. 2004b Two variations on the theme of Lamb-Chaplygin: supersmooth dipole and rotating multipoles. Regular Chaotic Dyn. 9, 509–518.10.1070/RD2004v009n04ABEH000293Google Scholar
Kizner, Z., Khvoles, R. & McWilliams, J. C. 2007 Rotating multipoles on the f- and 𝛾-planes. Phys. Fluids 19 (1), 016603.10.1063/1.2432915Google Scholar
Kizner, Z., Shteinbuch-Fridman, B., Makarov, V. & Rabinovich, M. 2017 Cycloidal meandering of a mesoscale eddy. Phys. Fluids 29, 086601.10.1063/1.4996772Google Scholar
Kurakin, L. G. & Yudovich, V. I. 2002 The stability of stationary rotation of a regular vortex polygon. Chaos 12 (3), 574–595.10.1063/1.1482175Google Scholar
Morikawa, G. K. & Swenson, E. V. 1971 Interacting motion of rectilinear geostrophic vortices. Phys. Fluids 14 (6), 1058–1073.10.1063/1.1693564Google Scholar
Peterson, M. .R., Williams, S. J., Maltrud, M. E., Hecht, M. W. & Hamann, B. 2013 A three-dimensional eddy census of a high-resolution global ocean simulation. J. Geophys. Res. Oceans 118, 1757–1774.Google Scholar
Pierrehumbert, R. T. 1980 A family of steady, translating vortex pairs with distributed vorticity. J. Fluid Mech. 99, 129–144.10.1017/S0022112080000559Google Scholar
Reinaud, J. N. & Carton, X. 2015 Existence, stability and formation of baroclinic tripoles in quasi-geostrophic flows. J. Fluid Mech. 785, 1–30.10.1017/jfm.2015.614Google Scholar
Reinaud, J. N. & Carton, X. 2016 The interaction between two oppositely travelling, horizontally offset, antisymmetric quasi-geostrophic hetons. J. Fluid Mech. 794, 409–443.10.1017/jfm.2016.171Google Scholar
Reinaud, J. N. & Dritschel, D. G. 2002 The merger of vertically offset quasi-geostrophic vortices. J. Fluid Mech. 469, 297–315.10.1017/S0022112002001854Google Scholar
Reinaud, J. N. & Dritschel, D. G. 2005 The critical merger distance between two co-rotating quasi-geostrophic vortices. J. Fluid Mech. 522, 357–381.10.1017/S0022112004002022Google Scholar
Reinaud, J. N. & Dritschel, D. G. 2009 Destructive interactions between two counter-rotating quasi-geostrophic vortices. J. Fluid Mech. 639, 195–211.10.1017/S0022112009990954Google Scholar
Reinaud, J. N. & Dritschel, D. G. 2018 The merger of geophysical vortices at finite Rossby and Froude number. J. Fluid Mech. 848, 388–410.10.1017/jfm.2018.367Google Scholar
Reinaud, J. N. & Dritschel, D. G. 2019 The stability and nonlinear evolution of quasi-geostrophic toroidal vortices. J. Fluid Mech. 863, 60–78.10.1017/jfm.2018.1013Google Scholar
Reinaud, J. N., Dritschel, D. G. & Koudella, C. R. 2003 The shape of vortices in quasi-geostrophic turbulence. J. Fluid Mech. 474, 175–192.10.1017/S0022112002002719Google Scholar
Reinaud, J. N., Sokolovskiy, M. A. & Carton, X. 2017 Geostrophic tripolar vortices in a two-layer fluid: linear stability and nonlinear evolution of equilibria. Phys. Fluids 29 (3), 036601.10.1063/1.4978806Google Scholar
Safman, P. G. 1992 Vortex Dynamics. Cambridge University Press.Google Scholar
Shteinbuch-Fridman, B., Makarov, V. & Kizner, Z. 2015 Two-layer geostrophic tripoles comprised by patches of uniform potential vorticity. Phys. Fluids 27, 036602.10.1063/1.4916283Google Scholar
Shteinbuch-Fridman, B., Makarov, V. & Kizner, Z. 2017 Transitions and oscillatory regimes in two-layer geostrophic hetons and tripoles. J. Fluid Mech. 810, 535–553.10.1017/jfm.2016.738Google Scholar
Sokolovskiy, M. A. & Verron, J. 2008 On the motion of a + 1 vortices in a two-layer rotating fluid. IUTAM Symposium on Hamiltonian Dynamics, Vortex Structures, Turbulence, IUTAM Bookseries Vol. 6. p. 481. Springer.10.1007/978-1-4020-6744-0_43Google Scholar
Thomson, J. J. 1883 A Treatise of Vortex Rings. MacMillan.Google Scholar
Trieling, R. R., van Heijst, G. J. F. & Kizner, Z. 2010 Laboratory experiments on multipolar vortices in a rotating fluid. Phys. Fluids 22, 094104.10.1063/1.3481797Google Scholar
Vallis, G. K. 2006 Atmospheric and Oceanic Fluid Dynamics: Fundamentals and Large-scale Circulation. Cambridge University Press.10.1017/CBO9780511790447Google Scholar
Wu, H. M., Overman, E. A. II & Zabusky, N. J. 1984 Steady-state solutions of the Euler equations: rotating and translating v-states with limiting cases. Part i. Numerical algorithms and results. J. Comput. Phys. 53 (1), 42–71.10.1016/0021-9991(84)90051-2Google Scholar
Xue, J. J., Johnson, E. R. & McDonald, N. R. 2017 New families of vortex patch equilibria for the two-dimensional Euler equations. Phys. Fluids 29 (12), 123602.10.1063/1.5009536Google Scholar
Zabusky, N. J., Hughes, M. H. & Roberts, K. V. 1979 Contour dynamics for Euler equations in two dimensions. J. Comput. Phys. 30 (1), 96–106.10.1016/0021-9991(79)90089-5Google Scholar
Zhang, Z., Wang, W. & Qiu, B. 2014 Oceanic mass transport by mesoscale eddies. Science 345, 322–324.10.1126/science.1252418Google Scholar