Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Liu, Q.
Gómez, F.
and
Theofilis, V.
2015.
Linear Instability Analysis of Incompressible Flow Over A Cuboid Cavity.
Procedia IUTAM,
Vol. 14,
Issue. ,
p.
511.
Sridhar, V.
Gai, S. L.
and
Kleine, H.
2016.
Oscillatory Characteristics of Shallow Open Cavities in Supersonic Flow.
AIAA Journal,
Vol. 54,
Issue. 11,
p.
3495.
Beresh, Steven J.
Wagner, Justin L.
and
Casper, Katya M.
2016.
Compressibility effects in the shear layer over a rectangular cavity.
Journal of Fluid Mechanics,
Vol. 808,
Issue. ,
p.
116.
Beresh, Steven J.
Wagner, Justin L.
Demauro, Edward P.
Henfling, John
and
Spillers, Russell
2016.
Resonance Characteristics of Transonic Flow over a Rectangular Cavity using Pulse-Burst PIV.
Beresh, Steven J.
Wagner, Justin L.
and
Casper, Katya M.
2016.
Compressibility Effects in the Shear Layer over a Rectangular Cavity.
Liu, Qiong
Gómez, Francisco
and
Theofilis, Vassilis
2016.
Control of flow instabilities in an open aircraft bay model cavity.
Demauro, Edward P.
Beresh, Steven J.
Wagner, Justin L.
Henfling, John
and
Spillers, Russell
2016.
Three-Dimensional Measurement of Edge Effects in Open Cavities of Finite-Span.
Liu, Qiong
Gómez, Francisco
and
Theofilis, Vassilios
2016.
Linear instability analysis of low- incompressible flow over a long rectangular finite-span open cavity.
Journal of Fluid Mechanics,
Vol. 799,
Issue. ,
Demauro, Edward P.
Beresh, Steven J.
Wagner, Justin L.
Henfling, John
and
Spillers, Russell
2016.
Stereoscopic Particle Image Velocimetry measurement of transonic flow over three-dimensional open cavities of complex geometry.
DeMauro, Edward P.
Casper, Katya M.
Beresh, Steven J.
Wagner, Justin L.
Henfling, John
and
Spillers, Russell
2017.
Study of the Flow Within Finite-Span Complex Cavities Using Particle Image Velocimetry and Pressure Sensitive Paint.
Beresh, Steven J.
Wagner, Justin L.
Casper, Katya M.
DeMauro, Edward P.
Henfling, John F.
and
Spillers, Russell W.
2017.
Spatial Distribution of Resonance in the Velocity Field for Transonic Flow over a Rectangular Cavity.
AIAA Journal,
Vol. 55,
Issue. 12,
p.
4203.
Wagner, Justin L.
Casper, Katya M.
Beresh, Steven J.
Demauro, Edward P.
Spillers, Russell
and
Henfling, John
2017.
Effects of Cavity Width on Compressible Resonance Dynamics using Time-Resolved Pressure Sensitive Paint and Particle Image Velocimetry.
Liu, Qiong
Sun, Yiyang
Cattafesta, Louis N.
Ukeiley, Lawrence S.
and
Taira, Kunihiko
2018.
Resolvent Analysis of Compressible Flow over a Long Rectangular Cavity.
Luo, Kunyu
Zhu, Wenqing
Xiao, Zhixiang
Weng, Zhe
Deng, Lidong
Yang, Dangguo
and
Liu, Jun
2018.
Investigation of Spectral Characteristics by Passive Control Methods Past a Supersonic Cavity.
AIAA Journal,
Vol. 56,
Issue. 7,
p.
2669.
Wagner, Justin L.
Beresh, Steven J.
Casper, Katya M.
DeMauro, Edward P.
Lynch, Kyle P.
Spillers, Russell
Henfling, John
and
Spitzer, Seth
2018.
Effects of Cavity Width on Resonance Dynamics using Planform Time-Resolved Particle Image Velocimetry.
Yang, Dang-Guo
Lu, Bo
Cai, Jin-Sheng
Wu, Jun-Qiang
Qu, Kun
and
Liu, Jun
2018.
Investigation on flow oscillation modes and aero-acoustics generation mechanism in cavity.
Modern Physics Letters B,
Vol. 32,
Issue. 12n13,
p.
1840046.
Tian, Jin
Yuan, Guo-qing
and
Hua, Hong-xing
2018.
Flow induced structural vibration and sound radiation of a hydrofoil with a cavity.
Journal of Hydrodynamics,
Vol. 30,
Issue. 6,
p.
1022.
Sun, Yiyang
Liu, Qiong
Cattafesta, Louis N.
Ukeiley, Lawrence S.
and
Taira, Kunihiko
2019.
Effects of Sidewalls and Leading-Edge Blowing on Flows over Long Rectangular Cavities.
AIAA Journal,
Vol. 57,
Issue. 1,
p.
106.
Bacci, David
Saddington, Alistair J.
and
Bray, Derek
2019.
The effect of angle of attack on the aeroacoustic environment within the weapons bay of a generic UCAV.
Aerospace Science and Technology,
Vol. 93,
Issue. ,
p.
105315.
DeMauro, Edward P.
Beresh, Steven J.
Casper, Katya M.
Wagner, Justin L.
Henfling, John F.
and
Spillers, Russell W.
2019.
Volumetric Velocimetry of Complex Geometry Effects on Transonic Flow over Cavities.
AIAA Journal,
Vol. 57,
Issue. 5,
p.
1941.
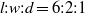 $l{: }w{: }d= 6{: }2{: }1$. Experimental surface pressure data (in air) and particle image velocimetry data (in water) were obtained at low speed with free-stream Reynolds numbers of
$l{: }w{: }d= 6{: }2{: }1$. Experimental surface pressure data (in air) and particle image velocimetry data (in water) were obtained at low speed with free-stream Reynolds numbers of 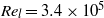 ${\mathit{Re}}_{l} = 3. 4\times 1{0}^{5} $ in air and
${\mathit{Re}}_{l} = 3. 4\times 1{0}^{5} $ in air and 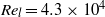 ${\mathit{Re}}_{l} = 4. 3\times 1{0}^{4} $ in water. The experimental results show that the three-dimensional cavity flow is of the ‘open’ type, with an overall flow structure that bears some similarity to the structure observed in nominally two-dimensional cavities, but with a high degree of three-dimensionality both in the flow near the walls and in the unsteady behaviour. The defining features of an open-type cavity flow include a shear layer that traverses the entire cavity opening ultimately impinging on the back surface of the cavity, and a large recirculation zone within the cavity itself. Other flow features that have been identified in the current study include two vortices at the back of the cavity, of which one is barely visible, a weak vortex at the front of the cavity, and a pair of counter-rotating streamwise vortices along the sides of the cavity near the cavity opening. These vortices are generally symmetric about the cavity centre-plane. However, the discovery of a single tornado vortex, located near the cavity centreline at the front of the cavity, indicated that the flow within the cavity is asymmetric. It is postulated that the observed asymmetry in the time-averaged flow field is due to the asymmetry in the instantaneous flow field, which switches between two extremes at large time scales.
${\mathit{Re}}_{l} = 4. 3\times 1{0}^{4} $ in water. The experimental results show that the three-dimensional cavity flow is of the ‘open’ type, with an overall flow structure that bears some similarity to the structure observed in nominally two-dimensional cavities, but with a high degree of three-dimensionality both in the flow near the walls and in the unsteady behaviour. The defining features of an open-type cavity flow include a shear layer that traverses the entire cavity opening ultimately impinging on the back surface of the cavity, and a large recirculation zone within the cavity itself. Other flow features that have been identified in the current study include two vortices at the back of the cavity, of which one is barely visible, a weak vortex at the front of the cavity, and a pair of counter-rotating streamwise vortices along the sides of the cavity near the cavity opening. These vortices are generally symmetric about the cavity centre-plane. However, the discovery of a single tornado vortex, located near the cavity centreline at the front of the cavity, indicated that the flow within the cavity is asymmetric. It is postulated that the observed asymmetry in the time-averaged flow field is due to the asymmetry in the instantaneous flow field, which switches between two extremes at large time scales.