Hostname: page-component-586b7cd67f-t7czq Total loading time: 0 Render date: 2024-11-28T23:05:36.580Z Has data issue: false hasContentIssue false
  • English
  • Français

Taylor–Couette turbulence at radius ratio ${\it\eta}=0.5$: scaling, flow structures and plumes

Published online by Cambridge University Press:  23 June 2016

Roeland C. A. van der Veen ,
Sander G. Huisman ,
Sebastian Merbold ,
Uwe Harlander ,
Christoph Egbers ,
Detlef Lohse  and
Chao Sun
Roeland C. A. van der Veen
Affiliation:
Physics of Fluids Group, MESA+ Institute and J.M. Burgers Centre for Fluid Dynamics, University of Twente, P.O. Box 217, 7500AE Enschede, The Netherlands
Sander G. Huisman
Affiliation:
Physics of Fluids Group, MESA+ Institute and J.M. Burgers Centre for Fluid Dynamics, University of Twente, P.O. Box 217, 7500AE Enschede, The Netherlands
Sebastian Merbold
Affiliation:
Department of Aerodynamics and Fluid Mechanics, Brandenburg University of Technology Cottbus-Senftenberg, Siemens-Halske-Ring 14, 03046 Cottbus, Germany
Uwe Harlander
Affiliation:
Department of Aerodynamics and Fluid Mechanics, Brandenburg University of Technology Cottbus-Senftenberg, Siemens-Halske-Ring 14, 03046 Cottbus, Germany
Christoph Egbers
Affiliation:
Department of Aerodynamics and Fluid Mechanics, Brandenburg University of Technology Cottbus-Senftenberg, Siemens-Halske-Ring 14, 03046 Cottbus, Germany
Detlef Lohse
Affiliation:
Physics of Fluids Group, MESA+ Institute and J.M. Burgers Centre for Fluid Dynamics, University of Twente, P.O. Box 217, 7500AE Enschede, The Netherlands Max Planck Institute for Dynamics and Self-Organization, Am Fassberg 17, 37077 Göttingen, Germany
Chao Sun*
Affiliation:
Physics of Fluids Group, MESA+ Institute and J.M. Burgers Centre for Fluid Dynamics, University of Twente, P.O. Box 217, 7500AE Enschede, The Netherlands Center for Combustion Energy and Department of Thermal Engineering, Tsinghua University, Beijing 100084, PR China
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

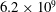Using high-resolution particle image velocimetry, we measure velocity profiles, the wind Reynolds number and characteristics of turbulent plumes in Taylor–Couette flow for a radius ratio of 0.5 and Taylor number of up to $6.2\times 10^{9}$. The extracted angular velocity profiles follow a log law more closely than the azimuthal velocity profiles due to the strong curvature of this 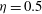${\it\eta}=0.5$ set-up. The scaling of the wind Reynolds number with the Taylor number agrees with the theoretically predicted 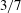$3/7$ scaling for the classical turbulent regime, which is much more pronounced than for the well-explored 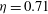${\it\eta}=0.71$ case, for which the ultimate regime sets in at much lower Taylor number. By measuring at varying axial positions, roll structures are found for counter-rotation while no clear coherent structures are seen for pure inner cylinder rotation. In addition, turbulent plumes coming from the inner and outer cylinders are investigated. For pure inner cylinder rotation, the plumes in the radial velocity move away from the inner cylinder, while the plumes in the azimuthal velocity mainly move away from the outer cylinder. For counter-rotation, the mean radial flow in the roll structures strongly affects the direction and intensity of the turbulent plumes. Furthermore, it is experimentally confirmed that, in regions where plumes are emitted, boundary layer profiles with a logarithmic signature are created.

JFM classification

Turbulent Flows: Turbulent boundary layers Turbulent Flows: Turbulent Flows
Type
Papers
Information
Journal of Fluid Mechanics , Volume 799 , 25 July 2016 , pp. 334 - 351
Check for updates
Copyright
© 2016 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ahlers, G., Bodenschatz, E. & He, X. 2014 Logarithmic temperature profiles of turbulent Rayleigh–Bénard convection in the classical and ultimate state for a Prandtl number of 0.8. J. Fluid Mech. 758, 436–467.CrossRefGoogle Scholar
Andereck, C. D., Liu, S. S. & Swinney, H. L. 1986 Flow regimes in a circular Couette system with independently rotating cylinders. J. Fluid Mech. 164, 155–183.Google Scholar
Brauckmann, H. J. & Eckhardt, B. 2013 Intermittent boundary layers and torque maxima in Taylor–Couette flow. Phys. Rev. E 87, 033004.Google Scholar
Brauckmann, H. J., Salewski, M. & Eckhardt, B. 2016 Momentum transport in Taylor–Couette flow with vanishing curvature. J. Fluid Mech. 790, 419–452.CrossRefGoogle Scholar
Chouippe, A., Climent, E., Legendre, D. & Gabillet, C. 2014 Numerical simulation of bubble dispersion in turbulent Taylor–Couette flow. Phys. Fluids 26 (4), 043304.CrossRefGoogle Scholar
Couette, M. 1890 Études sur le frottement des liquides. Ann. Chim. Phys. 21, 433.Google Scholar
Dong, S. 2007 Direct numerical simulation of turbulent Taylor–Couette flow. J. Fluid Mech. 587, 373–393.Google Scholar
Donnelly, R. J. 1991 Taylor–Couette flow: the early days. Phys. Today 44 (11), 32–39.Google Scholar
Dubrulle, B., Dauchot, O., Daviaud, F., Longgaretti, P. Y., Richard, D. & Zahn, J. P. 2005 Stability and turbulent transport in Taylor–Couette flow from analysis of experimental data. Phys. Fluids 17, 095103.Google Scholar
Eckhardt, B., Grossmann, S. & Lohse, D. 2007 Torque scaling in turbulent Taylor–Couette flow between independently rotating cylinders. J. Fluid Mech. 581, 221–250.CrossRefGoogle Scholar
Fardin, M. A., Perge, C. & Taberlet, N. 2014 ‘The hydrogen atom of fluid dynamics’ – Introduction to the Taylor–Couette flow for soft matter scientists. Soft Matt. 10, 3523–3535.Google Scholar
Fenstermacher, P. R., Swinney, H. L. & Gollub, J. P. 1979 Dynamical instabilities and the transition to chaotic Taylor vortex flow. J. Fluid Mech. 94, 103–128.Google Scholar
van Gils, D. P. M., Huisman, S. G., Grossmann, S., Sun, C. & Lohse, D. 2012 Optimal Taylor–Couette turbulence. J. Fluid Mech. 706, 118–149.Google Scholar
Grossmann, S. & Lohse, D. 2000 Scaling in thermal convection: a unifying theory. J. Fluid Mech. 407, 27–56.Google Scholar
Grossmann, S. & Lohse, D. 2011 Multiple scaling in the ultimate regime of thermal convection. Phys. Fluids 23, 045108.CrossRefGoogle Scholar
Grossmann, S., Lohse, D. & Sun, C. 2014 Velocity profiles in strongly turbulent Taylor–Couette flow. Phys. Fluids 26, 025114.Google Scholar
Grossmann, S., Lohse, D. & Sun, C. 2016 High Reynolds number Taylor–Couette turbulence. Annu. Rev. Fluid Mech. 48, 53–80.Google Scholar
He, X., Funfschilling, D., Nobach, H., Bodenschatz, E. & Ahlers, G. 2012 Transition to the ultimate state of turbulent Rayleigh–Bénard convection. Phys. Rev. Lett. 108, 024502.Google Scholar
van Hout, R. & Katz, J. 2011 Measurements of mean flow and turbulence characteristics in high-Reynolds number counter-rotating Taylor–Couette flow. Phys. Fluids 23 (10), 105102.CrossRefGoogle Scholar
Huisman, S. G., van Gils, D. P. M., Grossmann, S., Sun, C. & Lohse, D. 2012 Ultimate turbulent Taylor–Couette flow. Phys. Rev. Lett. 108, 024501.Google Scholar
Huisman, S. G., Scharnowski, S., Cierpka, C., Kähler, C., Lohse, D. & Sun, C. 2013 Logarithmic boundary layers in strong Taylor–Couette turbulence. Phys. Rev. Lett. 110, 264501.Google Scholar
Huisman, S. G., van der Veen, R. C. A., Sun, C. & Lohse, D. 2014 Multiple states in highly turbulent Taylor–Couette flow. Nat. Commun. 5, 3820.CrossRefGoogle ScholarPubMed
Ji, H., Burin, M., Schartman, E. & Goodman, J. 2006 Hydrodynamic turbulence cannot transport angular momentum effectively in astrophysical disks. Nature 444, 343–346.Google Scholar
Kiefer, J. 1953 Sequential minimax search for a maximum. Proc. Am. Math. Soc. 4, 502–506.Google Scholar
Kraichnan, R. H. 1962 Turbulent thermal convection at arbritrary Prandtl number. Phys. Fluids 5, 1374–1389.Google Scholar
Lakkaraju, R., Stevens, R. J. A. M., Verzicco, R., Grossmann, S., Prosperetti, A., Sun, C. & Lohse, D. 2012 Spatial distribution of heat flux and fluctuations in turbulent Rayleigh–Bénard convection. Phys. Rev. E 86, 056315.Google Scholar
Lathrop, D. P., Fineberg, J. & Swinney, H. S. 1992 Turbulent flow between concentric rotating cylinders at large Reynolds numbers. Phys. Rev. Lett. 68, 1515–1518.Google Scholar
Lewis, G. S. & Swinney, H. L. 1999 Velocity structure functions, scaling, and transitions in high-Reynolds-number Couette–Taylor flow. Phys. Rev. E 59, 5457–5467.Google Scholar
López-Caballero, M. & Burguete, J. 2013 Inverse cascades sustained by the transfer rate of angular momentum in a 3D turbulent flow. Phys. Rev. Lett. 110, 124501.Google Scholar
Mallock, A. 1896 Experiments on fluid viscosity. Phil. Trans. R. Soc. Lond. A 187, 41–56.Google Scholar
Marusic, I., Monty, J. P., Hultmark, M. & Smits, A. J. 2013 On the logarithmic region in wall turbulence. J. Fluid Mech. 716, R3.Google Scholar
Merbold, S., Brauckmann, H. J. & Egbers, C. 2013 Torque measurements and numerical determination in differentially rotating wide gap Taylor–Couette flow. Phys. Rev. E 87, 023014.Google Scholar
Merbold, S., Fischer, S. & Egbers, C. 2011 Torque scaling in Taylor–Couette flow – an experimental investigation. J. Phys.: Conf. Ser. 318 (8), 082017.Google Scholar
Ostilla-Mónico, R., Huisman, S. G., Jannink, T. J. G., van Gils, D. P. M., Verzicco, R., Grossmann, S., Sun, C. & Lohse, D. 2014a Optimal Taylor–Couette flow: radius ratio dependence. J. Fluid Mech. 747, 1–29.Google Scholar
Ostilla-Mónico, R., van der Poel, E. P., Verzicco, R., Grossmann, S. & Lohse, D. 2014b Boundary layer dynamics at the transition between the classical and the ultimate regime of Taylor–Couette flow. Phys. Fluids 26 (1), 015114.Google Scholar
Ostilla-Mónico, R., van der Poel, E. P., Verzicco, R., Grossmann, S. & Lohse, D. 2014c Exploring the phase diagram of fully turbulent Taylor–Couette flow. J. Fluid Mech. 761, 1–26.CrossRefGoogle Scholar
Ostilla-Mónico, R., Verzicco, R., Grossmann, S. & Lohse, D. 2016 The near-wall region of highly turbulent Taylor–Couette flow. J. Fluid Mech. 788, 95–117.Google Scholar
Paoletti, M. S., van Gils, D. P. M., Dubrulle, B., Sun, C., Lohse, D. & Lathrop, D. P. 2012 Angular momentum transport and turbulence in laboratory models of Keplerian flows. Astron. Astrophys. 547, A64.Google Scholar
van der Poel, E. P., Ostilla-Mónico, R., Verzicco, R., Grossmann, S. & Lohse, D. 2015 Logarithmic mean temperature profiles and their connection to plume emissions in turbulent Rayleigh–Bénard convection. Phys. Rev. Lett. 115, 154501.Google Scholar
Ravelet, F., Delfos, R. & Westerweel, J. 2010 Influence of global rotation and Reynolds number on the large-scale features of a turbulent Taylor–Couette flow. Phys. Fluids 22 (5), 055103.Google Scholar
Shang, X.-D., Tong, P. & Xia, K.-Q. 2008 Scaling of the local convective heat flux in turbulent Rayleigh–Bénard convection. Phys. Rev. Lett. 100, 244503.CrossRefGoogle ScholarPubMed
Taylor, G. I. 1923 Experiments on the motion of solid bodies in rotating fluids. Proc. R. Soc. Lond. A 104, 213–218.Google Scholar
Tokgoz, S., Elsinga, G. E., Delfos, R. & Westerweel, J. 2011 Experimental investigation of torque scaling and coherent structures in turbulent Taylor–Couette flow. J. Phys.: Conf. Ser. 318 (8), 082018.Google Scholar
van der Veen, R. C. A., Huisman, S. G., Dung, O.-Y., Tang, H. L., Sun, C. & Lohse, D. 2016 Exploring the phase space of multiple states in highly turbulent Taylor–Couette flow. Phys. Rev. Fluids 1, 024401.CrossRefGoogle Scholar
Wendt, F. 1933 Turbulente Strömungen zwischen zwei rotierenden konaxialen Zylindern. Ing.-Arch. 4, 577–595.Google Scholar