Published online by Cambridge University Press: 19 July 2018
We study the turbulent square duct flow of dense suspensions of neutrally buoyant spherical particles. Direct numerical simulations (DNS) are performed in the range of volume fractions 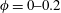 $\unicode[STIX]{x1D719}=0{-}0.2$, using the immersed boundary method (IBM) to account for the dispersed phase. Based on the hydraulic diameter a Reynolds number of 5600 is considered. We observe that for
$\unicode[STIX]{x1D719}=0{-}0.2$, using the immersed boundary method (IBM) to account for the dispersed phase. Based on the hydraulic diameter a Reynolds number of 5600 is considered. We observe that for 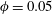 $\unicode[STIX]{x1D719}=0.05$ and 0.1, particles preferentially accumulate on the corner bisectors, close to the corners, as also observed for laminar square duct flows of the same duct-to-particle size ratio. At the highest volume fraction, particles preferentially accumulate in the core region. For plane channel flows, in the absence of lateral confinement, particles are found instead to be uniformly distributed across the channel. The intensity of the cross-stream secondary flows increases (with respect to the unladen case) with the volume fraction up to
$\unicode[STIX]{x1D719}=0.05$ and 0.1, particles preferentially accumulate on the corner bisectors, close to the corners, as also observed for laminar square duct flows of the same duct-to-particle size ratio. At the highest volume fraction, particles preferentially accumulate in the core region. For plane channel flows, in the absence of lateral confinement, particles are found instead to be uniformly distributed across the channel. The intensity of the cross-stream secondary flows increases (with respect to the unladen case) with the volume fraction up to  $\unicode[STIX]{x1D719}=0.1$, as a consequence of the high concentration of particles along the corner bisector. For
$\unicode[STIX]{x1D719}=0.1$, as a consequence of the high concentration of particles along the corner bisector. For 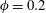 $\unicode[STIX]{x1D719}=0.2$ the turbulence activity is reduced and the intensity of the secondary flows reduces to below that of the unladen case. The friction Reynolds number increases with
$\unicode[STIX]{x1D719}=0.2$ the turbulence activity is reduced and the intensity of the secondary flows reduces to below that of the unladen case. The friction Reynolds number increases with  $\unicode[STIX]{x1D719}$ in dilute conditions, as observed for channel flows. However, for
$\unicode[STIX]{x1D719}$ in dilute conditions, as observed for channel flows. However, for 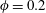 $\unicode[STIX]{x1D719}=0.2$ the mean friction Reynolds number is similar to that for
$\unicode[STIX]{x1D719}=0.2$ the mean friction Reynolds number is similar to that for 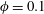 $\unicode[STIX]{x1D719}=0.1$. By performing the turbulent kinetic energy budget, we see that the turbulence production is enhanced up to
$\unicode[STIX]{x1D719}=0.1$. By performing the turbulent kinetic energy budget, we see that the turbulence production is enhanced up to 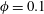 $\unicode[STIX]{x1D719}=0.1$, while for
$\unicode[STIX]{x1D719}=0.1$, while for 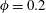 $\unicode[STIX]{x1D719}=0.2$ the production decreases below the values for
$\unicode[STIX]{x1D719}=0.2$ the production decreases below the values for 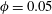 $\unicode[STIX]{x1D719}=0.05$. On the other hand, the dissipation and the transport monotonically increase with
$\unicode[STIX]{x1D719}=0.05$. On the other hand, the dissipation and the transport monotonically increase with  $\unicode[STIX]{x1D719}$. The interphase interaction term also contributes positively to the turbulent kinetic energy budget and increases monotonically with
$\unicode[STIX]{x1D719}$. The interphase interaction term also contributes positively to the turbulent kinetic energy budget and increases monotonically with 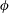 $\unicode[STIX]{x1D719}$, in a similar way as the mean transport. Finally, we show that particles move on average faster than the fluid. However, there are regions close to the walls and at the corners where they lag behind it. In particular, for
$\unicode[STIX]{x1D719}$, in a similar way as the mean transport. Finally, we show that particles move on average faster than the fluid. However, there are regions close to the walls and at the corners where they lag behind it. In particular, for  $\unicode[STIX]{x1D719}=0.05,0.1$, the slip velocity distribution at the corner bisectors seems correlated to the locations of maximum concentration: the concentration is higher where the slip velocity vanishes. The wall-normal hydrodynamic and collision forces acting on the particles push them away from the corners. The combination of these forces vanishes around the locations of maximum concentration. The total mean forces are generally low along the corner bisectors and at the core, also explaining the concentration distribution for
$\unicode[STIX]{x1D719}=0.05,0.1$, the slip velocity distribution at the corner bisectors seems correlated to the locations of maximum concentration: the concentration is higher where the slip velocity vanishes. The wall-normal hydrodynamic and collision forces acting on the particles push them away from the corners. The combination of these forces vanishes around the locations of maximum concentration. The total mean forces are generally low along the corner bisectors and at the core, also explaining the concentration distribution for 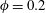 $\unicode[STIX]{x1D719}=0.2$.
$\unicode[STIX]{x1D719}=0.2$.