Article contents
Streamwise dispersion and mixing in quasi-two-dimensional steady turbulent jets
Published online by Cambridge University Press: 12 September 2012
Abstract
We investigate experimentally and theoretically the streamwise transport and dispersion properties of steady quasi-two-dimensional plane turbulent jets discharged vertically from a slot of width 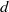 into a fluid confined between two relatively close rigid boundaries with gap
into a fluid confined between two relatively close rigid boundaries with gap 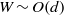 . We model the evolution in time and space of the concentration of passive tracers released in these jets using a one-dimensional time-dependent effective advection–diffusion equation. We make a mixing length hypothesis to model the streamwise turbulent eddy diffusivity such that it scales like
. We model the evolution in time and space of the concentration of passive tracers released in these jets using a one-dimensional time-dependent effective advection–diffusion equation. We make a mixing length hypothesis to model the streamwise turbulent eddy diffusivity such that it scales like 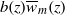 , where
, where  is the streamwise coordinate,
is the streamwise coordinate, 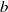 is the jet width,
is the jet width, 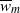 is the maximum time-averaged vertical velocity. Under these assumptions, the effective advection–diffusion equation for
is the maximum time-averaged vertical velocity. Under these assumptions, the effective advection–diffusion equation for 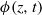 , the horizontal integral of the ensemble-averaged concentration, is of the form
, the horizontal integral of the ensemble-averaged concentration, is of the form  , where
, where  is time,
is time, 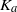 (the advection parameter) and
(the advection parameter) and 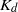 (the dispersion parameter) are empirical dimensionless parameters which quantify the importance of advection and dispersion, respectively, and
(the dispersion parameter) are empirical dimensionless parameters which quantify the importance of advection and dispersion, respectively, and 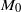 is the source momentum flux. We find analytical solutions to this equation for
is the source momentum flux. We find analytical solutions to this equation for 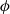 in the cases of a constant-flux release and an instantaneous finite-volume release. We also give an integral formulation for the more general case of a time-dependent release, which we solve analytically when tracers are released at a constant flux over a finite period of time. From our experimental results, whose concentration distributions agree with the model, we find that
in the cases of a constant-flux release and an instantaneous finite-volume release. We also give an integral formulation for the more general case of a time-dependent release, which we solve analytically when tracers are released at a constant flux over a finite period of time. From our experimental results, whose concentration distributions agree with the model, we find that  and
and  , for both finite-volume releases and constant-flux releases using either dye or virtual passive tracers. The experiments also show that streamwise dispersion increases in time as
, for both finite-volume releases and constant-flux releases using either dye or virtual passive tracers. The experiments also show that streamwise dispersion increases in time as 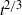 . As a result, in the case of finite-volume releases more than 50 % of the total volume of tracers is transported ahead of the purely advective front (i.e. the front location of the tracer distribution if all dispersion mechanisms are ignored and considering a ‘top-hat’ mean velocity profile in the jet); and in the case of constant-flux releases, at each instant in time, approximately 10 % of the total volume of tracers is transported ahead of the advective front.
. As a result, in the case of finite-volume releases more than 50 % of the total volume of tracers is transported ahead of the purely advective front (i.e. the front location of the tracer distribution if all dispersion mechanisms are ignored and considering a ‘top-hat’ mean velocity profile in the jet); and in the case of constant-flux releases, at each instant in time, approximately 10 % of the total volume of tracers is transported ahead of the advective front.
JFM classification
- Type
- Papers
- Information
- Copyright
- Copyright © Cambridge University Press 2012
References
- 15
- Cited by


