Hostname: page-component-cd9895bd7-7cvxr Total loading time: 0 Render date: 2024-12-27T03:39:37.752Z Has data issue: false hasContentIssue false
  • English
  • Français

Stratified turbulence forced with columnar dipoles: numerical study

Published online by Cambridge University Press:  25 March 2015

Pierre Augier ,
Paul Billant  and
Jean-Marc Chomaz
Pierre Augier*
Affiliation:
LadHyX, CNRS, Ecole Polytechnique, 91128 Palaiseau CEDEX, France LEGI, CNRS, Université Grenoble Alpes, 38041 Grenoble CEDEX 9, France
Paul Billant
Affiliation:
LadHyX, CNRS, Ecole Polytechnique, 91128 Palaiseau CEDEX, France
Jean-Marc Chomaz
Affiliation:
LadHyX, CNRS, Ecole Polytechnique, 91128 Palaiseau CEDEX, France
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

This paper builds upon the investigation of Augier et al. (Phys. Fluids, vol. 26 (4), 2014) in which a strongly stratified turbulent-like flow was forced by 12 generators of vertical columnar dipoles. In experiments, measurements start to provide evidence of the existence of a strongly stratified inertial range that has been predicted for large turbulent buoyancy Reynolds numbers 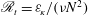$\mathscr{R}_{t}={\it\varepsilon}_{\!K}/({\it\nu}N^{2})$, where 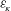${\it\varepsilon}_{\!K}$ is the mean dissipation rate of kinetic energy, ${\it\nu}$ the viscosity and 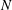$N$ the Brunt–Väisälä frequency. However, because of experimental constraints, the buoyancy Reynolds number could not be increased to sufficiently large values so that the inertial strongly stratified turbulent range is only incipient. In order to extend the experimental results toward higher buoyancy Reynolds number, we have performed numerical simulations of forced stratified flows. To reproduce the experimental vortex generators, columnar dipoles are periodically produced in spatial space using impulsive horizontal body force at the peripheries of the computational domain. For moderate buoyancy Reynolds number, these numerical simulations are able to reproduce the results obtained in the experiments, validating this particular forcing. For higher buoyancy Reynolds number, the simulations show that the flow becomes turbulent as observed in Brethouwer et al. (J. Fluid Mech., vol. 585, 2007, pp. 343–368). However, the statistically stationary flow is horizontally inhomogeneous because the dipoles are destabilized quite rapidly after their generation. In order to produce horizontally homogeneous turbulence, high-resolution simulations at high buoyancy Reynolds number have been carried out with a slightly modified forcing in which dipoles are forced at random locations in the computational domain. The unidimensional horizontal spectra of kinetic and potential energies scale like 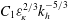$C_{1}{\it\varepsilon}_{\!K}^{2/3}k_{h}^{-5/3}$ and 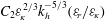$C_{2}{\it\varepsilon}_{\!K}^{2/3}k_{h}^{-5/3}({\it\varepsilon}_{\!P}/{\it\varepsilon}_{\!K})$, respectively, with 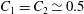$C_{1}=C_{2}\simeq 0.5$ as obtained by Lindborg (J. Fluid Mech., vol. 550, 2006, pp. 207–242). However, there is a depletion in the horizontal kinetic energy spectrum for scales between the integral length scale and the buoyancy length scale and an anomalous energy excess around the buoyancy length scale probably due to direct transfers from large horizontal scale to small scales resulting from the shear and gravitational instabilities. The horizontal buoyancy flux co-spectrum increases abruptly at the buoyancy scale corroborating the presence of overturnings. Remarkably, the vertical kinetic energy spectrum exhibits a transition at the Ozmidov length scale from a steep spectrum scaling like 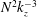$N^{2}k_{z}^{-3}$ at large scales to a spectrum scaling like $C_{K}{\it\varepsilon}_{\!K}^{2/3}k_{z}^{-5/3}$, with 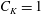$C_{K}=1$, the classical Kolmogorov constant.

JFM classification

Geophysical and Geological Flows: Geophysical and Geological Flows Turbulent Flows: Stratified turbulence Turbulent Flows: Turbulence simulation
Type
Papers
Information
Journal of Fluid Mechanics , Volume 769 , 25 April 2015 , pp. 403 - 443
Copyright
© 2015 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Alisse, J. R. & Sidi, C. 2000 Experimental probability density functions of small-scale fluctuations in the stably stratified atmosphere. J. Fluid Mech. 402, 137–162.CrossRefGoogle Scholar
Almalkie, S. & de Bruyn Kops, S. M. 2012 Kinetic energy dynamics in forced, homogeneous, and axisymmetric stably stratified turbulence. J. Turbul. 13 (29), 1–32.Google Scholar
Alvelius, K. 1999 Random forcing of three-dimensional homogeneous turbulence. Phys. Fluids 11, 1880–1889.CrossRefGoogle Scholar
Augier, P. & Billant, P. 2011 Onset of secondary instabilities on the zigzag instability in stratified fluids. J. Fluid Mech. 662, 120–131.CrossRefGoogle Scholar
Augier, P., Billant, P., Negretti, M. E. & Chomaz, J.-M. 2014 Experimental study of stratified turbulence forced with columnar dipoles. Phys. Fluids 26 (4).Google Scholar
Augier, P., Chomaz, J.-M. & Billant, P. 2012 Spectral analysis of the transition to turbulence from a dipole in stratified fluids. J. Fluid Mech. 713, 86–108.CrossRefGoogle Scholar
Augier, P. & Lindborg, E. 2013 A new formulation of the spectral energy budget of the atmosphere, with application to two high-resolution general circulation models. J. Atmos. Sci. 70, 2293–2308.CrossRefGoogle Scholar
Bartello, P. & Tobias, S. M. 2013 Sensitivity of stratified turbulence to the buoyancy Reynolds number. J. Fluid Mech. 725, 1–22.CrossRefGoogle Scholar
Billant, P. & Chomaz, J.-M. 2001 Self-similarity of strongly stratified inviscid flows. Phys. Fluids 13, 1645–1651.Google Scholar
Brethouwer, G., Billant, P., Lindborg, E. & Chomaz, J.-M. 2007 Scaling analysis and simulation of strongly stratified turbulent flows. J. Fluid Mech. 585, 343–368.Google Scholar
Cambon, C. 2001 Turbulence and vortex structures in rotating and stratified flows. Eur. J. Mech. (B/Fluids) 20, 489–510.Google Scholar
Carnevale, G. F., Briscolini, M. & Orlandi, P. 2001 Buoyancy- to inertial-range transition in forced stratified turbulence. J. Fluid Mech. 427, 205–239.Google Scholar
Cho, J. Y. N. & Lindborg, E. 2001 Horizontal velocity structure functions in the upper troposphere and lower stratosphere 1. Observations. J. Geophys. Res. 106 (D10), 10223–10232.CrossRefGoogle Scholar
Craya, A. D.1958 Contribution à l’Analyse de la Turbulence Associée à des Vitesses Moyennes. Ministère de l’air, France PST 345.Google Scholar
Deloncle, A., Billant, P. & Chomaz, J.-M. 2008 Nonlinear evolution of the zigzag instability in stratified fluids: a shortcut on the route to dissipation. J. Fluid Mech. 599, 229–238.CrossRefGoogle Scholar
Dewan, E. 1997 Saturated-cascade similitude theory of gravity wave spectra. J. Geophys. Res. 102 (D25), 29799–29817.CrossRefGoogle Scholar
Dewan, E. M. & Good, R. E. 1986 Saturation and the universal spectrum for vertical profiles of horizontal scalar winds in the atmosphere. J. Geophys. Res. 91 (D2), 2742–2748.CrossRefGoogle Scholar
Fincham, A. M., Maxworthy, T. & Spedding, G. R. 1996 Energy dissipation and vortex structure in freely decaying, stratified grid turbulence. Dyn. Atmos. Oceans 23 (1–4), 155–169.Google Scholar
Gargett, A. E., Hendricks, P. J., Sanford, T. B., Osborn, T. R. & Williams, A. J. 1981 A composite spectrum of vertical shear in the upper ocean. J. Phys. Oceanogr. 11 (9), 1258–1271.2.0.CO;2>CrossRefGoogle Scholar
Garrett, C. & Munk, W. 1979 Internal waves in the ocean. Annu. Rev. Fluid Mech. 11, 339–369.Google Scholar
Godoy-Diana, R., Chomaz, J.-M. & Billant, P. 2004 Vertical length scale selection for pancake vortices in strongly stratified viscous fluids. J. Fluid Mech. 504, 229–238.CrossRefGoogle Scholar
Gotoh, T., Fukayama, D. & Nakano, T. 2002 Velocity field statistics in homogeneous steady turbulence obtained using a high-resolution direct numerical simulation. Phys. Fluids 14 (3), 1065–1081.Google Scholar
Hamilton, K., Takahashi, Y. O. & Ohfuchi, W. 2008 Mesoscale spectrum of atmospheric motions investigated in a very fine resolution global general circulation model. J. Geophys. Res. 113 (D18), 2156–2202.Google Scholar
Hebert, D. A. & de Bruyn Kops, S. M. 2006a Predicting turbulence in flows with strong stable stratification. Phys. Fluids 18 (6), 066602.Google Scholar
Hebert, D. A. & de Bruyn Kops, S. M. 2006b Relationship between vertical shear rate and kinetic energy dissipation rate in stable stratified flows. Geophys. Res. Lett. 18 (6), doi:10.1063/1.2204987.Google Scholar
Herring, J. R. 1974 Approach of axisymmetric turbulence to isotropy. Phys. Fluids 17, 859–872.Google Scholar
Hines, C. O. 1991 The saturation of gravity-waves in the middle atmosphere. 1. Critique of linear-instability theory. J. Atmos. Sci. 48 (11), 1348–1359.2.0.CO;2>CrossRefGoogle Scholar
Holloway, G. 1988 The buoyancy flux from internal gravity wave breaking. Dyn. Atmos. Oceans 12, 107–125.Google Scholar
Howard, L. N. 1961 Note on a paper of John W. Miles. J. Fluid Mech. 10, 509–512.CrossRefGoogle Scholar
Ivey, G. N. & Imberger, J. 1991 On the nature of turbulence in a stratified fluid. Part I: the energetics of mixing. J. Phys. Oceanogr. 21 (5), 650–658.2.0.CO;2>CrossRefGoogle Scholar
Kimura, Y. & Herring, J. R. 2012 Energy spectra of stably stratified turbulence. J. Fluid Mech. 698, 19–50.CrossRefGoogle Scholar
Koshyk, J. N. & Hamilton, K. 2001 The horizontal kinetic energy spectrum and spectral budget simulated by a high-resolution troposphere–stratosphere–mesosphere GCM. J. Atmos. Sci. 58 (4), 329–348.2.0.CO;2>CrossRefGoogle Scholar
Laval, J. P., McWilliams, J. C. & Dubrulle, B. 2003 Forced stratified turbulence: successive transitions with Reynolds number. Phys. Rev. E 68 (3), 036308.Google Scholar
Lesieur, M. 1997 Turbulence in Fluids, 3rd edn. Kluwer Academic.Google Scholar
Lilly, D. K. 1983 Stratified turbulence and the mesoscale variability of the atmosphere. J. Atmos. Sci. 40, 749–761.Google Scholar
Lindborg, E.2002 Strongly stratified turbulence: a special type of motion. In Advances in Turbulence IX, Proceedings of the Ninth European Turbulence Conference. Southampton.Google Scholar
Lindborg, E. 2006 The energy cascade in a strongly stratified fluid. J. Fluid Mech. 550, 207–242.CrossRefGoogle Scholar
Lindborg, E. 2007 Horizontal wavenumber spectra of vertical vorticity and horizontal divergence in the upper troposphere and lower stratosphere. J. Atmos. Sci. 64 (3), 1017–1025.Google Scholar
Lindborg, E. & Brethouwer, G. 2007 Stratified turbulence forced in rotational and divergent modes. J. Fluid Mech. 586, 83–108.CrossRefGoogle Scholar
Lumley, J. L. 1964 The spectrum of nearly inertial turbulence in a stably stratified fluid. J. Atmos. Sci. 21 (1), 99–102.2.0.CO;2>CrossRefGoogle Scholar
Lundbladh, A., Berlin, S., Skote, M., Hildings, C., Choi, J., Kim, J. & Henningson, D. S.1999 An efficient spectral method for simulation of incompressible flow over a flat plate. Trita-mek. Tech. Rep. 11.Google Scholar
Miles, J. W. 1961 On the stability of heterogeneous shear flows. J. Fluid Mech. 10, 496–508.CrossRefGoogle Scholar
Monin, A. S. & Yaglom, A. M. 1975 Statistical Fluid Mechanics vol. 2. MIT Press.Google Scholar
Nastrom, G. D. & Gage, K. S. 1985 A climatology of atmospheric wavenumber spectra of wind and temperature observed by commercial aircraft. J. Atmos. Sci. 42 (9), 950–960.Google Scholar
Nastrom, G. D., Gage, K. S. & Jasperson, W. H. 1984 Kinetic-energy spectrum of largescale and mesoscale atmospheric processes. Nature 310 (5972), 36–38.Google Scholar
Ozmidov, R. V. 1965 On the turbulent exchange in a stably stratified ocean. Izv. Acad. Sci. USSR, Atmos. Ocean. Phys. 1, 493–497.Google Scholar
Praud, O., Fincham, A. M. & Sommeria, J. 2005 Decaying grid turbulence in a strongly stratified fluid. J. Fluid Mech. 522, 1–33.Google Scholar
Riley, J. J. & de Bruyn Kops, S. M. 2003 Dynamics of turbulence strongly influenced by buoyancy. Phys. Fluids 15 (7), 2047–2059.Google Scholar
Riley, J. J. & Lelong, M.-P. 2000 Fluid motions in the presence of strong stable stratification. Annu. Rev. Fluid Mech. 32, 613–657.Google Scholar
Riley, J. J. & Lindborg, E. 2008 Stratified turbulence: a possible interpretation of some geophysical turbulence measurements. J. Atmos. Sci. 65, 2416–2424.CrossRefGoogle Scholar
Riley, J. J., Metcalfe, R. W. & Weissman, M. A. 1981 Direct numerical simulations of homogeneous turbulence in density-stratified fluids. Proc. AIP Conf. 76, 79–112.CrossRefGoogle Scholar
Skamarock, W. C. 2004 Evaluating mesoscale NWP models using kinetic energy spectra. Mon. Weath. Rev. 132 (12), 3019–3032.CrossRefGoogle Scholar
Smith, S. A., Fritts, D. C. & Vanzandt, T. E.  1987 Evidence for a saturated spectrum of atmospheric gravity-waves. J. Atmos. Sci. 44 (10), 1404–1410.2.0.CO;2>CrossRefGoogle Scholar
Smith, L. M. & Waleffe, F. 2002 Generation of slow large scales in forced rotating stratified turbulence. J. Fluid Mech. 451, 145–168.Google Scholar
Sreenivasan, K. R. 1995 On the universality of the Kolmogorov constant. Phys. Fluids 7 (11), 2778–2784.Google Scholar
Sreenivasan, K. R. 1996 The passive scalar spectrum and the Obukhov–Corrsin constant. Phys. Fluids 8 (1), 189–196.CrossRefGoogle Scholar
Staquet, C. & Godeferd, F. S. 1998 Statistical modelling and direct numerical simulations of decaying stably stratified turbulence. Part 1. Flow energetics. J. Fluid Mech. 360, 295–340.CrossRefGoogle Scholar
Waite, M. L. 2011 Stratified turbulence at the buoyancy scale. Phys. Fluids 23 (6), 066602.CrossRefGoogle Scholar
Waite, M. L. & Bartello, P. 2004 Stratified turbulence dominated by vortical motion. J. Fluid Mech. 517, 281–308.CrossRefGoogle Scholar
Waite, M. L. & Bartello, P. 2006 Stratified turbulence generated by internal gravity waves. J. Fluid Mech. 546, 313–339.Google Scholar
Waite, M. L. & Smolarkiewicz, P. K. 2008 Instability and breakdown of a vertical vortex pair in a strongly stratified fluid. J. Fluid Mech. 606, 239–273.Google Scholar