Hostname: page-component-78c5997874-j824f Total loading time: 0 Render date: 2024-11-17T14:02:41.131Z Has data issue: false hasContentIssue false
  • English
  • Français

Stochastic theory and direct numerical simulations of the relative motion of high-inertia particle pairs in isotropic turbulence

Published online by Cambridge University Press:  17 January 2017

Rohit Dhariwal ,
Sarma L. Rani  and
Donald L. Koch
Rohit Dhariwal
Affiliation:
Department of Mechanical and Aerospace Engineering, University of Alabama in Huntsville, Huntsville, AL 35899, USA
Sarma L. Rani*
Affiliation:
Department of Mechanical and Aerospace Engineering, University of Alabama in Huntsville, Huntsville, AL 35899, USA
Donald L. Koch
Affiliation:
School of Chemical and Biomolecular Engineering, Cornell University, Ithaca, NY 14853, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The relative velocities and positions of monodisperse high-inertia particle pairs in isotropic turbulence are studied using direct numerical simulations (DNS), as well as Langevin simulations (LS) based on a probability density function (PDF) kinetic model for pair relative motion. In a prior study (Rani et al., J. Fluid Mech., vol. 756, 2014, pp. 870–902), the authors developed a stochastic theory that involved deriving closures in the limit of high Stokes number for the diffusivity tensor in the PDF equation for monodisperse particle pairs. The diffusivity contained the time integral of the Eulerian two-time correlation of fluid relative velocities seen by pairs that are nearly stationary. The two-time correlation was analytically resolved through the approximation that the temporal change in the fluid relative velocities seen by a pair occurs principally due to the advection of smaller eddies past the pair by large-scale eddies. Accordingly, two diffusivity expressions were obtained based on whether the pair centre of mass remained fixed during flow time scales, or moved in response to integral-scale eddies. In the current study, a quantitative analysis of the (Rani et al. 2014) stochastic theory is performed through a comparison of the pair statistics obtained using LS with those from DNS. LS consist of evolving the Langevin equations for pair separation and relative velocity, which is statistically equivalent to solving the classical Fokker–Planck form of the pair PDF equation. Langevin simulations of particle-pair dispersion were performed using three closure forms of the diffusivity – i.e. the one containing the time integral of the Eulerian two-time correlation of the seen fluid relative velocities and the two analytical diffusivity expressions. In the first closure form, the two-time correlation was computed using DNS of forced isotropic turbulence laden with stationary particles. The two analytical closure forms have the advantage that they can be evaluated using a model for the turbulence energy spectrum that closely matched the DNS spectrum. The three diffusivities are analysed to quantify the effects of the approximations made in deriving them. Pair relative-motion statistics obtained from the three sets of Langevin simulations are compared with the results from the DNS of (moving) particle-laden forced isotropic turbulence for 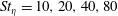$St_{\unicode[STIX]{x1D702}}=10,20,40,80$ and 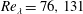$Re_{\unicode[STIX]{x1D706}}=76,131$. Here, $St_{\unicode[STIX]{x1D702}}$ is the particle Stokes number based on the Kolmogorov time scale and 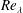$Re_{\unicode[STIX]{x1D706}}$ is the Taylor micro-scale Reynolds number. Statistics such as the radial distribution function (RDF), the variance and kurtosis of particle-pair relative velocities and the particle collision kernel were computed using both Langevin and DNS runs, and compared. The RDFs from the stochastic runs were in good agreement with those from the DNS. Also computed were the PDFs 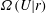$\unicode[STIX]{x1D6FA}(U|r)$ and 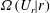$\unicode[STIX]{x1D6FA}(U_{r}|r)$ of relative velocity 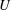$U$ and of the radial component of relative velocity 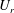$U_{r}$ respectively, both PDFs conditioned on separation $r$. The first closure form, involving the Eulerian two-time correlation of fluid relative velocities, showed the best agreement with the DNS results for the PDFs.

JFM classification

Multiphase and Particle-laden Flows: Multiphase and Particle-laden Flows Multiphase and Particle-laden Flows: Particle/fluid flow
Type
Papers
Information
Journal of Fluid Mechanics , Volume 813 , 25 February 2017 , pp. 205 - 249
Check for updates
Copyright
© 2017 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Abrahamson, J. 1975 Collision rates of small particles in a vigorously turbulent fluid. Chem. Engng Sci. 30 (11), 1371–1379.Google Scholar
Batchelor, G. K. 1953 The Theory of Homogeneous Turbulence. Cambridge University Press.Google Scholar
Bec, J., Biferale, L., Cencini, M., Lanotte, A. S. & Toschi, F. 2010 Intermittency in the velocity distribution of heavy particles in turbulence. J. Fluid Mech. 646, 527–536.CrossRefGoogle Scholar
Bragg, A. D. & Collins, L. R. 2014a New insights from comparing statistical theories for inertial particles in turbulence: I. spatial distribution of particles. New J. Phys. 16 (5), 055013.Google Scholar
Bragg, A. D. & Collins, L. R. 2014b New insights from comparing statistical theories for inertial particles in turbulence: Ii. relative velocities. New J. Phys. 16 (5), 055014.Google Scholar
Brucker, K. A., Isaza, J. C., Vaithianathan, T. & Collins, L. R. 2007 Efficient algorithm for simulating homogeneous turbulent shear flow without remeshing. J. Comput. Phys. 225, 20–32.CrossRefGoogle Scholar
Brunk, B. K., Koch, D. L. & Lion, L. W. 1997 Hydrodynamic pair diffusion in isotropic random velocity fields with application to turbulent coagulation. Phys. Fluids 9, 2670–2691.Google Scholar
Chiang, E. & Youdin, A. 2005 Forming planetesimals in solar and extrasolar nebulae. Annu. Rev. Earth Planet. Sci. 38, 493–522.Google Scholar
Chun, J., Koch, D. L., Rani, S. L., Ahluwalia, A. & Collins, L. R. 2005 Clustering of aerosol particles in isotropic turbulence. J. Fluid Mech. 536, 219–251.CrossRefGoogle Scholar
Eswaran, V. & Pope, S. B. 1988 An examination of forcing in direct numerical simulations of turbulence. Comput. Fluids 16, 257–278.CrossRefGoogle Scholar
Février, P., Simonin, O. & Legendre, D. 2001 Particle dispersion and preferential concentration dependence on turbulent reynolds number from direct and large-eddy simulations of isotropic homogeneous turbulence. In Proceedings of the Fourth International Conference on Multiphase Flow, New Orleans. Elsevier.Google Scholar
Gustavsson, K. & Mehlig, B. 2011 Distribution of relative velocities in turbulent aerosols. Phys. Rev. E 84 (4), 045304.Google ScholarPubMed
Ireland, P. J., Bragg, A. D. & Collins, L. R. 2016 The effect of Reynolds number on inertial particle dynamics in isotropic turbulence. Part I. Simulations without gravitational effects. J. Fluid Mech. 796, 617–658.CrossRefGoogle Scholar
Ireland, P. J., Vaithianathan, T., Sukheswalla, P. S., Ray, B. & Collins, L. R. 2013 Highly parallel particle-laden flow solver for turbulence research. Comput. Fluids 76, 170–177.Google Scholar
Jung, J., Yeo, K. & Lee, C. 2008 Behavior of heavy particles in isotropic turbulence. Phys. Rev. E 77, 016307.Google Scholar
Kraichnan, R. H. 1977 Eulerian and lagrangian renormalization in turbulence theory. J. Fluid Mech. 83 (2), 349–374.Google Scholar
Lundgren, T. S. 1981 Turbulent pair dispersion and scalar diffusion. J. Fluid Mech. 111, 27–57.CrossRefGoogle Scholar
Maxey, M. R. & Riley, J. J. 1983 Equation of motion for a small rigid sphere in a nonuniform flow. Phys. Fluids 26 (4), 883–889.Google Scholar
Mehlig, B., Uski, V. & Wilkinson, M. 2007 Colliding particles in highly turbulent flows. Phys. Fluids 19 (9), 098107.Google Scholar
Pan, L. & Padoan, P. 2010 Relative velocity of inertial particles in turbulent flows. J. Fluid Mech. 661, 73–107.Google Scholar
Pan, L. & Padoan, P. 2013 Turbulence-induced relative velocity of dust particles. I. Identical particles. Astrophys. J. 776, 1–37.Google Scholar
Pan, L. & Padoan, P. 2014a Turbulence-induced relative velocity of dust particles. II. The bidisperse case. Astrophys. J. 791, 1–20.Google Scholar
Pan, L. & Padoan, P. 2014b Turbulence-induced relative velocity of dust particles. III. The probability distribution. Astrophys. J. 792, 1–24.CrossRefGoogle Scholar
Pan, L. & Padoan, P. 2014c Turbulence-induced relative velocity of dust particles. IV. The collision kernel. Astrophys. J. 797, 1–18.Google Scholar
Pan, L. & Padoan, P. 2015 Turbulence-induced relative velocity of dust particles. V. Testing previous models. Astrophys. J. 812, 1–21.Google Scholar
Pan, L., Padoan, P., Scalo, J., Kritsuk, A. G. & Norman, M. L. 2011 Turbulent clustering of protoplanetary dust and planetesimal formation. Astrophys. J. 740 (1), 6.Google Scholar
Pope, S. B. 2000 Turbulent Flows. Cambridge University Press.Google Scholar
Rani, S. L., Dhariwal, R. & Koch, D. L. 2014 A stochastic model for the relative motion of high stokes number particles in isotropic turbulence. J. Fluid Mech. 756, 870–902.Google Scholar
Ray, B. & Collins, L. R. 2011 Preferential concentration and relative velocity statistics of inertial particles in Navier–Stokes turbulence with and without filtering. J. Fluid Mech. 680, 488–510.CrossRefGoogle Scholar
Reeks, M. W. 1992 On the continuum equations for dispersed particles in nonuniform flows. Phys. Fluids A 4, 1290–1303.Google Scholar
Wang, L.-P., Wexler, A. S. & Zhou, Y. 1998 Statistical mechanical descriptions of turbulent coagulation. Phys. Fluids 10 (10), 2647–2651.Google Scholar
Wang, L.-P., Wexler, A. S. & Zhou, Y. 2000 Statistical mechanical description and modelling of turbulent collision of inertial particles. J. Fluid Mech. 415, 117–153.Google Scholar
Yeung, P. K. & Pope, S. B. 1989 Lagrangian statistics from direct numerical simulations of isotropic turbulence. J. Fluid Mech. 207, 536.Google Scholar
Zaichik, L. I. & Alipchenkov, V. M. 2003 Pair dispersion and preferential concentration of particles in isotropic turbulence. Phys. Fluids 15, 1776–1787.Google Scholar
Zaichik, L. I. & Alipchenkov, V. M. 2007 Refinement of the probability density function model for preferential concentration of aerosol particles in isotropic turbulence. Phys. Fluids 19 (11), 113308.Google Scholar
Zaichik, L. I. & Alipchenkov, V. M. 2009 Statistical models for predicting pair dispersion and particle clustering in isotropic turbulence and their applications. New J. Phys. 11 (10), 103018.CrossRefGoogle Scholar
Zaichik, L. I., Simonin, O. & Alipchenkov, V. M. 2003 Two statistical models for predicting collision rates of inertial particles in homogeneous isotropic turbulence. Phys. Fluids 15 (10), 2995–3005.Google Scholar
Zaichik, L. I., Simonin, O. & Alipchenkov, V. M. 2006 Collision rates of bidisperse inertial particles in isotropic turbulence. Phys. Fluids 18, 035110.CrossRefGoogle Scholar