Published online by Cambridge University Press: 25 May 2021
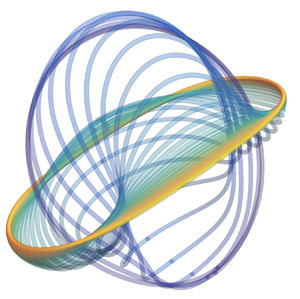
We study the behaviour of circular flexible loops sedimenting in a viscous fluid by numerical simulations and linear stability analysis. The numerical model involves a local slender-body theory approximation for the flow coupled to the Euler–Bernoulli elastic forces for an inextensible fibre. Starting from an inclined circle, we simulate the dynamics using truncated Fourier modes to observe three distinct regimes of motion: absolute stability, two- and three-dimensional dynamics, depending on the relative importance of the elastic and gravitational forces. We identify the governing parameter and develop a simple semi-analytic stability criterion, which we verify numerically. In all cases, sedimenting loops converge to stable, planar shape equilibria with one free parameter related to the initial conditions and material properties of the fibre.