Published online by Cambridge University Press: 07 January 2020
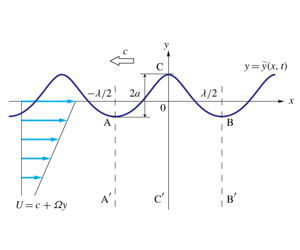
This paper describes linear stability analysis of the two-dimensional steady motion of periodic deep-water waves with symmetric non-overhanging profiles propagating on a linear shear current, namely a vertically sheared current with constant vorticity. In order to investigate numerically with high accuracy the stability of large-amplitude waves, we adopt a formulation using conformal mapping, in which the time-varying water surface is always mapped onto the real axis of a complex plane. This formulation allows us to apply numerical methods developed for large-amplitude irrotational waves without a shear current directly to the present problem, and reduces the linear stability problem to a generalized eigenvalue problem. Numerical solutions describe both super- and sub-harmonic instabilities of the periodic waves for a wide range of wave amplitudes and clarify how the behaviours of dominant eigenvalues change with the strength of the shear current. In particular, it is shown that, even in the presence of a linear shear current, the steady periodic waves lose stability due to superharmonic disturbances at the wave amplitude where the wave energy attains an extremum, similarly to the case of irrotational waves without a shear current. It is also found that re-stabilization with an increase in wave amplitude characterizes subharmonic instability for weak shear currents, but the re-stabilization disappears for strong shear currents.