Article contents
Spin-up of a two-component superfluid: analytic theory in arbitrary geometry
Published online by Cambridge University Press: 18 July 2013
Abstract
The impulsive spin-up of a two-component superfluid and its container is solved analytically for the first time in arbitrary geometry, generalizing the extensively studied case of single-fluid spin-up. The superfluid is modelled by the Hall–Vinen–Bekarevich–Khalatnikov (HVBK) two-fluid equations, neglecting non-hydrodynamic processes like vortex tension and pinning, which are weak in certain applications (e.g. neutron stars) and confined to boundary layers in the inviscid HVBK component in other applications (e.g. helium II spin-up in smooth-walled containers). Both components of the flow are found to be columnar. The spin-up time depends on the geometry, mutual friction coefficients, 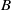 $B$,
$B$, 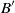 ${B}^{\prime } $, the Ekman number
${B}^{\prime } $, the Ekman number 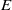 $E$, and the superfluid density fraction
$E$, and the superfluid density fraction 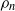 ${\rho }_{n} $. For
${\rho }_{n} $. For 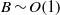 $B\sim O(1)$, the inviscid component undergoes Ekman pumping due to strong coupling to the viscous component, and the azimuthal velocities are ‘locked together’ during the spin-up. For
$B\sim O(1)$, the inviscid component undergoes Ekman pumping due to strong coupling to the viscous component, and the azimuthal velocities are ‘locked together’ during the spin-up. For 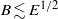 $B\lesssim {E}^{1/ 2} $, there is no Ekman pumping in the inviscid component and the inviscid azimuthal velocity spins up via mutual friction on a combination of the mutual friction and Ekman time scales. The spin-up process is studied in spheres, cylinders (with co- and counter-rotating lids), and cones, and occurs faster in spheres and cones. Ekman pumping is (anti-) clockwise adjacent to the (lower-) upper-right boundary, and mirrored about the rotation axis. The solution obtained by Reisenegger (J. Low Temp. Phys., vol. 92, 1993, p. 77) between slowly accelerating parallel plates is recovered in the associated limit. The hydrodynamic torque on the container decays dual-exponentially with time in a cylinder but not in a sphere or cone, where it is a superposition of exponentials whose time scales vary with latitude. The torque is a good diagnostic of the flow; e.g. it is steepest initially in a sphere with strong mutual friction.
$B\lesssim {E}^{1/ 2} $, there is no Ekman pumping in the inviscid component and the inviscid azimuthal velocity spins up via mutual friction on a combination of the mutual friction and Ekman time scales. The spin-up process is studied in spheres, cylinders (with co- and counter-rotating lids), and cones, and occurs faster in spheres and cones. Ekman pumping is (anti-) clockwise adjacent to the (lower-) upper-right boundary, and mirrored about the rotation axis. The solution obtained by Reisenegger (J. Low Temp. Phys., vol. 92, 1993, p. 77) between slowly accelerating parallel plates is recovered in the associated limit. The hydrodynamic torque on the container decays dual-exponentially with time in a cylinder but not in a sphere or cone, where it is a superposition of exponentials whose time scales vary with latitude. The torque is a good diagnostic of the flow; e.g. it is steepest initially in a sphere with strong mutual friction.
JFM classification
- Type
- Papers
- Information
- Copyright
- ©2013 Cambridge University Press
References
- 11
- Cited by


