Hostname: page-component-586b7cd67f-tf8b9 Total loading time: 0 Render date: 2024-11-26T02:02:56.088Z Has data issue: false hasContentIssue false
  • English
  • Français

Spectral broadening and flow randomization in free shear layers

Published online by Cambridge University Press:  06 July 2012

Xuesong Wu  and
Feng Tian
Xuesong Wu*
Affiliation:
Department of Mechanics, Tianjin University, Tianjin 300072, PR China Department of Mathematics, Imperial College London 180 Queen’s Gate, London SW7 2AZ, UK
Feng Tian
Affiliation:
Department of Mechanics, Tianjin University, Tianjin 300072, PR China
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

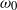It has been observed experimentally that when a free shear layer is perturbed by a disturbance consisting of two waves with frequencies and 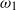, components with the combination frequencies ( and being integers) develop to a significant level thereby causing flow randomization. This spectral broadening process is investigated theoretically for the case where the frequency difference 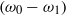is small, so that the perturbation can be treated as a modulated wavetrain. A nonlinear evolution system governing the spectral dynamics is derived by using the non-equilibrium nonlinear critical layer approach. The formulation provides an appropriate mathematical description of the physical concepts of sideband instability and amplitude–phase modulation, which were suggested by experimentalists. Numerical solutions of the nonlinear evolution system indicate that the present theory captures measurements and observations rather well.

JFM classification

Instability: Nonlinear instability Instability: Parametric instability
Type
Papers
Information
Journal of Fluid Mechanics , Volume 706 , 10 September 2012 , pp. 431 - 469
Copyright
Copyright © Cambridge University Press 2012

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Brown, G. L. & Roshko, A. 1974 On density effects and large structure in turbulent mixing layers. J. Fluid Mech. 64, 775–816.CrossRefGoogle Scholar
2. Cheung, L. C. & Lele, S. K. 2009 Linear and nonlinear processes in two-dimensional mixing layer dynamics and sound radiation. J. Fluid Mech. 625, 321–351.CrossRefGoogle Scholar
3. Cowley, S. J. & Wu, X. 1994 Asymptotic approaches to transition modelling. In Progress in Transition Modelling, AGARD Report 793, Chapter 3, pp. 1–38.Google Scholar
4. Freymuth, P. 1966 On transition in a separated laminar boundary layer. J. Fluid Mech. 25, 683–704.CrossRefGoogle Scholar
5. Goldstein, M. E. 1994 Nonlinear interactions between oblique instability waves on nearly parallel shear flows. Phys. Fluids A 6, 724–735.CrossRefGoogle Scholar
6. Goldstein, M. E. & Hultgren, L. S. 1988 Nonlinear spatial evolution of an externally excited instability wave in a free shear layer. J. Fluid Mech. 197, 295–330.CrossRefGoogle Scholar
7. Goldstein, M. E. & Leib, S. J. 1988 Nonlinear roll-up of externally excited shear layers. J. Fluid Mech. 191, 481–515.CrossRefGoogle Scholar
8. Haberman, R. 1972 Critical layers in parallel shear flows. Stud. Appl. Math. 51, 139–161.CrossRefGoogle Scholar
9. Hajj, M. R. 1997 Stability characteristics of a periodically unsteady mixing layer. Phys. Fluids A 9 (2), 392–398.CrossRefGoogle Scholar
10. Herbert, Th. 1997 Parabolized stability equations. Annu. Rev. Fluid Mech. 29, 245–283.CrossRefGoogle Scholar
11. Ho, C. M. & Huerre, P. 1984 Perturbed free shear layers. Annu. Rev. Fluid Mech. 16, 365–422.Google Scholar
12. Huerre, P. & Monkewitz, P. A. 1990 Local and global instabilities in spatially developing flows. Annu. Rev. Fluid Mech. 22, 473–537.CrossRefGoogle Scholar
13. Hultgren, L. S. 1992 Nonlinear spatial equilibration of an externally excited instability wave in a free shear layer. J. Fluid Mech. 236, 635–664.CrossRefGoogle Scholar
14. Kachanov, Y. S., Kozlov, V. V. & Levchenko, V. Y. 1979 Experiments on nonlinear interaction of waves in boundary layer. In IUTAM Symposium on Laminar–Turbulent Transition, Stuttgart, Germany (ed. Eppler, E. & Fasel, H. ). Springer.Google Scholar
15. Kim, Y. C., Khadra, L. & Powers, E. J. 1980 Wave modulation in a nonlinear dispersive medium. Phys. Fluids A 23 (11), 2250–2257.CrossRefGoogle Scholar
16. Leib, S. J. & Goldstein, M. E. 1989 Nonlinear interaction between the sinuous and varicose instability modes in a plane wake. Phys. Fluids A 1, 513–521.CrossRefGoogle Scholar
17. Liu, J. T. C. 1989 Coherent structures in transitional and turbulent free shear flows. Annu. Rev. Fluid Mech. 21, 285–315.CrossRefGoogle Scholar
18. Mankbadi, R. R. 1991 Multifrequency excited jets. Phys. Fluids 3 (4), 595–605.Google Scholar
19. Mattingly, G. E. & Criminale, W. O. 1972 The stability of an incompressible wake. J. Fluid Mech. 51, 233–272.CrossRefGoogle Scholar
20. Michalke, A. 1965 On spatially growing disturbances in an inviscid shear layer. J. Fluid Mech. 22, 371–383.CrossRefGoogle Scholar
21. Miksad, R. W. 1972 Experiments on the nonlinear stages of free-shear-layer transition. J. Fluid Mech. 56, 695–719.CrossRefGoogle Scholar
22. Miksad, R. W. 1973 Experiments on nonlinear interactions in the transition of a free shear layer. J. Fluid Mech. 59, 1–21.CrossRefGoogle Scholar
23. Miksad, R. W., Jones, F. L., Powers, E. J., Kim, Y. C. & Khadra, L. 1982 Experiments on the role of amplitude and phase modulations during transition to turbulence. J. Fluid Mech. 123, 1–29.CrossRefGoogle Scholar
24. Miksad, R. W., Jones, F. L. & Powers, E. J. 1983 Measurements of nonlinear interaction during natural transition of a symmetric wake. Phys. Fluids 26 (6), 1402–1409.CrossRefGoogle Scholar
25. Motohashi, T. 1979 A higher-order nonlinear interaction among spectral components. Phys. Fluids 22 (6), 1212–1213.CrossRefGoogle Scholar
26. Ritz, Ch. P., Powers, E. J., Miksad, R. W. & Solis, R. S. 1988 Nonlinear spectral dynamics of a transitioning flow. Phys. Fluids 31 (12), 3577–3588.CrossRefGoogle Scholar
27. Sandham, N. D., Morfey, C. L. & Hu, Z. W. 2006 Nonlinear mechanisms of sound generation in a perturbed parallel jet flow. J. Fluid Mech. 565, 1–23.Google Scholar
28. Sandham, N. D. & Salgado, A. 1876 Nonlinear interaction model of subsonic jet noise. Phil. Trans. R. Soc. 366, 2745–2760.CrossRefGoogle Scholar
29. Sato, H. 1956 Experimental investigation on the transition of laminar separated layer. J. Phys. Soc. Japan 11, 702–709.CrossRefGoogle Scholar
30. Sato, H. 1959 Further investigation on the transition of two-dimensional separated layer at subsonic speeds. J. Phys. Soc. Japan 14, 1797–1810.CrossRefGoogle Scholar
31. Sato, H. 1970 An experimental study of nonlinear interaction of velocity fluctuations in the transition region of a two-dimensional wake. J. Fluid Mech. 44, 741–765.Google Scholar
32. Sato, H. & Kuriki, K. 1961 The mechanism of transition in the wake of a thin flat plate placed parallel to a uniform flow. J. Fluid Mech. 11, 321–353.CrossRefGoogle Scholar
33. Sato, H. & Saito, H. 1975 Fine structure of energy spectra of velocity fluctuations in the transition region of a two-dimensional wake. J. Fluid Mech. 67, 539–559.CrossRefGoogle Scholar
34. Sparks, C. A. & Wu, X. 2008 Nonlinear development of subsonic modes on compressible mixing layers: a unified strongly nonlinear critical-layer theory. J. Fluid Mech. 614, 105–144.CrossRefGoogle Scholar
35. Suponitsky, V., Sandham, N. D. & Morfey, C. L. 2010 Linear and nonlinear mechanisms of sound radiation by instability waves in subsonic jets. J. Fluid Mech. 658, 509–538.CrossRefGoogle Scholar
36. Stuart, J. T. & Diprima, R. C. 1978 The Eckhaus and Benjamin–Feir resonance mechanisms. Proc. R. Soc. Lond. A 362, 27–41.Google Scholar
37. Winant, C. D. & Browand, F. K. 1974 Vortex pairing: the mechanism of turbulent mixing-layer growth at moderate Reynolds number. J. Fluid Mech. 63, 237–255.CrossRefGoogle Scholar
38. Wu, X. 1996 On an active resonant triad of mixed modes in symmetric shear flows: a plane wake as a paradigm. J. Fluid Mech. 317, 337–368.CrossRefGoogle Scholar
39. Wu, X. 2004 Non-equilibrium, nonlinear critical layers in laminar–turbulent transition. Acta Mechanica Sin. 20 (4), 327–339.Google Scholar
40. Wu, X. & Huerre, P. 2009 Low-frequency sound radiated by a nonlinearly modulated wavepacket of helical modes on a subsonic circular jet. J. Fluid Mech. 637, 173–211.CrossRefGoogle Scholar