Hostname: page-component-78c5997874-g7gxr Total loading time: 0 Render date: 2024-11-17T13:21:11.116Z Has data issue: false hasContentIssue false
  • English
  • Français

Spatial structure of shock formation

Published online by Cambridge University Press:  05 May 2017

J. Eggers Open the ORCID record for J. Eggers [Opens in a new window] ,
T. Grava ,
M. A. Herrada  and
G. Pitton
J. Eggers*
Affiliation:
School of Mathematics, University of Bristol, University Walk, Bristol BS8 1TW, UK
T. Grava
Affiliation:
School of Mathematics, University of Bristol, University Walk, Bristol BS8 1TW, UK SISSA, via Bonomea 265, 34136 Trieste, Italy
M. A. Herrada
Affiliation:
School of Mathematics, University of Bristol, University Walk, Bristol BS8 1TW, UK E.S.I., Universidad de Sevilla, Camino de los Descubrimientos s/n 41092, Spain
G. Pitton
Affiliation:
SISSA, via Bonomea 265, 34136 Trieste, Italy
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The formation of a singularity in a compressible gas, as described by the Euler equation, is characterized by the steepening and eventual overturning of a wave. Using self-similar variables in two space dimensions and a power series expansion based on powers of $|t_{0}-t|^{1/2}$, 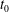$t_{0}$ being the singularity time, we show that the spatial structure of this process, which starts at a point, is equivalent to the formation of a caustic, i.e. to a cusp catastrophe. The lines along which the profile has infinite slope correspond to the caustic lines, from which we construct the position of the shock. By solving the similarity equation, we obtain a complete local description of wave steepening and of the spreading of the shock from a point. The shock spreads in the transversal direction as $|t_{0}-t|^{1/2}$ and in the direction of propagation as 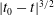$|t_{0}-t|^{3/2}$, as also found in a one-dimensional model problem.

JFM classification

Compressible Flows: Compressible Flows Compressible Flows: Shock waves
Type
Papers
Information
Journal of Fluid Mechanics , Volume 820 , 10 June 2017 , pp. 208 - 231
Check for updates
Copyright
© 2017 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Alinhac, S. 1993 Temps de vie des solutions régulières des équations d’Euler compressibles. Invent. Math. 111, 627–670.CrossRefGoogle Scholar
Arnold, V. I. 1989 Mathematical Methods of Classical Mechanics, 2nd edn. Springer.CrossRefGoogle Scholar
Arnold, V. I. 1990 Singularities of Caustics and Wave Fronts. Kluwer.CrossRefGoogle Scholar
Berry, M. V. 1981 Singularities in waves and rays. In Les Houches, Session XXXV (ed. Balian, R., Kleman, M. & Poirier, J.-P.), pp. 453–543. North-Holland.Google Scholar
Bianchini, S. & Bressan, A. 2005 Vanishing viscosity solutions of nonlinear hyperbolic systems. Ann. Maths 161, 223–342.CrossRefGoogle Scholar
Bordemann, M. & Hoppe, J. 1993 The dynamics of relativistic membranes. Reduction to 2-dimensional fluid dynamics. Phys. Lett. B 317, 315–320.CrossRefGoogle Scholar
Canuto, C., Hussaini, M. Y., Quarteroni, A. & Zhang, T. 2006 Spectral Methods. vol. 1. Springer.CrossRefGoogle Scholar
Cates, A. T. & Crighton, D. G. 1990 Nonlinear diffraction and caustic formation. Proc. R. Soc. Lond. A 430, 69–88.Google Scholar
Cates, A. T. & Sturtevant, B. 1997 Shock wave focusing using geometrical shock dynamics. Phys. Fluids 9, 3058–3068.CrossRefGoogle Scholar
Chiodaroli, E. & De Lellis, C. 2015 Global ill-posedness of the isentropic system of gas dynamics. Commun. Pure Appl. Maths 68, 1157–1190.CrossRefGoogle Scholar
Christodoulou, D. 2007 The Formation of Shocks in 3-dimensional Fluids. EMS Monographs in Mathematics.CrossRefGoogle Scholar
Cole, J. D. 1951 On a quasi-linear parabolic equation occurring in aerodynamics. Q. Appl. Maths 9, 225–236.CrossRefGoogle Scholar
Courant, R. & Friedrichs, K. O. 1948 Supersonic Flow and Shock Waves. Springer.Google Scholar
Cramer, M. S. & Seebass, A. R. 1978 Focusing of weak shock waves at an arête. J. Fluid Mech. 88, 209–222.CrossRefGoogle Scholar
Dubrovin, B., Grava, T., Klein, C. & Moro, A. 2015 On critical behaviour in systems of hamiltonian partial differential equations. J. Nonlinear Sci. 25, 631–707.Google Scholar
Dubrovin, B., Grava, T. & Klein, C. 2016 On critical behaviour in generalized Kadomtsev-Petviashvili equations. Physica D 333, 157–170.Google Scholar
Eggers, J. & Fontelos, M. A. 2009 The role of self-similarity in singularities of partial differential equations. Nonlinearity 22, R1–R44.CrossRefGoogle Scholar
Eggers, J. & Fontelos, M. A. 2015 Singularities: Formation, Structure, and Propagation. Cambridge University Press.Google Scholar
Eggers, J., Hoppe, J., Hynek, M. & Suramlishvili, N. 2014 Singularities of relativistic membranes. Geom. Flows 1, 17–33.Google Scholar
Elling, V. 2006 A possible counterexample to well posdness of entropy solutions and to Godunov scheme convergence. Maths Comput. 75, 1721–1733.Google Scholar
Grava, T., Klein, C. & Eggers, J. 2016 Shock formation in the dispersionless Kadomtsev–Petviashvili equation. Nonlinearity 29, 1384–1416.CrossRefGoogle Scholar
Hopf, E. 1950 The partial differential equation u t + uu x =𝜇 u xx . Commun. Pure Appl. Maths 3, 201–230.CrossRefGoogle Scholar
Hou, T. Y. 2009 Blow-up or no blow-up? A unified computational and analytic approach to 3D incompressible Euler and Navier–Stokes equations. Acta Numerica 18, 277–346.CrossRefGoogle Scholar
Kruzkov, S. N. 1969 Generalized solutions of the Cauchy problem in the large for first order nonlinear equations. Dokl. Akad. Nauk SSSR 187, 29–32.Google Scholar
Kurganov, A. & Levy, D. 2002 Central-upwind schemes for the Saint-Venant system. Math. Modelling Numer. Anal. 36, 397–425.Google Scholar
Landau, L. D. & Lifshitz, E. M. 1984 Fluid Mechanics. Pergamon.Google Scholar
Lax, P. D. 1973 Hyperbolic Systems of Conservation Laws and the Mathematical Theory of Shock Waves. (CBMS Regional Conf. Ser. in Appl. Math.) , vol. 11. SIAM.Google Scholar
van Leer, B. 1979 Towards the ultimate conservative difference scheme. V. A second order sequel to Godunov’s method. J. Comput. Phys. 32, 101–136.Google Scholar
Majda, A. 1984 Smooth solutions for the equations of compressible and incompressible fluid flow. In Fluid Dynamics (ed. Beirão da Veiga, H.), vol. 1047, pp. 75–126. Springer.Google Scholar
Manakov, S. V. & Santini, P. M. 2008 On the solutions of the dKP equation: the nonlinear Riemann Hilbert problem, longtime behaviour, implicit solutions and wave breaking. Nonlinearity 41, 055204.Google Scholar
Manakov, S. V. & Santini, P. M. 2012 Wave breaking in the solutions of the dispersionless Kadomtsev–Petviashvili equation at a finite time. Theor. Math. Phys. 172, 1118–1126.Google Scholar
Nye, J. 1999 Natural Focusing and Fine Structure of Light: Caustics and Wave Dislocations. Institute of Physics Publishing.Google Scholar
Pomeau, Y., Jamin, T., Le Bars, M., Le Gal, P. & Audoly, B. 2008a Law of spreading of the crest of a breaking wave. Proc. R. Soc. Lond. A 464, 1851–1866.Google Scholar
Pomeau, Y., Le Berre, M., Guyenne, P. & Grilli, S. 2008b Wave-breaking and generic singularities of nonlinear hyperbolic equations. Nonlinearity 21, T61–T79.CrossRefGoogle Scholar
Popinet, S. 2011 Quadtree-adaptive tsunami modelling. Ocean Dyn. 61, 1261–1285.Google Scholar
Poston, T. & Stewart, I. 1978 Catastrophe Theory and Its Applications. Dover.Google Scholar
Riemann, B. 1860 Über die Fortpflanzung ebener Luftwellen von endlicher Schwingungsweite. Abhandlungen der Gesellschaft der Wissenschaften zu Göttingen, Mathematisch-Physikalische Klasse 8, 43–66.Google Scholar
Rosenblum, S., Bechler, O., Shomroni, I., Kaner, R., Arusi-Parpar, T., Raz, O. & Dayan, B. 2014 Demonstration of fold and cusp catastrophes in an atomic cloud reflected from an optical barrier in the presence of gravity. Phys. Rev. Lett. 112, 120403.CrossRefGoogle Scholar
Sturtevant, B. & Kulkarny, V. A. 1976 The focusing of weak shock waves. J. Fluid Mech. 73, 651–671.Google Scholar
Thom, R. 1976 The two-fold way of catastrophe theory. In Structural Stability, the Theory of Catastrophes, and Applications in the Sciences (ed. Hilton, P. J.), pp. 235–252. Springer.Google Scholar