Published online by Cambridge University Press: 02 February 2018
Mixing and collisions of inertial particles at the small scales of turbulence can be investigated by considering how pairs of particles move relative to each other. In real problems the two particles will have different sizes, i.e. they are bidisperse, and the effect of gravity on their motion is often important. However, how turbulence and gravity compete to control the motion of bidisperse inertial particles is poorly understood. Motivated by this, we use direct numerical simulations (DNS) to investigate the dynamics of settling, bidisperse particles in isotropic turbulence. In agreement with previous studies, we find that without gravity (i.e.  $Fr=\infty$, where
$Fr=\infty$, where 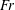 $Fr$ is the Froude number), bidispersity leads to an enhancement of the relative velocities, and a suppression of their spatial clustering. For
$Fr$ is the Froude number), bidispersity leads to an enhancement of the relative velocities, and a suppression of their spatial clustering. For 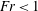 $Fr<1$, the relative velocities in the direction of gravity are enhanced by the differential settling velocities of the bidisperse particles, as expected. However, we also find that gravity can strongly enhance the relative velocities in the ‘horizontal’ directions (i.e. in the plane normal to gravity). This non-trivial behaviour occurs because fast settling particles experience rapid fluctuations in the fluid velocity field along their trajectory, leading to enhanced particle accelerations and relative velocities. Indeed, the results show that even when
$Fr<1$, the relative velocities in the direction of gravity are enhanced by the differential settling velocities of the bidisperse particles, as expected. However, we also find that gravity can strongly enhance the relative velocities in the ‘horizontal’ directions (i.e. in the plane normal to gravity). This non-trivial behaviour occurs because fast settling particles experience rapid fluctuations in the fluid velocity field along their trajectory, leading to enhanced particle accelerations and relative velocities. Indeed, the results show that even when  $Fr\ll 1$, turbulence can still play an important role, not only on the horizontal motion, but also on the vertical motion of the particles. This is related to the fact that
$Fr\ll 1$, turbulence can still play an important role, not only on the horizontal motion, but also on the vertical motion of the particles. This is related to the fact that 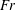 $Fr$ only characterizes the importance of gravity compared with some typical acceleration of the fluid, yet accelerations in turbulence are highly intermittent. As a consequence, there is a significant probability for particles to be in regions of the flow where the Froude number based on the local, instantaneous fluid acceleration is
$Fr$ only characterizes the importance of gravity compared with some typical acceleration of the fluid, yet accelerations in turbulence are highly intermittent. As a consequence, there is a significant probability for particles to be in regions of the flow where the Froude number based on the local, instantaneous fluid acceleration is 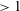 ${>}1$, even though the typically defined Froude number is
${>}1$, even though the typically defined Froude number is 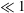 $\ll 1$. This could imply, for example, that extreme events in the mixing of settling, bidisperse particles are only weakly affected by gravity even when
$\ll 1$. This could imply, for example, that extreme events in the mixing of settling, bidisperse particles are only weakly affected by gravity even when 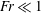 $Fr\ll 1$. We also find that gravity drastically reduces the clustering of bidisperse particles. These results are strikingly different to the monodisperse case, for which recent results have shown that when
$Fr\ll 1$. We also find that gravity drastically reduces the clustering of bidisperse particles. These results are strikingly different to the monodisperse case, for which recent results have shown that when 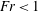 $Fr<1$, gravity strongly suppresses the relative velocities in all directions, and can enhance clustering. Finally, we consider the implications of these results for the collision rates of settling, bidisperse particles in turbulence. We find that for
$Fr<1$, gravity strongly suppresses the relative velocities in all directions, and can enhance clustering. Finally, we consider the implications of these results for the collision rates of settling, bidisperse particles in turbulence. We find that for 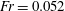 $Fr=0.052$, the collision kernel is almost perfectly predicted by the collision kernel for bidisperse particles settling in quiescent flow, such that the effect of turbulence may be ignored. However, for
$Fr=0.052$, the collision kernel is almost perfectly predicted by the collision kernel for bidisperse particles settling in quiescent flow, such that the effect of turbulence may be ignored. However, for  $Fr=0.3$, turbulence plays an important role, and the collisions are only dominated by gravitational settling when the difference in the particle Stokes numbers is
$Fr=0.3$, turbulence plays an important role, and the collisions are only dominated by gravitational settling when the difference in the particle Stokes numbers is 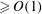 ${\geqslant}O(1)$.
${\geqslant}O(1)$.