Hostname: page-component-cd9895bd7-mkpzs Total loading time: 0 Render date: 2024-12-25T14:31:34.407Z Has data issue: false hasContentIssue false
  • English
  • Français

Similarity in Mach stem evolution and termination in unsteady shock-wave reflection

Published online by Cambridge University Press:  03 September 2020

E. Koronio ,
G. Ben-Dor ,
O. Sadot Open the ORCID record for O. Sadot [Opens in a new window]  and
M. Geva
E. Koronio
Affiliation:
Pearlstone Center for Aeronautical Engineering Studies, Department of Mechanical Engineering, Faculty of Engineering Sciences, Ben-Gurion University of the Negev, Beer Sheva8410501, Israel
G. Ben-Dor
Affiliation:
Pearlstone Center for Aeronautical Engineering Studies, Department of Mechanical Engineering, Faculty of Engineering Sciences, Ben-Gurion University of the Negev, Beer Sheva8410501, Israel
O. Sadot*
Affiliation:
Pearlstone Center for Aeronautical Engineering Studies, Department of Mechanical Engineering, Faculty of Engineering Sciences, Ben-Gurion University of the Negev, Beer Sheva8410501, Israel
M. Geva
Affiliation:
School of Mechanical Engineering, Tel-Aviv University, Tel-Aviv69978, Israel
*
† Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

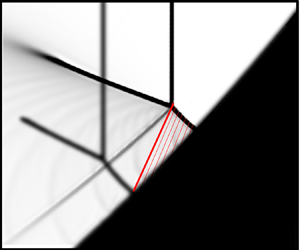
Shock-wave reflection over concave surfaces poses a difficulty in its analysis due to the unsteady nature of the reflection process and the occurrence of various types of Mach reflections caused by it. In a pseudo-steady flow, the reflection's configuration is self-similar since the shock wave reflects over a surface with constant inclination. The unsteady Mach reflection introduces an additional complexity as it is affected by the changing inclination of the surface, forcing the reflection to continuously adjust itself to the varying boundary condition. In this study, validated simulations of Mach reflection (MR) over cylindrical concave surfaces with different radii were performed for three inviscid perfect gases with moderate incident shock Mach numbers (Ms) ranging from 1.3 to 1.5. The reflection was investigated up to the point of transition from MR to transitioned regular reflection. A similar behaviour of the configuration and evolution of the Mach stem was observed, one that is independent of the surface radius and type of gas. With regards to different gases, the speed of sound a0 is a dominant factor since it dictates the propagation of wall disturbances. A universal condition of the rate of surface change was found, accounting for different radii, different gases and Ms variation. Analysis based on shock dynamics is employed to explain how disturbances caused by surface variations play a significant role in the behaviour of the reflection. This method successfully supports the similarity that was demonstrated and facilitates a more informed perception of the MR process.

JFM classification

Compressible Flows: Shock waves Compressible Flows: Compressible Flows Waves/Free-surface Flows: Wave-structure interactions
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 902 , 10 November 2020 , A1
Check for updates
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Barth, T. & Jespersen, D. 1989 The design and application of upwind schemes on unstructured meshes. In 27th Aerospace Sciences Meeting, American Institute of Aeronautics and Astronautics.CrossRefGoogle Scholar
Ben-Dor, G. 2007 Shock Wave Reflection Phenomena, 2nd edn. Springer.Google Scholar
Ben-Dor, G., Dewey, J. M. & Takayama, K. 1987 a The reflection of a planar shock wave over a double wedge. J. Fluid Mech. 176, 483–520.CrossRefGoogle Scholar
Ben-Dor, G. & Takayama, K. 1985 Analytical prediction of the transition from Mach to regular reflection over cylindrical concave wedges. J. Fluid Mech. 158, 365–380.CrossRefGoogle Scholar
Ben-Dor, G. & Takayama, K. 1986/7 The dynamics of the transition from Mach to regular reflection over concave cylinders. Isr. J. Tech. 23, 71–74.Google Scholar
Ben-Dor, G., Takayama, K. & Dewey, J. M. 1987 b Further analytical considerations of weak planar shock wave reflections over a concave wedge. Fluid Dyn. Res. 2, 77–85.CrossRefGoogle Scholar
Ben-Dor, G., Takayama, K. & Kawauchi, T. 1980 The transition from regular to Mach reflection and from Mach to regular reflexion in truly non-stationary flows. J. Fluid Mech. 100, 147–160.CrossRefGoogle Scholar
Geva, M., Ram, O. & Sadot, O. 2013 The non-stationary hysteresis phenomenon in shock wave reflections. J. Fluid Mech. 732 (R1), 1–11.CrossRefGoogle Scholar
Geva, M., Ram, O. & Sadot, O. 2018 The RR→MR transition in unsteady flow over convex surfaces. J. Fluid Mech. 837, 48–79.CrossRefGoogle Scholar
Gruber, S. & Skews, B. W. 2013 Weak shock wave reflection from concave surfaces. Exp. Fluids 54 (7), 1–14.CrossRefGoogle Scholar
Han, Z. & Yin, X. 1993 Shock Dynamics: Fluid Mechanics and its Application, vol. 11. Kluwer Academic.CrossRefGoogle Scholar
Holmes, D. & Connel, S. 1989 Solution of the 2D Navier–Stokes equations on unstructured adaptive grids. In 9th Computational Fluid Dynamics Conference, American Institute of Aeronautics and Astronautics.CrossRefGoogle Scholar
Hornung, H. G., Oertel, H. J. & Sanderman, R. J. 1979 Transition to Mach reflection of shock waves in steady and pseudo-steady flows with and without relaxation. J. Fluid Mech. 90, 541–560.CrossRefGoogle Scholar
Itoh, S., Okazaki, N. & Itaya, M. 1981 On the transition between regular and Mach reflection in truly non-stationary flows. J. Fluid Mech. 108, 383–400.CrossRefGoogle Scholar
Jameson, A., Schmidt, W. & Turkel, E. 1981 Numerical solution of the euler equations by finite volume methods schemes. In 14th Fluid and Plasma Dynamic Conference, vol. M, pp. 1–19. American Institute of Aeronautics and Astronautics.CrossRefGoogle Scholar
Kleine, H., Timofeev, E., Hakkaki-Fard, A. & Skews, B. 2014 The influence of Reynolds number on the triple point trajectories at shock reflection off cylindrical surfaces. J. Fluid Mech. 740, 47–60.CrossRefGoogle Scholar
Krassovskaya, V. & Berezkina, M. K. 2017 Mechanism of formation of reflection configurations over concave surfaces. Shock Waves 27, 431–439.CrossRefGoogle Scholar
Ram, O., Geva, M. & Sadot, O. 2015 High spatial and temporal resolution study of shock wave reflection over a coupled convex-concave cylindrical surface. J. Fluid Mech. 768, 219–239.CrossRefGoogle Scholar
Rausch, R. D., Batina, J. T. & Yang, H. T. Y. 1992 Spatial adaptation of unstructured meshes for unsteady aerodynamic flow computations. AIAA J. 30 (5), 1243–1251.CrossRefGoogle Scholar
Skews, B. W. 1967 The shape of a diffracting shock wave. J. Fluid Mech. 29, 297–304.CrossRefGoogle Scholar
Skews, B. W. & Kleine, H. 2007 Flow features resulting from shock wave impact on a cylindrical cavity. J. Fluid Mech. 580, 481–493.CrossRefGoogle Scholar
Skews, B. W. & Kleine, H. 2009 Unsteady flow diagnostics using weak perturbations. Exp. Fluids 46, 65–76.CrossRefGoogle Scholar
Soni, V., Hadjadj, A., Chaudhuri, A. & Ben-Dor, G. 2017 Shock-wave reflections over double-concave cylindrical reflectors. J. Fluid Mech. 813, 70–84.CrossRefGoogle Scholar
Takayama, K. & Ben-Dor, G. 1989 A reconsideration of the transition criterion form Mach to regular reflection over cylindrical concave surfaces. KSME J. 3, 6–9.CrossRefGoogle Scholar
Takayama, K. & Sasaki, M. 1983 Effects of radius of curvature and initial angle on the shock transition over concave and convex walls. Rep. Inst. High-Speed Mech. 46, 1–30.Google Scholar
Vasilev, E. I., Elperin, T. & Ben-Dor, G. 2008 Analytical reconsideration of the von Neumann paradox in the reflection of a shock wave over a wedge. Phys. Fluids 20 (4), 046101.CrossRefGoogle Scholar
Wang, H. & Zhai, Z. 2020 On regular reflection to Mach reflection transition in inviscid flow for shock reflection on a convex or straight wedge. J. Fluid Mech. 884, A27.CrossRefGoogle Scholar
Whitham, G. B. 1957 A new approach to problems of shock dynamics. Part I. Two-dimensional problems. J. Fluid Mech. 2 (02), 145–171.CrossRefGoogle Scholar
Whitham, G. B. 1973 Linear and Nonlinear Wave. Wiley.Google Scholar