Hostname: page-component-586b7cd67f-t8hqh Total loading time: 0 Render date: 2024-11-27T13:08:45.911Z Has data issue: false hasContentIssue false
  • English
  • Français

Scaling of detonation velocity in cylinder and slab geometries for ideal, insensitive and non-ideal explosives

Published online by Cambridge University Press:  21 May 2015

Scott I. Jackson  and
Mark Short
Scott I. Jackson*
Affiliation:
Shock and Detonation Physics Group, Los Alamos National Laboratory, Los Alamos, NM 87545, USA
Mark Short
Affiliation:
Shock and Detonation Physics Group, Los Alamos National Laboratory, Los Alamos, NM 87545, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Experiments were conducted to characterize the detonation phase-velocity dependence on charge thickness for two-dimensional detonation in condensed-phase explosive slabs of PBX 9501, PBX 9502 and ANFO. In combination with previous diameter-effect measurements from a cylindrical rate-stick geometry, these data permit examination of the relative scaling of detonation phase velocity between axisymmetric and two-dimensional detonation. 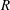We find that the ratio of cylinder radius ($R$) to slab thickness (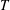$T$) at each detonation phase velocity (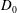$D_{0}$) is such that 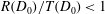$R(D_{0})/T(D_{0})<1$. The variation in the 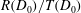$R(D_{0})/T(D_{0})$ scaling is investigated with two detonation shock dynamics (DSD) models: a lower-order model relates the normal detonation velocity to local shock curvature, while a higher-order model includes the effect of front acceleration and transverse flow. The experimentally observed 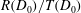$R(D_{0})/T(D_{0})$ (${<}1$) scaling behaviour for PBX 9501 and PBX 9502 is captured by the lower-order DSD theory, revealing that the variation in the scale factor is due to a difference in the slab and axisymmetric components of the curvature along the shock in the cylindrical geometry. The higher-order DSD theory is required to capture the observed $R(D_{0})/T(D_{0})$ (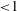${<}1$) scaling behaviour for ANFO. An asymptotic analysis of the lower-order DSD formulation describes the geometric scaling of the detonation phase velocity between the cylinder and slab geometries as the detonation phase velocity approaches the Chapman–Jouguet value.

JFM classification

Compressible Flows: Compressible Flows Compressible Flows: Detonation waves
Type
Papers
Information
Journal of Fluid Mechanics , Volume 773 , 25 June 2015 , pp. 224 - 266
Check for updates
Copyright
© 2015 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Anderson, E. K., Aslam, T. D. & Jackson, S. I. 2015 The effect of transverse shock propagation on the shock-to-detonation transition process for an insensitive explosive. Proc. Combust. Inst. 35 (2), 2033–2040.CrossRefGoogle Scholar
Aslam, T. D. 2007 Detonation shock dynamics calibration of PBX 9501. In Shock Compression of Condensed Matter (ed. Elert, M., Furnish, M. D., Chau, R., Holmes, N. & Nguyen, J.), AIP Conference Proceedings, vol. 955, pp. 813–816. American Institute of Physics.Google Scholar
Aslam, T. D., Bdzil, J. B. & Hill, L. G.1998 Extensions to DSD theory: analysis of PBX 9502 rate tick data. In 11th (International) Detonation Symposium, pp. 21–29. Office of Naval Research, ONR 33300-2.Google Scholar
Aslam, T., Jackson, S. I. & Morris, J. S. 2009 Proton radiography of PBX 9502 detonation shock dynamics confinement sandwich test. In Shock Compression of Condensed Matter (ed. Elert, M. L., Buttler, W. T., Furnish, M. D., Anderson, W. W. & Proud, W. G.), AIP Conference Proceedings, vol. 1195, pp. 241–244. American Institute of Physics.Google Scholar
Aslam, T. D. & Stewart, D. S. 1999 Detonation shock dynamics and comparisons with direct numerical simulations. Combust. Theor. Model. 3, 77–101.Google Scholar
Bdzil, J. B. 1981 Steady-state two-dimensional detonation. J. Fluid Mech. 108, 195–226.CrossRefGoogle Scholar
Bdzil, J. B., Aslam, T. D., Catanach, R. A., Hill, L. G. & Short, M.2002 DSD front models: nonideal explosive detonation in ANFO. In 12th International Detonation Symposium, pp. 409–417. Office of Naval Research, ONR 333-05-2.Google Scholar
Bdzil, J. B., Aslam, T. D., Henninger, R. & Quirk, J. J.2003 High-explosives performance: understanding the effects of a finite-length reaction zone. In Los Alamos Science, no. 28, pp. 96–110. Los Alamos National Laboratory.Google Scholar
Bdzil, J. B. & Davis, W. C.1975 Time-dependent detonations. Tech. Rep. LA-5926-MS. Los Alamos National Laboratory.Google Scholar
Bdzil, J. B., Fickett, W. & Stewart, D. S.1989 Detonation shock dynamics: a new approach to modeling multi-dimensional detonation waves. In 9th Symposium (International) on Detonation, pp. 730–742. Office of the Chief of Naval Research, OCNR 113291-7.Google Scholar
Bdzil, J. B., Short, M., Sharpe, G. J., Aslam, T. D. & Quirk, J. J.2006 Higher-order DSD for detonation propagation: DSD for detonation driven by multi-step chemistry models with disparate rates. In 13th International Detonation Symposium, pp. 726–736. Office of Naval Research, ONR 351-07-01.Google Scholar
Bdzil, J. B. & Stewart, D. S. 1986 Time-dependent two-dimensional detonation: the interaction of edge rarefactions with finite-length reaction zones. J. Fluid Mech. 171, 1–26.Google Scholar
Bdzil, J. B. & Stewart, D. S. 1989 Modelling two-dimensional detonations with detonation shock dynamics. Phys. Fluids A 1, 1261–1267.Google Scholar
Bdzil, J. B. & Stewart, D. S. 2007 The dynamics of detonation in explosive systems. Annu. Rev. Fluid Mech. 39, 263–292.Google Scholar
Brun, L., Kneib, J. M. & Lascaux, P.1994 Computing the transient self-sustained detonation after a new model. In 10th International Detonation Symposium, pp. 43–49. Office of Naval Research, ONR 33395-12.Google Scholar
Campbell, A. W. & Engelke, R.1976 The diameter effect in high-density heterogeneous explosives. In 6th Symposium (International) on Detonation, pp. 642–652. Office of Naval Research, ACR-221.Google Scholar
Chiquete, C., Jackson, S. I. & Short, M.2014 DSD calibration of PBX 9501 via slab geometry experiments. In 15th International Detonation Symposium. Office of Naval Research (in press).Google Scholar
Collyer, A. M., Dunnett, J. D., Swift, D. C. & White, S. J.1998 WBL detonation wave propagation for EDC35 and EDC37. In 11th International Detonation Symposium, pp. 12–20. Office of Naval Research, ONR 33300-5.Google Scholar
Eden, G. & Belcher, R. A.1989 The effects of inert walls on the velocity of detonation in EDC35, an insensitive high explosive. In 9th Symposium (International) on Detonation, pp. 831–841. Office of the Chief of Naval Research, OCNR 113291-7.Google Scholar
Eden, G. & Wright, P. W.1965 A technique for the precise measurement of the motion of a plane free surface. In 4th Symposium (International) on Detonation, pp. 573–583. Office of Naval Research, ACR-126.Google Scholar
Engelke, R. & Bdzil, J. B. 1983 A study of the steady-state reaction-zone structure of a homogenous and a heterogenous explosive. Phys. Fluids 26, 1210–1221.Google Scholar
Gibbs, T. R. & Popolato, A. 1980 LASL Explosive Property Data. University of California Press.Google Scholar
Gois, J. C., Campos, J. & Mendes, R. 1996 Extinction and initiation of detonation of NM-PMMA-GMB mixtures. In Shock Compression of Condensed Matter (ed. Schmidt, S. C. & Tao, W. C.), AIP Conference Proceedings, vol. 370, pp. 827–830. American Institute of Physics.Google Scholar
Gois, C., Presles, H. N. & Vidal, P. 1991 Effect of hollow heterogenities on nitromethane detonation. In Dynamic Aspects of Detonations (ed. Kuhl, A. L., Leyer, J.-C., Borisov, A. A. & Sirignano, W. A.), Progress in Aeronautics and Astronautics, vol. 153, pp. 462–470. American Institute of Aeronautics and Astronautics.Google Scholar
Helm, F., Finger, M., Hayes, B., Lee, E., Cheung, H. & Walton, J.1976 High explosive characterization for the Dice Throw Event. Tech. Rep. UCRL-52042. Lawrence Livermore Laboratory.Google Scholar
Higgins, A. 2009 Measurement of detonation velocity for a nonideal heterogenous explosive in axisymmetric and two-dimensional geometries. In Shock Compression of Condensed Matter (ed. Elert, M. L., Buttler, W. T., Furnish, M. D., Anderson, W. W. & Proud, W. G.), AIP Conference Proceedings, vol. 1195, pp. 193–196. American Institute of Physics.Google Scholar
Hill, L. G.2012 Compilation of ambient PBX 9501 detonation speed data. Tech. Rep. Los Alamos National Laboratory.Google Scholar
Hill, L. G. & Aslam, T. D. 2004 The LANL detonation-confinement test: prototype development and sample results. In Shock Compression of Condensed Matter (ed. Furnish, M. D., Gupta, Y. M. & Forbes, J. W.), AIP Conference Proceedings, vol. 706, pp. 847–850. American Institute of Physics.Google Scholar
Hill, L. G. & Aslam, T. D.2010 Detonation shock dynamics calibration for PBX 9502 with temperature, density, and material lot variations. In 14th International Detonation Symposium, pp. 779–788. Office of Naval Research, ONR-351-10-185.Google Scholar
Hill, L. G., Bdzil, J. B., Davis, W. C. & Critchfield, R. R.2006 PBX 9502 front curvature rate stick data: repeatability and the effects of temperature and material variation. In 13th International Detonation Symposium, pp. 331–341. Office of Naval Research, ONR 351-07-01.Google Scholar
Jackson, S. I., Austin, J. M. & Shepherd, J. E. 2006 Planar detonation wave initiation in large-aspect-ratio channels. AIAA J. 44 (10), 2422–2425.Google Scholar
Jackson, S. I., Kiyanda, C. B. & Short, M. 2011 Experimental observations of detonation in ammonium-nitrate-fuel-oil (ANFO) surrounded by a high-sound-speed, shockless, aluminum confiner. Proc. Combust. Inst. 33, 2219–2226.Google Scholar
Jackson, S. I. & Short, M. 2012 Determination of the velocity-curvature relationship for unknown front shapes. In Shock Compression of Condensed Matter (ed. Elert, M. L., Buttler, W. T., Borg, J. P., Jordan, J. L. & Vogler, T. J.), AIP Conference Proceedings, vol. 1426, pp. 347–350. American Institute of Physics.Google Scholar
Kasimov, A. R. & Stewart, D. S. 2005 Asymptotic theory of evolution and failure of self-sustained detonations. J. Fluid Mech. 525, 161–192.Google Scholar
Kennedy, D. L.1998 Multi-valued normal shock velocity versus curvature relationships for highly non-ideal explosives. In 11th (International) Detonation Symposium, pp. 181–192. Office of Naval Research, ONR 33300-2.Google Scholar
Kennedy, J. E. 1997 The gurney model of explosive output for driving metal. In Explosive Effects and Applications (ed. Zukas, J. A. & Walters, W. P.), pp. 221–257. Springer.Google Scholar
Mack, D. B., Petel, O. E. & Higgins, A. J. 2007 Detonation failure thickness measurement in an annular geometry. In Shock Compression of Condensed Matter (ed. Elert, M., Furnish, M. D., Chau, R., Holmes, N. & Nguyen, J.), AIP Conference Proceedings, vol. 955, pp. 833–836. American Institute of Physics.Google Scholar
Morris, J. S., Jackson, S. I. & Hill, L. G. 2009 A simple line wave generator using commercial explosives. In Shock Compression of Condensed Matter (ed. Elert, M. L., Buttler, W. T., Furnish, M. D., Anderson, W. W. & Proud, W. G.), AIP Conference Proceedings, vol. 1195, pp. 408–411. American Institute of Physics.Google Scholar
Petel, O. E. & Higgins, A. J. 2006 Comparison of failure thickness and critical diameter of nitromethane. In Shock Compression of Condensed Matter (ed. Furnish, M. D., Elert, M., Russell, T. P. & White, C. T.), AIP Conference Proceedings, vol. 845, pp. 994–997. American Institute of Physics.Google Scholar
Petel, O. E., Mack, D., Higgins, A. J., Turcotte, R. & Chan, S. K.2006 Comparison of the detonation failure mechanism in homogenous and heterogenous explosives. In 13th International Detonation Symposium, pp. 2–11. Office of Naval Research, ONR 351-07-01.Google Scholar
Petel, O. E., Mack, D., Higgins, A. J., Turcotte, R. & Chan, S. K. 2007 Minimum propagation diameter and thickness of high explosives. J. Loss Prev. Process. Ind. 20, 578–583.Google Scholar
Ramsay, J.1985 Effect of confinement on failure in 95 TATB/5 Kel-F. In 8th Symposium (International) on Detonation, pp. 372–379. Naval Surface Weapons Center, NSWC MP 86-194.Google Scholar
Sharpe, G. J. & Bdzil, J. B. 2006 Interactions of inert confiners with explosives. J. Engng Maths 54 (3), 273–298.Google Scholar
Short, M. & Jackson, S. I. 2015 Dynamics of high sound-speed metal confiners driven by non-ideal high-explosive detonation. Combust. Flame 162 (5), 1857–1867.Google Scholar
Short, M., Quirk, J. J., Kiyanda, C. K., Jackson, S. I., Briggs, M. E. & Shinas, M. A.2010 Simulation of detonation of ammonium nitrate fuel oil mixture confined by aluminium: edge angles for DSD. In 14th International Detonation Symposium, pp. 769–778. Office of Naval Research, ONR-351-10-185.Google Scholar
Silvestrov, V. V., Plastinin, A. V., Karakhanov, S. M. & Zykov, V. V. 2008 Critical diameter and critical thickness of an emulsion explosive. Combust. Explos. Shock Waves 44 (3), 354–359.Google Scholar
Stewart, D. S. & Bdzil, J. B. 1988a A lecture on detonation-shock dynamics. In Mathematical Modeling in Combustion Science (ed. Buckmaster, J. D. & Takeno, T.), Lecture Notes in Physics, vol. 299, pp. 17–30. Springer.CrossRefGoogle Scholar
Stewart, D. S. & Bdzil, J. B. 1988b The shock dynamics of stable multidimensional detonation. Combust. Flame 72, 311–323.Google Scholar
Yao, J. & Stewart, D. S. 1996 On the dynamics of multi-dimensional detonation. J. Fluid Mech. 309, 225–275.Google Scholar
Yoshinaka, A. & Zhang, F. 2004 Shock initiation and detonability of liquid nitroethane. In Shock Compression of Condensed Matter (ed. Furnish, M. D., Gupta, Y. M. & Forbes, J. W.), AIP Conference Proceedings, vol. 706, pp. 1069–1072. American Institute of Physics.Google Scholar