Published online by Cambridge University Press: 22 August 2013
We study the nature of archetypal, incompressible, planar splitter-plate wakes, specifically the effects of the exit boundary layer state on multiple approximate self-similarity. Temporally developing direct numerical simulations, at a Reynolds number of 1500 based on the volume-flux defect, are performed to investigate three distinct wake evolution scenarios: Kelvin–Helmholtz transition, bypass transition in an asymmetric wake, and an initially fully turbulent wake. The differences in the evolution and far-wake statistics are analysed in detail. The individual approximately self-similar states exhibit a relative variation of up to 48 % in the spread rate, in second-order statistics, and in peak values of the energy budget terms. The multiplicity of self-similar states is tied to the non-universality of the large-scale coherent structures. These structures maintain the memory of the initial conditions. In the far wake, two distinct spanwise-coherent motions are identified: (i) staggered, segregated spanwise rollers on either side of the centreplane, dominant in wakes transitioning via anti-symmetric instability modes; and, (ii) larger spanwise rollers spanning across the centreplane, emerging in the absence of a near-wake characteristic length scale. The latter structure is characterized by strong spanwise coherence, cross-wake velocity correlations and a larger entrainment rate caused by deep pockets of irrotational fluid within the folds of the turbulent/non-turbulent interface. The mid-sized structures, primarily vortical rods, are generic for all initial conditions and are inclined at ∼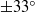 $\pm 3{3}^{\circ } $ to the downstream, shallower than the preferential
$\pm 3{3}^{\circ } $ to the downstream, shallower than the preferential 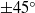 $\pm 4{5}^{\circ } $ inclination of the vorticity vector. The spread rate is driven by the inner-wake dynamics, more specifically the advective flux of spanwise vorticity across the centreplane, which depends on the large-scale coherent structures.
$\pm 4{5}^{\circ } $ inclination of the vorticity vector. The spread rate is driven by the inner-wake dynamics, more specifically the advective flux of spanwise vorticity across the centreplane, which depends on the large-scale coherent structures.