Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Wang, Yang
and
Dong, Gang
2022.
A numerical study of shock-interface interaction and prediction of the mixing zone growth in inhomogeneous medium.
Acta Mechanica Sinica,
Vol. 38,
Issue. 12,
Liang, Yu
and
Luo, Xisheng
2022.
On shock-induced evolution of a gas layer with two fast/slow interfaces.
Journal of Fluid Mechanics,
Vol. 939,
Issue. ,
Abarzhi, Snezhana I.
Hill, Desmon L.
Naveh, Annie
Williams, Kurt C.
and
Wright, Cameron E.
2022.
Supernovae and the Arrow of Time.
Entropy,
Vol. 24,
Issue. 6,
p.
829.
Wang, Hui
Wang, He
Zhai, Zhigang
and
Luo, Xisheng
2022.
Effects of obstacles on shock-induced perturbation growth.
Physics of Fluids,
Vol. 34,
Issue. 8,
Liang, Yu
2022.
Fundamental Studies of Shock-Driven Hydrodynamic Instabilities.
p.
103.
Wang, He
Cao, Qing
Chen, Chenren
Zhai, Zhigang
and
Luo, Xisheng
2022.
Experimental study on a light–heavy interface evolution induced by two successive shock waves.
Journal of Fluid Mechanics,
Vol. 953,
Issue. ,
Liang, Yu
2022.
The phase effect on the Richtmyer–Meshkov instability of a fluid layer.
Physics of Fluids,
Vol. 34,
Issue. 3,
Liang, Yu
Liu, Lili
and
Luo, Xisheng
2022.
Experimental study of bubble competition and spike competition in Richtmyer–Meshkov flows.
Journal of Fluid Mechanics,
Vol. 949,
Issue. ,
Liang, Yu
2022.
Fundamental Studies of Shock-Driven Hydrodynamic Instabilities.
p.
37.
Liang, Yu
and
Luo, Xisheng
2023.
Review on hydrodynamic instabilities of a shocked gas layer.
Science China Physics, Mechanics & Astronomy,
Vol. 66,
Issue. 10,
Xu, Jinru
Wang, He
Zhai, Zhigang
and
Luo, Xisheng
2023.
Convergent Richtmyer–Meshkov instability on two-dimensional dual-mode interfaces.
Journal of Fluid Mechanics,
Vol. 965,
Issue. ,
Liang, Yu
and
Luo, Xisheng
2023.
Hydrodynamic instabilities of two successive slow/fast interfaces induced by a weak shock.
Journal of Fluid Mechanics,
Vol. 955,
Issue. ,
Qin, Jianhua
and
Dong, Guodan
2023.
Anisotropic evolutions of the magnetohydrodynamic Richtmyer-Meshkov instability induced by a converging shock.
Physical Review E,
Vol. 108,
Issue. 5,
Liang, Yu
Liu, Lili
Luo, Xisheng
and
Wen, Chih-Yung
2023.
Hydrodynamic instabilities of a dual-mode air–SF6 interface induced by a cylindrically convergent shock.
Journal of Fluid Mechanics,
Vol. 963,
Issue. ,
Zhang, Duo
Ding, Juchun
Si, Ting
and
Luo, Xisheng
2023.
Divergent Richtmyer–Meshkov instability on a heavy gas layer.
Journal of Fluid Mechanics,
Vol. 959,
Issue. ,
Zhang, Duo
Ding, Juchun
Li, Ming
Zou, Liyong
and
Luo, Xisheng
2023.
Effects of reverberating waves and interface coupling on a divergent Richtmyer–Meshkov instability.
Journal of Fluid Mechanics,
Vol. 975,
Issue. ,
Li, Jiaxuan
Cao, Qing
Wang, He
Zhai, Zhigang
and
Luo, Xisheng
2023.
New interface formation method for shock–interface interaction studies.
Experiments in Fluids,
Vol. 64,
Issue. 11,
Chen, Chenren
Wang, He
Zhai, Zhigang
and
Luo, Xisheng
2024.
Mode coupling between two different interfaces of a gas layer subject to a shock.
Journal of Fluid Mechanics,
Vol. 984,
Issue. ,
Sadler, James D.
Powell, Philip D.
Schalles, Mark
Louie, Carlton
Jacobs, Jeffrey W.
and
Zhou, Ye
2024.
Simulations of three-layer Richtmyer–Meshkov mixing in a shock tube.
Physics of Fluids,
Vol. 36,
Issue. 1,
Zhai, Zhigang
Xu, Jinru
and
Luo, Xisheng
2024.
Convergent Richtmyer-Meshkov instability on two-dimensional tri-mode interfaces.
Science China Physics, Mechanics & Astronomy,
Vol. 67,
Issue. 12,
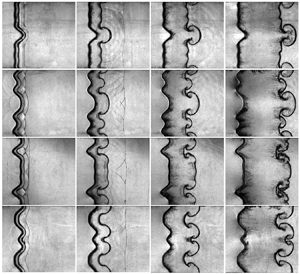
 $_6$ interface with controllable initial conditions are performed to examine the dependence of perturbation growth on initial spectra. We deduce and demonstrate experimentally that the amplitude development of each mode is influenced by the mode-competition effect from quasi-linear stages. It is confirmed that the mode-competition effect is closely related to initial spectra, including the wavenumber, the phase and the initial amplitude of constituent modes. By considering both the mode-competition effect and the high-order harmonics effect, a nonlinear model is established based on initial spectra to predict the amplitude growth of each individual mode. The nonlinear model is validated by the present experiments and data in the literature by considering diverse initial spectra, shock intensities and density ratios. Moreover, the nonlinear model is successfully extended based on the superposition principle to predict the growths of the total perturbation width and the bubble/spike width from quasi-linear to nonlinear stages.
$_6$ interface with controllable initial conditions are performed to examine the dependence of perturbation growth on initial spectra. We deduce and demonstrate experimentally that the amplitude development of each mode is influenced by the mode-competition effect from quasi-linear stages. It is confirmed that the mode-competition effect is closely related to initial spectra, including the wavenumber, the phase and the initial amplitude of constituent modes. By considering both the mode-competition effect and the high-order harmonics effect, a nonlinear model is established based on initial spectra to predict the amplitude growth of each individual mode. The nonlinear model is validated by the present experiments and data in the literature by considering diverse initial spectra, shock intensities and density ratios. Moreover, the nonlinear model is successfully extended based on the superposition principle to predict the growths of the total perturbation width and the bubble/spike width from quasi-linear to nonlinear stages.


