Published online by Cambridge University Press: 30 April 2012
Viscoelastic properties of complex fluids are usually extracted by applying an oscillatory shear rate (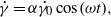 where
where  is a constant which is small for a linear response to make sense) to the fluid. This leads to a complex effective viscosity where its real part carries information on viscous effects while its imaginary part informs us on elastic properties. We show here theoretically, by taking a dilute vesicle suspension as an example, that application of a pure shearing oscillation misses several interesting microscopic features of the suspension. It is shown that if, in addition to the oscillatory part, a basic constant shear rate is applied to the suspension (so that the total shear rate is
is a constant which is small for a linear response to make sense) to the fluid. This leads to a complex effective viscosity where its real part carries information on viscous effects while its imaginary part informs us on elastic properties. We show here theoretically, by taking a dilute vesicle suspension as an example, that application of a pure shearing oscillation misses several interesting microscopic features of the suspension. It is shown that if, in addition to the oscillatory part, a basic constant shear rate is applied to the suspension (so that the total shear rate is 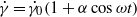 , with
, with  a constant), then the complex viscosity reveals much more insightful properties of the suspension. First, it is found that the complex viscosity exhibits a resonance for tank-treading vesicles as a function of the frequency of oscillation. This resonance is linked to the fact that vesicles, while being in the stable tank-treading regime (with their main axis having a steady orientation with respect to the flow direction), possess damped oscillatory modes. Second, in the region of parameter space where the vesicle exhibits either vacillating-breathing (permanent oscillations of the main axis about the flow direction and breathing of the shape) or tumbling modes, the complex viscosity shows an infinite number of resonances as a function of the frequency. It is shown that these behaviours markedly differ from that obtained when only the classical oscillation
a constant), then the complex viscosity reveals much more insightful properties of the suspension. First, it is found that the complex viscosity exhibits a resonance for tank-treading vesicles as a function of the frequency of oscillation. This resonance is linked to the fact that vesicles, while being in the stable tank-treading regime (with their main axis having a steady orientation with respect to the flow direction), possess damped oscillatory modes. Second, in the region of parameter space where the vesicle exhibits either vacillating-breathing (permanent oscillations of the main axis about the flow direction and breathing of the shape) or tumbling modes, the complex viscosity shows an infinite number of resonances as a function of the frequency. It is shown that these behaviours markedly differ from that obtained when only the classical oscillation  is applied. The results are obtained numerically by solution of the analytical constitutive equation of a dilute vesicle suspension and confirmed analytically by a linear-response phenomenological theory. It is argued that the same type of behaviour is expected for any suspension of soft entities (capsules, red blood cells, etc.) that exhibit periodic motion under constant shear flow. We shall also discuss the reason why this type of behaviour could not have been captured by existing constitutive laws of complex fluids.
is applied. The results are obtained numerically by solution of the analytical constitutive equation of a dilute vesicle suspension and confirmed analytically by a linear-response phenomenological theory. It is argued that the same type of behaviour is expected for any suspension of soft entities (capsules, red blood cells, etc.) that exhibit periodic motion under constant shear flow. We shall also discuss the reason why this type of behaviour could not have been captured by existing constitutive laws of complex fluids.