Published online by Cambridge University Press: 13 February 2024
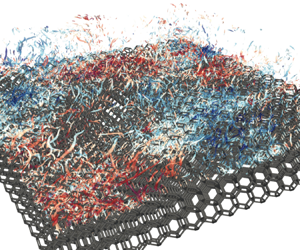
Direct numerical simulations of turbulent flows over highly permeable porous walls were performed at various Reynolds numbers to examine the effects of the Reynolds number on permeable wall turbulence. The porous medium consisted of Kelvin cell arrays with porosity  $0.95$, and the permeability Reynolds number
$0.95$, and the permeability Reynolds number  $Re_K$ ranged from approximately 7 to 50. Simulations with thin and thick porous walls were performed to investigate the effects of spanwise roller vortices associated with the Kelvin–Helmholtz instability. The results show that the effect of the Kelvin–Helmholtz instability becomes more significant with increasing the permeability Reynolds number, and spanwise rollers, for which length scale is an order of channel height, dominate turbulence when
$Re_K$ ranged from approximately 7 to 50. Simulations with thin and thick porous walls were performed to investigate the effects of spanwise roller vortices associated with the Kelvin–Helmholtz instability. The results show that the effect of the Kelvin–Helmholtz instability becomes more significant with increasing the permeability Reynolds number, and spanwise rollers, for which length scale is an order of channel height, dominate turbulence when  $Re_K \gtrsim 30$. Spanwise rollers reinforce the negative correlation between the wall-normal and streamwise velocity fluctuations close to the porous/fluid interface, and intensify the turbulent velocity fluctuations away from the porous walls, leading to increased frictional resistance. An investigation of the Reynolds number dependence of the modified logarithmic law indicates that the zero-plane displacement and equivalent roughness height are proportional to the square root of permeability, whereas the von Kármán constant increases with the permeability Reynolds number because of the increased mixing length resulting from the relatively large-scale velocity fluctuations induced by spanwise rollers. We developed a model for the modified log law for permeable wall turbulence based on permeability, and confirmed that the skin friction coefficient obtained from the model reasonably predicts the skin friction coefficient for several types of high-porosity porous media. Hence, permeability is a key parameter that characterizes the logarithmic mean velocity profiles over a variety of porous media with high porosity.
$Re_K \gtrsim 30$. Spanwise rollers reinforce the negative correlation between the wall-normal and streamwise velocity fluctuations close to the porous/fluid interface, and intensify the turbulent velocity fluctuations away from the porous walls, leading to increased frictional resistance. An investigation of the Reynolds number dependence of the modified logarithmic law indicates that the zero-plane displacement and equivalent roughness height are proportional to the square root of permeability, whereas the von Kármán constant increases with the permeability Reynolds number because of the increased mixing length resulting from the relatively large-scale velocity fluctuations induced by spanwise rollers. We developed a model for the modified log law for permeable wall turbulence based on permeability, and confirmed that the skin friction coefficient obtained from the model reasonably predicts the skin friction coefficient for several types of high-porosity porous media. Hence, permeability is a key parameter that characterizes the logarithmic mean velocity profiles over a variety of porous media with high porosity.