Published online by Cambridge University Press: 26 May 2023
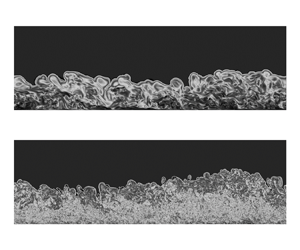
Direct numerical simulations (DNS) of temporally developing turbulent boundary layers are performed with a wide range of Reynolds numbers based on the momentum thickness  $Re_{\theta } = 2000$–
$Re_{\theta } = 2000$– $13\,000$ for investigating the Reynolds number dependence of the turbulent/non-turbulent interface (TNTI) layer. The grid spacing in the DNS is determined carefully such that small-scale turbulent motions near the TNTI are well resolved. The outer edge of the TNTI layer, called the irrotational boundary, is detected with vorticity magnitude. The mean thicknesses of the TNTI layer,
$13\,000$ for investigating the Reynolds number dependence of the turbulent/non-turbulent interface (TNTI) layer. The grid spacing in the DNS is determined carefully such that small-scale turbulent motions near the TNTI are well resolved. The outer edge of the TNTI layer, called the irrotational boundary, is detected with vorticity magnitude. The mean thicknesses of the TNTI layer,  $\delta _{TNTI}$, turbulent sublayer,
$\delta _{TNTI}$, turbulent sublayer,  $\delta _{TSL}$, and viscous superlayer,
$\delta _{TSL}$, and viscous superlayer,  $\delta _{VSL}$, are found to be approximately
$\delta _{VSL}$, are found to be approximately  $15\eta _{TI}$,
$15\eta _{TI}$,  $10\eta _{TI}$ and
$10\eta _{TI}$ and  $5\eta _{TI}$, respectively, where
$5\eta _{TI}$, respectively, where  $\eta _{TI}$ is the Kolmogorov scale taken in the turbulent region near the TNTI layer. The mean curvature of the irrotational boundary is also characterized by
$\eta _{TI}$ is the Kolmogorov scale taken in the turbulent region near the TNTI layer. The mean curvature of the irrotational boundary is also characterized by  $\eta _{TI}$. The shear parameter and the shear-to-vorticity ratio show that the mean shear effects near the TNTI layer are not significant for both large and small scales. The anisotropy tensors of Reynolds stress and vorticity suggest that the turbulence under the TNTI layer tends to be isotropic at high
$\eta _{TI}$. The shear parameter and the shear-to-vorticity ratio show that the mean shear effects near the TNTI layer are not significant for both large and small scales. The anisotropy tensors of Reynolds stress and vorticity suggest that the turbulence under the TNTI layer tends to be isotropic at high  $Re_{\theta }$, for which
$Re_{\theta }$, for which  $\eta _{TI}/\delta \sim Re_{\theta }^{-3/4}$ is valid with the boundary layer thickness
$\eta _{TI}/\delta \sim Re_{\theta }^{-3/4}$ is valid with the boundary layer thickness  $\delta$. The surface area of the irrotational boundary is consistent with the fractal analysis of the interface, where the fractal dimension
$\delta$. The surface area of the irrotational boundary is consistent with the fractal analysis of the interface, where the fractal dimension  $D_f$ is found to be
$D_f$ is found to be  $2.14$–
$2.14$– $2.20$. The present results suggest that the mean entrainment rate per unit horizontal area normalized by the friction velocity varies slowly as
$2.20$. The present results suggest that the mean entrainment rate per unit horizontal area normalized by the friction velocity varies slowly as  $Re_{\theta }^{(3/4)(D_f-2)}$ for
$Re_{\theta }^{(3/4)(D_f-2)}$ for  $Re_{\theta } \geq 4000$.
$Re_{\theta } \geq 4000$.