1. Introduction and aim of the work
Over decades and still continuing, the two-point velocity–velocity and velocity–pressure gradient correlation experiments are of fundamental importance in theories of turbulence and understanding dynamics of wall-bounded (i.e. boundary layer, pipe and plane channel) shear flows; see e.g. Townsend (Reference Townsend1956), Monin & Yaglom (Reference Monin and Yaglom1971), Cantwell (Reference Cantwell1981), Oberlack & Peters (Reference Oberlack and Peters1993), Jovanović, Ye & Durst (Reference Jovanović, Ye and Durst1995), Jovanović (Reference Jovanović2004), Wallace (Reference Wallace2014) and Chen (Reference Chen2019). Among the three classes of wall-bounded shear flows, an increasing interest addressing pipe flow structures requires velocity and pressure data with high enough spatial and temporal resolutions using optical, thermal and pressure probes; e.g. Hellström et al. (Reference Hellström, Sinha and Smits2011) and Monty et al. (Reference Monty, Stewart, Williams and Chong2007). The correlations, probability density functions and power spectra of such velocity and pressure signals represent vital statistical tools to analyse the structure of the organized motions of turbulent pipe flow data. The nature of such organized motions – in particular, their spatial details and dynamical significance – was addressed in various papers; see e.g. Theodorsen (Reference Theodorsen1952), Townsend (Reference Townsend1956), Lumley (Reference Lumley1967), Sabot, Renault & Comte-Bellot (Reference Sabot, Renault and Comte-Bellot1973), Cantwell (Reference Cantwell1981), Hussain (Reference Hussain1983, Reference Hussain1986), Robinson (Reference Robinson1991), Wark & Nagib (Reference Wark and Nagib1991), Tomkins & Adrian (Reference Tomkins and Adrian2003), Monty et al. (Reference Monty, Stewart, Williams and Chong2007), Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008), Bailey & Smits (Reference Bailey and Smits2010), Smits, McKeon & Marusic (Reference Smits, McKeon and Marusic2011a) and Chung et al. (Reference Chung, Marusic, Monty, Vallikivi and Smits2015). Nevertheless, a concrete definition of the origin, nature and time evolution of the coherent structures is still under debate (see e.g. Marusic et al. Reference Marusic, McKeon, Monkewitz, Nagib, Smits and Sreenivasan2010; Jiménez Reference Jiménez2018), motivating further investigations to properly characterize the large-scale coherent motions, in particular at high Reynolds numbers.
The early work to isolate the coherent features of the large-scale motions in turbulent boundary layer flows using the temporal correlation of the streamwise velocity component goes back to Townsend (Reference Townsend1956) and Grant (Reference Grant1958). Townsend (Reference Townsend1956) defined the large-scale motions as energetic structures that are characterized by a long tail on the temporal correlation of the streamwise velocity fluctuations, implying correspondingly long structures in the streamwise direction in the buffer layer where low-speed streaks are revealed, as well as throughout the logarithmic layer and the core/wake region.
Using spatio-temporal correlation, further studies have been carried out – see Hussain (Reference Hussain1983), Blackwelder (Reference Blackwelder1988), Fiedler (Reference Fiedler1988), Robinson (Reference Robinson1991) and Holmes et al. (Reference Holmes, Lumley, Berkooz and Rowley1996) – providing further definitions for such large-scale organized motions. Robinson (Reference Robinson1991, p. 602), for instance, defined the organized flow structures as ‘a three-dimensional region of the flow over which at least one fundamental flow variable (velocity, pressure, temperature, etc.) exhibits significant correlation with itself or with another variable over a range of space and/or time that is significantly larger than the smallest local scales of the flow’, motivating studies of turbulent pipe flow structures using two-point velocity correlations; e.g. Monty et al. (Reference Monty, Stewart, Williams and Chong2007), Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008), Baidya et al. (Reference Baidya2019) and Han et al. (Reference Han, Hwang, Yoon, Ahn and Sung2019).
Monty et al. (Reference Monty, Stewart, Williams and Chong2007) investigated the azimuthal scales of the large-scale motions in pipe flow through the two-point velocity correlations measured, utilizing a rake of hot-wire probes fixed at the top of the logarithmic region. They claimed that the azimuthal structures in pipe flow were nearly identical to those determined from similar measurements in plane channel and boundary layer flows. However, due to the geometrical considerations of a pipe when compared with either plane channel and/or flat-plate boundary layer flows, its flow structure might be different; see Holmes et al. (Reference Holmes, Lumley, Berkooz, Mattinglya and Wittenberg1997), Kim & Adrian (Reference Kim and Adrian1999), Marusic et al. (Reference Marusic, McKeon, Monkewitz, Nagib, Smits and Sreenivasan2010) and Chung et al. (Reference Chung, Marusic, Monty, Vallikivi and Smits2015). Chung et al. (Reference Chung, Marusic, Monty, Vallikivi and Smits2015) provided a good explanation for the geometrical consideration effects, revealing subtle differences between the boundary layer and the pipe flow in terms of the structure functions for the streamwise turbulence intensity profile at high Reynolds numbers versus the wall distance, in particular in the logarithmic layer. Marusic et al. (Reference Marusic, McKeon, Monkewitz, Nagib, Smits and Sreenivasan2010) attributed also the quantitative differences between the two classes of flows to the interaction with the opposite wall in internal flows, i.e. pipe and channel, and to the intermittency of the outer region in boundary layers, but concluded that it remains a matter of speculation. Therefore, the finding of Monty et al. (Reference Monty, Stewart, Williams and Chong2007) cannot be generalized since it was based on a single fixed wall-normal position, motivating Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008) to further study changes in the azimuthal scales of the pipe flow.
The objective of the Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008) study was to investigate the wall-normal dependence of the azimuthal scales of the large-scale and very-large-scale structures within the wall-normal distances ![]() $0.1\le {x_2}/R\le {0.5}$ for a large range of Reynolds number
$0.1\le {x_2}/R\le {0.5}$ for a large range of Reynolds number ![]() $7.6\times {10^4}\le {Re_{b}}\le {8.3\times {10^6}}$ based on the pipe diameter. The experiments by Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008) were conducted with two single hot-wire probes calibrated where the pipe flow was assured to be fully developed turbulent. They calibrated both hot-wire probes using a Pitot probe fixed at the pipe centreline, while the two probes were positioned offset from the pipe centreline, yielding second-order statistics within 10 % uncertainty. The uncertainty level would be even higher if the hot-wire probes were moved towards the vicinity of the pipe wall where the turbulence level is higher, in particular at large Reynolds number. The high uncertainty level in the calibration equations of both hot-wire probes utilized weakened their dynamic responses to small velocity fluctuations that would give rise to substantial levels of deviation in the conclusions drawn (Talluru, Kulandaivelu & Hutchins Reference Talluru, Kulandaivelu and Hutchins2014; Boufidi, Lavagnoli & Fontaneto Reference Boufidi, Lavagnoli and Fontaneto2020). This could result, for instance, in an improper effect of the Reynolds number on scaling the azimuthal large-scale structures.
$7.6\times {10^4}\le {Re_{b}}\le {8.3\times {10^6}}$ based on the pipe diameter. The experiments by Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008) were conducted with two single hot-wire probes calibrated where the pipe flow was assured to be fully developed turbulent. They calibrated both hot-wire probes using a Pitot probe fixed at the pipe centreline, while the two probes were positioned offset from the pipe centreline, yielding second-order statistics within 10 % uncertainty. The uncertainty level would be even higher if the hot-wire probes were moved towards the vicinity of the pipe wall where the turbulence level is higher, in particular at large Reynolds number. The high uncertainty level in the calibration equations of both hot-wire probes utilized weakened their dynamic responses to small velocity fluctuations that would give rise to substantial levels of deviation in the conclusions drawn (Talluru, Kulandaivelu & Hutchins Reference Talluru, Kulandaivelu and Hutchins2014; Boufidi, Lavagnoli & Fontaneto Reference Boufidi, Lavagnoli and Fontaneto2020). This could result, for instance, in an improper effect of the Reynolds number on scaling the azimuthal large-scale structures.
Measurement uncertainty is thus of importance for evaluating turbulence statistics and their dependence on the Reynolds number; see Appendix A. Therefore, we adopted a unique in situ calibration approach that provided calibration equations for hot-wire probes with least-squares error better than ![]() ${\pm }1\,\%$, resulting in very accurate experimental pipe data. Our main objective is to study, precisely, the effect of wider wall-normal distances
${\pm }1\,\%$, resulting in very accurate experimental pipe data. Our main objective is to study, precisely, the effect of wider wall-normal distances ![]() ${0.1}\le {x_{{2}}/R}\le {0.7}$ and azimuthal space separations
${0.1}\le {x_{{2}}/R}\le {0.7}$ and azimuthal space separations ![]() ${10^{\circ }}\le {{{\Delta }}}{\theta }\le {210^{\circ }}$ on the velocity correlation
${10^{\circ }}\le {{{\Delta }}}{\theta }\le {210^{\circ }}$ on the velocity correlation ![]() $R_{u_{{1}}u_{{1}}}({{{\Delta }}}s, t)$, the cross-power spectral density
$R_{u_{{1}}u_{{1}}}({{{\Delta }}}s, t)$, the cross-power spectral density ![]() $G({{{\Delta }}}s, k_{x_{{1}}})$ and the coherence function
$G({{{\Delta }}}s, k_{x_{{1}}})$ and the coherence function ![]() $\gamma ({{{\Delta }}}s, k_{x_{{1}}})$, and consequently on pipe flow azimuthal scales of the large- and very-large-scale structures over the relatively large Reynolds number range
$\gamma ({{{\Delta }}}s, k_{x_{{1}}})$, and consequently on pipe flow azimuthal scales of the large- and very-large-scale structures over the relatively large Reynolds number range ![]() $2\times {10^3}\le {Re_\tau }\le {16\times {10^3}}$.
$2\times {10^3}\le {Re_\tau }\le {16\times {10^3}}$.
Two-point joint statistics were adopted to investigate the effects of the various parameters discussed earlier and the effect of scale averaging on the azimuthal scales of the large-scale flow motions. The two-point spatial correlation of the streamwise velocity fluctuations ![]() $R_{u_{{1}}u_{{1}}}({{\Delta }}{s},t)$ is therefore of fundamental interest in the present study for the statistical analysis of the azimuthal pipe flow data. It is defined for two locations at the same time instant
$R_{u_{{1}}u_{{1}}}({{\Delta }}{s},t)$ is therefore of fundamental interest in the present study for the statistical analysis of the azimuthal pipe flow data. It is defined for two locations at the same time instant ![]() $t$ as
$t$ as
where ![]() ${\langle }{{\cdot }}{\rangle }$ denotes an ensemble average of velocity fluctuating signals,
${\langle }{{\cdot }}{\rangle }$ denotes an ensemble average of velocity fluctuating signals, ![]() ${{{\Delta }}}{s}$ is the azimuthal space separation, and
${{{\Delta }}}{s}$ is the azimuthal space separation, and ![]() $u_{{1}}(s,t)$ is the streamwise velocity fluctuation as a function of space (
$u_{{1}}(s,t)$ is the streamwise velocity fluctuation as a function of space (![]() $s$) and time (
$s$) and time (![]() $t$). The use of the two-point velocity correlations
$t$). The use of the two-point velocity correlations ![]() $R_{u_{{1}}u_{{1}}}({s+{{\Delta }}}{s},t)$ to address the azimuthal structures of turbulence in pipe flow requires either using an optical measuring technique such as particle image velocimetry and/or multiple thermal hot-wire probes. The multiple hot-wire probes separated in space are in common use to characterize the coherence structures in wall-bounded shear flows as reported earlier (see e.g. Monty et al. Reference Monty, Stewart, Williams and Chong2007; Bailey et al. Reference Bailey, Hultmark, Smits and Schultz2008), providing highly temporal-resolved velocity data. However, when the near-wall motions are targeted, the length
$R_{u_{{1}}u_{{1}}}({s+{{\Delta }}}{s},t)$ to address the azimuthal structures of turbulence in pipe flow requires either using an optical measuring technique such as particle image velocimetry and/or multiple thermal hot-wire probes. The multiple hot-wire probes separated in space are in common use to characterize the coherence structures in wall-bounded shear flows as reported earlier (see e.g. Monty et al. Reference Monty, Stewart, Williams and Chong2007; Bailey et al. Reference Bailey, Hultmark, Smits and Schultz2008), providing highly temporal-resolved velocity data. However, when the near-wall motions are targeted, the length ![]() $\ell$ of the sensing element of the hot-wire probe (see table 1) is an important issue to be considered, in particular at high Reynolds numbers; see Blackwelder & Haritonidis (Reference Blackwelder and Haritonidis1983), Ligrani & Bradshaw (Reference Ligrani and Bradshaw1987), Wei & Willmarth (Reference Wei and Willmarth1989) and Gad-el-Hak & Bandyopadhyay (Reference Gad-el-Hak and Bandyopadhyay1994). At high Reynolds numbers, the turbulence viscous length scale
$\ell$ of the sensing element of the hot-wire probe (see table 1) is an important issue to be considered, in particular at high Reynolds numbers; see Blackwelder & Haritonidis (Reference Blackwelder and Haritonidis1983), Ligrani & Bradshaw (Reference Ligrani and Bradshaw1987), Wei & Willmarth (Reference Wei and Willmarth1989) and Gad-el-Hak & Bandyopadhyay (Reference Gad-el-Hak and Bandyopadhyay1994). At high Reynolds numbers, the turbulence viscous length scale ![]() $\ell _{c}=\nu /u_{\tau }$ is much smaller than probe size. The measured velocity signals are thus attenuated, and consequently the small-scale turbulence is not well resolved because of the averaging process. Blackwelder & Haritonidis (Reference Blackwelder and Haritonidis1983) suggested sensors having a spatial scale that is less than twenty viscous length scales to provide experimental data free from spatial averaging effects. This physical constraint, combined with the requirement for a hot-wire length-to-diameter ratio
$\ell _{c}=\nu /u_{\tau }$ is much smaller than probe size. The measured velocity signals are thus attenuated, and consequently the small-scale turbulence is not well resolved because of the averaging process. Blackwelder & Haritonidis (Reference Blackwelder and Haritonidis1983) suggested sensors having a spatial scale that is less than twenty viscous length scales to provide experimental data free from spatial averaging effects. This physical constraint, combined with the requirement for a hot-wire length-to-diameter ratio ![]() $\ell /d\approx {250}$, limited turbulence–structure experiments using commercial hot-wire probes at high Reynolds numbers. To account for such inadequate spatial and temporal resolutions of hot-wire probes, correction techniques for the streamwise velocity fluctuations proposed by Hutchins et al. (Reference Hutchins, Nickels, Marusic and Chong2009), Smits et al. (Reference Smits, Monty, HUltmark, Bailey, Hutchins and Marusic2011b) and Hutchins, Monty & Hultmark (Reference Hutchins, Monty and Hultmark2015) for a wide range of the Reynolds number and wire lengths were adopted with success in the present study. Table 1 summarizes the relevant parameters from experiments performed and presented in the paper. The main sections of this paper are summarized as follows. Section 1 highlighted briefly the state of the art of the velocity correlation in wall-bounded flows, in particular in pipe flow. The experimental facility and the hot-wire module are presented in § 2. Section 3 presents the resultant data over a relatively wide range of Reynolds numbers. Section 4 summarizes the outcome of the present study with some concluding remarks. Appendices A and B are presented at the end of the paper. Overall, this paper is intended to provide a good insight into the characteristics of azimuthal and streamwise pipe flow structures, using two-point velocity correlations and spectral analysis.
$\ell /d\approx {250}$, limited turbulence–structure experiments using commercial hot-wire probes at high Reynolds numbers. To account for such inadequate spatial and temporal resolutions of hot-wire probes, correction techniques for the streamwise velocity fluctuations proposed by Hutchins et al. (Reference Hutchins, Nickels, Marusic and Chong2009), Smits et al. (Reference Smits, Monty, HUltmark, Bailey, Hutchins and Marusic2011b) and Hutchins, Monty & Hultmark (Reference Hutchins, Monty and Hultmark2015) for a wide range of the Reynolds number and wire lengths were adopted with success in the present study. Table 1 summarizes the relevant parameters from experiments performed and presented in the paper. The main sections of this paper are summarized as follows. Section 1 highlighted briefly the state of the art of the velocity correlation in wall-bounded flows, in particular in pipe flow. The experimental facility and the hot-wire module are presented in § 2. Section 3 presents the resultant data over a relatively wide range of Reynolds numbers. Section 4 summarizes the outcome of the present study with some concluding remarks. Appendices A and B are presented at the end of the paper. Overall, this paper is intended to provide a good insight into the characteristics of azimuthal and streamwise pipe flow structures, using two-point velocity correlations and spectral analysis.
Table 1. Summary of the present main experimental parameters: ![]() $U_{{b}}$ is the bulk velocity,
$U_{{b}}$ is the bulk velocity, ![]() ${Re_b}$ is the bulk Reynolds number,
${Re_b}$ is the bulk Reynolds number, ![]() $u_{\tau }$ is the wall friction velocity,
$u_{\tau }$ is the wall friction velocity, ![]() ${Re_{\tau }}$ is the shear Reynolds number,
${Re_{\tau }}$ is the shear Reynolds number, ![]() $\ell ^+=\ell {u_{\tau }}/\nu$ is the hot-wire length in wall units,
$\ell ^+=\ell {u_{\tau }}/\nu$ is the hot-wire length in wall units, ![]() $\ell _c$ is the viscous length scale,
$\ell _c$ is the viscous length scale, ![]() $T$ is the sampling time, and
$T$ is the sampling time, and ![]() $S_s$ is the sampling size.
$S_s$ is the sampling size.

2. Experimental set-up and hot-wire calibration
2.1. The pipe facility
Experiments were carried out utilizing the large pipe facility (CoLaPipe) located at Brandenburg University of Technology Cottbus–Senftenberg. The CoLaPipe (figure 1a) is a closed-return facility equipped with a water cooler to keep the air temperature constant inside the pipe test section. The facility allows measurements for shear Reynolds number ![]() ${Re}_{\tau }$ in the range
${Re}_{\tau }$ in the range ![]() ${8\times {10^2}}\le {Re}_{\tau }\le {2\times {10^4}}$, where
${8\times {10^2}}\le {Re}_{\tau }\le {2\times {10^4}}$, where ![]() ${Re}_{\tau }$ is defined as
${Re}_{\tau }$ is defined as ![]() ${Re}_{\tau }=R/\ell _c$,
${Re}_{\tau }=R/\ell _c$, ![]() $R$ is the pipe radius,
$R$ is the pipe radius, ![]() $\ell _c=\nu /u_\tau$ is the viscous length scale,
$\ell _c=\nu /u_\tau$ is the viscous length scale, ![]() $u_\tau$ is the wall friction velocity, and
$u_\tau$ is the wall friction velocity, and ![]() $\nu$ is the fluid kinematic viscosity. At the pipe inlet, i.e.
$\nu$ is the fluid kinematic viscosity. At the pipe inlet, i.e. ![]() $x_{{1}}/D=0$, the facility provides air with uniform profiles, i.e.
$x_{{1}}/D=0$, the facility provides air with uniform profiles, i.e. ![]() $\bar {U}_{{1}}(r)\approx \text {constant}$ (figure 2), having turbulence level
$\bar {U}_{{1}}(r)\approx \text {constant}$ (figure 2), having turbulence level ![]() $\sqrt {\overline {u_{{1}}}^2}/\bar {U}_{{1}}\le {0.5\,\%}$ (figure 3), where
$\sqrt {\overline {u_{{1}}}^2}/\bar {U}_{{1}}\le {0.5\,\%}$ (figure 3), where ![]() $x_1$ is the streamwise distance,
$x_1$ is the streamwise distance, ![]() ${D}$ is the pipe inner diameter,
${D}$ is the pipe inner diameter, ![]() $\bar {U}_{{1}}$ is the local mean streamwise velocity, and
$\bar {U}_{{1}}$ is the local mean streamwise velocity, and ![]() $r$ is the local radial distance. The facility has suction and return pipe sections made out of high-precision smooth acrylic glass with inner diameters
$r$ is the local radial distance. The facility has suction and return pipe sections made out of high-precision smooth acrylic glass with inner diameters ![]() $190\pm 0.23$ mm and
$190\pm 0.23$ mm and ![]() $342\pm 0.35$ mm, respectively. The total length
$342\pm 0.35$ mm, respectively. The total length ![]() $L$ of each pipe test section is 26 m, providing length-to-diameter ratios
$L$ of each pipe test section is 26 m, providing length-to-diameter ratios ![]() $L/D$ of 136 and 77 for the suction and return sections, respectively. Hot-wire anemometry represents the major instrumentation used to carry out measurements. The two hot-wire probe module (figure 1b) is utilized to measure the streamwise velocity component at different radial and azimuthal positions. The probe module (figure 1b) hosts two hot-wire probes: one probe moves radially, while the other moves in both the radial and the azimuthal directions via a rotating pipe section. Both probe holders are engraved with mm scales to facilitate locating both probes at various wall-normal locations. The rotational pipe section is scaled as well for various azimuthal positions. The azimuthal displacement
$L/D$ of 136 and 77 for the suction and return sections, respectively. Hot-wire anemometry represents the major instrumentation used to carry out measurements. The two hot-wire probe module (figure 1b) is utilized to measure the streamwise velocity component at different radial and azimuthal positions. The probe module (figure 1b) hosts two hot-wire probes: one probe moves radially, while the other moves in both the radial and the azimuthal directions via a rotating pipe section. Both probe holders are engraved with mm scales to facilitate locating both probes at various wall-normal locations. The rotational pipe section is scaled as well for various azimuthal positions. The azimuthal displacement ![]() ${{{\Delta }}}{s}$ is calculated based on the angular separation
${{{\Delta }}}{s}$ is calculated based on the angular separation ![]() ${{{\Delta }}}{\theta }$ desired and the local radial distance
${{{\Delta }}}{\theta }$ desired and the local radial distance ![]() $r$;
$r$; ![]() ${{{\Delta }}}{s}/r=({{{\Delta }}}{\theta })\times {2{\rm \pi} /360}$. In this work, all two-point velocity correlations were calculated based on data obtained with the radial probe kept fixed at a preselected pipe wall-normal location while the radial azimuthal probe, located at the same wall-normal distance, was displaced 35–41 times azimuthally between
${{{\Delta }}}{s}/r=({{{\Delta }}}{\theta })\times {2{\rm \pi} /360}$. In this work, all two-point velocity correlations were calculated based on data obtained with the radial probe kept fixed at a preselected pipe wall-normal location while the radial azimuthal probe, located at the same wall-normal distance, was displaced 35–41 times azimuthally between ![]() $10^{\circ }$ minimum and
$10^{\circ }$ minimum and ![]() $210^{\circ }$ maximum separation with step
$210^{\circ }$ maximum separation with step ![]() ${{{\Delta }}}{\theta }=5^{\circ }$ with respect to the fixed radial probe.
${{{\Delta }}}{\theta }=5^{\circ }$ with respect to the fixed radial probe.

Figure 1. (a) The Cottbus large pipe facility. (b) The two hot-wire probe module. Here, ![]() $CR$ is the nozzle contraction ratio, CTA means constant temperature anemometry,
$CR$ is the nozzle contraction ratio, CTA means constant temperature anemometry, ![]() ${D}$ is the pipe inner diameter,
${D}$ is the pipe inner diameter, ![]() ${D_{exit}}$ is the contraction exit diameter,
${D_{exit}}$ is the contraction exit diameter, ![]() ${D_{in}}$ is the contraction base diameter,
${D_{in}}$ is the contraction base diameter, ![]() ${D_s}$ is the settling chamber inner diameter,
${D_s}$ is the settling chamber inner diameter, ![]() $ER$ is the diffuser expansion ratio,
$ER$ is the diffuser expansion ratio, ![]() ${L}$ is the pipe/contraction/settling chamber length,
${L}$ is the pipe/contraction/settling chamber length, ![]() $P$ is the power, and
$P$ is the power, and ![]() $\Delta {p}$ is the pressure rise.
$\Delta {p}$ is the pressure rise.

Figure 2. Selected normalized mean velocity profile ![]() ${\bar {U}_{{1}}(r)/\bar {U}_{{b}}}$ measured at
${\bar {U}_{{1}}(r)/\bar {U}_{{b}}}$ measured at ![]() $x_1/D=0$, using a 2 mm Pitot-static tube, versus the local wall distance
$x_1/D=0$, using a 2 mm Pitot-static tube, versus the local wall distance ![]() $r/R$ for bulk Reynolds number
$r/R$ for bulk Reynolds number ![]() ${Re_b=10^5}$ (
${Re_b=10^5}$ (![]() ${Re_\tau }\approx {2.2\times {10^3}}$).
${Re_\tau }\approx {2.2\times {10^3}}$).

Figure 3. The centreline turbulence level ![]() $\sqrt {\overline {u_{{1}}}^2}/\bar {U}_{{1}}$ measured by hot-wire probe at the pipe inlet for bulk Reynolds numbers
$\sqrt {\overline {u_{{1}}}^2}/\bar {U}_{{1}}$ measured by hot-wire probe at the pipe inlet for bulk Reynolds numbers ![]() ${Re_b\le {2.4\times }10^5}$ (
${Re_b\le {2.4\times }10^5}$ (![]() ${Re_\tau }\le {5.2\times {10^3}}$).
${Re_\tau }\le {5.2\times {10^3}}$).
All experiments in the present study were conducted at four wall-normal locations ![]() ${x_{{2}}/R}$, namely 0.1, 0.2, 0.5 and 0.7 measured from the pipe wall. The wall-normal locations were chosen to have
${x_{{2}}/R}$, namely 0.1, 0.2, 0.5 and 0.7 measured from the pipe wall. The wall-normal locations were chosen to have ![]() ${x_{{2}}/R}=0.1$ inside the logarithmic region,
${x_{{2}}/R}=0.1$ inside the logarithmic region, ![]() ${x_{{2}}/R}=0.2$ at the top of the logarithmic region, and
${x_{{2}}/R}=0.2$ at the top of the logarithmic region, and ![]() ${x_{{2}}/R}=0.5$ and 0.7 in the core region of the pipe. Measurements were performed using Dantec Streamline 9091N0102 constant temperature anemometry (CTA) with commercial Dantec probes, having a sensing element with dimensions
${x_{{2}}/R}=0.5$ and 0.7 in the core region of the pipe. Measurements were performed using Dantec Streamline 9091N0102 constant temperature anemometry (CTA) with commercial Dantec probes, having a sensing element with dimensions ![]() $\ell \times {d}={1250}\,{{\mathrm {\mu }}{{\rm m}}}\times {5}\,{{\mathrm {\mu }{{\rm m}}}}$, where
$\ell \times {d}={1250}\,{{\mathrm {\mu }}{{\rm m}}}\times {5}\,{{\mathrm {\mu }{{\rm m}}}}$, where ![]() $\ell$ is the sensor length, and
$\ell$ is the sensor length, and ![]() $d$ stands for the sensor diameter. To account for inadequate spatial resolutions of both hot-wire probes, the correction technique proposed by Hutchins et al. (Reference Hutchins, Nickels, Marusic and Chong2009) for the streamwise velocity fluctuations was adopted with success in the present study. Experiments were conducted with a 30 kHz sampling frequency, providing high enough time-resolved velocity data.
$d$ stands for the sensor diameter. To account for inadequate spatial resolutions of both hot-wire probes, the correction technique proposed by Hutchins et al. (Reference Hutchins, Nickels, Marusic and Chong2009) for the streamwise velocity fluctuations was adopted with success in the present study. Experiments were conducted with a 30 kHz sampling frequency, providing high enough time-resolved velocity data.
2.2. Hot-wire calibration
Accurate and individual calibration for both hot-wire probes using an appropriate calibration technique is desirable for high-fidelity experimental data. The calibration is to be carried out in a well-defined flow field, i.e. the profile of the local mean velocity ![]() $\bar {U}_{{1}}(r)$ is uniform (figure 2), with a low turbulence level
$\bar {U}_{{1}}(r)$ is uniform (figure 2), with a low turbulence level ![]() $\sqrt {\overline {u_{{1}}}^2}/\bar {U}_{{1}}\le 0.5\,\%$ (figure 3), whether in situ or ex situ calibration is proposed. The ex situ calibration might be adopted if the above calibration conditions cannot be fulfilled in the facility that is to be utilized later to carry out further experiments. The ex situ calibration, however, has several disadvantages. For instance, the contraction's exit diameter of an external calibrator might not be large enough to host, simultaneously, two hot-wire probes. Thus a sizeable blockage by the two probes in front of the flow field is expected, causing considerable error due to aerodynamic disturbances. In addition, after the completion of the ex situ calibration, transferring and integrating both probes into the pipe test section might not be possible.
$\sqrt {\overline {u_{{1}}}^2}/\bar {U}_{{1}}\le 0.5\,\%$ (figure 3), whether in situ or ex situ calibration is proposed. The ex situ calibration might be adopted if the above calibration conditions cannot be fulfilled in the facility that is to be utilized later to carry out further experiments. The ex situ calibration, however, has several disadvantages. For instance, the contraction's exit diameter of an external calibrator might not be large enough to host, simultaneously, two hot-wire probes. Thus a sizeable blockage by the two probes in front of the flow field is expected, causing considerable error due to aerodynamic disturbances. In addition, after the completion of the ex situ calibration, transferring and integrating both probes into the pipe test section might not be possible.
An alternative approach to an ex situ calibration was adopted by Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008), conducting in situ calibration at a pipe location where flow was assured to be fully developed turbulent and actual measurements were carried out. It is known, however, that the centreline turbulence level ![]() $\sqrt {\overline {u_{{1}}}^2}/\bar {U}_{{1}}$, as figure 4 shows, for fully developed turbulent pipe flow is approximately 3.5 %, i.e.
$\sqrt {\overline {u_{{1}}}^2}/\bar {U}_{{1}}$, as figure 4 shows, for fully developed turbulent pipe flow is approximately 3.5 %, i.e. ![]() $\approx$10 times greater than the turbulence level at the pipe inlet test section; see figure 3 and Hinze (Reference Hinze1975). To perform such in situ calibration for two hot-wire probes, simultaneously, using a Pitot probe located at the pipe centreline, both probes must be positioned offset from the pipe centreline, where the turbulence level is greater than 3.5 % depending on the offset in the wall-normal direction; see figure 4 and table 2.
$\approx$10 times greater than the turbulence level at the pipe inlet test section; see figure 3 and Hinze (Reference Hinze1975). To perform such in situ calibration for two hot-wire probes, simultaneously, using a Pitot probe located at the pipe centreline, both probes must be positioned offset from the pipe centreline, where the turbulence level is greater than 3.5 % depending on the offset in the wall-normal direction; see figure 4 and table 2.

Figure 4. The local turbulence level ![]() $\sqrt {\overline {u_{{1}}}^2}/\bar {U}_{{1}}$ in fully developed turbulent pipe flow regime using a single hot-wire probe for
$\sqrt {\overline {u_{{1}}}^2}/\bar {U}_{{1}}$ in fully developed turbulent pipe flow regime using a single hot-wire probe for ![]() ${Re}_{\tau }\approx {3650}$.
${Re}_{\tau }\approx {3650}$.
Table 2. The local turbulence level ![]() $\sqrt {\overline {u_{{1}}}^2}/\bar {U}_{{1}}$ in fully developed turbulent pipe flow regime for
$\sqrt {\overline {u_{{1}}}^2}/\bar {U}_{{1}}$ in fully developed turbulent pipe flow regime for ![]() ${Re_{\tau }}=3600$ at various wall-normal locations:
${Re_{\tau }}=3600$ at various wall-normal locations: ![]() $x_{{2}}^+=150$ is the bottom of the logarithmic region,
$x_{{2}}^+=150$ is the bottom of the logarithmic region, ![]() ${x_{{2}}/R}=0.15$ is the top of the logarithmic region, and
${x_{{2}}/R}=0.15$ is the top of the logarithmic region, and ![]() ${x_{{2}}^{}/R}=1$ is the pipe centreline, where
${x_{{2}}^{}/R}=1$ is the pipe centreline, where ![]() $x_{{2}}^+=x_{{2}}^{}u_{\tau }/\nu$.
$x_{{2}}^+=x_{{2}}^{}u_{\tau }/\nu$.
The high turbulence level received by hot-wire probes during calibration would give rise to substantial levels of uncertainty in measured velocity fluctuations, resulting in error in evaluating the turbulence statistics (Talluru et al. Reference Talluru, Kulandaivelu and Hutchins2014; Boufidi et al. Reference Boufidi, Lavagnoli and Fontaneto2020). For instance, in spectral analysis, the inaccurate hot-wire calibration would result in errors in the estimation of wavenumbers and consequently wavelengths in correspondence with the large-scale motions as well as magnitudes of the spectral peaks.
The present pipe facility provides a unique opportunity to perform precise in situ calibration (see e.g. Durst, Zanoun & Pashtrapanska Reference Durst, Zanoun and Pashtrapanska2001) for both hot-wire probes, simultaneously, with blockage range ![]() $0.4\,\%\unicode{x2013}{2.8\,\%}$ and minimal aerodynamic disturbances. Two identical 25 cm long portable pipe sections of 190 mm inner diameter were produced (see figure 1a). One piece is produced to replace the other that hosts the two hot-wire probes. The one that hosts the two hot-wire probes is presented in figure 1(b), allowing the calibration of both probes at the inlet of the pipe test section where the flow is assured to be uniform and laminar for the full range of the Reynolds number, i.e.
$0.4\,\%\unicode{x2013}{2.8\,\%}$ and minimal aerodynamic disturbances. Two identical 25 cm long portable pipe sections of 190 mm inner diameter were produced (see figure 1a). One piece is produced to replace the other that hosts the two hot-wire probes. The one that hosts the two hot-wire probes is presented in figure 1(b), allowing the calibration of both probes at the inlet of the pipe test section where the flow is assured to be uniform and laminar for the full range of the Reynolds number, i.e. ![]() $6\times {10^2}<{Re_\tau }<2\times {10^4}$, with a very low turbulence level (see figure 3). The streamwise mean velocity component
$6\times {10^2}<{Re_\tau }<2\times {10^4}$, with a very low turbulence level (see figure 3). The streamwise mean velocity component ![]() $\bar {U}_1=\sqrt {2(P_0-p_{st})/\rho (1-k)}$ of the air flow at the pipe inlet was obtained via pressure drop (
$\bar {U}_1=\sqrt {2(P_0-p_{st})/\rho (1-k)}$ of the air flow at the pipe inlet was obtained via pressure drop (![]() $P_0-p_{st}$) measurements along the pipe inlet contraction (see figure 1a), using a differential pressure transducer and the Bernoulli equation, where
$P_0-p_{st}$) measurements along the pipe inlet contraction (see figure 1a), using a differential pressure transducer and the Bernoulli equation, where ![]() $\rho$ is the air density,
$\rho$ is the air density, ![]() $k=(D/D_{in})^4$ is the pipe-to-contraction base area ratio,
$k=(D/D_{in})^4$ is the pipe-to-contraction base area ratio, ![]() $D_{in}$ is the contraction base diameter, and
$D_{in}$ is the contraction base diameter, and ![]() $P_0$ and
$P_0$ and ![]() $p_{st}$ are the mean pressures at base and exit of the inlet contraction, respectively. After the calibration data acquisition
$p_{st}$ are the mean pressures at base and exit of the inlet contraction, respectively. After the calibration data acquisition ![]() $\bar {E}=f(\bar {U}_1)$, a fourth-degree polynomial was chosen to fit the calibration data for each hot-wire probe, with least-squares error better than
$\bar {E}=f(\bar {U}_1)$, a fourth-degree polynomial was chosen to fit the calibration data for each hot-wire probe, with least-squares error better than ![]() ${\pm }1\,\%$ (see figure 5), where
${\pm }1\,\%$ (see figure 5), where ![]() $\bar {E}$ is the mean hot-wire output. After ensuring high-precision calibration for the two hot-wire probes at the pipe inlet, the CTA control unit was set to standby. Then the pipe segment that hosts the two hot-wire probes was completely transferred to the measurement station at
$\bar {E}$ is the mean hot-wire output. After ensuring high-precision calibration for the two hot-wire probes at the pipe inlet, the CTA control unit was set to standby. Then the pipe segment that hosts the two hot-wire probes was completely transferred to the measurement station at ![]() $x_{{2}}/D= 120$, where the pipe flow was assured to be fully developed turbulent, and the CTA was set back to on. To ensure that the original calibration curve was maintained during one entire set of measurements, the calibration curves were rechecked after each set of measurements covering the entire range of velocities experienced. It is worth noting that each hot-wire probe was integrated with a 20 m long PNC cable, i.e. 105 pipe diameters, connecting it to the CTA. In addition, the CTA control unit was set up on a movable cabinet that is located near the middle of the pipe facility, i.e. at length
$x_{{2}}/D= 120$, where the pipe flow was assured to be fully developed turbulent, and the CTA was set back to on. To ensure that the original calibration curve was maintained during one entire set of measurements, the calibration curves were rechecked after each set of measurements covering the entire range of velocities experienced. It is worth noting that each hot-wire probe was integrated with a 20 m long PNC cable, i.e. 105 pipe diameters, connecting it to the CTA. In addition, the CTA control unit was set up on a movable cabinet that is located near the middle of the pipe facility, i.e. at length ![]() $\approx$70 pipe diameters from the pipe inlet. Thus the set-up allows exchanging the two hot-wire probe module between the pipe inlet
$\approx$70 pipe diameters from the pipe inlet. Thus the set-up allows exchanging the two hot-wire probe module between the pipe inlet ![]() $x_1/D=0$ and the measurement station at
$x_1/D=0$ and the measurement station at ![]() $x_1/D=120$ without disconnecting them.
$x_1/D=120$ without disconnecting them.

Figure 5. The calibration curves for both hot-wire probes with least-squares errors 0.25 % and 0.27 % for the radial and azimuthal probes, respectively.
To summarize, the inconsistencies observed in the available two-point correlation hot-wire data in literature might be attributed to:
(i) uncertainties in the calibration of hot-wire probes due to difficulties in conducting simultaneous in situ calibration, resulting in errors in the statistical analysis and in the conclusions drawn;
(ii) at high Reynolds numbers, inadequate spatial (Blackwelder & Haritonidis Reference Blackwelder and Haritonidis1983; Hutchins et al. Reference Hutchins, Nickels, Marusic and Chong2009) and temporal (Hutchins et al. Reference Hutchins, Monty and Hultmark2015) resolutions, in particular, in the vicinity of the wall where the flow exhibits a strong gradient, introducing an additional source of error in the calibration equation and in the statistical analysis;
(iii) temperature drift (Talluru et al. Reference Talluru, Kulandaivelu and Hutchins2014) and uncertainties in positioning both probes with respect to the pipe wall (Örlü, Fransson & Alfredsson Reference Örlü, Fransson and Alfredsson2010), also of importance during the calibration and measurements.
The present work was therefore undertaken with the aim of obtaining accurate pipe flow data, utilizing the CoLaPipe in the fully developed turbulent flow regime for ![]() $2\times {10^3}\le {Re_{\tau }}\le {16\times {10^3}}$ and wall-normal distances
$2\times {10^3}\le {Re_{\tau }}\le {16\times {10^3}}$ and wall-normal distances ![]() $0.1\le {x_{{2}}/R}\le {0.7}$.
$0.1\le {x_{{2}}/R}\le {0.7}$.
3. Data analysis
3.1. Uncertainty and energy spectra
We start by examining briefly the measurement uncertainty that might be introduced into the streamwise velocity component due to the blockage of the probe holders and probable eccentricity of the hot-wire probes. The blockage of the pipe cross-section due to probe holders ranged from 0.4 % to 2.8 % for wall-normal locations ![]() ${x_{{2}}/R}=0.1$ and
${x_{{2}}/R}=0.1$ and ![]() ${x_{{2}}/R}=0.7$, respectively. The experimental deviations in the local mean and fluctuations of the streamwise velocity component obtained from both hot-wire probes were examined at the four wall-normal locations
${x_{{2}}/R}=0.7$, respectively. The experimental deviations in the local mean and fluctuations of the streamwise velocity component obtained from both hot-wire probes were examined at the four wall-normal locations ![]() ${x_{{2}}/R}=0.1, 0.2, 0.5, 0.7$ and the 35–41 azimuthal positions. Selected samples at the wall-normal locations
${x_{{2}}/R}=0.1, 0.2, 0.5, 0.7$ and the 35–41 azimuthal positions. Selected samples at the wall-normal locations ![]() ${x_{{2}}/R}=0.1$ and
${x_{{2}}/R}=0.1$ and ![]() ${x_{{2}}/R}=0.7$, representing the minimum and maximum blockages, respectively, are shown in figure 6 for two different Reynolds numbers.
${x_{{2}}/R}=0.7$, representing the minimum and maximum blockages, respectively, are shown in figure 6 for two different Reynolds numbers.

Figure 6. The local deviation in the streamwise velocity component (a,b) mean and (c,d) fluctuations at two wall-normal locations of the pipe cross-section, and two Reynolds numbers: (a,c) ![]() ${x_{{2}}/R}=0.1$ (0.4 % blockage) and (b,d)
${x_{{2}}/R}=0.1$ (0.4 % blockage) and (b,d) ![]() ${x_{{2}}/R}=0.7$ (2.8 % blockage).
${x_{{2}}/R}=0.7$ (2.8 % blockage).
For each measure, the two probes were located at the same wall-normal distance, therefore both probes are expected to receive and measure the same local time-averaged and higher-order statistics of the streamwise velocity component for all azimuthal positions. Using the least-squares method, figures 6(a,b) illustrate an uncertainty/deviation ![]() ${{\Delta }}{U_{{1}}}/\bar {U}_{{1}}$ within the range
${{\Delta }}{U_{{1}}}/\bar {U}_{{1}}$ within the range ![]() ${{\pm }{0.4\,\%}}$ to
${{\pm }{0.4\,\%}}$ to ![]() ${\pm }1.6\,\%$ in the local mean velocity obtained from either the fixed or the traversing azimuthal hot-wire probes at
${\pm }1.6\,\%$ in the local mean velocity obtained from either the fixed or the traversing azimuthal hot-wire probes at ![]() ${x_{{2}}/R}=0.1,0.7$, respectively. Note that the deviation in the local mean is defined as
${x_{{2}}/R}=0.1,0.7$, respectively. Note that the deviation in the local mean is defined as ![]() ${{\Delta }}{U_{{1}}}/\bar {U}_{{1}}=(U_{{1}}-\bar {U}_{{1}})/(\bar {U}_{{1}})$, where
${{\Delta }}{U_{{1}}}/\bar {U}_{{1}}=(U_{{1}}-\bar {U}_{{1}})/(\bar {U}_{{1}})$, where ![]() $U_{{1}}$ is the individual local mean of the streamwise velocity component, and
$U_{{1}}$ is the individual local mean of the streamwise velocity component, and ![]() $\bar {U}_{{1}}$ represents the average of all local means of the streamwise velocity component across all azimuthal locations. On the other hand, figures 6(c,d) show the uncertainty/deviation in the turbulence intensity level
$\bar {U}_{{1}}$ represents the average of all local means of the streamwise velocity component across all azimuthal locations. On the other hand, figures 6(c,d) show the uncertainty/deviation in the turbulence intensity level ![]() $TI=\sqrt {\overline {u_{{1}}}^2}/U_{{1}}$ for the streamwise velocity component from both probes, with average values within the range
$TI=\sqrt {\overline {u_{{1}}}^2}/U_{{1}}$ for the streamwise velocity component from both probes, with average values within the range ![]() ${{\pm }{0.7\,\%}}$ to
${{\pm }{0.7\,\%}}$ to ![]() ${\pm }2.67\,\%$ based on the least-squares method at
${\pm }2.67\,\%$ based on the least-squares method at ![]() ${x_{{2}}/R}=0.1,0.7$, respectively. Note also that the deviation in
${x_{{2}}/R}=0.1,0.7$, respectively. Note also that the deviation in ![]() $TI$ is defined as
$TI$ is defined as ![]() ${{\Delta }}{TI}/\overline {TI}=(TI-\overline {TI})/(\overline {TI})$, where
${{\Delta }}{TI}/\overline {TI}=(TI-\overline {TI})/(\overline {TI})$, where ![]() $TI$ is the individual local turbulence intensity level, and
$TI$ is the individual local turbulence intensity level, and ![]() $\overline {TI}$ represents the average of all local values of
$\overline {TI}$ represents the average of all local values of ![]() $TI$ across all azimuthal locations. The small discrepancies observed in figure 6 between the two probes might be attributed to aerodynamic interference effects between the two probes, regardless of the separation, i.e.
$TI$ across all azimuthal locations. The small discrepancies observed in figure 6 between the two probes might be attributed to aerodynamic interference effects between the two probes, regardless of the separation, i.e. ![]() ${{\Delta }}{\theta }$, as well as to some probe eccentricity. The local turbulence intensity
${{\Delta }}{\theta }$, as well as to some probe eccentricity. The local turbulence intensity ![]() $TI$ levels received from either the fixed or the traversing azimuthal probes at the four wall-normal locations agree well with similar data presented earlier in figure 4 and obtained from a single hot-wire probe at various wall-normal locations.
$TI$ levels received from either the fixed or the traversing azimuthal probes at the four wall-normal locations agree well with similar data presented earlier in figure 4 and obtained from a single hot-wire probe at various wall-normal locations.
To further assess effects of probe-holder blockage and/or probe eccentricity on the present pipe data analysis, the streamwise energy spectra measured at 35–41 azimuthal positions were calculated versus spectra measured 35–41 times at identical locations and a pre-selected wall-normal location for the same Reynolds number. The solid line presented in each spectrum subplot in both figures 7 and 8 represents the average across all azimuthal locations between the minimum probe separation ![]() ${{{\Delta }}{\theta }}=10^{\circ }$ and the maximum probe separation
${{{\Delta }}{\theta }}=10^{\circ }$ and the maximum probe separation ![]() ${{{\Delta }}{\theta }}=210^{\circ }$. All power-spectral dashed lines presented in both figures 7 and 8 were obtained in a similar manner to the solid lines, representing the average for all measured cases, utilizing the radial probe at the same radial location. It is worth noting here that the frequency spectra
${{{\Delta }}{\theta }}=210^{\circ }$. All power-spectral dashed lines presented in both figures 7 and 8 were obtained in a similar manner to the solid lines, representing the average for all measured cases, utilizing the radial probe at the same radial location. It is worth noting here that the frequency spectra ![]() ${\varPhi }_{u_{{1}}u_{{1}}}(f)$ were estimated using Welch's power-spectral density method, i.e. fast Fourier transform. The time trace data of the streamwise velocity fluctuations received from each hot-wire probe were acquired at a 30 kHz sampling frequency with a 60–120 sampling time, providing a total number of
${\varPhi }_{u_{{1}}u_{{1}}}(f)$ were estimated using Welch's power-spectral density method, i.e. fast Fourier transform. The time trace data of the streamwise velocity fluctuations received from each hot-wire probe were acquired at a 30 kHz sampling frequency with a 60–120 sampling time, providing a total number of ![]() $1.8\unicode{x2013}3.6\times {10^6}$ samples (see table 1). The data were then divided into eight successive blocks with 50 % overlap, and each segment was windowed with a Hamming window. Thereafter, to map the frequency spectra
$1.8\unicode{x2013}3.6\times {10^6}$ samples (see table 1). The data were then divided into eight successive blocks with 50 % overlap, and each segment was windowed with a Hamming window. Thereafter, to map the frequency spectra ![]() ${\varPhi _{u_{{1}}u_{{1}}}(f)}$ into the wavenumber spectra
${\varPhi _{u_{{1}}u_{{1}}}(f)}$ into the wavenumber spectra ![]() ${\varPhi _{u_{{1}}u_{{1}}}(k_{x_{{1}}})}$, Taylor's convected frozen-turbulence hypothesis
${\varPhi _{u_{{1}}u_{{1}}}(k_{x_{{1}}})}$, Taylor's convected frozen-turbulence hypothesis
was implemented (Taylor Reference Taylor1938), assuming convection of the pipe flow structure with the local mean velocity (for more details, see de Kat & Ganapathisubramani Reference de Kat and Ganapathisubramani2015).

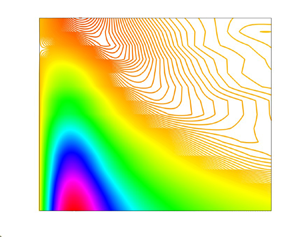
Figure 7. Effect of wall-normal location ![]() ${x_{{2}}/R}$. Premultiplied energy spectra
${x_{{2}}/R}$. Premultiplied energy spectra ![]() $k_{x_{{1}}}R{{\varPhi _{u_{{1}}u_{{1}}}^{++}}}$ in outer scaling versus the streamwise normalized wavenumber
$k_{x_{{1}}}R{{\varPhi _{u_{{1}}u_{{1}}}^{++}}}$ in outer scaling versus the streamwise normalized wavenumber ![]() $k_{x_{{1}}}R$ for shear Reynolds number
$k_{x_{{1}}}R$ for shear Reynolds number ![]() ${Re}_{\tau }\approx {4100}$: (a)
${Re}_{\tau }\approx {4100}$: (a) ![]() $x_{{2}}/R=0.1$, (b)
$x_{{2}}/R=0.1$, (b) ![]() $x_{{2}}/R=0.2$, (c)
$x_{{2}}/R=0.2$, (c) ![]() $x_{{2}}/R=0.5$, and (d)
$x_{{2}}/R=0.5$, and (d) ![]() $x_2/R=0.7$.
$x_2/R=0.7$.

Figure 8. Effect of the Reynolds number ![]() ${Re}_{\tau }$. Premultiplied energy spectra
${Re}_{\tau }$. Premultiplied energy spectra ![]() $k_{x_{{1}}}R{{\varPhi _{u_{{1}}u_{{1}}}^{++}}}$ in outer scaling versus the streamwise normalized wavenumber
$k_{x_{{1}}}R{{\varPhi _{u_{{1}}u_{{1}}}^{++}}}$ in outer scaling versus the streamwise normalized wavenumber ![]() $k_{x_{{1}}}R$ at a wall-normal location
$k_{x_{{1}}}R$ at a wall-normal location ![]() ${x_{{2}}/R}= 0.5$: (a)
${x_{{2}}/R}= 0.5$: (a) ![]() ${Re}_{\tau }=4100$, (b)
${Re}_{\tau }=4100$, (b) ![]() ${Re}_{\tau }=7700$, (c)
${Re}_{\tau }=7700$, (c) ![]() ${Re}_{\tau }=11\,500$, and (d)
${Re}_{\tau }=11\,500$, and (d) ![]() ${Re}_{\tau }=15\,300$.
${Re}_{\tau }=15\,300$.
Note that Taylor's hypothesis relates the longitudinal spatial correlation to the temporal correlation at a single point measured by the hot-wire probe. Thus the wavenumber relation ![]() $k_{x_{{1}}}=2{\rm \pi} {f}/{\bar {U}_1(x_{{2}})}$ converts the spectral argument from the frequency domain
$k_{x_{{1}}}=2{\rm \pi} {f}/{\bar {U}_1(x_{{2}})}$ converts the spectral argument from the frequency domain ![]() ${f}$ to the wavenumber
${f}$ to the wavenumber ![]() $k_{x_{{1}}}$ domain, where
$k_{x_{{1}}}$ domain, where ![]() $k_{x_{{1}}}$ is the streamwise wavenumber, and
$k_{x_{{1}}}$ is the streamwise wavenumber, and ![]() $\bar {U}_1(x_{{2}})$ is the local mean convective streamwise velocity. The streamwise spectra
$\bar {U}_1(x_{{2}})$ is the local mean convective streamwise velocity. The streamwise spectra ![]() ${\varPhi _{u_{{1}}u_{{1}}}}(k_{x_{{1}}})$ are scaled with the wall friction velocity
${\varPhi _{u_{{1}}u_{{1}}}}(k_{x_{{1}}})$ are scaled with the wall friction velocity ![]() $u_{\tau }$ (Zanoun et al. Reference Zanoun, Egbers, Nagib, Durst, Bellani and Talamelli2021), the streamwise wavelength
$u_{\tau }$ (Zanoun et al. Reference Zanoun, Egbers, Nagib, Durst, Bellani and Talamelli2021), the streamwise wavelength ![]() $k_{x_{{1}}}$ and the pipe radius
$k_{x_{{1}}}$ and the pipe radius ![]() $R$, resulting in the so-called premultiplied spectra
$R$, resulting in the so-called premultiplied spectra ![]() $k_{x_{{1}}}R\,{{\varPhi _{u_{{1}}u_{{1}}}(k_{x_{{1}}}}})/{u_{\tau }}^2\equiv {k_{x_{{1}}}R{{\varPhi _{u_{{1}}u_{{1}}}^{++}}}}$ presented in both figures 7 and 8 versus the normalized wavenumber
$k_{x_{{1}}}R\,{{\varPhi _{u_{{1}}u_{{1}}}(k_{x_{{1}}}}})/{u_{\tau }}^2\equiv {k_{x_{{1}}}R{{\varPhi _{u_{{1}}u_{{1}}}^{++}}}}$ presented in both figures 7 and 8 versus the normalized wavenumber ![]() $k_{x_{{1}}}R$. Both figures show satisfactory collapse of the premultiplied spectra lines obtained from the two hot-wire probes at pre-selected wall-normal locations and Reynolds numbers. The small differences observed between the two hot-wire probes,
$k_{x_{{1}}}R$. Both figures show satisfactory collapse of the premultiplied spectra lines obtained from the two hot-wire probes at pre-selected wall-normal locations and Reynolds numbers. The small differences observed between the two hot-wire probes, ![]() ${x_{{2}}/R}=0.1$ in figure 7(a) and
${x_{{2}}/R}=0.1$ in figure 7(a) and ![]() ${x_{{2}}/R}=0.2$ in figure 7(b), might either reflect changes in the azimuthal structure of the large-scale motions, in particular, in the inner wall layer as will be shown later, or be due to the wall interaction with both the radial and azimuthal probes. The amplitudes of the peaks observed in the energy spectra of the two probes in both figures agree within
${x_{{2}}/R}=0.2$ in figure 7(b), might either reflect changes in the azimuthal structure of the large-scale motions, in particular, in the inner wall layer as will be shown later, or be due to the wall interaction with both the radial and azimuthal probes. The amplitudes of the peaks observed in the energy spectra of the two probes in both figures agree within ![]() ${\pm }{1.5\,\%}$ to
${\pm }{1.5\,\%}$ to ![]() ${\pm }{5\,\%}$, indicating minimal effects of the blockage due to probe holders and/or probe eccentricity. In both figures 7 and 8, two discernible peaks are observable: one at low streamwise wavenumber associated with the very-large-scale motion (VLSM), and the second at moderate streamwise wavenumber associated with the large-scale motion (LSM). The two observable peaks in the energy spectra obtained at low and moderate wavenumbers are clear evidence or footprints for the LSMs in the pipe flow. Thus the two modes, i.e. a low-wavenumber mode and moderate-wavenumber mode, adopted from both figures 7 and 8 are in agreement with Kim & Adrian (Reference Kim and Adrian1999) for the pipe flow. The VLSMs and LSMs are located at the streamwise wavenumbers
${\pm }{5\,\%}$, indicating minimal effects of the blockage due to probe holders and/or probe eccentricity. In both figures 7 and 8, two discernible peaks are observable: one at low streamwise wavenumber associated with the very-large-scale motion (VLSM), and the second at moderate streamwise wavenumber associated with the large-scale motion (LSM). The two observable peaks in the energy spectra obtained at low and moderate wavenumbers are clear evidence or footprints for the LSMs in the pipe flow. Thus the two modes, i.e. a low-wavenumber mode and moderate-wavenumber mode, adopted from both figures 7 and 8 are in agreement with Kim & Adrian (Reference Kim and Adrian1999) for the pipe flow. The VLSMs and LSMs are located at the streamwise wavenumbers ![]() $k_{x_{{1}}}{R} \approx {0.4\unicode{x2013}1}$ and
$k_{x_{{1}}}{R} \approx {0.4\unicode{x2013}1}$ and ![]() $k_{x_{{1}}}{R} \approx {2\unicode{x2013}6}$, respectively, in alignment with Kim & Adrian (Reference Kim and Adrian1999) and Balakumar & Adrian (Reference Balakumar and Adrian2007). One can observe from
$k_{x_{{1}}}{R} \approx {2\unicode{x2013}6}$, respectively, in alignment with Kim & Adrian (Reference Kim and Adrian1999) and Balakumar & Adrian (Reference Balakumar and Adrian2007). One can observe from ![]() ${x_{{2}}/R}=0.1$ in figure 7(a) and
${x_{{2}}/R}=0.1$ in figure 7(a) and ![]() ${x_{{2}}/R}=0.2$ in figure 7(b) that the inner peak, representing the VLSM, is more prominent than the outer peak of the LSM, in agreement with a similar observation made by Kim & Adrian (Reference Kim and Adrian1999) and Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008). Both peaks decrease in energy content with increase in the wall-normal distance, as indicated for
${x_{{2}}/R}=0.2$ in figure 7(b) that the inner peak, representing the VLSM, is more prominent than the outer peak of the LSM, in agreement with a similar observation made by Kim & Adrian (Reference Kim and Adrian1999) and Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008). Both peaks decrease in energy content with increase in the wall-normal distance, as indicated for ![]() ${x_{{2}}/R}=0.5$ in figure 7(c) and
${x_{{2}}/R}=0.5$ in figure 7(c) and ![]() ${x_{{2}}/R}=0.7$ in figure 7(d); however, the energy contained in the VLSM peak decreases faster than that for the LSM. On the other hand, the LSM peak observed in figures 7(c) and (d) (
${x_{{2}}/R}=0.7$ in figure 7(d); however, the energy contained in the VLSM peak decreases faster than that for the LSM. On the other hand, the LSM peak observed in figures 7(c) and (d) (![]() ${x_{{2}}/R}=0.5$ and
${x_{{2}}/R}=0.5$ and ![]() ${x_{{2}}/R}=0.7$, respectively), i.e. in the core region, is more prominent than the VLSM peak. The VLSMs seem from figure 7(c) to continue until
${x_{{2}}/R}=0.7$, respectively), i.e. in the core region, is more prominent than the VLSM peak. The VLSMs seem from figure 7(c) to continue until ![]() ${x_{{2}}/R}=0.5$, and thereafter one large-scale structure forms, i.e. LSM structures, and extends to the pipe centreline, as will be shown later. The physical model proposed by Kim & Adrian (Reference Kim and Adrian1999) and Meinhart & Adrian (Reference Meinhart and Adrian1995) – that the VLSMs are associated with the zones of uniform low momentum found in the boundary layer that extend only up to approximately one-half of the boundary layer thickness or the pipe radius – would be appropriate to interpret the disappearance of the VLSMs peak beyond
${x_{{2}}/R}=0.5$, and thereafter one large-scale structure forms, i.e. LSM structures, and extends to the pipe centreline, as will be shown later. The physical model proposed by Kim & Adrian (Reference Kim and Adrian1999) and Meinhart & Adrian (Reference Meinhart and Adrian1995) – that the VLSMs are associated with the zones of uniform low momentum found in the boundary layer that extend only up to approximately one-half of the boundary layer thickness or the pipe radius – would be appropriate to interpret the disappearance of the VLSMs peak beyond ![]() $x_2/R={0.5}$ as indicated in figure 7(d).
$x_2/R={0.5}$ as indicated in figure 7(d).
To study further the effect of the Reynolds number on both the inner and outer peaks of the LSMs, a selected sample is presented in figure 8 at ![]() ${x_{{2}}/R}=0.5$ for
${x_{{2}}/R}=0.5$ for ![]() $4100\le {Re_{\tau }}\le {15\,300}$. The overall behaviour is similar in all plots in terms of the appearance of the inner and outer peaks and their wavenumbers. However, a clear dependence of the magnitude of the energy peaks at the corresponding wavenumbers for the LSM and VLSM on the Reynolds number is observable.
$4100\le {Re_{\tau }}\le {15\,300}$. The overall behaviour is similar in all plots in terms of the appearance of the inner and outer peaks and their wavenumbers. However, a clear dependence of the magnitude of the energy peaks at the corresponding wavenumbers for the LSM and VLSM on the Reynolds number is observable.
3.2. Azimuthal velocity correlation
To characterize the azimuthal pipe flow structure, the two-point velocity correlation coefficient ![]() $R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$, defined in § 1, was calculated and illustrated in both figures 9 and 10, addressing the effect of the wall-normal location, the azimuthal separation, and the Reynolds number on
$R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$, defined in § 1, was calculated and illustrated in both figures 9 and 10, addressing the effect of the wall-normal location, the azimuthal separation, and the Reynolds number on ![]() $R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$. Both figures represent profiles of
$R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$. Both figures represent profiles of ![]() $R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$ obtained at four wall-normal locations,
$R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$ obtained at four wall-normal locations, ![]() ${x_{{2}}/R}=0.1, 0.2, 0.5, 0.7$, for 35–41 azimuthal positions and Reynolds numbers in the range
${x_{{2}}/R}=0.1, 0.2, 0.5, 0.7$, for 35–41 azimuthal positions and Reynolds numbers in the range ![]() $4100\le {{Re_{\tau }}}\le {15\,300}$.
$4100\le {{Re_{\tau }}}\le {15\,300}$.

Figure 9. Effect of the wall-normal location ![]() ${x_{{2}}/R}$, the azimuthal separation
${x_{{2}}/R}$, the azimuthal separation ![]() ${{{\Delta }}}{s}/R$ and the Reynolds number
${{{\Delta }}}{s}/R$ and the Reynolds number ![]() ${Re}_{\tau }$ on the azimuthal velocity correlation
${Re}_{\tau }$ on the azimuthal velocity correlation ![]() $R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$ of the streamwise velocity fluctuations at four wall-normal locations,
$R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$ of the streamwise velocity fluctuations at four wall-normal locations, ![]() $0.1\le {{x_{{2}}/R}}\le {0.7}$ and
$0.1\le {{x_{{2}}/R}}\le {0.7}$ and ![]() ${4100\le {Re_{\tau }}}\le {15\,300}$: (a)
${4100\le {Re_{\tau }}}\le {15\,300}$: (a) ![]() ${Re}_{\tau }=4100$, (b)
${Re}_{\tau }=4100$, (b) ![]() ${Re}_{\tau }=7700$, (c)
${Re}_{\tau }=7700$, (c) ![]() ${Re}_{\tau }=11\,500$, and (d)
${Re}_{\tau }=11\,500$, and (d) ![]() ${Re}_{\tau }=15\,300$.
${Re}_{\tau }=15\,300$.

Figure 10. Effect of the wall-normal location ![]() ${x_{{2}}/R}$, the azimuthal separation
${x_{{2}}/R}$, the azimuthal separation ![]() ${{{\Delta }}}{s}/R$ and the Reynolds number
${{{\Delta }}}{s}/R$ and the Reynolds number ![]() ${Re}_{\tau }$ on the azimuthal velocity correlation
${Re}_{\tau }$ on the azimuthal velocity correlation ![]() $R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$. Isocontours of the azimuthal correlation of the streamwise velocity component
$R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$. Isocontours of the azimuthal correlation of the streamwise velocity component ![]() $R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$ at four wall-normal locations,
$R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$ at four wall-normal locations, ![]() $0.1\le {{x_{{2}}/R}}\le {0.7}$ and
$0.1\le {{x_{{2}}/R}}\le {0.7}$ and ![]() ${4100\le {Re_{\tau }}}\le {15\,300}$:(a)
${4100\le {Re_{\tau }}}\le {15\,300}$:(a) ![]() ${Re}_{\tau }=4100$, (b)
${Re}_{\tau }=4100$, (b) ![]() ${Re}_{\tau }=7700$, (c)
${Re}_{\tau }=7700$, (c) ![]() ${Re}_{\tau }=11\,500$, and (d)
${Re}_{\tau }=11\,500$, and (d) ![]() ${Re}_{\tau }=15\,300$.
${Re}_{\tau }=15\,300$.
It is worth noting that the wall-normal locations were chosen to show changes in the azimuthal structure in both the logarithmic and core regions of the fully developed turbulent pipe flow. In figure 9, for small azimuthal separation distances between the two hot-wire probes, high correlation values were obtained, indicating strong coherence between the two signals where both probes would receive velocity fluctuations with similar signs. By increasing the azimuthal separation distance between the two probes, the coherence is weakened, reaching zero at various separation distances, depending on the wall-normal location and the Reynolds number, and then its sign changes to negative. All plots of the figure illustrate regions of positive–negative velocity correlations within various ranges of ![]() ${{{\Delta }}}{s}/R$, expressing clear dependence on the wall-normal location, the azimuthal separation and the Reynolds number. The positive–negative trends of the correlation coefficient shown in figure 9 represent an indication of the vortex-packets/hairpin pipe flow structure in agreement with similar observations made by Tomkins & Adrian (Reference Tomkins and Adrian2003) and Monty et al. (Reference Monty, Stewart, Williams and Chong2007) for flat-plate boundary layer and pipe flows, respectively.
${{{\Delta }}}{s}/R$, expressing clear dependence on the wall-normal location, the azimuthal separation and the Reynolds number. The positive–negative trends of the correlation coefficient shown in figure 9 represent an indication of the vortex-packets/hairpin pipe flow structure in agreement with similar observations made by Tomkins & Adrian (Reference Tomkins and Adrian2003) and Monty et al. (Reference Monty, Stewart, Williams and Chong2007) for flat-plate boundary layer and pipe flows, respectively.
Each plot in figure 9 presents the azimuthal velocity correlation ![]() $R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$ at four wall-normal locations,
$R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$ at four wall-normal locations, ![]() ${x_{{2}}/R}=0.1,0.2,0.5,0.7$, for similar Reynolds numbers, namely,
${x_{{2}}/R}=0.1,0.2,0.5,0.7$, for similar Reynolds numbers, namely, ![]() ${{Re_{\tau }}}={4100}$ (figure 9a),
${{Re_{\tau }}}={4100}$ (figure 9a), ![]() ${{Re_{\tau }}}={7700}$ (figure 9b),
${{Re_{\tau }}}={7700}$ (figure 9b), ![]() ${{Re_{\tau }}}={11\,500}$ (figure 9c) and
${{Re_{\tau }}}={11\,500}$ (figure 9c) and ![]() ${{Re_{\tau }}}={15\,300}$ (figure 9d). The positive–negative values obtained are attributed to the azimuthal LSMs in alignment with the earlier definition of the LSMs as scales that produce energetic flow motions of long meandering positive-negative velocity fluctuations, see Tomkins & Adrian (Reference Tomkins and Adrian2003) and Monty et al. (Reference Monty, Stewart, Williams and Chong2007). It turns out to be also in alignment with Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008), who reported that the negative values of
${{Re_{\tau }}}={15\,300}$ (figure 9d). The positive–negative values obtained are attributed to the azimuthal LSMs in alignment with the earlier definition of the LSMs as scales that produce energetic flow motions of long meandering positive-negative velocity fluctuations, see Tomkins & Adrian (Reference Tomkins and Adrian2003) and Monty et al. (Reference Monty, Stewart, Williams and Chong2007). It turns out to be also in alignment with Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008), who reported that the negative values of ![]() $R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$ obtained for the pipe flow can be attributed to the contribution of the LSMs to the coherent structure. For similar Reynolds numbers, changes in the magnitude and the range of the negative values of
$R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$ obtained for the pipe flow can be attributed to the contribution of the LSMs to the coherent structure. For similar Reynolds numbers, changes in the magnitude and the range of the negative values of ![]() $R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$ for the various wall-normal locations observed in figure 9 reflect a clear dependence of the azimuthal scales of the LSMs on the wall-normal location.
$R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$ for the various wall-normal locations observed in figure 9 reflect a clear dependence of the azimuthal scales of the LSMs on the wall-normal location.
From a first look, it seems from figures 9(a–d), however, that the Reynolds number effect is not predictable. Near the wall, i.e. ![]() $x_2/R=0.1, 0.2$, the two-point correlation function
$x_2/R=0.1, 0.2$, the two-point correlation function ![]() $R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$ narrows in figures 9(c,d) for
$R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$ narrows in figures 9(c,d) for ![]() ${Re_\tau }=11\,500, 15\,300$, while it broadens in figures 9(a,b) for
${Re_\tau }=11\,500, 15\,300$, while it broadens in figures 9(a,b) for ![]() ${Re_\tau }=4100, 7700$, respectively. At high Reynolds numbers, the measured velocity signals were attenuated, and due to the averaging process introduced into (1.1) over a wide range of turbulence scales, the
${Re_\tau }=4100, 7700$, respectively. At high Reynolds numbers, the measured velocity signals were attenuated, and due to the averaging process introduced into (1.1) over a wide range of turbulence scales, the ![]() $Re$ dependence was not properly revealed in figure 9. The cross-power spectral density is thus introduced in § 3.3 to highlight the Reynolds number effects on the azimuthal length scales at various wall-normal locations. However, figure 10 is produced here to see whether the azimuthal velocity correlation data presented in figure 9 are dependent only on the wall-normal location and the azimuthal separation.
$Re$ dependence was not properly revealed in figure 9. The cross-power spectral density is thus introduced in § 3.3 to highlight the Reynolds number effects on the azimuthal length scales at various wall-normal locations. However, figure 10 is produced here to see whether the azimuthal velocity correlation data presented in figure 9 are dependent only on the wall-normal location and the azimuthal separation.
Figure 10 is an isocontour plot of the azimuthal velocity correlation ![]() $R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$ for the four Reynolds numbers reported earlier at the wall-normal locations
$R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$ for the four Reynolds numbers reported earlier at the wall-normal locations ![]() $0.1\le {{x_{{2}}/R}}\le {0.7}$. The figure allows better visualization for the effect of the Reynolds number on
$0.1\le {{x_{{2}}/R}}\le {0.7}$. The figure allows better visualization for the effect of the Reynolds number on ![]() $R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$ across the pipe versus the azimuthal separations
$R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$ across the pipe versus the azimuthal separations ![]() $({{{\Delta }}}{s})$ for the wall-normal locations reported in figure 9. Due to axisymmetry of the pipe flow, it is worth noting that the data presented in figure 10 are for
$({{{\Delta }}}{s})$ for the wall-normal locations reported in figure 9. Due to axisymmetry of the pipe flow, it is worth noting that the data presented in figure 10 are for ![]() $0^{{\circ }}\le {{\Delta }}{\theta }\le {180^{{\circ }}}$ and imaged to the second half of the pipe cross-section. The contours and the colour levels in all plots are similar, i.e. the colour indicates the magnitude of
$0^{{\circ }}\le {{\Delta }}{\theta }\le {180^{{\circ }}}$ and imaged to the second half of the pipe cross-section. The contours and the colour levels in all plots are similar, i.e. the colour indicates the magnitude of ![]() $R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$ given by the scale at the right of the plot. Qualitatively, the overall views of all four plots are similar; however, with deeper insight one can observe differences among all four plots, indicating a Reynolds number dependence, in disagreement with Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008), who reported Reynolds number independence for the correlation coefficient. Figure 10 is believed to be more illustrative, indicating slight dependence of the two-point velocity correlation on the Reynolds number, the wall-normal distance and the azimuthal separation.
$R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$ given by the scale at the right of the plot. Qualitatively, the overall views of all four plots are similar; however, with deeper insight one can observe differences among all four plots, indicating a Reynolds number dependence, in disagreement with Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008), who reported Reynolds number independence for the correlation coefficient. Figure 10 is believed to be more illustrative, indicating slight dependence of the two-point velocity correlation on the Reynolds number, the wall-normal distance and the azimuthal separation.
To better highlight the Reynolds number effects at various wall-normal locations, the cross-power spectral analysis is introduced in the next subsection. It might be noted, however, that slightly positive correlation values observed in figure 10 at large azimuthal separation and low Reynolds number would reflect possible flow periodicity in agreement with the conclusion made by Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008). More analysis to quantify the sizes of the azimuthal scales of the LSMs for the present Reynolds number range ![]() $4100\le {{Re_{\tau }}}\le {15\,300}$ and the wall-normal distances
$4100\le {{Re_{\tau }}}\le {15\,300}$ and the wall-normal distances ![]() $0.1\le {x_2/R}\le {0.7}$ is discussed in the following subsection.
$0.1\le {x_2/R}\le {0.7}$ is discussed in the following subsection.
3.3. Cross-power spectral density
In principle, the cross-correlation and cross-power spectral density functions are pairs of Fourier transforms. For specific purposes, one form is often preferred over the other, for instance, when the contribution of the LSMs and VLSMs to the azimuthal scale structures is to be evaluated. The velocity cross-correlation ![]() ${R_{u_{{1}}u_{{1}}}}({{{\Delta }}}{s})$ data illustrated in both figures 9 and 10 were estimated using (1.1), which was defined based on ensemble averaging of the hot-wire data, comprising contributions from a broad band of turbulence scales that mix the contributions of all wavenumbers to the azimuthal pipe LSMs. It was concluded by Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008) that the averaging introduced in (1.1) obscures the proper contributions of both the LSMs and the VLSMs to the azimuthal scales. Thus the cross-power spectral density
${R_{u_{{1}}u_{{1}}}}({{{\Delta }}}{s})$ data illustrated in both figures 9 and 10 were estimated using (1.1), which was defined based on ensemble averaging of the hot-wire data, comprising contributions from a broad band of turbulence scales that mix the contributions of all wavenumbers to the azimuthal pipe LSMs. It was concluded by Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008) that the averaging introduced in (1.1) obscures the proper contributions of both the LSMs and the VLSMs to the azimuthal scales. Thus the cross-power spectral density ![]() $G({{{\Delta }}}{s},k_{x_{{1}}})$ for the streamwise velocity fluctuations as a function of the azimuthal separation
$G({{{\Delta }}}{s},k_{x_{{1}}})$ for the streamwise velocity fluctuations as a function of the azimuthal separation ![]() ${{{\Delta }}}{s}$ and the streamwise wavenumber
${{{\Delta }}}{s}$ and the streamwise wavenumber ![]() $k_{x_{{1}}}$ was proposed (Bailey et al. Reference Bailey, Hultmark, Smits and Schultz2008), to overcome the averaging introduced in (1.1). The cross-power spectral density
$k_{x_{{1}}}$ was proposed (Bailey et al. Reference Bailey, Hultmark, Smits and Schultz2008), to overcome the averaging introduced in (1.1). The cross-power spectral density ![]() ${G}({{{\Delta }}}{s},k_{x_{{1}}})$ is defined as
${G}({{{\Delta }}}{s},k_{x_{{1}}})$ is defined as
where ![]() ${{G}({{{\Delta }}}{s},k_{x_{{1}}})}$ is calculated using Welch's averaging method, i.e. a modified periodogram method of spectral estimation. It is the fast Fourier transform of the cross-correlation function between the two signals recorded by the two hot-wire probes, simultaneously, each of sampling size indicated in table 1. It is worth noting that the cross-power spectral density is single-sided, yielding information about the power/energy shared over a range of wavenumbers as well as the coherence or the phase difference between the two signals received from the two hot-wire probes. It is therefore used to specify the power/energy shared at specific flow wavenumbers, for instance, corresponding to the LSM and the VLSM of the streamwise velocity fluctuations. The quantity
${{G}({{{\Delta }}}{s},k_{x_{{1}}})}$ is calculated using Welch's averaging method, i.e. a modified periodogram method of spectral estimation. It is the fast Fourier transform of the cross-correlation function between the two signals recorded by the two hot-wire probes, simultaneously, each of sampling size indicated in table 1. It is worth noting that the cross-power spectral density is single-sided, yielding information about the power/energy shared over a range of wavenumbers as well as the coherence or the phase difference between the two signals received from the two hot-wire probes. It is therefore used to specify the power/energy shared at specific flow wavenumbers, for instance, corresponding to the LSM and the VLSM of the streamwise velocity fluctuations. The quantity ![]() $F^*(0,k_{x_{{1}}})$ is a complex conjugate of the finite Fourier transform of the streamwise velocity fluctuations
$F^*(0,k_{x_{{1}}})$ is a complex conjugate of the finite Fourier transform of the streamwise velocity fluctuations ![]() ${u_{{1}}(0,t)}$ measured by the fixed radial probe, while
${u_{{1}}(0,t)}$ measured by the fixed radial probe, while ![]() $F({{{\Delta }}}{s},k_{x_{{1}}})$ is the finite Fourier transform of the streamwise velocity fluctuations
$F({{{\Delta }}}{s},k_{x_{{1}}})$ is the finite Fourier transform of the streamwise velocity fluctuations ![]() ${u_{{1}}({{{\Delta }}}{s},t)}$ measured by the traversing azimuthal probe. The real component
${u_{{1}}({{{\Delta }}}{s},t)}$ measured by the traversing azimuthal probe. The real component ![]() ${\varPsi }({{{\Delta }}}{s},k_{x_{{1}}})$ of the cross-power spectral density
${\varPsi }({{{\Delta }}}{s},k_{x_{{1}}})$ of the cross-power spectral density ![]() $G({{{\Delta }}}{s},k_{x_{{1}}})$, defined as
$G({{{\Delta }}}{s},k_{x_{{1}}})$, defined as
is extracted and used to discuss further the distribution of the correlation of the streamwise velocity fluctuations as a function of the azimuthal separation ![]() ${{{\Delta }}}{s}$ and the streamwise wavenumber
${{{\Delta }}}{s}$ and the streamwise wavenumber ![]() $k_{x_{{1}}}$ for various Reynolds numbers and wall-normal locations. On the other hand, the imaginary part of the cross-power spectral density function indicates whether the signals received from the two hot-wire probes are in phase or not. If both signals are in phase, then the cross-power spectral density is positive, while if they are
$k_{x_{{1}}}$ for various Reynolds numbers and wall-normal locations. On the other hand, the imaginary part of the cross-power spectral density function indicates whether the signals received from the two hot-wire probes are in phase or not. If both signals are in phase, then the cross-power spectral density is positive, while if they are ![]() ${\pm }{180^{\circ }}$ out of phase, then the cross-power spectral density is negative.
${\pm }{180^{\circ }}$ out of phase, then the cross-power spectral density is negative.
Figures 11 and 12 represent in outer scaling the real component ![]() ${\varPsi }({{{\Delta }}}{s},k_{x_{{1}}})$ of the cross-power spectral density normalized using the streamwise wavenumber
${\varPsi }({{{\Delta }}}{s},k_{x_{{1}}})$ of the cross-power spectral density normalized using the streamwise wavenumber ![]() $k_{x_{{1}}}$, the pipe radius
$k_{x_{{1}}}$, the pipe radius ![]() $R$ and the wall friction velocity
$R$ and the wall friction velocity ![]() $u_{\tau }$, resulting in the so-called premultiplied cross-power spectral density
$u_{\tau }$, resulting in the so-called premultiplied cross-power spectral density ![]() $k_{x_{{1}}}R\,{\varPsi }({{{\Delta }}}{s},k_{x_{{1}}})/u_{\tau }u_{\tau }\equiv {k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}}$, versus the streamwise wavenumber
$k_{x_{{1}}}R\,{\varPsi }({{{\Delta }}}{s},k_{x_{{1}}})/u_{\tau }u_{\tau }\equiv {k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}}$, versus the streamwise wavenumber ![]() $k_{x_{{1}}}$ normalized with the pipe radius
$k_{x_{{1}}}$ normalized with the pipe radius ![]() $R$, i.e.
$R$, i.e. ![]() $k_{x_{{1}}}R$. Both figures 11 and 12 reflect the effect of the Reynolds number and the wall-normal location, respectively, on premultiplied cross-power spectral density
$k_{x_{{1}}}R$. Both figures 11 and 12 reflect the effect of the Reynolds number and the wall-normal location, respectively, on premultiplied cross-power spectral density ![]() $k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$. At fixed wall-normal location
$k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$. At fixed wall-normal location ![]() $x_2/R=0.7$, figure 11 presents
$x_2/R=0.7$, figure 11 presents ![]() $k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ versus
$k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ versus ![]() $k_{x_{{1}}}R$ for various azimuthal separations
$k_{x_{{1}}}R$ for various azimuthal separations ![]() $10^\circ \le {\Delta {\theta }\le {210^\circ }}$ and Reynolds numbers
$10^\circ \le {\Delta {\theta }\le {210^\circ }}$ and Reynolds numbers ![]() $4100\le {{Re_{\tau }}}\le {15\,300}$. The figure shows a dependence of the peak–trough values of
$4100\le {{Re_{\tau }}}\le {15\,300}$. The figure shows a dependence of the peak–trough values of ![]() $k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ and consequently the azimuthal structures on the Reynolds number as well as on the azimuthal separation for a fixed wall-normal location
$k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ and consequently the azimuthal structures on the Reynolds number as well as on the azimuthal separation for a fixed wall-normal location ![]() ${x_{{2}}/R}=0.7$. Further, figure 12 illustrates
${x_{{2}}/R}=0.7$. Further, figure 12 illustrates ![]() $k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ versus the normalized streamwise wavenumber
$k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ versus the normalized streamwise wavenumber ![]() $k_{x_{{1}}}R$ at four wall-normal locations
$k_{x_{{1}}}R$ at four wall-normal locations ![]() $0.1\le {x_{{2}}/R}\le {0.7}$ for various azimuthal separations
$0.1\le {x_{{2}}/R}\le {0.7}$ for various azimuthal separations ![]() $10^\circ \le {\Delta {\theta }\le {210^\circ }}$ and selected Reynolds number
$10^\circ \le {\Delta {\theta }\le {210^\circ }}$ and selected Reynolds number ![]() ${Re_{\tau }}=4100$. In both figures, the decrease in plot-line thickness as well as the downward arrow on all subplots indicate the direction of increasing azimuthal separation
${Re_{\tau }}=4100$. In both figures, the decrease in plot-line thickness as well as the downward arrow on all subplots indicate the direction of increasing azimuthal separation ![]() $\Delta {\theta }$ between the two probes. Figure 12 indicates a peak–trough mode with comparable behaviour for the wall-normal locations
$\Delta {\theta }$ between the two probes. Figure 12 indicates a peak–trough mode with comparable behaviour for the wall-normal locations ![]() ${x_{{2}}/R}=0.1, 0.2$. In all four plots, the peak of
${x_{{2}}/R}=0.1, 0.2$. In all four plots, the peak of ![]() $k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ attenuates with increasing the azimuthal separation
$k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ attenuates with increasing the azimuthal separation ![]() ${{{\Delta }}}{\theta }$ between the two probes, and the rate of attenuation/decay is higher for the closest pipe wall-normal locations
${{{\Delta }}}{\theta }$ between the two probes, and the rate of attenuation/decay is higher for the closest pipe wall-normal locations ![]() ${x_{{2}}/R}=0.1, 0.2$, where the hot-wire probes in both plots belong to the logarithmic layer. For similar Reynolds number
${x_{{2}}/R}=0.1, 0.2$, where the hot-wire probes in both plots belong to the logarithmic layer. For similar Reynolds number ![]() $Re_{\tau }=4100$, the peak value of
$Re_{\tau }=4100$, the peak value of ![]() $k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ attenuates quickly with increasing both the azimuthal separation
$k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ attenuates quickly with increasing both the azimuthal separation ![]() ${{{\Delta }}}{\theta }$ and the wall-normal location
${{{\Delta }}}{\theta }$ and the wall-normal location ![]() $x_{{2}}/R$; in particular, see figures 12(a–c) for
$x_{{2}}/R$; in particular, see figures 12(a–c) for ![]() $0.1\le {x_{{2}}}/R\le {0.5}$ up to azimuthal separation
$0.1\le {x_{{2}}}/R\le {0.5}$ up to azimuthal separation ![]() $\Delta {\theta } \approx {45}^{\circ }$. Changes in trough/negative values are also observable versus both the wall-normal location and the azimuthal separations. Those observations would be considered good indications of the coherence weaknesses as the azimuthal separation and the wall-normal locations increase. For the low Reynolds number cases, the viscous effect, in particular, near the pipe wall play an important role, contrary to the higher Reynolds number cases. Such rapid decay observed in
$\Delta {\theta } \approx {45}^{\circ }$. Changes in trough/negative values are also observable versus both the wall-normal location and the azimuthal separations. Those observations would be considered good indications of the coherence weaknesses as the azimuthal separation and the wall-normal locations increase. For the low Reynolds number cases, the viscous effect, in particular, near the pipe wall play an important role, contrary to the higher Reynolds number cases. Such rapid decay observed in ![]() $(k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++})_{peak}$ might also be interpreted by the hairpin vortex model proposed by Theodorsen (Reference Theodorsen1952). On the contrary, figures 11(d) and 12(d) for
$(k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++})_{peak}$ might also be interpreted by the hairpin vortex model proposed by Theodorsen (Reference Theodorsen1952). On the contrary, figures 11(d) and 12(d) for ![]() ${x_{{2}}/R}=0.7$ showed slow decay rate in
${x_{{2}}/R}=0.7$ showed slow decay rate in ![]() $(k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++})_{peak}$ versus the azimuthal separation
$(k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++})_{peak}$ versus the azimuthal separation ![]() $\Delta {\theta }$, which could be attributed to the relatively long lifetimes of the azimuthal vortices found predominantly in the core region compared to figures 11(a–c) and 12(a–c).
$\Delta {\theta }$, which could be attributed to the relatively long lifetimes of the azimuthal vortices found predominantly in the core region compared to figures 11(a–c) and 12(a–c).

Figure 11. Effect of Reynolds number on premultiplied cross-power spectral density ![]() $k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ at fixed wall-normal location
$k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ at fixed wall-normal location ![]() ${x_{{2}}/R}=0.7$. The downward arrow indicates increasing azimuthal separation
${x_{{2}}/R}=0.7$. The downward arrow indicates increasing azimuthal separation ![]() $\Delta {\theta }$ between the two hot-wire probes. Plots are for
$\Delta {\theta }$ between the two hot-wire probes. Plots are for ![]() ${x_{{2}}/R}=0.7$ and (a)
${x_{{2}}/R}=0.7$ and (a) ![]() ${{Re_{\tau }}}=4100$, (b)
${{Re_{\tau }}}=4100$, (b) ![]() ${{Re_{\tau }}}=7700$, (c)
${{Re_{\tau }}}=7700$, (c) ![]() ${{Re_{\tau }}}=11\,500$, and (d)
${{Re_{\tau }}}=11\,500$, and (d) ![]() ${{Re_{\tau }}}=15\,300$.
${{Re_{\tau }}}=15\,300$.

Figure 12. Effect of wall-normal location ![]() ${x_{{2}}/R}$ on premultiplied cross-power spectral density
${x_{{2}}/R}$ on premultiplied cross-power spectral density ![]() $k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ for constant Reynolds number
$k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ for constant Reynolds number ![]() ${{Re_{\tau }}}=4100$. The downward arrow indicates increasing azimuthal separation
${{Re_{\tau }}}=4100$. The downward arrow indicates increasing azimuthal separation ![]() $\Delta {\theta }$. Plots are for
$\Delta {\theta }$. Plots are for ![]() ${{Re_{\tau }}}=4100$ and (a)
${{Re_{\tau }}}=4100$ and (a) ![]() $x_2/R=0.1$, (b)
$x_2/R=0.1$, (b) ![]() $x_2/R=0.2$, (c)
$x_2/R=0.2$, (c) ![]() $x_2/R=0.5$, and (d)
$x_2/R=0.5$, and (d) ![]() $x_2/R=0.7$.
$x_2/R=0.7$.
To further support the data presented in figures 11 and 12, figures 13 and 14 are produced. Both figures 13 and 14 depict in outer scaling the isocontours of the real-part of premultiplied cross-power spectral density ![]() $k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ versus the streamwise wavenumber normalized
$k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ versus the streamwise wavenumber normalized ![]() $k_{x_{{1}}}R$. Figure 13 presents isocontours of
$k_{x_{{1}}}R$. Figure 13 presents isocontours of ![]() $k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ versus
$k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ versus ![]() $k_{x_{{1}}}R$ at
$k_{x_{{1}}}R$ at ![]() $x_2/R=0.7$ for
$x_2/R=0.7$ for ![]() $4100\le {{Re_{\tau }}}\le {15\,300}$ and
$4100\le {{Re_{\tau }}}\le {15\,300}$ and ![]() $10^\circ \le {\Delta {\theta }\le {210^\circ }}$. Similar to figure 11, figure 13 shows a clear dependence of the peak of
$10^\circ \le {\Delta {\theta }\le {210^\circ }}$. Similar to figure 11, figure 13 shows a clear dependence of the peak of ![]() $k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ on the Reynolds number as well as on the azimuthal separation for fixed wall-normal location. For the four wall-normal locations
$k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ on the Reynolds number as well as on the azimuthal separation for fixed wall-normal location. For the four wall-normal locations ![]() ${x_{{2}}/R}= 0.1, 0.2, 0.5, 0.7$, figures 14(a–d) were produced, representing isocontours of
${x_{{2}}/R}= 0.1, 0.2, 0.5, 0.7$, figures 14(a–d) were produced, representing isocontours of ![]() $k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ for
$k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ for ![]() ${{Re_{\tau }}}=4100$. The figure allows, for the same Reynolds number, better visualization of the effect of changing the wall-normal position and the azimuthal separation on
${{Re_{\tau }}}=4100$. The figure allows, for the same Reynolds number, better visualization of the effect of changing the wall-normal position and the azimuthal separation on ![]() $k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$. One would, clearly, observe the regions of positive and negative values of
$k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$. One would, clearly, observe the regions of positive and negative values of ![]() $k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ over various ranges of the azimuthal probe separation
$k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ over various ranges of the azimuthal probe separation ![]() ${{{\Delta }}}{\theta }$. Mostly, the negatively correlated signals occur at low and moderate streamwise wavenumbers
${{{\Delta }}}{\theta }$. Mostly, the negatively correlated signals occur at low and moderate streamwise wavenumbers ![]() $k_{x_{{1}}}R$ – however, at large enough azimuthal probe separation. The positive–negative values of
$k_{x_{{1}}}R$ – however, at large enough azimuthal probe separation. The positive–negative values of ![]() $k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ shown in both figures 13 and 14 emphasize the contribution of the LSMs to the azimuthal coherent structure. One thus could deduce from figures 11–14 clear evidence for the dependence of the cross-power spectral density and consequently the azimuthal pipe flow structure on the Reynolds number, the wall-normal location for various azimuthal separations.
$k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ shown in both figures 13 and 14 emphasize the contribution of the LSMs to the azimuthal coherent structure. One thus could deduce from figures 11–14 clear evidence for the dependence of the cross-power spectral density and consequently the azimuthal pipe flow structure on the Reynolds number, the wall-normal location for various azimuthal separations.

Figure 13. Isocontours of ![]() $k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ at fixed wall-normal location
$k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ at fixed wall-normal location ![]() ${x_{{2}}/R}=0.7$ and four Reynolds numbers: (a)
${x_{{2}}/R}=0.7$ and four Reynolds numbers: (a) ![]() ${Re_{\tau }}=4100$, (b)
${Re_{\tau }}=4100$, (b) ![]() ${Re_{\tau }}=7700$, (c)
${Re_{\tau }}=7700$, (c) ![]() ${Re_{\tau }}=11\,500$, and (d)
${Re_{\tau }}=11\,500$, and (d) ![]() ${Re_{\tau }}=15\,300$.
${Re_{\tau }}=15\,300$.

Figure 14. Isocontours of the real component of premultiplied cross-spectral density ![]() $k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ at four wall-normal locations for Reynolds number
$k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ at four wall-normal locations for Reynolds number ![]() ${{Re_{\tau }}}=4100$ and
${{Re_{\tau }}}=4100$ and ![]() $10^{\circ }\le \Delta {\theta }\le {80^{\circ }}$: (a)
$10^{\circ }\le \Delta {\theta }\le {80^{\circ }}$: (a) ![]() ${x_{{2}}/R}=0.1$, (b)
${x_{{2}}/R}=0.1$, (b) ![]() ${x_{{2}}/R}=0.2$, (c)
${x_{{2}}/R}=0.2$, (c) ![]() ${x_{{2}}/R}=0.5$, and (d)
${x_{{2}}/R}=0.5$, and (d) ![]() ${x_{{2}}/R}=0.7$.
${x_{{2}}/R}=0.7$.
In figures 11–14, at each azimuthal separation within the range ![]() ${10^{\circ }}\le {\Delta {\theta }}\le {70^{\circ }}$, positive and negative values for
${10^{\circ }}\le {\Delta {\theta }}\le {70^{\circ }}$, positive and negative values for ![]() $k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ were obtained – however, along different ranges of the streamwise wavenumber
$k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ were obtained – however, along different ranges of the streamwise wavenumber ![]() $k_{x_{{1}}}R$. To investigate further the effect of changing the azimuthal separation
$k_{x_{{1}}}R$. To investigate further the effect of changing the azimuthal separation ![]() ${\Delta {\theta }}$, the wall-normal location
${\Delta {\theta }}$, the wall-normal location ![]() ${x_{{2}}/R}$ and the Reynolds number
${x_{{2}}/R}$ and the Reynolds number ![]() ${Re_{\tau }}$ on
${Re_{\tau }}$ on ![]() $k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$, figure 15 was produced. Figure 15 consists of four plots. Figure 15(a) is a zoomed version of figure 12(d) to highlight the positive–negative/peak–trough values of
$k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$, figure 15 was produced. Figure 15 consists of four plots. Figure 15(a) is a zoomed version of figure 12(d) to highlight the positive–negative/peak–trough values of ![]() $k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ versus
$k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ versus ![]() $k_{x_{{1}}}R$ at
$k_{x_{{1}}}R$ at ![]() ${x_{{2}}/R}=0.7$ for azimuthal separations
${x_{{2}}/R}=0.7$ for azimuthal separations ![]() ${10^{\circ }}\le {\Delta {\theta }}\le {210^{\circ }}$ and
${10^{\circ }}\le {\Delta {\theta }}\le {210^{\circ }}$ and ![]() ${Re_{\tau }}=4100$. Furthermore, each plot in figures 12(a)–(c) is zoomed and treated in a similar manner to the zoomed version of figure 12(d), and the outcomes, i.e. both peak/positive and trough/negative values of
${Re_{\tau }}=4100$. Furthermore, each plot in figures 12(a)–(c) is zoomed and treated in a similar manner to the zoomed version of figure 12(d), and the outcomes, i.e. both peak/positive and trough/negative values of ![]() $k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$, for all wall-normal locations
$k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$, for all wall-normal locations ![]() $0.1\le {x_{{2}}/R}\le 0.7$ and
$0.1\le {x_{{2}}/R}\le 0.7$ and ![]() ${Re_{\tau }}=4100$, are presented in figures 15(b,c). The effect of the wall-normal location
${Re_{\tau }}=4100$, are presented in figures 15(b,c). The effect of the wall-normal location ![]() ${x_{{2}}/R}$ on either the peak/positive and trough/negative values of
${x_{{2}}/R}$ on either the peak/positive and trough/negative values of ![]() $k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ is observable in both figures 15(b,c) for the same Reynolds number. However, such peak/positive, trough/negative behaviour of
$k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ is observable in both figures 15(b,c) for the same Reynolds number. However, such peak/positive, trough/negative behaviour of ![]() $(k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++})_{peak\unicode{x2013}trough}$ is more prominent for the wall-normal locations
$(k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++})_{peak\unicode{x2013}trough}$ is more prominent for the wall-normal locations ![]() ${x_{{2}}/R}\le {0.1}$ and
${x_{{2}}/R}\le {0.1}$ and ![]() ${x_{{2}}/R}\le {0.2}$, as figure 15(b) indicates. In figure 15(c), for similar azimuthal probe separation and constant Reynolds number, a strong effect for the wall-normal location on either the peak/positive or trough/negative values of
${x_{{2}}/R}\le {0.2}$, as figure 15(b) indicates. In figure 15(c), for similar azimuthal probe separation and constant Reynolds number, a strong effect for the wall-normal location on either the peak/positive or trough/negative values of ![]() $k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ is observable, in particular for
$k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ is observable, in particular for ![]() ${x_{{2}}/R}=0.1$ and 0.2. On the other hand, the peak values of
${x_{{2}}/R}=0.1$ and 0.2. On the other hand, the peak values of ![]() $k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ attenuate fast on moving away from the pipe wall, as both figures 15(b,c) illustrate. One would also observe from figure 15(c) that along an azimuthal separation range
$k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ attenuate fast on moving away from the pipe wall, as both figures 15(b,c) illustrate. One would also observe from figure 15(c) that along an azimuthal separation range ![]() ${45^{\circ }}\le {\Delta {\theta }}\le {150^{\circ }}$,
${45^{\circ }}\le {\Delta {\theta }}\le {150^{\circ }}$, ![]() $k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ shows almost zero values, indicating that the two hot-wire signals are uncorrelated along this azimuthal separation range. Additionally, figure 15(d) represents the effect of the Reynolds number within the range
$k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ shows almost zero values, indicating that the two hot-wire signals are uncorrelated along this azimuthal separation range. Additionally, figure 15(d) represents the effect of the Reynolds number within the range ![]() ${4100}\le {{Re_{\tau }}}\le {15\,300}$ on the peak–trough values of
${4100}\le {{Re_{\tau }}}\le {15\,300}$ on the peak–trough values of ![]() $k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ at
$k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ at ![]() ${x_{{2}}/R}=0.7$ fixed wall-normal location. The figure shows a clear effect for the Reynolds number on peak/positive and/or trough/negative values of
${x_{{2}}/R}=0.7$ fixed wall-normal location. The figure shows a clear effect for the Reynolds number on peak/positive and/or trough/negative values of ![]() $k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ at similar azimuthal separation
$k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ at similar azimuthal separation ![]() $\Delta {\theta }$. Therefore, one would conclude that figure 15 is clear evidence for the dependence of the azimuthal scale structure of the LSMs on the azimuthal separation
$\Delta {\theta }$. Therefore, one would conclude that figure 15 is clear evidence for the dependence of the azimuthal scale structure of the LSMs on the azimuthal separation ![]() $\Delta {\theta }$, the wall-normal location
$\Delta {\theta }$, the wall-normal location ![]() ${x_{{2}}/R}$ and the Reynolds number
${x_{{2}}/R}$ and the Reynolds number ![]() ${Re_{\tau }}$.
${Re_{\tau }}$.

Figure 15. Peak–trough behaviour of the real component of premultiplied cross-power spectral density ![]() $k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}=f(\Delta {\theta },{x_{{2}}/R},{Re_{\tau }})$: (a) zoomed
$k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}=f(\Delta {\theta },{x_{{2}}/R},{Re_{\tau }})$: (a) zoomed ![]() $k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ versus
$k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}$ versus ![]() $k_{x_{{1}}}R$; (b)
$k_{x_{{1}}}R$; (b) ![]() $(k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++})_{peak\unicode{x2013}trough}$ versus
$(k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++})_{peak\unicode{x2013}trough}$ versus ![]() ${x_{{2}}/R}$; (c)
${x_{{2}}/R}$; (c) ![]() $(k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++})_{peak\unicode{x2013}trough}$ versus
$(k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++})_{peak\unicode{x2013}trough}$ versus ![]() $\Delta {\theta }$ for
$\Delta {\theta }$ for ![]() $0.1\le {x_{{2}}/R}\le {0.7}$; (d)
$0.1\le {x_{{2}}/R}\le {0.7}$; (d) ![]() $(k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++})_{peak\unicode{x2013}trough}$ versus
$(k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++})_{peak\unicode{x2013}trough}$ versus ![]() $\Delta {\theta }$ for
$\Delta {\theta }$ for ![]() $4.1\times {10^3}\le {{Re_{\tau }}}\le {15.3\times {10^3}}$. Plots are for (a)
$4.1\times {10^3}\le {{Re_{\tau }}}\le {15.3\times {10^3}}$. Plots are for (a) ![]() ${Re_{\tau }}=4100$ and
${Re_{\tau }}=4100$ and ![]() ${x_{{2}}/R}=0.7$, (b)
${x_{{2}}/R}=0.7$, (b) ![]() ${Re_{\tau }}=4100$ and
${Re_{\tau }}=4100$ and ![]() ${10^{\circ }}\le {\Delta {\theta }}\le {210^{\circ }}$, (c)
${10^{\circ }}\le {\Delta {\theta }}\le {210^{\circ }}$, (c) ![]() ${Re_{\tau }}=4100$ and
${Re_{\tau }}=4100$ and ![]() $0.1\le {x_{{2}}/R}\le {0.7}$, and (d)
$0.1\le {x_{{2}}/R}\le {0.7}$, and (d) ![]() $4100\le {{Re_{\tau }}}\le {15\,300}$ and
$4100\le {{Re_{\tau }}}\le {15\,300}$ and ![]() ${x_{{2}}/R}={0.7}$.
${x_{{2}}/R}={0.7}$.
To assess quantitatively the fractional contribution of the large-scale motions, i.e. VLSM and LSM, to the azimuthal pipe flow structure, a spectral filtration for the cross-power spectral density was performed; see Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008). The contribution of the VLSM was made via
where ![]() $({{k_{x_{1}}})_{cut}}$ is the streamwise wavenumber corresponding to the first peak appearing in the premultiplied power spectra presented in both figures 7 and 8, and it depends on the wall-normal location and Reynolds number. Thus the contribution of the VLSMs to the pipe flow azimuthal structure was evaluated for
$({{k_{x_{1}}})_{cut}}$ is the streamwise wavenumber corresponding to the first peak appearing in the premultiplied power spectra presented in both figures 7 and 8, and it depends on the wall-normal location and Reynolds number. Thus the contribution of the VLSMs to the pipe flow azimuthal structure was evaluated for ![]() ${{k_{x_{{1}}}}}\le {{(k_{x_{{1}}}})_{cut}}$. On the other hand, the contribution of the LSMs is examined for
${{k_{x_{{1}}}}}\le {{(k_{x_{{1}}}})_{cut}}$. On the other hand, the contribution of the LSMs is examined for ![]() ${{k_{x_{{1}}}}}\ge {{(k_{x_{{1}}}})_{cut}}$ using
${{k_{x_{{1}}}}}\ge {{(k_{x_{{1}}}})_{cut}}$ using
The azimuthal distribution of both ![]() $\rho _{{VL}}({{{\Delta }}}{s},{k_{{x}_{{1}}}})$ and
$\rho _{{VL}}({{{\Delta }}}{s},{k_{{x}_{{1}}}})$ and ![]() $\rho _{L}({{{\Delta }}}{s},{k_{{x}_{{1}}}})$ for
$\rho _{L}({{{\Delta }}}{s},{k_{{x}_{{1}}}})$ for ![]() ${{k_{x_{{1}}}}}\le {{(k_{x_{{1}}}})_{cut}}$ and
${{k_{x_{{1}}}}}\le {{(k_{x_{{1}}}})_{cut}}$ and ![]() ${{k_{x_{{1}}}}}\ge {{(k_{x_{{1}}}})_{cut}}$, respectively, are presented in figure 16 for
${{k_{x_{{1}}}}}\ge {{(k_{x_{{1}}}})_{cut}}$, respectively, are presented in figure 16 for ![]() $0.1\le {{x_{{2}}/R}}\le {0.7}$ and
$0.1\le {{x_{{2}}/R}}\le {0.7}$ and ![]() ${{Re_{\tau }}}=7700$, and compared to the azimuthal distribution of the streamwise velocity correlation
${{Re_{\tau }}}=7700$, and compared to the azimuthal distribution of the streamwise velocity correlation ![]() ${R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s}})$. For similar Reynolds numbers, a clear dependence of both
${R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s}})$. For similar Reynolds numbers, a clear dependence of both ![]() $\rho _{VL}({{{\Delta }}}{s},k_{x_{{1}}})$ and
$\rho _{VL}({{{\Delta }}}{s},k_{x_{{1}}})$ and ![]() $\rho _{L}({{{\Delta }}}{s},k_{x_{{1}}})$ on the azimuthal separation
$\rho _{L}({{{\Delta }}}{s},k_{x_{{1}}})$ on the azimuthal separation ![]() ${{{\Delta }}}{s}$ and the wall-normal location
${{{\Delta }}}{s}$ and the wall-normal location ![]() $x_{{2}}$ is observable. For small azimuthal separation
$x_{{2}}$ is observable. For small azimuthal separation ![]() ${{{\Delta }}}{s}/R\le {1}$, one would observe agreement with Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008), but with the discernible difference that the magnitude of
${{{\Delta }}}{s}/R\le {1}$, one would observe agreement with Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008), but with the discernible difference that the magnitude of ![]() $\rho _{VL}({{{\Delta }}}{s},k_{x_{{1}}})$ is greater than that of
$\rho _{VL}({{{\Delta }}}{s},k_{x_{{1}}})$ is greater than that of ![]() $\rho _{L}({{{\Delta }}}{s},k_{x_{{1}}})$, reflecting an increased contribution of the VLSM to the azimuthal pipe flow structures. This observation is also a good indication that the azimuthal scales vary with the wall-normal location as well as with the azimuthal separation. Figure 16 stresses the effect of scale averaging when using (1.1) compared to the azimuthal scales estimated based on both (3.4) and (3.5), addressing the contributions of the large-scale motions, i.e. VLSM and LSM, respectively, as will be shown later, in figure 19. However, one might observe from figure 16 changes in the magnitude and ranges of the negative values of both
$\rho _{L}({{{\Delta }}}{s},k_{x_{{1}}})$, reflecting an increased contribution of the VLSM to the azimuthal pipe flow structures. This observation is also a good indication that the azimuthal scales vary with the wall-normal location as well as with the azimuthal separation. Figure 16 stresses the effect of scale averaging when using (1.1) compared to the azimuthal scales estimated based on both (3.4) and (3.5), addressing the contributions of the large-scale motions, i.e. VLSM and LSM, respectively, as will be shown later, in figure 19. However, one might observe from figure 16 changes in the magnitude and ranges of the negative values of both ![]() $\rho _{{VL}}({{{\Delta }}}{s},k_{x_{{1}}})$ and
$\rho _{{VL}}({{{\Delta }}}{s},k_{x_{{1}}})$ and ![]() $\rho _{L}({{{\Delta }}}{s},k_{x_{{1}}})$ versus the wall-normal distance for fixed Reynolds number. Referring to figure 7(a) for
$\rho _{L}({{{\Delta }}}{s},k_{x_{{1}}})$ versus the wall-normal distance for fixed Reynolds number. Referring to figure 7(a) for ![]() ${x_{{2}}/R}=0.1$, where the VLSM is more energetic than the LSM, the VLSM contributes more to the azimuthal correlation coefficient, as figure 16(a) indicates. The contribution of the VLSM decreases, however, on moving away from the pipe wall, and on reaching
${x_{{2}}/R}=0.1$, where the VLSM is more energetic than the LSM, the VLSM contributes more to the azimuthal correlation coefficient, as figure 16(a) indicates. The contribution of the VLSM decreases, however, on moving away from the pipe wall, and on reaching ![]() ${x_{{2}}/R}=0.5$, one would observe, as figure 7(c) indicated, that the energy contained in the LSM is larger relative to that contained in the VLSM, reflecting changes in the contribution of the LSM to the azimuthal correlation coefficient
${x_{{2}}/R}=0.5$, one would observe, as figure 7(c) indicated, that the energy contained in the LSM is larger relative to that contained in the VLSM, reflecting changes in the contribution of the LSM to the azimuthal correlation coefficient ![]() $\rho _{L}({{{\Delta }}}{s},k_{x_{{1}}})$ when compared to the contribution of the VLSM, as figure 16 illustrates.
$\rho _{L}({{{\Delta }}}{s},k_{x_{{1}}})$ when compared to the contribution of the VLSM, as figure 16 illustrates.

Figure 16. Azimuthal distributions of ![]() $\rho _{VL}({{{\Delta }}}{s},k_{x_{{1}}})$ and
$\rho _{VL}({{{\Delta }}}{s},k_{x_{{1}}})$ and ![]() $\rho _{{L}}({{{\Delta }}}{s},k_{x_{{1}}})$ for (a)
$\rho _{{L}}({{{\Delta }}}{s},k_{x_{{1}}})$ for (a) ![]() ${x_{{2}}/R}= 0.1$, (b)
${x_{{2}}/R}= 0.1$, (b) ![]() ${x_{{2}}/R}=0.2$, (c)
${x_{{2}}/R}=0.2$, (c) ![]() ${x_{{2}}/R}= 0.5$ and (d)
${x_{{2}}/R}= 0.5$ and (d) ![]() ${x_{{2}}/R}= 0.7$, for Reynolds number
${x_{{2}}/R}= 0.7$, for Reynolds number ![]() ${{Re_{\tau }}}=7700$.
${{Re_{\tau }}}=7700$.
3.4. Coherence function
To investigate further the behaviour of the azimuthal large scales at some specific wavenumbers in correspondence with the VLSM and LSM, the coherence function is introduced. It is a measure of the correlation between the two streamwise velocity fluctuating signals received from both probes, but in terms of the energy contained at specific wavenumbers. Bendat & Piersol (Reference Bendat and Piersol2000) introduced the so-called magnitude-squared coherence or the coherence function, defined as
This definition of the coherence function results in loss of its negative values, which have potential to assess the azimuthal scales of the coherent structures. Thus, in alignment with Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008), we adopted the following definition of the coherence function:
quantifying the differences in the behaviour of the azimuthal scales as a result of the VLSM and LSM, where ![]() ${\varPsi ({{{{\Delta }}}{s},k_{x_{{1}}})}}$ as defined earlier is the real component of the cross-power spectral density
${\varPsi ({{{{\Delta }}}{s},k_{x_{{1}}})}}$ as defined earlier is the real component of the cross-power spectral density ![]() ${G}({{{\Delta }}}{s},k_{x_{{1}}})$,
${G}({{{\Delta }}}{s},k_{x_{{1}}})$, ![]() ${\varPhi ({{0}},k_{x_{{1}}})}$ is the auto-spectral density of the streamwise velocity fluctuations received from the fixed radial hot-wire probe, and
${\varPhi ({{0}},k_{x_{{1}}})}$ is the auto-spectral density of the streamwise velocity fluctuations received from the fixed radial hot-wire probe, and ![]() ${\varPhi ({{{{\Delta }}}{s},k_{x_{{1}}})}}$ is the auto-spectral density of the streamwise velocity fluctuations measured by the traversing azimuthal probe.
${\varPhi ({{{{\Delta }}}{s},k_{x_{{1}}})}}$ is the auto-spectral density of the streamwise velocity fluctuations measured by the traversing azimuthal probe.
It was shown earlier in power spectra presented in both figures 7 and 8 that two specific wavenumbers related to the VLSMs and LSMs were determined. Hence the coherence function ![]() $\gamma ({{{\Delta }}}{s},k_{x_{{1}}})$ should be computed at such corresponding wavenumbers. In spite of the fact that the coherence function and the spectral density functions are pairs of the Fourier transform, the coherence function is more convenient for evaluating the azimuthal scales of the LSMs; see Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008) and Bendat & Piersol (Reference Bendat and Piersol2000). Figure 17 represents the azimuthal distribution of
$\gamma ({{{\Delta }}}{s},k_{x_{{1}}})$ should be computed at such corresponding wavenumbers. In spite of the fact that the coherence function and the spectral density functions are pairs of the Fourier transform, the coherence function is more convenient for evaluating the azimuthal scales of the LSMs; see Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008) and Bendat & Piersol (Reference Bendat and Piersol2000). Figure 17 represents the azimuthal distribution of ![]() $\gamma ({{{\Delta }}}{s},k_{x_{{1}}})$ estimated at the streamwise wavenumbers corresponding to both the VLSM and the LSM at four wall-normal locations for
$\gamma ({{{\Delta }}}{s},k_{x_{{1}}})$ estimated at the streamwise wavenumbers corresponding to both the VLSM and the LSM at four wall-normal locations for ![]() ${Re_{\tau }}=4100$. At first glance, one concludes that the distribution of
${Re_{\tau }}=4100$. At first glance, one concludes that the distribution of ![]() $\gamma ({{{\Delta }}}{s},k_{x_{{1}}})$ is similar to those of
$\gamma ({{{\Delta }}}{s},k_{x_{{1}}})$ is similar to those of ![]() $R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$ shown earlier in both figures 9 and 10. It is observable from figure 17, however, that at the same azimuthal separation
$R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$ shown earlier in both figures 9 and 10. It is observable from figure 17, however, that at the same azimuthal separation ![]() ${{\Delta }}{s/R}$, the magnitude of
${{\Delta }}{s/R}$, the magnitude of ![]() $\gamma ({{{\Delta }}}{s},k_{x_{{1}}})$ has higher negative values than those for
$\gamma ({{{\Delta }}}{s},k_{x_{{1}}})$ has higher negative values than those for ![]() $R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$, reflecting the effect of the ensemble averaging of the various scales of flow motions introduced in the traditional definition of the streamwise velocity correlation
$R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$, reflecting the effect of the ensemble averaging of the various scales of flow motions introduced in the traditional definition of the streamwise velocity correlation ![]() $R_{u_{{1}}u_{{1}}}({{\Delta }}{s})$. On the contrary, the effect of the averaging in
$R_{u_{{1}}u_{{1}}}({{\Delta }}{s})$. On the contrary, the effect of the averaging in ![]() $\gamma ({{{\Delta }}}{s},k_{x_{{1}}})$ is limited to two main wavelengths corresponding to the two-mode peaks observed in both figures 7 and 8 identifying the VLSM and LSMs.
$\gamma ({{{\Delta }}}{s},k_{x_{{1}}})$ is limited to two main wavelengths corresponding to the two-mode peaks observed in both figures 7 and 8 identifying the VLSM and LSMs.

Figure 17. Azimuthal coherence function ![]() $\gamma ({{{\Delta }}}{s},k_{x_{{1}}})$ based on streamwise wavenumbers corresponding to LSMs and VLSMs at four wall-normal locations for Reynolds number
$\gamma ({{{\Delta }}}{s},k_{x_{{1}}})$ based on streamwise wavenumbers corresponding to LSMs and VLSMs at four wall-normal locations for Reynolds number ![]() ${{Re_{\tau }}}=4100$: (a)
${{Re_{\tau }}}=4100$: (a) ![]() $x_2/R=0.1$, (b)
$x_2/R=0.1$, (b) ![]() $x_2/R=0.2$, (c)
$x_2/R=0.2$, (c) ![]() $x_2/R=0.5$, and (d)
$x_2/R=0.5$, and (d) ![]() $x_2/R=0.7$.
$x_2/R=0.7$.
To quantify the azimuthal length scale (![]() $\ell _z^+$) of the LSMs, selected samples of the streamwise velocity correlation
$\ell _z^+$) of the LSMs, selected samples of the streamwise velocity correlation ![]() $R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$ for
$R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$ for ![]() ${Re_{\tau }}=4100$ and
${Re_{\tau }}=4100$ and ![]() $7700$ are presented versus the azimuthal probe separation in wall units
$7700$ are presented versus the azimuthal probe separation in wall units ![]() ${{{\Delta }}}{s^+}$ in figure 18, where
${{{\Delta }}}{s^+}$ in figure 18, where ![]() ${{{\Delta }}}{{s}^+}={{{\Delta }}}{s}\,u_{\tau }/\nu$. The
${{{\Delta }}}{{s}^+}={{{\Delta }}}{s}\,u_{\tau }/\nu$. The ![]() $R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$ correlation drops from 1 to negative values at a range of azimuthal displacement
$R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$ correlation drops from 1 to negative values at a range of azimuthal displacement ![]() ${{{\Delta }}}{s}^+\approx {100\unicode{x2013}5500}$ wall units, depending on the Reynolds number. Both plots in figure 18 show a similar trend where the value of
${{{\Delta }}}{s}^+\approx {100\unicode{x2013}5500}$ wall units, depending on the Reynolds number. Both plots in figure 18 show a similar trend where the value of ![]() $R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$ drops to negative values, indicating the occurrence of alternating high-speed and low-speed regions of velocity fluctuations in the azimuthal direction. A threshold 0.05 is proposed in figure 18 to estimate the azimuthal length scale (
$R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$ drops to negative values, indicating the occurrence of alternating high-speed and low-speed regions of velocity fluctuations in the azimuthal direction. A threshold 0.05 is proposed in figure 18 to estimate the azimuthal length scale (![]() $\ell _z^+$) of the LSMs based on
$\ell _z^+$) of the LSMs based on ![]() $R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$. In a similar manner, the azimuthal length scale is thus computed across the whole range of the wall-normal locations, i.e.
$R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$. In a similar manner, the azimuthal length scale is thus computed across the whole range of the wall-normal locations, i.e. ![]() $0.1\le {{x_{{2}}/R}}\le {0.7}$, and Reynolds numbers
$0.1\le {{x_{{2}}/R}}\le {0.7}$, and Reynolds numbers ![]() $4100\le {Re_{\tau }}\le {15\,300}$, and the results are illustrated in figures 19(a,b). The results indicate that typical azimuthal scales of the large flow structures increase in diameter with increase in the wall-normal distance, in qualitative agreement with a similar observation made by Ganapathisubramani et al. (Reference Ganapathisubramani, Hutchins, Hambleton, Longgmire and Marusic2005) for flat-plate boundary layer flow. The figure shows linear behaviour of the azimuthal growth of the large-scale structures across the logarithmic layer, in close agreement with Tomkins & Adrian (Reference Tomkins and Adrian2003), Monty et al. (Reference Monty, Stewart, Williams and Chong2007) and Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008). On the other hand, a nonlinear increase versus the wall distance through the core region, i.e.
$4100\le {Re_{\tau }}\le {15\,300}$, and the results are illustrated in figures 19(a,b). The results indicate that typical azimuthal scales of the large flow structures increase in diameter with increase in the wall-normal distance, in qualitative agreement with a similar observation made by Ganapathisubramani et al. (Reference Ganapathisubramani, Hutchins, Hambleton, Longgmire and Marusic2005) for flat-plate boundary layer flow. The figure shows linear behaviour of the azimuthal growth of the large-scale structures across the logarithmic layer, in close agreement with Tomkins & Adrian (Reference Tomkins and Adrian2003), Monty et al. (Reference Monty, Stewart, Williams and Chong2007) and Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008). On the other hand, a nonlinear increase versus the wall distance through the core region, i.e. ![]() ${x_{{2}}/R}\ge {0.5}$, is remarkable. Focusing more on the
${x_{{2}}/R}\ge {0.5}$, is remarkable. Focusing more on the ![]() $\ell _z/R$ data trend in figure 19(a), it does indeed appear nonlinear/concave-up, whereas in figure 9(a) of Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008), the
$\ell _z/R$ data trend in figure 19(a), it does indeed appear nonlinear/concave-up, whereas in figure 9(a) of Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008), the ![]() $\ell _z/\delta$ results from a couple of experiments seem quite linear, modestly far from the wall – however, overall concave-down. It is worth noting here that most of the earlier azimuthal studies did not cover either the core region of the pipe flow or the outer region of the boundary layer, thus the nonlinear behaviour of the azimuthal structure was not discovered. For instance, using high spatial resolution particle image velocimetry, Tomkins & Adrian (Reference Tomkins and Adrian2003) reported that several spanwise length scales vary, remarkably, linearly along the logarithmic layer in support of the attached-eddy hypothesis (Townsend Reference Townsend1956). Note that measurements of Tomkins & Adrian (Reference Tomkins and Adrian2003) were limited to the wall-normal distances
$\ell _z/\delta$ results from a couple of experiments seem quite linear, modestly far from the wall – however, overall concave-down. It is worth noting here that most of the earlier azimuthal studies did not cover either the core region of the pipe flow or the outer region of the boundary layer, thus the nonlinear behaviour of the azimuthal structure was not discovered. For instance, using high spatial resolution particle image velocimetry, Tomkins & Adrian (Reference Tomkins and Adrian2003) reported that several spanwise length scales vary, remarkably, linearly along the logarithmic layer in support of the attached-eddy hypothesis (Townsend Reference Townsend1956). Note that measurements of Tomkins & Adrian (Reference Tomkins and Adrian2003) were limited to the wall-normal distances ![]() $x_2/\delta \le {0.2}$. The discrepancy observed between the present finding versus the literature might be attributed partially to unequal ranges of the wall-normal distances and the Reynolds numbers. The uncertainty in calibration equations and spatial resolutions of the hot-wire probes to resolve small-scale turbulent fluctuations also has to be taken into consideration.
$x_2/\delta \le {0.2}$. The discrepancy observed between the present finding versus the literature might be attributed partially to unequal ranges of the wall-normal distances and the Reynolds numbers. The uncertainty in calibration equations and spatial resolutions of the hot-wire probes to resolve small-scale turbulent fluctuations also has to be taken into consideration.

Figure 18. The azimuthal velocity correlation ![]() $R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$ of the streamwise velocity fluctuations at four wall-normal locations
$R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$ of the streamwise velocity fluctuations at four wall-normal locations ![]() $0.1\le {{x_{{2}}/R}}\le {0.7}$ versus the azimuthal displacement in wall units
$0.1\le {{x_{{2}}/R}}\le {0.7}$ versus the azimuthal displacement in wall units ![]() ${{\Delta }}{s}^+$: (a)
${{\Delta }}{s}^+$: (a) ![]() ${Re}_{\tau }=4100$, (b)
${Re}_{\tau }=4100$, (b) ![]() ${Re}_{\tau }=7700$.
${Re}_{\tau }=7700$.

Figure 19. Normalized azimuthal length scale ![]() $\ell _z/R$ of azimuthal LSMs based on velocity correlations of the streamwise velocity fluctuations (a) compared to
$\ell _z/R$ of azimuthal LSMs based on velocity correlations of the streamwise velocity fluctuations (a) compared to ![]() $\gamma _{_{L}}({{{\Delta }}}{s},k_{x_{{1}}})$ and
$\gamma _{_{L}}({{{\Delta }}}{s},k_{x_{{1}}})$ and ![]() $\gamma _{_{VL}}({{{\Delta }}}{s},k_{x_{{1}}})$ (present data), and (b) locally scaled by
$\gamma _{_{VL}}({{{\Delta }}}{s},k_{x_{{1}}})$ (present data), and (b) locally scaled by ![]() $2{\rm \pi} {r}$ and compared to Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008) (
$2{\rm \pi} {r}$ and compared to Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008) (![]() ${Re_b}=7.6\times {10^4}\text { and } 8.3\times {10^6}$).
${Re_b}=7.6\times {10^4}\text { and } 8.3\times {10^6}$).
Moreover, Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008) claimed that the azimuthal length scale ![]() $\ell _z$ variation versus the wall-normal location
$\ell _z$ variation versus the wall-normal location ![]() $x_2$ is completely linear when scaled appropriately by the local circumference (
$x_2$ is completely linear when scaled appropriately by the local circumference (![]() $2{\rm \pi} {r}$) at a given height to account for the pipe geometry. Note that the Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008) data were limited to the wall-normal distances
$2{\rm \pi} {r}$) at a given height to account for the pipe geometry. Note that the Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008) data were limited to the wall-normal distances ![]() $0.1\le {x_2/R}\le {0.5}$. The current pipe data for
$0.1\le {x_2/R}\le {0.5}$. The current pipe data for ![]() $0.1\le {x_2/R}\le {0.7}$ are examined in a similar manner to Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008) and plotted in figure 19(b). The present
$0.1\le {x_2/R}\le {0.7}$ are examined in a similar manner to Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008) and plotted in figure 19(b). The present ![]() $\ell _z/(2 {\rm \pi}r)$ data showed very strong deviation from linearity in the core region of the pipe, i.e. for the final measurement location at
$\ell _z/(2 {\rm \pi}r)$ data showed very strong deviation from linearity in the core region of the pipe, i.e. for the final measurement location at ![]() $x_2/R = 0.7$, in which case this would constitute a significant deviation from Tomkins & Adrian (Reference Tomkins and Adrian2003), Monty et al. (Reference Monty, Stewart, Williams and Chong2007) and Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008). Figure 19(b) might support the idea that the nonlinearity is not a result of the pipe geometry alone (Townsend Reference Townsend1956), as Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008) suggested. The nonlinear behaviour observed in the present study is beyond the top of the logarithmic layer, i.e. in the core region of the pipe where the attached-eddy hypothesis deviates. However, the significant difference in the wall-normal behaviours of the azimuthal scales of the observed LSM and VLSM is in alignment with a conclusion made by Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008) that the LSM and VLSM are independent entities.
$x_2/R = 0.7$, in which case this would constitute a significant deviation from Tomkins & Adrian (Reference Tomkins and Adrian2003), Monty et al. (Reference Monty, Stewart, Williams and Chong2007) and Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008). Figure 19(b) might support the idea that the nonlinearity is not a result of the pipe geometry alone (Townsend Reference Townsend1956), as Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008) suggested. The nonlinear behaviour observed in the present study is beyond the top of the logarithmic layer, i.e. in the core region of the pipe where the attached-eddy hypothesis deviates. However, the significant difference in the wall-normal behaviours of the azimuthal scales of the observed LSM and VLSM is in alignment with a conclusion made by Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008) that the LSM and VLSM are independent entities.
3.5. Streamwise large-scale structure
Intensive efforts have been recognized – see e.g. Theodorsen (Reference Theodorsen1952), Townsend (Reference Townsend1956), Grant (Reference Grant1958), Lumley (Reference Lumley1967), Sabot et al. (Reference Sabot, Renault and Comte-Bellot1973), Cantwell (Reference Cantwell1981), Hussain (Reference Hussain1983, Reference Hussain1986), Robinson (Reference Robinson1991), Wark & Nagib (Reference Wark and Nagib1991), Kim & Adrian (Reference Kim and Adrian1999), Tomkins & Adrian (Reference Tomkins and Adrian2003), Morrison (Reference Morrison2007), Monty et al. (Reference Monty, Stewart, Williams and Chong2007), Marusic et al. (Reference Marusic, McKeon, Monkewitz, Nagib, Smits and Sreenivasan2010), Smits et al. (Reference Smits, Monty, HUltmark, Bailey, Hutchins and Marusic2011b), Chung et al. (Reference Chung, Marusic, Monty, Vallikivi and Smits2015), Baidya et al. (Reference Baidya2019) and Zanoun et al. (Reference Zanoun, Öngüner, Egbers, Bellani and Talamelli2019) – addressing questions often raised in the literature concerning the large-scale structures in wall-bounded shear flows. Nevertheless, a concrete definition of the origin, nature and time evolution of such structures is still under debate (see e.g. Marusic et al. Reference Marusic, McKeon, Monkewitz, Nagib, Smits and Sreenivasan2010; Jiménez Reference Jiménez2018), motivating further investigations to characterize, appropriately, the large-scale structures, in particular at high Reynolds numbers. For instance, a number of scenarios have been proposed by Panton (Reference Panton2001), characterizing mechanisms for the sustenance of wall turbulence which related to the turbulence structures of wall-bounded shear flows. They have been classified recently by Marusic et al. (Reference Marusic, McKeon, Monkewitz, Nagib, Smits and Sreenivasan2010) into two broad classes: one class is based on instability and transient growth mechanisms principally in the inner region, and the other is based on vortex-structure regeneration mechanisms. In alignment with the view of Marusic et al. (Reference Marusic, McKeon, Monkewitz, Nagib, Smits and Sreenivasan2010), Del Álamo & Jiménez (Reference Del Álamo and Jiménez2006) suggested that the LSMs might arise from nonlinear instabilities in pipe flow turbulence. However, the interpretation of the LSMs in most earlier studies agreed with the hairpin vortex paradigm of Theodorsen (Reference Theodorsen1952). Thus the hairpin concept might be considered a universal element or a building block of the large-scale structure of turbulent flow as proposed by Theodorsen (Reference Theodorsen1952) and later adopted by Kim & Adrian (Reference Kim and Adrian1999) and Adrian (Reference Adrian2007). And adopting the conceptual model by Kim & Adrian (Reference Kim and Adrian1999), the very-large-scale structure was defined as a consequence of coherence in the pattern of hairpin packets, i.e. the hairpin eddies that align coherently in groups to form long packets, and then packets align coherently to form the VLSMs.
In the present work, to highlight the sizes of the LSMs in the streamwise direction, intensive measurements using a single hot-wire probe were carried out, covering the inner and core regions of the pipe, i.e. ![]() ${0.04}\le {{x_{{2}}/R}}\le {1}$, through multiple radial locations. The analysis of the spectra shown earlier in both figures 7 and 8 proposed two-mode structures, i.e. a low-wavenumber mode and a moderate-wavenumber mode.
${0.04}\le {{x_{{2}}/R}}\le {1}$, through multiple radial locations. The analysis of the spectra shown earlier in both figures 7 and 8 proposed two-mode structures, i.e. a low-wavenumber mode and a moderate-wavenumber mode.
Further data analysis was therefore carried out, allowing estimations of the streamwise sizes of the large-scale structures, and the outcome is presented in figure 20. Figure 20 illustrates for different Reynolds numbers the values of the wavelengths ![]() $\lambda _{max}/R$ of the two-mode flow structures versus the wall-normal location
$\lambda _{max}/R$ of the two-mode flow structures versus the wall-normal location ![]() $x_2/R$, both normalized by the pipe radius
$x_2/R$, both normalized by the pipe radius ![]() $R$. Although the validity of Taylor's hypothesis to convert temporal hot-wire signals into spatial domain data remains a big concern (see e.g. Zaman & Hussain Reference Zaman and Hussain1981; Marusic et al. Reference Marusic, McKeon, Monkewitz, Nagib, Smits and Sreenivasan2010), it was used in the present study to estimate the sizes of the streamwise large-scale structures. Thus, based on similar analysis for the spectral peaks observed in figures 7 and 8, the sizes of the large-scale structures were estimated using the Taylor hypothesis discussed in § 3.1, and the results are presented in figure 20. Briefly, the indices/wavenumbers at which the first and second local maxima/peaks of the energy spectra occur are determined in correspondence with the VLSMs and LSMs, respectively. Thereafter, the wavelengths
$R$. Although the validity of Taylor's hypothesis to convert temporal hot-wire signals into spatial domain data remains a big concern (see e.g. Zaman & Hussain Reference Zaman and Hussain1981; Marusic et al. Reference Marusic, McKeon, Monkewitz, Nagib, Smits and Sreenivasan2010), it was used in the present study to estimate the sizes of the streamwise large-scale structures. Thus, based on similar analysis for the spectral peaks observed in figures 7 and 8, the sizes of the large-scale structures were estimated using the Taylor hypothesis discussed in § 3.1, and the results are presented in figure 20. Briefly, the indices/wavenumbers at which the first and second local maxima/peaks of the energy spectra occur are determined in correspondence with the VLSMs and LSMs, respectively. Thereafter, the wavelengths ![]() ${\lambda _{x_{{1}}}}={2{\rm \pi} }/{{k_{x_{{1}}}}}$ associated with the low and moderate wavenumbers for the very-large-scale and large-scale structures were estimated and then normalized with the pipe radius
${\lambda _{x_{{1}}}}={2{\rm \pi} }/{{k_{x_{{1}}}}}$ associated with the low and moderate wavenumbers for the very-large-scale and large-scale structures were estimated and then normalized with the pipe radius ![]() ${\lambda _{x_{{1}}}}/R$, respectively. In figure 20, it is observed that the wavelengths of those structures change significantly along the pipe wall-normal distance as well as with the Reynolds number. Figure 20(a) is a semi-logarithmic representation of the large-scale structures. Thus one might observe slight differences between the LSMs and VLSMs within the buffer layer. The figure illustrates also that the very-large-scale structures start roughly at the top of the buffer layer, in agreement with a similar conclusion made by Kim & Adrian (Reference Kim and Adrian1999), and grow rapidly in wavelength
${\lambda _{x_{{1}}}}/R$, respectively. In figure 20, it is observed that the wavelengths of those structures change significantly along the pipe wall-normal distance as well as with the Reynolds number. Figure 20(a) is a semi-logarithmic representation of the large-scale structures. Thus one might observe slight differences between the LSMs and VLSMs within the buffer layer. The figure illustrates also that the very-large-scale structures start roughly at the top of the buffer layer, in agreement with a similar conclusion made by Kim & Adrian (Reference Kim and Adrian1999), and grow rapidly in wavelength ![]() $\lambda _{x_{{1}}}$ through the logarithmic region, reaching maximum wavelengths
$\lambda _{x_{{1}}}$ through the logarithmic region, reaching maximum wavelengths ![]() $\lambda _x\approx {12}R$,
$\lambda _x\approx {12}R$, ![]() $\lambda _x\approx {16}R$ and
$\lambda _x\approx {16}R$ and ![]() $\lambda _{x_{{1}}}\approx {18}R$ for
$\lambda _{x_{{1}}}\approx {18}R$ for ![]() ${Re_{\tau }}=3200$,
${Re_{\tau }}=3200$, ![]() ${Re_{\tau }}=11\,000$ nd
${Re_{\tau }}=11\,000$ nd ![]() ${Re_{\tau }}\approx 16\,000$, respectively, at approximately half of the pipe radius
${Re_{\tau }}\approx 16\,000$, respectively, at approximately half of the pipe radius ![]() ${x_{{2}}/R}\approx {0.5}$, i.e. outside the logarithmic layer. A sudden drop in the wavelength of the VLSM occurs at
${x_{{2}}/R}\approx {0.5}$, i.e. outside the logarithmic layer. A sudden drop in the wavelength of the VLSM occurs at ![]() ${x_{{2}}/R}\approx {0.5}$, which is also in alignment with an observation made by Meinhart & Adrian (Reference Meinhart and Adrian1995) and Kim & Adrian (Reference Kim and Adrian1999) that the VLSMs are associated with the zones of uniform low momentum found in the boundary layer, which extend only up to approximately one-half of the boundary layer thickness or the pipe radius. Figure 20(b) is a linear plot, better showing the LSM along the core region of the pipe. One structure beyond
${x_{{2}}/R}\approx {0.5}$, which is also in alignment with an observation made by Meinhart & Adrian (Reference Meinhart and Adrian1995) and Kim & Adrian (Reference Kim and Adrian1999) that the VLSMs are associated with the zones of uniform low momentum found in the boundary layer, which extend only up to approximately one-half of the boundary layer thickness or the pipe radius. Figure 20(b) is a linear plot, better showing the LSM along the core region of the pipe. One structure beyond ![]() $x_2/R=0.5$, having wavelength
$x_2/R=0.5$, having wavelength ![]() $\lambda _x\approx {3R}$, is observable. Hence one concludes from figures 20(a,b) that the LSM spans the pipe cross-section from a location close to the pipe wall, i.e. within the buffer layer, to the centreline of the pipe, having wavelength
$\lambda _x\approx {3R}$, is observable. Hence one concludes from figures 20(a,b) that the LSM spans the pipe cross-section from a location close to the pipe wall, i.e. within the buffer layer, to the centreline of the pipe, having wavelength ![]() $\lambda _{x}$ around
$\lambda _{x}$ around ![]() $3R$ in plausible agreement also with Kim & Adrian (Reference Kim and Adrian1999). The constant behaviour of the LSM along the buffer and the logarithmic layers might be attributed to the attached-eddy model of wall turbulence; see e.g. Marusic & Monty (Reference Marusic and Monty2019).
$3R$ in plausible agreement also with Kim & Adrian (Reference Kim and Adrian1999). The constant behaviour of the LSM along the buffer and the logarithmic layers might be attributed to the attached-eddy model of wall turbulence; see e.g. Marusic & Monty (Reference Marusic and Monty2019).

Figure 20. (a) Semi-logarithmic and (b) linear representations of the dimensionless wavelengths of the LSMs and VLSMs versus the normalized wall-normal distances for ![]() ${2150}\le {{Re}_{\tau }}\le {16\times {10^3}}$.
${2150}\le {{Re}_{\tau }}\le {16\times {10^3}}$.
4. Discussions and conclusions
Experimental studies identifying the growth mechanisms of the azimuthal and/or spanwise flow scales are scarce, motivating the present work. To shed some light on the azimuthal scales of the pipe flow, experimental data for the streamwise velocity component have been documented in light of two-point joint statistics and spectral analysis at a pipe location where flow was assured to be fully developed for shear Reynolds number in the range ![]() $2\times {10^3}\le {{Re_{\tau }}}\le {{16\times {10^3}}}$. The streamwise velocity fluctuations were measured at two equal radial locations simultaneously, within
$2\times {10^3}\le {{Re_{\tau }}}\le {{16\times {10^3}}}$. The streamwise velocity fluctuations were measured at two equal radial locations simultaneously, within ![]() $0.1\le {x_{{2}}/R}\le {0.7}$, for various azimuthal probe separations, utilizing two single hot-wire probes. Precise in situ calibration was adopted with least-squares errors better than
$0.1\le {x_{{2}}/R}\le {0.7}$, for various azimuthal probe separations, utilizing two single hot-wire probes. Precise in situ calibration was adopted with least-squares errors better than ![]() ${\pm }1\,\%$ for both hot-wire probes. The blockage of the pipe cross-section due to probe holders during calibration and measurements ranged from 0.4 % to 2.8 % for wall-normal locations
${\pm }1\,\%$ for both hot-wire probes. The blockage of the pipe cross-section due to probe holders during calibration and measurements ranged from 0.4 % to 2.8 % for wall-normal locations ![]() ${x_{{2}}/R}=0.1$ and
${x_{{2}}/R}=0.1$ and ![]() ${x_{{2}}/R}=0.7$, respectively. The streamwise velocity component was measured with uncertainty within the ranges
${x_{{2}}/R}=0.7$, respectively. The streamwise velocity component was measured with uncertainty within the ranges ![]() ${{\pm }{0.4\,\%}}$ to
${{\pm }{0.4\,\%}}$ to ![]() ${\pm }1.6\,\%$ and
${\pm }1.6\,\%$ and ![]() ${{\pm }{0.7\,\%}}$ to
${{\pm }{0.7\,\%}}$ to ![]() ${\pm }2.67\,\%$ in the local mean velocity and the turbulence intensity level, respectively, obtained from either the fixed or the movable azimuthal hot-wire probes.
${\pm }2.67\,\%$ in the local mean velocity and the turbulence intensity level, respectively, obtained from either the fixed or the movable azimuthal hot-wire probes.
The physical mechanism of azimuthal growing of the LSMs throughout the logarithmic layer and core region shown in figure 19 can be explained well using earlier attempts made to quantify the growth of the azimuthal scales via streaks merging on an eddy-by-eddy basis: splitting, increased spacing, or coalescence and divisions; see e.g. Nakagawa & Nezu (Reference Nakagawa and Nezu1981), Smith & Metzler (Reference Smith and Metzler1983) and Wark & Nagib (Reference Wark and Nagib1991). Recent measurements by Adrian, Meinhart & Tomkins (Reference Adrian, Meinhart and Tomkins2000) revealed also a degree of vortex organization in the logarithmic layer, supporting the data in figure 19. In addition, Tomkins & Adrian (Reference Tomkins and Adrian2003) extended, experimentally, the vortex packet model in similarity to the ![]() ${\varLambda }$ vortex growth model of Perry & Chong (Reference Perry and Chong1982), observing that the structure becomes dependent on the distance from the wall at elevated Reynolds number. It was also observed by Monty et al. (Reference Monty, Stewart, Williams and Chong2007) that the hairpin structures project into the pipe flow core region, however, resulting in azimuthally large hairpin packets within it, verifying data presented in figure 19. The large spanwise scales were also shown to be related to the large streamwise scales, on average, providing a link to the results of Wark & Nagib (Reference Wark and Nagib1991), interpreting the growth of the azimuthal turbulent flow structures versus the wall-normal distance Hutchins & Marusic (Reference Hutchins and Marusic2007) and Monty et al. (Reference Monty, Stewart, Williams and Chong2007). Thus as the alignment of the large scale is believed to be the source of the VLSM, the resulting VLSM imposed within the logarithmic layer can be expected to be of wider scale, supporting the present findings.
${\varLambda }$ vortex growth model of Perry & Chong (Reference Perry and Chong1982), observing that the structure becomes dependent on the distance from the wall at elevated Reynolds number. It was also observed by Monty et al. (Reference Monty, Stewart, Williams and Chong2007) that the hairpin structures project into the pipe flow core region, however, resulting in azimuthally large hairpin packets within it, verifying data presented in figure 19. The large spanwise scales were also shown to be related to the large streamwise scales, on average, providing a link to the results of Wark & Nagib (Reference Wark and Nagib1991), interpreting the growth of the azimuthal turbulent flow structures versus the wall-normal distance Hutchins & Marusic (Reference Hutchins and Marusic2007) and Monty et al. (Reference Monty, Stewart, Williams and Chong2007). Thus as the alignment of the large scale is believed to be the source of the VLSM, the resulting VLSM imposed within the logarithmic layer can be expected to be of wider scale, supporting the present findings.
Further, to conclude, using energy spectra, azimuthal two-point velocity correlation and cross-power spectral density, the present experimental data were evaluated, resulting in the following.
(i) Two discernible peaks in energy spectra were observed: one at a low streamwise wavenumber associated with the very-large-scale motion (VLSM), and the second at moderate streamwise wavenumber associated with the large-scale motion (LSM). The two observable peaks in the energy spectra obtained are clear evidences or footprints for the LSMs in the pipe flow. Both peaks decrease in energy content with increase in the wall-normal distance; however, the energy contained in the VLSM peak decreases faster than that for the LSM for
 ${x_{{2}}/R}<{0.5}$. It was observed that the LSM peak at
${x_{{2}}/R}<{0.5}$. It was observed that the LSM peak at  ${x_{{2}}/R}\ge {0.5}$ is more prominent than the VLSM peak, which disappeared for wall-normal locations
${x_{{2}}/R}\ge {0.5}$ is more prominent than the VLSM peak, which disappeared for wall-normal locations  ${x_{{2}}/R}>{0.5}$. The physical model proposed by Meinhart & Adrian (Reference Meinhart and Adrian1995) and Kim & Adrian (Reference Kim and Adrian1999) is appropriate to interpret the disappearance of the VLSM peak beyond
${x_{{2}}/R}>{0.5}$. The physical model proposed by Meinhart & Adrian (Reference Meinhart and Adrian1995) and Kim & Adrian (Reference Kim and Adrian1999) is appropriate to interpret the disappearance of the VLSM peak beyond  $x_2/R={0.5}$, as discussed earlier. Clear dependence of the magnitude of the energy peaks at the corresponding wavenumbers for the VLSM and the LSM on both the wall-normal location and the Reynolds number was observable.
$x_2/R={0.5}$, as discussed earlier. Clear dependence of the magnitude of the energy peaks at the corresponding wavenumbers for the VLSM and the LSM on both the wall-normal location and the Reynolds number was observable.(ii) The two-point velocity correlation coefficient
 $R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$ was discussed, revealing a clear effect for the wall-normal location, the azimuthal separation; however, the weak Reynolds number effect on the azimuthal pipe flow structure was not addressed earlier in the literature. The positive–negative trends of the correlation coefficient obtained represent an indication of the vortex-packets/hairpin pipe flow structure, in agreement with similar observations made by Tomkins & Adrian (Reference Tomkins and Adrian2003) and Monty et al. (Reference Monty, Stewart, Williams and Chong2007) for flat-plate boundary layer and pipe flows, respectively.
$R_{u_{{1}}u_{{1}}}({{{\Delta }}}{s})$ was discussed, revealing a clear effect for the wall-normal location, the azimuthal separation; however, the weak Reynolds number effect on the azimuthal pipe flow structure was not addressed earlier in the literature. The positive–negative trends of the correlation coefficient obtained represent an indication of the vortex-packets/hairpin pipe flow structure, in agreement with similar observations made by Tomkins & Adrian (Reference Tomkins and Adrian2003) and Monty et al. (Reference Monty, Stewart, Williams and Chong2007) for flat-plate boundary layer and pipe flows, respectively.(iii) Appropriate contributions of the LSMs to the azimuthal pipe flow structures were treated using the cross-power spectral density as a function of the wall-normal location, the azimuthal separation and the Reynolds number. The real part of the cross-power spectral density normalized
 ${k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}}$ as a function of the azimuthal separation and the streamwise wavenumber, indicating a peak–trough mode for various wall-normal locations and Reynolds numbers. The peak was observed to attenuate rapidly for wall-normal locations
${k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}}$ as a function of the azimuthal separation and the streamwise wavenumber, indicating a peak–trough mode for various wall-normal locations and Reynolds numbers. The peak was observed to attenuate rapidly for wall-normal locations  ${x_{{2}}/R}\le {0.2}$, and in particular for azimuthal separation
${x_{{2}}/R}\le {0.2}$, and in particular for azimuthal separation  $\Delta {\theta } \le {45}^{\circ }$. Such rapid decay observed in
$\Delta {\theta } \le {45}^{\circ }$. Such rapid decay observed in  ${k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}}$ might be interpreted by the hairpin vortex model proposed by Theodorsen (Reference Theodorsen1952). On the contrary, for the wall-normal locations
${k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}}$ might be interpreted by the hairpin vortex model proposed by Theodorsen (Reference Theodorsen1952). On the contrary, for the wall-normal locations  ${x_{{2}}/R}>{0.2}$,
${x_{{2}}/R}>{0.2}$,  ${k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}}$ showed slow rate of decay versus the azimuthal separation
${k_{x_{{1}}}R{\varPsi }_{u_{{1}}u_{{1}}}^{++}}$ showed slow rate of decay versus the azimuthal separation  $\Delta {\theta }$, which could be attributed to the relatively long lifetimes of the azimuthal vortices found predominantly in the pipe core region. A clear dependence of the cross-power spectral density and consequently the azimuthal-scale structure of the LSMs on the azimuthal separation
$\Delta {\theta }$, which could be attributed to the relatively long lifetimes of the azimuthal vortices found predominantly in the pipe core region. A clear dependence of the cross-power spectral density and consequently the azimuthal-scale structure of the LSMs on the azimuthal separation  ${\Delta {\theta }}$, the wall-normal location
${\Delta {\theta }}$, the wall-normal location  ${x_{{2}}/R}$ and the Reynolds number
${x_{{2}}/R}$ and the Reynolds number  ${Re_{\tau }}$ was emphasized.
${Re_{\tau }}$ was emphasized.(iv) Spectral filtration for the cross-power spectral density was discussed, addressing well the energetic contribution of the large-scale motions, i.e. VLSM and LSM, to the azimuthal pipe flow structures. The contribution of the LSMs was observed to change on moving away from the pipe wall, indicating that the azimuthal scales vary with the wall-normal location as well as with the azimuthal separation for similar Reynolds numbers.
(v) The coherence function was discussed to address the behaviour of the azimuthal structure due to the LSMs. The azimuthal scales were identified in correspondence with wavenumbers that have been associated with the two-mode peaks observed in the energy spectra of the streamwise velocity fluctuations. They were computed along the four wall-normal locations
 $0.1\le {{x_{{2}}/R}}\le {0.7}$, showing sizes in the range
$0.1\le {{x_{{2}}/R}}\le {0.7}$, showing sizes in the range  $0.1\le {\ell _z^+}\le {0.5}R$. The azimuthal scales increase in diameter with increase in the wall-normal distance, in qualitative agreement with a similar observation made by Ganapathisubramani et al. (Reference Ganapathisubramani, Hutchins, Hambleton, Longgmire and Marusic2005) for flat-plate boundary layer flow. A linear increase in the azimuthal length scale through the log region was obtained, in plausible agreement with Tomkins & Adrian (Reference Tomkins and Adrian2003), Monty et al. (Reference Monty, Stewart, Williams and Chong2007) and Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008). On the other hand, considering the wall-normal locations
$0.1\le {\ell _z^+}\le {0.5}R$. The azimuthal scales increase in diameter with increase in the wall-normal distance, in qualitative agreement with a similar observation made by Ganapathisubramani et al. (Reference Ganapathisubramani, Hutchins, Hambleton, Longgmire and Marusic2005) for flat-plate boundary layer flow. A linear increase in the azimuthal length scale through the log region was obtained, in plausible agreement with Tomkins & Adrian (Reference Tomkins and Adrian2003), Monty et al. (Reference Monty, Stewart, Williams and Chong2007) and Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008). On the other hand, considering the wall-normal locations  $0.1\le {{x_{{2}}/R}}\le {0.7}$, a nonlinear increase in azimuthal scales versus the wall distance was remarkable in the core region of the pipe. The nonlinearity behaviour observed is beyond the top of the log layer, i.e. in the core region of the pipe where the attached-eddy hypothesis deviates, constituting also a significant deviation from experimental data in the literature, e.g. Ganapathisubramani et al. (Reference Ganapathisubramani, Hutchins, Hambleton, Longgmire and Marusic2005), Monty et al. (Reference Monty, Stewart, Williams and Chong2007) and Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008). The azimuthal scales of both the VLSM and LSM provided a basis proposing that the large-scale structures are independent entities in alignment with Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008), and supporting the possibility that such large structures arise from linear and/or nonlinear models within the turbulent flow; see Jiménez, Del Álamo & Flores (Reference Jiménez, Del Álamo and Flores2004) and Del Álamo & Jiménez (Reference Del Álamo and Jiménez2006).
$0.1\le {{x_{{2}}/R}}\le {0.7}$, a nonlinear increase in azimuthal scales versus the wall distance was remarkable in the core region of the pipe. The nonlinearity behaviour observed is beyond the top of the log layer, i.e. in the core region of the pipe where the attached-eddy hypothesis deviates, constituting also a significant deviation from experimental data in the literature, e.g. Ganapathisubramani et al. (Reference Ganapathisubramani, Hutchins, Hambleton, Longgmire and Marusic2005), Monty et al. (Reference Monty, Stewart, Williams and Chong2007) and Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008). The azimuthal scales of both the VLSM and LSM provided a basis proposing that the large-scale structures are independent entities in alignment with Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008), and supporting the possibility that such large structures arise from linear and/or nonlinear models within the turbulent flow; see Jiménez, Del Álamo & Flores (Reference Jiménez, Del Álamo and Flores2004) and Del Álamo & Jiménez (Reference Del Álamo and Jiménez2006).(vi) Additionally, the streamwise scales of the LSMs were identified using the auto-spectral energy analysis in correspondence with wavenumbers associated with the two-mode peaks observed in the energy spectra of the streamwise velocity fluctuations. The streamwise VLSMs and LSMs showed wavelengths
 ${\approx }{18}R$ and
${\approx }{18}R$ and  ${\approx }{3}R$, for
${\approx }{3}R$, for  ${Re_{\tau }}\approx {16\times {10^3}}$ at half of the pipe radius, respectively. The streamwise large-scale structure, however, spans from the buffer layer to the centreline of the pipe, partially in alignment with the wall-attached-eddy concept.
${Re_{\tau }}\approx {16\times {10^3}}$ at half of the pipe radius, respectively. The streamwise large-scale structure, however, spans from the buffer layer to the centreline of the pipe, partially in alignment with the wall-attached-eddy concept.
To summarize, the present experiments emphasize clear dependence of the azimuthal scales on the Reynolds number and the azimuthal separation, as well as on the wall-normal location, that has not been reported previously. The present results in the pipe core/outer region disagree with experiments in the literature, none of which showed a nonlinear increase in azimuthal and/or spanwise length scales with increasing wall-normal distance. The streamwise LSMs were addressed, showing also clear dependence on both the wall-normal locations and the Reynolds number.
Acknowledgements
The authors thank Dr P. Szabo (LAS, BTU C-S) for his careful reading of the manuscript and constructive comments.
Funding
E.-S.Z. and Y.D. are supported by the Priority Programme (no. DFG-SPP 1881) ‘Turbulent Superstructures’ of the Deutsche Forschungsgemeinschaft (grant nos EG100/24-1, 100/24-2).
Declaration of interests
The authors report no conflict of interest.
Appendix A. Uncertainty in energy spectra of the streamwise velocity fluctuations
It is evident that precise measurement of higher-order streamwise velocity correlations requires calibrating hot-wire probes in potential flows with a large number of independent data points. The streamwise instantaneous velocity component ![]() $\hat {U}_{{1}}$ can be written as
$\hat {U}_{{1}}$ can be written as
where ![]() $\bar {U}_{{1}}$ is the ensemble-averaged streamwise velocity, and
$\bar {U}_{{1}}$ is the ensemble-averaged streamwise velocity, and ![]() $u_{{1}}$ represents the streamwise velocity fluctuation. The instantaneous hot-wire output
$u_{{1}}$ represents the streamwise velocity fluctuation. The instantaneous hot-wire output ![]() $\hat {E}$ can be also written as
$\hat {E}$ can be also written as
where ![]() $\bar {E}$ is the average hot-wire voltage output, and
$\bar {E}$ is the average hot-wire voltage output, and ![]() $e'$ represents fluctuations in hot-wire output; both are due to the streamwise velocity component. The instantaneous hot-wire output
$e'$ represents fluctuations in hot-wire output; both are due to the streamwise velocity component. The instantaneous hot-wire output ![]() $\hat {E}$ is related to the instantaneous streamwise velocity component
$\hat {E}$ is related to the instantaneous streamwise velocity component ![]() $\hat {U}_{{1}}$ based on the power-law calibration function as follows:
$\hat {U}_{{1}}$ based on the power-law calibration function as follows:
where ![]() $A$,
$A$, ![]() $B$ and
$B$ and ![]() $n$ are constants. Using the Taylor series expansion, the hot-wire output
$n$ are constants. Using the Taylor series expansion, the hot-wire output ![]() $\hat {E}(\hat {U}_{{1}})$ can be expanded around the mean of the streamwise velocity component
$\hat {E}(\hat {U}_{{1}})$ can be expanded around the mean of the streamwise velocity component ![]() $\bar {U}_{{1}}$ as
$\bar {U}_{{1}}$ as
 \begin{equation}
\hat{E}(\hat{U}_{{1}})=\bar{E}(\bar{U}_{{1}})
+\left[\frac{\partial{\hat{E}(\hat{U}_{{1}})}}{\partial{\hat{U}_{{1}}}}\right]_{\hat{U}_{{1}}
={\bar{U}_{{1}}}}(\hat{U}_{{1}}-\bar{U}_{{1}})+{{\rm HOT}}.
\end{equation}
\begin{equation}
\hat{E}(\hat{U}_{{1}})=\bar{E}(\bar{U}_{{1}})
+\left[\frac{\partial{\hat{E}(\hat{U}_{{1}})}}{\partial{\hat{U}_{{1}}}}\right]_{\hat{U}_{{1}}
={\bar{U}_{{1}}}}(\hat{U}_{{1}}-\bar{U}_{{1}})+{{\rm HOT}}.
\end{equation}The constant temperature anemometer features significant nonlinearity that might require considering higher-order terms (HOT) in the Taylor series expansion. However, for the sake of simplicity, neglecting the HOT results in
 \begin{equation} \hat{E}(\hat{U}_{{1}})-\bar{E}(\bar{U}_{{1}})\approx{\left[\frac{\partial{\hat{E}}}{\partial{\hat{U}}}\right]_{\hat{U}={\bar{U}_{{1}}}}(\hat{U}_{{1}}-\bar{U})}\quad \Rightarrow\quad e'\approx{\left[\frac{\partial{\hat{E}}}{\partial{\hat{U}}}\right]_{\hat{U}={\bar{U}_{{1}}}}u_{{1}}}. \end{equation}
\begin{equation} \hat{E}(\hat{U}_{{1}})-\bar{E}(\bar{U}_{{1}})\approx{\left[\frac{\partial{\hat{E}}}{\partial{\hat{U}}}\right]_{\hat{U}={\bar{U}_{{1}}}}(\hat{U}_{{1}}-\bar{U})}\quad \Rightarrow\quad e'\approx{\left[\frac{\partial{\hat{E}}}{\partial{\hat{U}}}\right]_{\hat{U}={\bar{U}_{{1}}}}u_{{1}}}. \end{equation}Differentiating (A3) results in
 \begin{equation} {\left[\frac{\partial{\hat{E}}}{\partial{\hat{U}_{{1}}}}\right]_{\hat{U}_{{1}}={\bar{U}_{{1}}}}=\frac{nB\bar{U}_{{1}}^{n-1}}{2\bar{E}}}. \end{equation}
\begin{equation} {\left[\frac{\partial{\hat{E}}}{\partial{\hat{U}_{{1}}}}\right]_{\hat{U}_{{1}}={\bar{U}_{{1}}}}=\frac{nB\bar{U}_{{1}}^{n-1}}{2\bar{E}}}. \end{equation}Substituting (A6) into (A5) and rearranging results in the streamwise turbulence intensity
The vigour/intensity of turbulence is usually evaluated via velocity fluctuations ![]() $u_{{1}}$. Moreover, errors in estimating the mean values of the streamwise velocity
$u_{{1}}$. Moreover, errors in estimating the mean values of the streamwise velocity ![]() $\bar {U}_{{1}}$ and hot-wire output
$\bar {U}_{{1}}$ and hot-wire output ![]() $\bar {E}$ are negligible compared to the error in measuring hot-wire fluctuations
$\bar {E}$ are negligible compared to the error in measuring hot-wire fluctuations ![]() $e'$. Thus one can write the turbulent kinetic energy or energy spectra
$e'$. Thus one can write the turbulent kinetic energy or energy spectra ![]() $u_{{1}}u_{{1}}$ of the streamwise velocity fluctuations as
$u_{{1}}u_{{1}}$ of the streamwise velocity fluctuations as
where ![]() $C=[{2\bar {E}{\bar {U}_{{1}}}}/{n(\bar {E}^2-A)}]^2$. To determine the sensitivity of the turbulent kinetic energy
$C=[{2\bar {E}{\bar {U}_{{1}}}}/{n(\bar {E}^2-A)}]^2$. To determine the sensitivity of the turbulent kinetic energy ![]() $u_{{1}}u_{{1}}$ of the streamwise velocity fluctuations to any small perturbations/errors in hot-wire fluctuations
$u_{{1}}u_{{1}}$ of the streamwise velocity fluctuations to any small perturbations/errors in hot-wire fluctuations ![]() $\epsilon _{e'}$ in (A8), the following notations were introduced to estimate how far the measured energy
$\epsilon _{e'}$ in (A8), the following notations were introduced to estimate how far the measured energy ![]() $u_{{1}}u_{{1}}$ deviates from the true value:
$u_{{1}}u_{{1}}$ deviates from the true value:
where ![]() $\epsilon$ refers to the perturbation or error in the measured quantity, while parameters with an asterisk indicate exact values. Introducing the notations used in (A9a,b) into (A8) results in
$\epsilon$ refers to the perturbation or error in the measured quantity, while parameters with an asterisk indicate exact values. Introducing the notations used in (A9a,b) into (A8) results in
From (A8) and (A10), one can derive the following expression for the error estimation in the turbulent kinetic energy or the energy spectra:
The partial derivatives of ![]() $\epsilon _{u_{{1}}u_{{1}}}$ with respect to the perturbation parameter,
$\epsilon _{u_{{1}}u_{{1}}}$ with respect to the perturbation parameter, ![]() $\epsilon _{e'}$ give
$\epsilon _{e'}$ give
This principal analysis indicates a direct linear relation between error introduced into the turbulent kinetic energy/energy spectra of the streamwise velocity fluctuations ![]() $u_{{1}}u_{{1}}$ and error in fluctuating hot-wire output
$u_{{1}}u_{{1}}$ and error in fluctuating hot-wire output ![]() $e'$. Thus a
$e'$. Thus a ![]() ${\pm }{10\,\%}$ calibration error or uncertainty results in
${\pm }{10\,\%}$ calibration error or uncertainty results in ![]() ${\approx }{\pm {20\,\%}}$ error in the turbulent energy spectra of the streamwise velocity fluctuations measured and consequently on scales of the turbulence structures.
${\approx }{\pm {20\,\%}}$ error in the turbulent energy spectra of the streamwise velocity fluctuations measured and consequently on scales of the turbulence structures.
Appendix B. Comparative summary
Due to some common similarities and differences between the present study and the earlier work done by Bailey et al. (Reference Bailey, Hultmark, Smits and Schultz2008), the authors present in table 3 a short comparison between the two studies.
Table 3. A comparative summary, where ![]() $\ell ^+=\ell {u_{\tau }}/\nu$ is the hot-wire length in wall units, and
$\ell ^+=\ell {u_{\tau }}/\nu$ is the hot-wire length in wall units, and ![]() $\ell _c$ is the viscous length scale.
$\ell _c$ is the viscous length scale.