Hostname: page-component-cd9895bd7-jn8rn Total loading time: 0 Render date: 2024-12-28T11:42:12.187Z Has data issue: false hasContentIssue false
  • English
  • Français

Relaxation and coalescence of two equal-sized viscous drops in a quiescent matrix

Published online by Cambridge University Press:  25 January 2012

Carolina Vannozzi
Carolina Vannozzi*
Affiliation:
Chemical Engineering Department, University of California Santa Barbara, Santa Barbara, CA 93106-5080, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Head-on collisions of two equal-sized viscous drops in a biaxial extensional flow were simulated using the boundary integral method in the Stokes flow limit, for capillary numbers of the order of 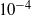–, typical of flow-induced coalescence experiments. At a certain point in time, during the drainage process, the flow was abruptly stopped and the time-dependent dynamics of drop deformation (relaxation) was followed to discern whether the pair of drops would eventually coalesce. The concept of coalescence probability was used to study the evolution of probable collisions. The polymeric system of polybutadiene (PBd) drops undergoing head-on collisions in a polydimethylsiloxane (PDMS) matrix, previously well-characterized both experimentally and numerically by Yoon et al. (Phys. Fluid, vol. 19, 2007, 102102), in which both fluids were Newtonian under the experimental conditions, was used as our reference. Film shapes, velocity profiles and pressure distributions were studied for initially parabolic or dimpled thin film shapes. It was shown that micrometre-sized drops undergoing relaxation can coalesce in the capillary number range studied, which also included cases of hindered coalescence and cases in which the flow interaction time for the collision was smaller than the drainage time; thus, this phenomenon could influence the final drop size distribution of blends. Further, these findings could be of interest in interpreting stop–strain experiments, in the case of a sudden change in flow conditions and in population balance studies of drops in blends.

JFM classification

Drops and Bubbles: Breakup/coalescence Low-Reynolds-number flows: Low-Reynolds-number flows Interfacial Flows (free surface): Thin films
Type
Papers
Information
Journal of Fluid Mechanics , Volume 694 , 10 March 2012 , pp. 408 - 425
Copyright
Copyright © Cambridge University Press 2012

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Assighaou, S. & Benyahia, L. 2008 Universal retraction process of a droplet shape after a large strain jump. Phys. Rev. E 77, 036305.CrossRefGoogle ScholarPubMed
2. Baldessari, F. & Leal, L. G. 2006 Effect of overall drop deformation on flow-induced coalescence at low capillary numbers. Phys. Fluids 18, 013602.CrossRefGoogle Scholar
3. Borrell, M., Yoon, Y. & Leal, L. G. 2004 Experimental analysis of the coalescence process via head-on collisions in a time-dependent flow. Phys. Fluids 16, 3945.CrossRefGoogle Scholar
4. Bremond, N., Thiam, A. R. & Bibette, J. 2008 Decompressing emulsion droplets favours coalescence. Phys. Rev. Lett. 100, 024501.CrossRefGoogle ScholarPubMed
5. Chesters, A. K. 1991 The modeling of coalescence processes in fluid liquid dispersions – a review of current understanding. Chem. Engng Res. Des. 69, 259–270.Google Scholar
6. Cristini, V., Blawzdziewiczy, J. & Loewenberg, M. 1998 Near-contact motion of surfactant-covered spherical drops. J. Fluid Mech. 366, 259–287.CrossRefGoogle Scholar
7. Dai, B. & Leal, L. G. 2008 The mechanism of surfactant effects on drop coalescence. Phys. Fluids 20, 040802.CrossRefGoogle Scholar
8. Eggleton, C. D., Pawar, Y. P. & Stebe, K. J. 1999 Insoluble surfactants on a drop in an extensional flow: a generalization of the stagnated surface limit to deforming interfaces. J. Fluid Mech. 385, 79–99.CrossRefGoogle Scholar
9. Eggleton, C. D., Tsai, T. M. & Stebe, K. J. 2001 Tip streaming from a drop in the presence of surfactants. Phys. Rev. Lett. 87, 048302.CrossRefGoogle ScholarPubMed
10. Fortelny, I. & Zivny, A. 2003 Extensional flow induced coalescence in polymer blends. Rheol. Acta 42, 454–461.CrossRefGoogle Scholar
11. Gunes, D. Z., Clain, X., Breton, O., Mayor, G. & Burbidge, A. S. 2010 Avalanches of coalescence events and local extensional flows – stabilization or destabilization due to surfactant. J. Colloid Interface Sci. 343, 79–86.CrossRefGoogle ScholarPubMed
12. Hu, Y. T., Pine, D. J. & Leal, L. G. 2000 Drop deformation, breakup, and coalescence with compatibilizer. Phys. Fluids 12, 484.CrossRefGoogle Scholar
13. Janssen, P. J. A., Anderson, P. D., Peters, G. W. M. & Meijer, H. E. H. 2006 Axisymmetric boundary integral simulations of film drainage between two viscous drops. J. Fluid Mech. 567, 65–90.CrossRefGoogle Scholar
14. Kruijt-Stegeman, Y. W., Van de Vosse, F. N. & Meijer, H. E. H. 2004 Droplet behavior in the presence of insoluble surfactants. Phys. Fluids 16, 2785.CrossRefGoogle Scholar
15. Lai, A., Bremond, N. & Stone, H. A. 2009 Separation-driven coalescence of droplets: an analytical criterion for the approach to contact. J. Fluid Mech. 632, 97–107.CrossRefGoogle Scholar
16. Leal, L. G. 2004 Flow induced coalescence of drops in a viscous fluid. Phys. Fluids 16, 1833–1851.CrossRefGoogle Scholar
17. Leal, L. G. 2007 Advanced Transport Phenomena: Fluid Mechanics and Convective Transport Processes. Cambridge Series in Chemical Engineering , Cambridge University Press.CrossRefGoogle Scholar
18. Nemer, M. B., Chen, X., Papadopoulos, D. H., Blawzdziewicz, J. & Loewenberg, M. 2004 Hindered and enhanced coalescence of drops in Stokes flows. Phys. Rev. Lett. 92, 114501.CrossRefGoogle ScholarPubMed
19. Pawar, Y. P. & Stebe, K. J. 1996 Marangoni effects on drop deformation in an extensional flow: the role of surfactant physical chemistry I. Insoluble surfactants. Phys. Fluids 8, 1738.CrossRefGoogle Scholar
20. Pozrikidis, C. 1992 Boundary Integral and Singularity Methods for Linearized Viscous Flow. Cambridge University Press.CrossRefGoogle Scholar
21. Rallison, J. M. & Acrivos, A. 1978 A numerical study of the deformation and burst of a viscous drop in an extensional flow. J. Fluid Mech. 89, 191–200.CrossRefGoogle Scholar
22. Rallison, J. M. 1984 The deformation of small viscous drops and bubbles in shear flows. Annu. Rev. Fluid Mech. 16, 45–66.CrossRefGoogle Scholar
23. Stone, H. A. 1994 Dynamics of drop deformation and breakup in viscous fluids. Annu. Rev. Fluid Mech. 26, 65–102.CrossRefGoogle Scholar
24. Stone, H. A., Bentley, B. J. & Leal, L. G. 1986 An experimental-study of transient effects in the breakup of viscous drops. J. Fluid Mech. 173, 131–158.CrossRefGoogle Scholar
25. Stone, H. A. & Leal, L. G. 1989a Relaxation and breakup of an initially extended drop in an otherwise quiescent fluid. J. Fluid Mech. 198, 399–427.CrossRefGoogle Scholar
26. Stone, H. A. & Leal, L. G. 1989b A note concerning drop deformation and break up in biaxial extensional flows at low Reynolds numbers. J. Colloid Interface Sci. 133, 340–347.CrossRefGoogle Scholar
27. Sundararaj, U. & Macosko, C. W. 1995 Drop breakup and coalescence in polymer blends - the effects of concentration and compatibilization. Macromolecules 28 (8), 2647–2657.CrossRefGoogle Scholar
28. Taylor, G. I. 1932 The viscosity of a fluid containing small drops of another fluid. Proc. R. Soc. Lond. Ser. A 138, 41–48.Google Scholar
29. Taylor, G. I. 1934 The formation of emulsions in definable fields of flow. Proc. R. Soc. Lond. Ser. A 146, 501–523.Google Scholar
30. Vinckier, I., Moldenaers, P., Terracciano, A. M. & Grizzuti, N. 1998 Droplet size evolution during coalescence in semiconcentrated model blends. AICHE J. 44, 951–958.CrossRefGoogle Scholar
31. Yang, H., Park, C. C., Hu, Y. T. & Leal, L. G. 2001 The coalescence of two equal-sized drops in a two-dimensional linear flow. Phys. Fluids 13, 1087–1106.CrossRefGoogle Scholar
32. Yoon, Y., Baldessari, F., Ceniceros, H. D. & Leal, L. G. 2007 Coalescence of two equal-sized deformable drops in an axisymmetric flow. Phys. Fluids 19, 102102.CrossRefGoogle Scholar