Hostname: page-component-78c5997874-xbtfd Total loading time: 0 Render date: 2024-11-17T13:19:53.045Z Has data issue: false hasContentIssue false
  • English
  • Français

Reduced-order analysis of buffet flow of space launchers

Published online by Cambridge University Press:  14 February 2017

Vladimir Statnikov ,
Matthias Meinke  and
Wolfgang Schröder
Vladimir Statnikov*
Affiliation:
Institute of Aerodynamics and Chair of Fluid Mechanics, RWTH Aachen University, Wüllnerstr. 5a, 52062 Aachen, Germany
Matthias Meinke
Affiliation:
Institute of Aerodynamics and Chair of Fluid Mechanics, RWTH Aachen University, Wüllnerstr. 5a, 52062 Aachen, Germany
Wolfgang Schröder
Affiliation:
Institute of Aerodynamics and Chair of Fluid Mechanics, RWTH Aachen University, Wüllnerstr. 5a, 52062 Aachen, Germany
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

A reduced-order analysis based on optimized dynamic mode decomposition (DMD) is performed on the turbulent wake of a generic axisymmetric space launcher configuration computed via a zonal large-eddy simulation at the free stream Mach number 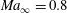$Ma_{\infty }=0.8$ and the Reynolds number based on the main body diameter 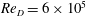$Re_{D}=6\times 10^{5}$ to investigate the buffet phenomenon. The transonic wake is characterized by an unsteady recirculation region occurring around the nozzle due to the separation of the turbulent boundary layer at the main body shoulder and subsequent dynamic interaction of the unstable free-shear layer with the nozzle surface. This results in strongly periodic and antisymmetric wall pressure fluctuations, for which three distinct frequency ranges are identified using conventional spectral analysis, i.e. 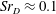$Sr_{D}\approx 0.1$, 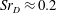$Sr_{D}\approx 0.2$ and 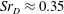$Sr_{D}\approx 0.35$. For the spatially integrated side (buffet) loads on the nozzle, the second range is found to be energetically most dominant. To clarify the origin of the detected wake dynamics, the underlying spatio-temporal coherent modes are extracted using DMD. Subsequent analysis of the reduced-order modelled flow field based on the identified DMD modes reveals that at $Sr_{D}\approx 0.1$ a longitudinal cross-pumping motion of the separation bubble takes place, caused by a harmonic antisymmetric oscillation of the main recirculation vortex in the streamwise direction. At $Sr_{D}\approx 0.2$, a cross-flapping motion of the shear layer is determined, triggered by antisymmetric vortex shedding which is in phase with the cross-pumping motion such that it occurs at twice the frequency value. The last range of 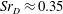$Sr_{D}\approx 0.35$ is attributed to a swinging motion of the shear layer caused by a higher harmonic of the vortex shedding mode. Conclusively, the controversial aspect of the true three-dimensional shape of the antisymmetric mode at $Sr_{D}\approx 0.2$ that dominates the buffet phenomenon is scrutinized. Inclined elongated closed-loop vortices are identified that are shed in alternating sequence from azimuthally opposite positions in a longitudinal plane of symmetry that changes its momentary orientation irregularly, maintaining an axisymmetric time-averaged field and spatially isotropic buffet loads.

JFM classification

Nonlinear Dynamical Systems: Low-dimensional models Nonlinear Dynamical Systems: Nonlinear Dynamical Systems Wakes/Jets: Wakes/Jets
Type
Papers
Information
Journal of Fluid Mechanics , Volume 815 , 25 March 2017 , pp. 1 - 25
Check for updates
Copyright
© 2017 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Alkishriwi, N., Meinke, M. & Schröder, W. 2006 A large-eddy simulation method for low Mach number flows using preconditioning and multigrid. Comput. Fluids 35 (10), 1126–1136.CrossRefGoogle Scholar
Ariane Space2003 Report on Flight 157 – Ariane 5 ECA, Tech. Rep. European Space Agency.Google Scholar
Boris, J., Grinstein, F., Oran, E. & Kolbe, R. 1992 New insights into large eddy simulation. Fluid Dyn. Res. 10, 199–228.CrossRefGoogle Scholar
Boyd, S. & Vandenberghe, L. 2004 Convex Optimization. Cambridge University Press.CrossRefGoogle Scholar
Brown, G. L. & Roshko, A. 1974 On density effects and large structure in turbulent mixing layers. J. Fluid Mech. 64, 775–816.CrossRefGoogle Scholar
Choi, H. & Moin, P. 2012 Grid-point requirements for large eddy simulation: Chapman’s estimates revisited. Phys. Fluids 24, 011702.CrossRefGoogle Scholar
Coe, C. F. & Nute, J. B.1962 Steady and fluctuating pressures at transonic speeds on hammerhead launch vehicles. NASA Tech. Memo No. TMX-778.Google Scholar
David, C. & Radulovic, S. 2005 Prediction of buffet loads on the Ariane 5 afterbody. In 6th International Symposium on Launcher Technologies, Munich. CNES.Google Scholar
Deck, S. & Thorigny, P. 2007 Unsteadiness of an axisymmetric separating-reattaching flow: numerical investigation. Phys. Fluids 19, 065103.CrossRefGoogle Scholar
Deprés, D., Reijasse, P. & Dussauge, J.-P. 2004 Analysis of unsteadiness in afterbody transonic flows. AIAA J. 42 (12), 2541–2550.CrossRefGoogle Scholar
Fares, E. & Schröder, W. 2004 A general one-equation turbulence model for free shear and wall-bounded flows. Flow Turbul. Combust. 73, 187–215.Google Scholar
Grilli, M., Schmid, P. J., Hickel, S. & Adams, N. A. 2012 Analysis of unsteady behaviour in shockwave turbulent boundary layer interaction. J. Fluid Mech. 700, 16–28.CrossRefGoogle Scholar
Hannemann, K., Lüdeke, H., Pallegoix, J.-F., Ollivier, A., Lambare, H., Maseland, H., Geurts, E. G. M., Frey, M., Deck, S., Schrijer, F. F. J. et al. 2011 Launch vehicle base buffeting – recent experimental and numerical investigations. In Proceedings of the 7th European Symposium on Aerothermodynamics for Space Vehicles, Brugge, Belgium 9–12 May, vol. 692, p. 102. European Space Agency.Google Scholar
Hua, J.-C., Gunaratne, G. H., Talley, D. G., Gord, J. R. & Roy, S. 2016 Dynamic-mode decomposition based analysis of shear coaxial jets with and without transverse acoustic driving. J. Fluid Mech. 790, 5–32.CrossRefGoogle Scholar
Jarrin, N., Benhamadouche, N., Laurence, S. & Prosser, D. 2006 A synthetic-eddy-method for generating inflow conditions for large-eddy simulations. Intl J. Heat Fluid Flow 27, 585–593.CrossRefGoogle Scholar
Jones, G. W. Jr. & Foughner, J. T. Jr. 1963 Investigation of buffet pressures on models of large manned launch vehicle configurations NASA Tech. Note D-1633.Google Scholar
Jovanović, M. R., Schmid, P. J. & Nichols, J. W. 2014 Sparsity-promoting dynamic mode decomposition. Phys. Fluids 26, 024103.CrossRefGoogle Scholar
Larchevêque, L., Sagaut, P., Lê, T.-H. & Comte, P. 2004 Large-eddy simulation of a compressible flow in a three-dimensional open cavity at high Reynolds number. J. Fluid Mech. 516, 265–301.CrossRefGoogle Scholar
Lele, S. K. 1988 Direct numerical simulation of compressible free shear layer flows. Center for Turbulence Research Annual Research Briefs N89, 22827.Google Scholar
Liou, M.-S. & Steffen, C. J. 1993 A new flux splitting scheme. J. Comput. Phys. 107, 23–39.CrossRefGoogle Scholar
Lüdeke, H., Mulot, J. D. & Hannemann, K. 2015 Launch vehicle base flow analysis using improved delayed detached-eddy simulation. AIAA J. 53 (9), 2454–2471.CrossRefGoogle Scholar
Mabey, D. G. 1972 Analysis and correlation of data on pressure fluctuations in separated flow. J. Aircraft 9, 642–645.CrossRefGoogle Scholar
Marié, S., Druault, Ph., Lambaré, H. & Schrijer, F. 2013 Experimental analysis of the pressure–velocity correlations of external unsteady flow over rocket launchers. Aerosp. Sci. Technol. 30 (1), 83–93.CrossRefGoogle Scholar
Meinke, M., Schröder, W., Krause, E. & Rister, Th. 2002 A comparison of second- and sixth-order methods for large-eddy simulations. Comput. Fluids 31, 695–718.CrossRefGoogle Scholar
Meliga, P. & Reijasse, P.2007 Unsteady transonic flow behind an axisymmetric afterbody equipped with two boosters. AIAA Paper 4564.CrossRefGoogle Scholar
Mezić, I. 2013 Analysis of fluid flows via spectral properties of the Koopman operator. Annu. Rev. Fluid Mech. 45, 357–378.CrossRefGoogle Scholar
Pain, R., Weiss, P.-E. & Deck, S. 2014 Zonal detached eddy simulation of the flow around a simplified launcher afterbody. AIAA J. 52 (9), 1967–1979.CrossRefGoogle Scholar
Pamiès, M., Weiss, P.-É., Garnier, E., Deck, S. & Sagaut, P. 2009 Generation of synthetic turbulent inflow data for large eddy simulation of spatially evolving wall-bounded flows. Phys. Fluids 21, 045103.CrossRefGoogle Scholar
Roidl, B., Meinke, M. & Schröder, W. 2013 Reformulated synthetic turbulence generation method for a zonal RANS–LES method and its application to zero-pressure gradient boundary layers. Intl J. Heat Fluid Flow 44, 28–40.CrossRefGoogle Scholar
Roidl, B., Meinke, M. & Schröder, W. 2014 Boundary layers affected by different pressure gradients investigated computationally by a zonal RANS–LES method. Intl J. Heat Fluid Flow 45, 1–13.CrossRefGoogle Scholar
Rowley, C. W., Colonius, T. & Basu, A. J. 2002 On self-sustained oscillations in two-dimensional compressible flow over rectangular cavities. J. Fluid Mech. 455, 315–346.CrossRefGoogle Scholar
Rowley, C. W., Mezic, I., Bagheri, S., Schlatter, P. & Henningson, D. S. 2009 Spectral analysis of nonlinear flows. J. Fluid Mech. 641, 115–127.CrossRefGoogle Scholar
Sayadi, T., Chenadec, V. L., Schmid, P. J., Richecoeur, F. & Massot, M. 2014a Thermoacoustic instability – a dynamical system and time domain analysis. J. Fluid Mech. 753, 448–471.CrossRefGoogle Scholar
Sayadi, T., Schmid, P., Nichols, J. W. & Moin, P. 2014b Reduced-order representation of near-wall structures in the late transitional boundary layer. J. Fluid Mech. 748, 278–301.CrossRefGoogle Scholar
Scharnowski, S., Statnikov, V., Meinke, M., Schröder, W. & Kähler, C. J. 2015 Combined experimental and numerical investigation of a transonic space launcher wake. EUCASS Prog. Flight Phys. 7, 311–328.CrossRefGoogle Scholar
Schmid, P. J. 2010 Dynamic mode decomposition of numerical and experimental data. J. Fluid Mech. 656, 5–28.CrossRefGoogle Scholar
Schrijer, F. F. J., Sciacchitano, A. & Scarano, F. 2014 Spatio-temporal and modal analysis of unsteady fluctuations in a high-subsonic base flow. Phys. Fluids 26, 086101.CrossRefGoogle Scholar
Schrijer, F. F. J., Sciacchitano, A., Scarano, F., Hanneman, K., Pallegoix, J. F., Maseland, J. E. J. & Schwane, R. 2011 Experimental investigation of base flow buffeting on the Ariane 5 launcher using high speed PIV. In Proceedings of the 7th European Symposium on Aerothermodynamics for Space Vehicles, Brugge, Belgium 9–12 May, vol. 692, p. 103. European Space Agency.Google Scholar
Schwane, R. 2015 Numerical prediction and experimental validation of unsteady loads on Ariane 5 and Vega. J. Spacecr. Rockets 52 (1), 54–62.CrossRefGoogle Scholar
Statnikov, V., Bolgar, I., Scharnowski, S., Meinke, M., Kähler, C. J. & Schröder, W. 2016a Analysis of characteristic wake flow modes on a generic transonic backward-facing step configuration. Eur. J. Mech. Fluids 59, 124–134.CrossRefGoogle Scholar
Statnikov, V., Meinke, M. & Schröder, W.2016b Analysis of spatio-temporal wake modes of space launchers at transonic flow. AIAA Paper 2016-1116.CrossRefGoogle Scholar
Statnikov, V., Sayadi, T., Meinke, M., Schmid, P. & Schröder, W. 2015 Analysis of pressure perturbation sources on a generic space launcher after-body in supersonic flow using zonal RANS/LES and dynamic mode decomposition. Phys. Fluids 27, 016103.CrossRefGoogle Scholar
Weiss, P.-E. & Deck, S. 2011 Control of the antisymmetric mode (m = 1) for high Reynolds axisymmetric turbulent separating/reattaching flows. Phys. Fluids 23, 095102.CrossRefGoogle Scholar
Weiss, P.-E., Deck, S., Robinet, J.-C. & Sagaut, P. 2009 On the dynamics of axisymmetric turbulent separating/reattaching flows. Phys. Fluids 21, 075103.CrossRefGoogle Scholar

Statnikov et al. supplementary movie

Reduced-order modeled streamwise velocity field for the characteristic frequency SrD(λ1) ≈ 0.1

Download Statnikov et al. supplementary movie(Video)
Video 1.4 MB

Statnikov et al. supplementary movie

Reduced-order modeled streamwise velocity field for the characteristic frequency SrD(λ2) ≈ 0.2

Download Statnikov et al. supplementary movie(Video)
Video 1 MB

Statnikov et al. supplementary movie

Reduced-order modeled streamwise velocity field for the characteristic frequency SrD(λ3) ≈ 0.35

Download Statnikov et al. supplementary movie(Video)
Video 764.1 KB

Statnikov et al. supplementary movie

Streamline plots of the reduced-order modeled composite velocity field for the characteristic frequency SrD(λ1) ≈ 0.1

Download Statnikov et al. supplementary movie(Video)
Video 2.4 MB

Statnikov et al. supplementary movie

Streamline plots of the reduced-order modeled composite velocity field for the characteristic frequency SrD(λ2) ≈ 0.2

Download Statnikov et al. supplementary movie(Video)
Video 1.5 MB

Statnikov et al. supplementary movie

Streamline plots of the reduced-order modeled composite velocity field for the characteristic frequency SrD(λ3) ≈ 0.35

Download Statnikov et al. supplementary movie(Video)
Video 942.1 KB

Statnikov et al. supplementary movie

Reduced-order modeled pressure field for the characteristic frequency SrD(λ1) ≈ 0.1

Download Statnikov et al. supplementary movie(Video)
Video 2.8 MB

Statnikov et al. supplementary movie

Reduced-order modeled pressure field for the characteristic frequency SrD(λ2) ≈ 0.2

Download Statnikov et al. supplementary movie(Video)
Video 1.6 MB

Statnikov et al. supplementary movie

Reduced-order modeled pressure field for the characteristic frequency SrD(λ3) ≈ 0.35

Download Statnikov et al. supplementary movie(Video)
Video 1.1 MB

Statnikov et al. supplementary movie

Visualization of the three-dimensional shape of the antisymmetric modes at SrD ≈ 0.2 using pressure iso-surface in side and top view, i.e., x − z and x − y planes, color-coded by the streamwise coordinate x/D. The nozzle wall is color-coded by the pressure coefficient increment cp′

Download Statnikov et al. supplementary movie(Video)
Video 331.3 KB