Hostname: page-component-586b7cd67f-t7czq Total loading time: 0 Render date: 2024-11-26T21:50:19.075Z Has data issue: false hasContentIssue false
  • English
  • Français

Receptivity of a supersonic boundary layer to solid particulates

Published online by Cambridge University Press:  18 November 2013

Alexander V. Fedorov
Alexander V. Fedorov*
Affiliation:
Department of Aeromechanics and Flight Engineering, Moscow Institute of Physics and Technology, Zhukovski, 140180, Russia
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Laminar–turbulent transition in the boundary layer at supersonic speeds can be initiated by small solid particles present in the free stream. Particulates interacting with the boundary-layer flow generate unstable wavepackets related to Tollmien–Schlichting (TS) waves. The latter grow downstream and ultimately break down to turbulent spots. This scenario of TS-dominated transition is modelled using the Mack amplitude method. A theoretical model describing the receptivity mechanism is developed to predict the initial spectrum of TS waves. With these initial conditions the downstream growth of TS instability is calculated using the linear stability theory. The transition onset is associated with the point where the disturbance amplitude reaches a threshold value. As an example, calculations are carried out for a 14° half-angle sharp wedge flying in the standard atmosphere at altitude 20 km, Mach number 4 and zero angle of attack. It is shown that spherical particles of radius from 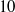$10$ to 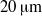$20~\unicode[.5,0][STIXGeneral,Times]{x03BC} \mathrm{m} $ and density 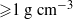${\geqslant }1~\mathrm{g} ~{\mathrm{cm} }^{- 3} $ can cause transition onset corresponding to the amplification factor $N= 9{\unicode{x2013}} 10$, which is in the empirical range of flight data. This indicates that atmospheric particulates may be a major source of TS-dominated transition on aerodynamically smooth surfaces at supersonic speeds. The receptivity model provides a foundation for further treatments of different cases associated with transition in dusty environments. It can also be used for predictions of particle-induced transition at subsonic and hypersonic speeds.

JFM classification

Boundary Layers: Boundary layer receptivity Boundary Layers: Boundary layer stability Instability: Transition to turbulence
Type
Papers
Information
Journal of Fluid Mechanics , Volume 737 , 25 December 2013 , pp. 105 - 131
Copyright
©2013 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Blackwelder, R. F., Browand, F. K., Fisher, C. & Tanaguichi, P. 1992 Initiation of turbulent spots in a laminar boundary layer by rigid particulates. Bull. Am. Phys. Soc. 37 (8), 1812.Google Scholar
Bushnell, D. 1990 Notes on initial disturbance fields for the transition problem. In Instability and Transition, vol. I (ed. Hussaini, M. Y. & Voigt, R. G.), pp. 217–232. Springer.Google Scholar
Chen, C. P., Goland, Y. & Reshotko, E. 1980 Generation rate of turbulent patches in the laminar boundary layer of a submersible. In Proceedings Symposium on Viscous Drag Reduction (ed. Hough, G. R.), Progress in Astronautics and Aeronautics, vol. 72, pp. 73–89. AIAA.Google Scholar
Cowley, S. J. & Wu, X. 1993 Asymptotic approaches for transition modelling. In Progress in Transition Modelling, AGARD Rep. 793, chap. 3, pp. 1–38.Google Scholar
Crowe, C. T. 1967 Drag coefficient on particles in a rocket nozzle. AIAA J. 5 (5), 1021–1022.CrossRefGoogle Scholar
Deepak, A. et al. 1999 Guide to global aerosol models. AIAA G-065-1999.Google Scholar
Ermolaev, Y. G., Kosinov, A. D. & Semionov, N. V. 1996 Experimental investigation of laminar–turbulent transition process in supersonic boundary layer using controlled disturbances. In Nonlinear Instability and Transition in Three-Dimensional Boundary Layers (ed. Duck, P. W. & Hall, P.), pp. 17–26. Kluwer.Google Scholar
Fedorov, A. V. & Khokhlov, A. P. 2002 Receptivity of hypersonic boundary layer to wall disturbances. Theor. Comput. Fluid Dyn. 15, 231–254.Google Scholar
Fedorov, A. & Tumin, A. 2003 Initial-value problem for hypersonic boundary-layer flows. AIAA J. 41 (3), 379–389.Google Scholar
Fedorov, A. & Tumin, A. 2011 High-speed boundary-layer instability: old terminology and a new framework. AIAA J. 49 (8), 1647–1657.CrossRefGoogle Scholar
Hall, G. R. 1967 Interaction of the wake from bluff bodies with an initially laminar boundary layer. AIAA J. 5 (8), 1386–1392.Google Scholar
Hefner, J. N. & Bushnell, D. M. 1979 Application of stability theory to laminar flow control. AIAA Paper 1979-1493.Google Scholar
Henderson, C. B. 1976 Drag coefficients for spheres in continuum and rarefied flows. AIAA J. 14 (6), 707–708.CrossRefGoogle Scholar
Holden, M. S. 1975 Studies of transitional flow, unsteady separation phenomena and particle induced augmentation heating on ablated nose tips. Rep. AFOSR-TR-76-1066, October.Google Scholar
Jaffe, N. A., Okamura, T. T. & Smith, A. M. O. 1970 Determination of spatial amplification factors and their application to predicting transition. AIAA J. 8 (2), 301–308.Google Scholar
Kosinov, A. D., Maslov, A. A. & Semionov, N. V. 1997 An experimental study of generation of unstable disturbances on the leading edge of a plate at $M= 2$ . J. Appl. Mech. Tech. Phys. 38 (1), 45–50.Google Scholar
Kosinov, A. D., Semionov, N. V., Shevelkov, S. G. & Zinin, O. I. 1994 Experiments on the nonlinear instability of supersonic boundary layers. In Nonlinear Instability of Nonparallel Flows (ed. Valentine, D. T., Lin, S. P. & Philips, W. R.C.), pp. 196–205. Springer.Google Scholar
Ladd, D. M. & Hendricks, E. W. 1985 The effect of background particulates on the delayed transition of a heated 9:1 ellipsoid. Exp. Fluids 3, 113–119.Google Scholar
Laible, A. C., Mayer, C. S. J. & Fasel, H. F. 2008 Numerical investigation of supersonic transition for a circular cone at Mach 3.5. AIAA Paper 2008-4397.Google Scholar
Lauchle, G. C., Pertie, H. L. & Stinebring, D. R. 1995 Laminar flow performance of a heated body in particle-laden water. Exp. Fluids 38, 305–312.Google Scholar
Leib, S. J. & Lee, S. S. 1995 Nonlinear evolution of a pair of oblique instability waves in a supersonic boundary layer. J. Fluid Mech. 282, 339–371.Google Scholar
Mack, L. M. 1969 Boundary-layer stability theory. Part B. Doc. 900-277. JPL, May.Google Scholar
Mack, L. M. 1977  Transition and laminar instability. NASA-CP-153203. JPL (May 15).Google Scholar
Malik, M. R. 1990 Stability theory for laminar flow control design. In Viscous Drag Reduction in Boundary Layers (ed. Bushnell, D. M. & Hefner, J. N.), Progress in Astronautics and Aeronautics, vol. 123, pp. 3–46. AIAA.Google Scholar
Malik, M. R. 1997 Boundary-layer transition prediction toolkit. AIAA Paper 1997-1904.CrossRefGoogle Scholar
Mayer, C. S. J., Von Terzi, D. A. & Fasel, H. F. 2011 Direct numerical simulation of complete transition to turbulence via oblique breakdown at Mach 3. J. Fluid Mech. 674, 5–42.Google Scholar
Morkovin, M. V., Reshotko, E. & Herbert, T. 1994 Transition in open flow systems – a reassessment. Bull. Am. Phys. Soc. 39 (9), 1–31.Google Scholar
Nayfeh, A. H. 1980 Stability of three-dimensional boundary layers. AIAA J. 18 (4), 406–416.Google Scholar
Reda, D. C. 2002 Review and synthesis of roughness-dominated transition correlations for reentry applications. J. Spacecr. Rockets 39 (2), 161–167.Google Scholar
Reshotko, E. 1976 Boundary layer stability and transition. Annu. Rev. Fluid Mech. 8, 311–349.Google Scholar
Saiki, E. M. & Bringen, S. 1996 Spatial numerical simulations of boundary layer transition – effects of a spherical particle. AIAA Paper 96-0779.Google Scholar
Salwen, H. & Grosch, C. E. 1981 The continuous spectrum of the Orr–Sommerfeld equation. Part 2. Eigenfunction expansion. J. Fluid Mech. 104, 445–465.Google Scholar
Saric, W. S. 1994 Görtler vortices. Annu. Rev. Fluid Mech. 26, 379–409.Google Scholar
Saric, W. S., Reed, H. L. & White, E. B. 2003 Stability and transition of three-dimensional boundary layers. Annu. Rev. Fluid Mech. 35, 413–440.Google Scholar
Schneider, S. P. 2008 Effects of roughness on hypersonic boundary-layer transition. J. Spacecr. Rockets 45 (2), 193–209.Google Scholar
Smith, A. M. O. & Clutter, D. W. 1959 The smallest height of roughness capable of affecting boundary-layer transition. J. Aerosp. Sci. 26, 229–256.Google Scholar
Taniguchi, P. H., Browand, F. K. & Blackwelder, R. F. 2000 Boundary layer transition due to entry of a small particle. In Laminar-Turbulent Transition, Proceedings of IUTAM Symposium (ed. Fasel, H. & Saric, W.), pp. 199–204. Springer.Google Scholar
Tumin, A. 2007 Three-dimensional spatial normal modes in compressible boundary layers. J. Fluid Mech. 586, 295–322.Google Scholar
Tumin, A. M. & Fedorov, A. V. 1983 Spatial growth of disturbances in a compressible boundary layer. J. Appl. Mech. Tech. Phys. 24, 548–554.Google Scholar
Turco, R. P. 1992 Upper-atmosphere aerosols: properties and natural cycles, In The Atmospheric Effects of Stratospheric Aircraft: A First Program Report, NASA RP-1272, Chap. 3B, pp. 63–91, January.Google Scholar
Wu, X. 2004 Non-equilibrium, nonlinear critical layer in laminar–turbulent transition. Acta Mechanica Sin. 20 (4), 327–339.Google Scholar
Zhigulev, V. N. & Tumin, A. M. 1987 Onset of Turbulence. Nauka.Google Scholar