Published online by Cambridge University Press: 28 March 2013
This article describes the radial drift of a suspended sphere in a cylinder-bound Poiseuille flow where the Reynolds number is small but finite. Unlike past studies, it considers a circular narrow conduit whose cross-sectional diameter is only  $1. 5$–
$1. 5$–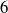 $6$ times the particle diameter. Thus, the analysis quantifies the effect of fluid inertia on the radial motion of the particle in the channel when the flow field is significantly influenced by the presence of the suspended body. To this end, the hydrodynamic fields are expanded as a series in Reynolds number, and a set of hierarchical equations for different orders of the expansion is derived. Accordingly, the zeroth-order fields in Reynolds number satisfy the Stokes equation, which is accurately solved in the presence of the spherical particle and the cylindrical conduit. Then, recognizing that in narrow vessels Stokesian scattered fields from the sphere decrease exponentially in the axial direction, a simpler regular perturbation scheme is used to quantify the first-order inertial correction to hydrodynamic quantities. Consequently, it is possible to obtain two results. First, the sphere is assumed to follow the axial motion of a freely suspended sphere in a Stokesian condition, and the radial lift force on it due to the presence of fluid inertia is evaluated. Then, the approximate motion is determined for a freely suspended body on which net hydrodynamic force including first-order inertial lift is zero. The results agree well with the available experimental results. Thus, this study along with the measured data would precisely describe particle dynamics inside narrow tubes.
$6$ times the particle diameter. Thus, the analysis quantifies the effect of fluid inertia on the radial motion of the particle in the channel when the flow field is significantly influenced by the presence of the suspended body. To this end, the hydrodynamic fields are expanded as a series in Reynolds number, and a set of hierarchical equations for different orders of the expansion is derived. Accordingly, the zeroth-order fields in Reynolds number satisfy the Stokes equation, which is accurately solved in the presence of the spherical particle and the cylindrical conduit. Then, recognizing that in narrow vessels Stokesian scattered fields from the sphere decrease exponentially in the axial direction, a simpler regular perturbation scheme is used to quantify the first-order inertial correction to hydrodynamic quantities. Consequently, it is possible to obtain two results. First, the sphere is assumed to follow the axial motion of a freely suspended sphere in a Stokesian condition, and the radial lift force on it due to the presence of fluid inertia is evaluated. Then, the approximate motion is determined for a freely suspended body on which net hydrodynamic force including first-order inertial lift is zero. The results agree well with the available experimental results. Thus, this study along with the measured data would precisely describe particle dynamics inside narrow tubes.