Published online by Cambridge University Press: 04 February 2015
This paper is the follow-up of a previous study (Manna, Vacca & Verzicco, J. Fluid Mech., vol. 700, 2012, pp. 246–282) that numerically investigated the effects of a harmonic volume forcing on the turbulent pipe flow at a bulk Reynolds number of
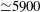 $\simeq 5900$
. There, the investigation was focused on the time- and space-averaged statistics of the first- and second-order moments of the velocity, the vorticity fluctuations and the Reynolds stress budgets in order to study the changes induced on the mean current by the oscillating component. The amplitude of the latter was used as a parameter for the analysis. However, as the flow is inherently unsteady, the phase-averaged statistics are also of interest, and this is the motivation and subject of the present study. Here, we show the variability of the above quantities during different phases of the flow cycle and how they are affected by the amplitude of the oscillation. It is observed that when the ratio of the oscillating to the time-constant velocity component is of the order of one (
$\simeq 5900$
. There, the investigation was focused on the time- and space-averaged statistics of the first- and second-order moments of the velocity, the vorticity fluctuations and the Reynolds stress budgets in order to study the changes induced on the mean current by the oscillating component. The amplitude of the latter was used as a parameter for the analysis. However, as the flow is inherently unsteady, the phase-averaged statistics are also of interest, and this is the motivation and subject of the present study. Here, we show the variability of the above quantities during different phases of the flow cycle and how they are affected by the amplitude of the oscillation. It is observed that when the ratio of the oscillating to the time-constant velocity component is of the order of one (
 ${\it\beta}\simeq O(1)$
), the phase-averaged profiles are appreciably influenced by the pulsation, although only small deviations of the time-averaged counterparts have been documented. In contrast, when that ratio is increased by one order of magnitude (
${\it\beta}\simeq O(1)$
), the phase-averaged profiles are appreciably influenced by the pulsation, although only small deviations of the time-averaged counterparts have been documented. In contrast, when that ratio is increased by one order of magnitude (
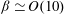 ${\it\beta}\simeq O(10)$
) the phase- and cycle-averaged quantities differ considerably, especially during the decelerating part of the cycle. In more detail, the amplitude and the phase of all turbulence statistics show significant variations with
${\it\beta}\simeq O(10)$
) the phase- and cycle-averaged quantities differ considerably, especially during the decelerating part of the cycle. In more detail, the amplitude and the phase of all turbulence statistics show significant variations with
 ${\it\beta}$
. This variability has important implications in the dynamics and modelling of these flows. Since the data have been obtained by direct numerical simulations and validated by comparisons with experimental studies, the results could be used for validation of codes, testing of turbulence models or measurement procedures.
${\it\beta}$
. This variability has important implications in the dynamics and modelling of these flows. Since the data have been obtained by direct numerical simulations and validated by comparisons with experimental studies, the results could be used for validation of codes, testing of turbulence models or measurement procedures.