Hostname: page-component-cd9895bd7-lnqnp Total loading time: 0 Render date: 2024-12-28T20:46:24.507Z Has data issue: false hasContentIssue false
  • English
  • Français

Performance augmentation mechanism of in-line tandem flapping foils

Published online by Cambridge University Press:  24 August 2017

L. E. Muscutt Open the ORCID record for L. E. Muscutt [Opens in a new window] ,
G. D. Weymouth  and
B. Ganapathisubramani
L. E. Muscutt*
Affiliation:
Aerodynamics and Flight Mechanics Group, Faculty of Engineering and the Environment, University of Southampton, SO17 1BJ, UK
G. D. Weymouth
Affiliation:
Southampton Marine and Maritime Institute, Faculty of Engineering and the Environment, University of Southampton, SO16 7QF, UK
B. Ganapathisubramani
Affiliation:
Aerodynamics and Flight Mechanics Group, Faculty of Engineering and the Environment, University of Southampton, SO17 1BJ, UK
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The propulsive performance of a pair of tandem flapping foils is sensitively dependent on the spacing and phasing between them. Large increases in thrust and efficiency of the hind foil are possible, but the mechanisms governing these enhancements remain largely unresolved. 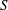Two-dimensional numerical simulations of tandem and single foils oscillating in heave and pitch at a Reynolds number of 7000 are performed over a broad and dense parameter space, allowing the effects of inter-foil spacing ($S$) and phasing (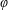$\unicode[STIX]{x1D711}$) to be investigated over a range of non-dimensional frequencies (or Strouhal number, 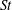$St$). Results indicate that the hind foil can produce from no thrust to twice the thrust of a single foil depending on its spacing and phasing with respect to the fore foil, which is consistent with previous studies that were carried out over a limited parameter space. Examination of instantaneous flow fields indicates that high thrust occurs when the hind foil weaves between the vortices that have been shed by the fore foil, and low thrust occurs when the hind foil intercepts these vortices. Contours of high thrust and minimal thrust appear as inclined bands in the 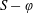$S-\unicode[STIX]{x1D711}$ parameter space and this behaviour is apparent over the entire range of Strouhal numbers considered 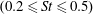$(0.2\leqslant St\leqslant 0.5)$. A novel quasi-steady model that utilises kinematics of a virtual hind foil together with data obtained from simulations of a single flapping foil shows that performance augmentation is primarily determined through modification of the instantaneous angle of attack of the hind foil by the vortex street established by the fore foil. This simple model provides estimates of thrust and efficiency for the hind foil, which is consistent with data obtained through full simulations. The limitations of the virtual hind foil method and its physical significance is also discussed.

JFM classification

Biological Fluid Dynamics: Biological Fluid Dynamics Biological Fluid Dynamics: Swimming/flying Wakes/Jets: Vortex streets
Type
Papers
Information
Journal of Fluid Mechanics , Volume 827 , 25 September 2017 , pp. 484 - 505
Check for updates
Copyright
© 2017 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Akhtar, I., Mittal, R., Lauder, G. V. & Drucker, E. 2007 Hydrodynamics of a biologically inspired tandem flapping foil configuration. Theor. Comput. Fluid Dyn. 21, 155–170.Google Scholar
Alexander, D. E. 1984 Unusual phase relationships between the forewings and hindwings in flying dragonflies. J. Expl Biol. 109, 379–383.Google Scholar
Anderson, J. M., Streitlien, K., Barrett, D. S. & Triantafyllou, M. S. 1998 Oscillating foils of high propulsive efficiency. J. Fluid Mech. 360, 41–72.Google Scholar
Boschitsch, B. M., Dewey, P. A. & Smits, A. J. 2014 Propulsive performance of unsteady tandem hydrofoils in an in-line configuration. Phys. Fluids 26, 131–139.CrossRefGoogle Scholar
Broering, T. M. & Lian, Y. 2010 Numerical investigation of energy extraction in a tendem flapping wing configuration. In 48th AIAA Aerospace Sciences Meeting.Google Scholar
Broering, T. M. & Lian, Y. 2012 The effect of phase angle and wing spacing on tandem flapping wings. Acta Mechanica Sin. 28, 1557–1571.CrossRefGoogle Scholar
Broering, T. M., Lian, Y. & Henshaw, W. 2012 Numerical study of two flapping airfoils in tandem configuration. AIAA J. 50, 2295–2307.Google Scholar
Ellington, C. P. 1984a The aerodynamics of hovering insect flight i. The quasi-steady analysis. Phil. Trans. R. Soc. Lond. B 305 (1122), 1–15.Google Scholar
Ellington, C. P. 1984b The aerodynamics of hovering insect flight iv. Aerodynamic mechanisms. Phil. Trans. R. Soc. Lond. B 305 (1122), 79–113.Google Scholar
Gong, W. Q., Jia, B. B. & Xi, G. 2015 Experimental study on mean thrust of two plunging wings in tandem. AIAA J. 53 (6), 1693–1705.Google Scholar
Gong, W. Q., Jia, B. B. & Xi, G. 2016 Experimental study on instantaneous thrust and lift of two plunging wings in tandem. Exp. Fluids 57, 8.Google Scholar
Gopalkrishnan, R., Triantafyllou, M. S., Triantafyllou, G. S. & Barrett, D. 1994 Active vorticity control in a shear flow using a flapping foil. J. Fluid Mech. 274, 1–21.Google Scholar
Jensen, M. 1956 Biology and Physics of locust flight iii. The aerodynamics of locust flight. Phil. Trans. R. Soc. Lond. B 239 (667), 511–552.Google Scholar
Kinsey, T. & Dumas, G. 2012 Optimal tandem configuration for oscillating-foils hydrokinetic turbine. Trans. ASME J. Fluids Engng 134 (3), 031103.Google Scholar
Kumar, A. G. & Hu, H. 2011 An experimental investigation on the wake flow characteristics of tandem flapping wings. In 6th AIAA Theoretical Fluid Mechanics Conference.Google Scholar
Lian, Y., Broering, T. M., Hord, K. & Prater, R. 2014 The characterisation of tandem and corrugated wings. Prog. Aerosp. Sci. 65, 41–69.CrossRefGoogle Scholar
Maertens, A. P. & Triantafyllou, M. S. 2014 The boundary layer instability of a gliding fish helps rather than prevents object identification. J. Fluid Mech. 757, 179–207.Google Scholar
Maertens, A. P. & Weymouth, G. D. 2015 Accurate cartesian-grid simulations of near-body flows at intermediate Reynolds numbers. Comput. Meth. Appl. Mech. Engng 283, 106–129.Google Scholar
Nakata, T., Liu, H. & Bomphrey, R. J. 2015 A cfd-informed quasi-steady model of flapping-wing aerodynamics. J. Fluid Mech. 783, 323–343.CrossRefGoogle ScholarPubMed
Nudds, R. L., Taylor, G. K. & Thomas, A. L. R. 2004 Tuning of Strouhal number for high propulsive efficiency accurately predicts how wingbeat frequency and stroke amplitude relate and scale with size and flight speed in birds. Proc. R. Soc. Lond. 271, 2071–2076.Google Scholar
Platzer, M. F. & Jones, K. D. 2006 Flapping-wing aerodynamics: progress and challenges. AIAA J. 46 (9), 2136–2149.CrossRefGoogle Scholar
Polet, D. T., Rival, D. E. & Weymouth, G. D. 2015 Unsteady dynamics of rapid perching manoeuvres. J. Fluid Mech. 767, 323–341.Google Scholar
Read, D. A., Hover, F. S. & Triantafyllou, M. S. 2003 Forces on oscillating foils for propulsion and maneuvering. J. Fluids Struct. 17, 163–183.CrossRefGoogle Scholar
Rival, D., Hass, G. & Tropea, C. 2011 Recovery of energy from leading and trailing edge vortices in tandem airfoil configurations. J. Aircraft 48, 203–211.CrossRefGoogle Scholar
Sane, S. P. & Dickinson, M. H. 2002 The aerodynamic effects of wing rotation and a revised quasi-steady model of flapping flight. J. Expl Biol. 205, 1087–1096.Google Scholar
Taylor, G. K., Nudds, R. L. & Thomas, A. L. R. 2003 Flying and swimming animals cruise at a Strouhal number tuned for high power efficiency. Nature 425 (6959), 707–711.Google Scholar
Thomas, A. L. R., Taylor, G. K., Srygley, R. B., Nudds, R. L. & Bomphrey, R. J. 2004 Dragonfly flight: free-flight and tethered flow visualizations reveal a diverse array of unsteady lift-generating mechanism, controlled primarily via angle of attack. J. Expl Biol. 207, 4299–4323.Google Scholar
Triantafyllou, M. S., Tariantafyllou, G. S. & Gopalkrishnan, R. 1991 Wake mechanics for thrust generation in oscillating foils. Phys. Fluids A 3, 2836–2837.CrossRefGoogle Scholar
Weis-Fogh, T. 1972 Energetics of hovering flight in hummingbirds and drosophila. J. Expl Biol. 56, 79–104.Google Scholar
Weis-Fogh, T. 1973 Quick estimates of flight fitness in hovering animals, including novel mechanisms for lift production. J. Expl Biol. 59, 169–230.Google Scholar
Weymouth, G. D. 2014 Chaotic rotation of a towed elliptical cylinder. J. Fluid Mech. 743, 385–398.Google Scholar
Weymouth, G. D.2015 Towards real-time interactive computational fluid dynamics. arXiv:1510.06886 [physics.comp-ph].Google Scholar
Weymouth, G. D. & Yue, D. K. P. 2011 Boundary data immersion method for Cartesian-grid simulations of fluid-body interaction problems. J. Comput. Phys. 230 (16), 6233–6247.Google Scholar
Weymouth, G. D. & Triantafyllou, M. S. 2012 Global vorticity shedding for a shrinking cylinder. J. Fluid Mech. 702, 470–487.Google Scholar

Muscutt et al. supplementary movie 1

Contour plot of instantaneous vorticity magnitude for single foil over one flapping cycle

Download Muscutt et al. supplementary movie 1(Video)
Video 642.7 KB

Muscutt et al. supplementary movie 2

Contour plot of instantaneous vorticity magnitude for tandem foil high-thrust case over one flapping cycle

Download Muscutt et al. supplementary movie 2(Video)
Video 1.1 MB

Muscutt et al. supplementary movie 3

Contour plot of instantaneous vorticity magnitude for tandem foil low-thrust case over one flapping cycle

Download Muscutt et al. supplementary movie 3(Video)
Video 970 KB