Hostname: page-component-848d4c4894-nmvwc Total loading time: 0 Render date: 2024-07-07T22:37:52.697Z Has data issue: false hasContentIssue false
  • English
  • Français

Optimal triggering of jet bifurcation: an example of optimal forcing applied to a time-periodic base flow

Published online by Cambridge University Press:  06 January 2020

Léopold Shaabani-Ardali Open the ORCID record for Léopold Shaabani-Ardali [Opens in a new window] ,
Denis Sipp Open the ORCID record for Denis Sipp [Opens in a new window]  and
Lutz Lesshafft Open the ORCID record for Lutz Lesshafft [Opens in a new window]
Léopold Shaabani-Ardali*
Affiliation:
LadHyX, CNRS – Ecole Polytechnique – Institut Polytechnique de Paris, 91120 Palaiseau, France DAAA, ONERA, Université Paris-Saclay, 92190 Meudon, France
Denis Sipp
Affiliation:
DAAA, ONERA, Université Paris-Saclay, 92190 Meudon, France
Lutz Lesshafft
Affiliation:
LadHyX, CNRS – Ecole Polytechnique – Institut Polytechnique de Paris, 91120 Palaiseau, France
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

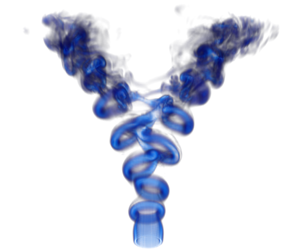
The present article aims at optimising the spread of a bifurcating jet: a jet that combines axisymmetric and helical forcing to achieve increased mixing in a preferential plane. Parekh et al. (Tech. Rep. TF-35, Stanford University, 1988) explained such a bifurcation as the result of nonlinear interaction between ring vortices (triggered by $m=0$ axisymmetric forcing), shifted off-axis in alternate directions (owing to $m=1$ helical forcing). Following this idea, we linearly optimise the periodic helical forcing to be applied at the inlet, in order to maximally displace the ring vortices of an axisymmetrically forced jet. Two norms are introduced for evaluating the effect of helical forcing onto the helical response: the standard ${\mathcal{L}}_{2}$-norm and a semi-norm reflecting the off-axis vortex displacement. The linear results show one dominant forcing mode over the entire Strouhal band studied ($0.35\leqslant St\leqslant 0.8$), with a large gain separation from suboptimals. The dominant forcing is mainly radial, independent of the chosen response norm, and provides a gain at least five times larger than what was achieved by previous ad hoc forcing strategies. Superposition of base flow and linear results show the alternate shifting and twisting provoked by the the small-amplitude helical forcing, which is an essential ingredient for triggering jet bifurcation. When tested in three-dimensional direct numerical simulations, low-amplitude helical forcing achieves efficient bifurcation at all Strouhal values studied. At high Strouhal numbers, an additional central branch emerges in the mean flow, leading to trifurcation. Across all frequencies, compared with ad hoc forcing strategies, the optimal forcing triggers a much stronger and robust spreading, by moving the bifurcation point upstream. As a result, bifurcating jets are observed over a much larger Strouhal band ($0.35\leqslant St\leqslant 0.8$) compared with the band where ad hoc forcing achieves bifurcation in our setting ($0.4\leqslant St\leqslant 0.5$).

JFM classification

Flow Control: Mixing enhancement Vortex Flows: Vortex interactions Wakes/Jets: Jets
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 885 , 25 February 2020 , A34
Copyright
© 2020 Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Abramowitz, M. & Stegun, I. A. 1964 Handbook of Mathematical Functions: with Formulas, Graphs, and Mathematical Tables, vol. 55. Courier Corporation.Google Scholar
Åkervik, E., Brandt, L., Henningson, D. S., Hœpffner, J., Marxen, O. & Schlatter, P. 2006 Steady solutions of the Navier–Stokes equations by selective frequency damping. Phys. Fluids 18 (6), 068102.CrossRefGoogle Scholar
Arbey, H. & Ffowcs Williams, J. E. 1984 Active cancellation of pure tones in an excited jet. J. Fluid Mech. 149, 445–454.CrossRefGoogle Scholar
Blackburn, H. M., Sherwin, S. J. & Barkley, D. 2008 Convective instability and transient growth in steady and pulsatile stenotic flows. J. Fluid Mech. 607, 267–277.CrossRefGoogle Scholar
Boujo, E. & Gallaire, F. 2015 Sensitivity and open-loop control of stochastic response in a noise amplifier flow: the backward-facing step. J. Fluid Mech. 762, 361–392.CrossRefGoogle Scholar
Danaila, I. & Boersma, B. J. 1998 Mode interaction in a forced homogeneous jet at low Reynolds numbers. In Proceedings of the Summer Program, pp. 141–158. Center for Turbulence Research, Stanford University.Google Scholar
Danaila, I. & Boersma, B. J. 2000 Direct numerical simulation of bifurcating jets. Phys. Fluids 12 (5), 1255–1257.CrossRefGoogle Scholar
Dick, E. 2009 Introduction to finite element methods in computational fluid dynamics. In Computational Fluid Dynamics (ed. Wendt, J. F.), pp. 235–274. Springer.CrossRefGoogle Scholar
Fischer, P., Kruse, J., Mullen, J., Tufo, H., Lottes, J. & Kerkemeier, S.2008 NEK5000: Open source spectral element CFD solver. Available at: https://nek5000.mcs.anl.gov/index.php/MainPage.Google Scholar
Floquet, G. 1883 Sur les équations différentielles linéaires à coefficients périodiques. Ann. Sci. École Norm. Sup. 12, 47–88.CrossRefGoogle Scholar
Freund, J. B. & Moin, P. 1998 Mixing enhancement in jet exhaust using fluidic actuators: direct numerical simulations. In Proceedings of FEDSM98, p. 5235. ASME.Google Scholar
Freund, J. B. & Moin, P. 2000 Jet mixing enhancement by high-amplitude fluidic actuation. AIAA J. 38 (10), 1863–1870.CrossRefGoogle Scholar
Gohil, T. B., Saha, A. K. & Muralidhar, K. 2010 Control of flow in forced jets: a comparison of round and square cross sections. J. Vis. 13 (2), 141–149.Google Scholar
Gohil, T. B. & Saha, A. K. 2019 Numerical simulation of forced circular jets: effect of flapping perturbation. Phys. Fluids 31 (8), 083602.CrossRefGoogle Scholar
Gohil, T. B., Saha, A. K. & Muralidhar, K. 2015 Simulation of the blooming phenomenon in forced circular jets. J. Fluid Mech. 783, 567–604.CrossRefGoogle Scholar
Hecht, F. 2012 New development in FreeFem++. J. Numer. Math. 20 (3–4), 251–265.Google Scholar
Hilgers, A. & Boersma, B. J. 2001 Optimization of turbulent jet mixing. Fluid Dyn. Res. 29 (6), 345–368.CrossRefGoogle Scholar
Ho, C.-M. & Huerre, P. 1984 Perturbed free shear layers. Annu. Rev. Fluid Mech. 16 (1), 365–422.CrossRefGoogle Scholar
Hussain, A. K. M. F. & Zaman, K. B. M. Q. 1980 Vortex pairing in a circular jet under controlled excitation. Part 2. Coherent structure dynamics. J. Fluid Mech. 101 (03), 493–544.CrossRefGoogle Scholar
Koumoutsakos, P., Freund, J. & Parekh, D. 1998 Evolution strategies for parameter optimization in jet flow control. In Proceedings of the Summer Program, pp. 121–132. Center for Turbulence Research, Stanford University.Google Scholar
Lee, M. & Reynolds, W. C.1985 Bifurcating and blooming jets. Tech. Rep. TF-22. Thermosciences Division, Department of Mechanical Engineering, Stanford University.Google Scholar
Lesshafft, L. 2018 Artificial eigenmodes in truncated flow domains. Theor. Comput. Fluid Dyn. 32 (3), 245–262.CrossRefGoogle Scholar
Longmire, E. K. & Duong, L. H. 1996 Bifurcating jets generated with stepped and sawtooth nozzles. Phys. Fluids 8 (4), 978–992.CrossRefGoogle Scholar
Parekh, D. E., Kibens, V., Glezer, A., Wiltse, J. M. & Smith, D. M.1996 Innovative jet flow control: mixing enhancement experiments. AIAA Paper 308, 1996.Google Scholar
Parekh, D. E., Leonard, A. & Reynolds, W. C.1988 Bifurcating jets at high Reynolds numbers. Tech. Rep. TF-35. Thermosciences Division, Department of Mechanical Engineering, Stanford University.Google Scholar
Parekh, D. E., Reynolds, W. C. & Mungal, M. G. 1987 Bifurcation of round air jets by dual-mode acoustic excitation. In 25th AIAA Aerospace Sciences Meeting, American Institute of Aeronautics and Astronautics.Google Scholar
Pfizenmaier, E., Simon, J. & Monkewitz, P. A. 1993 Bouquet with bifurcating jet diffusion flame. Phys. Fluids A 5 (9), S9.CrossRefGoogle Scholar
Raman, G. & Rice, E. J. 1991 Axisymmetric jet forced by fundamental and subharmonic tones. AIAA J. 29 (7), 1114–1122.CrossRefGoogle Scholar
Reynolds, W. C., Parekh, D. E., Juvet, P. J. D. & Lee, M. J. D. 2003 Bifurcating and blooming jets. Annu. Rev. Fluid Mech. 35 (1), 295–315.CrossRefGoogle Scholar
Schmid, P. J. 2007 Nonmodal stability theory. Annu. Rev. Fluid Mech. 39, 129–162.CrossRefGoogle Scholar
Shaabani-Ardali, L., Sipp, D. & Lesshafft, L. 2017 Time-delayed feedback technique for suppressing instabilities in time-periodic flow. Phys. Rev. Fluids 2 (11), 113904.CrossRefGoogle Scholar
Shaabani-Ardali, L., Sipp, D. & Lesshafft, L. 2019 Vortex pairing in jets as a global floquet instability: modal and transient dynamics. J. Fluid Mech. 862, 951–989.CrossRefGoogle Scholar
da Silva, C. B. & Métais, O. 2002 Vortex control of bifurcating jets: a numerical study. Phys. Fluids 14 (11), 3798–3819.CrossRefGoogle Scholar
Smith, T. D., Cain, A. B. & Chenault, C. F. 2001 Numerical simulation of enhanced mixing in jet plumes using pulsed blowing. J. Aircraft 38 (3), 458–463.CrossRefGoogle Scholar
Theofilis, V. 2017 The linearized pressure Poisson equation for global instability analysis of incompressible flows. Theor. Comput. Fluid Dyn. 31 (5–6), 623–642.CrossRefGoogle Scholar
Tyliszczak, A. 2015 Multi-armed jets: a subset of the blooming jets. Phys. Fluids 27 (4), 041703.CrossRefGoogle Scholar
Tyliszczak, A. 2018 Parametric study of multi-armed jets. Intl J. Heat Fluid Flow 73, 82–100.CrossRefGoogle Scholar
Tyliszczak, A. & Boguslawski, A. 2006 LES of the jet in low mach variable density conditions. In Direct and Large-Eddy Simulation VI, pp. 575–582. Springer.CrossRefGoogle Scholar
Tyliszczak, A. & Boguslawski, A. 2007 LES of variable density bifurcating jets. In Complex Effects in Large Eddy Simulations, pp. 273–288. Springer.CrossRefGoogle Scholar
Tyliszczak, A. & Geurts, B. J. 2014 Parametric analysis of excited round jets – numerical study. Flow Turbul. Combust. 93 (2), 221–247.CrossRefGoogle Scholar
Webster, D. R. & Longmire, E. K. 1997 Vortex dynamics in jets from inclined nozzles. Phys. Fluids 9 (3), 655–666.CrossRefGoogle Scholar
Wu, Z., Fan, D., Zhou, Y., Li, R. & Noack, B. R. 2018 Jet mixing optimization using machine learning control. Exp. Fluids 59 (8), 131.CrossRefGoogle Scholar
Zaman, K. B. M. Q. & Hussain, A. K. M. F. 1980 Vortex pairing in a circular jet under controlled excitation. Part 1. General jet response. J. Fluid Mech. 101 (03), 449–491.CrossRefGoogle Scholar
Zaman, K. B. M. Q. & Raman, G. 1997 Reversal in spreading of a tabbed circular jet under controlled excitation. Phys. Fluids 9 (12), 3733–3741.CrossRefGoogle Scholar
Zaman, K. B. M. Q., Reeder, M. F. & Samimy, M. 1994 Control of an axisymmetric jet using vortex generators. Phys. Fluids 6 (2), 778–793.CrossRefGoogle Scholar