Hostname: page-component-745bb68f8f-b95js Total loading time: 0 Render date: 2025-01-09T21:58:53.675Z Has data issue: false hasContentIssue false
  • English
  • Français

On the transition from finite-volume negatively buoyant releases to continuous fountains

Published online by Cambridge University Press:  30 March 2012

O. J. Myrtroeen  and
G. R. Hunt
O. J. Myrtroeen
Affiliation:
Department of Civil and Environmental Engineering, Imperial College London, London SW7 2AZ, UK
G. R. Hunt*
Affiliation:
Department of Civil and Environmental Engineering, Imperial College London, London SW7 2AZ, UK
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

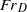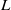An experimental investigation to identify the source conditions that distinguish finite-volume negatively buoyant fluid projectile behaviour from fountain behaviour in quiescent environments of uniform density is described. Finite-volume releases are governed by their source Froude number and the aspect ratio of the release, where denotes the length of the column of fluid dispensed vertically from the nozzle of diameter 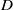. We establish the influence of 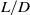on the peak rise heights of a release formed by dispensing saline solution into fresh water for and . Within these ranges, we determine the source conditions for which a flow may be regarded, in terms of the initial rise height attained, as either finite-volume or continuous flux. The critical aspect ratio 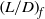, for a given , which when exceeded no longer influenced release behaviour, led to the determination of paired source conditions that give rise to solely Froude-number-dependent, i.e. fountain-like, behaviour. As such, we make the link between finite-volume releases and continuous fountains. The 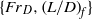pairs led us directly to the classification of a 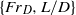space from which source conditions giving rise to either negatively buoyant projectiles or fountains may be readily identified. The variation of 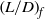with corresponds closely to established fountain regimes of very weak, weak and forced fountains. Moreover, our results indicate that the formation or otherwise of a primary vortex, as fluid is ejected, has a profound influence on the length of the dispensed fluid column that is necessary to achieve rise heights equal to fountain rise heights.

JFM classification

Convection: Plumes/thermals
Type
Papers
Information
Journal of Fluid Mechanics , Volume 698 , 10 May 2012 , pp. 168 - 184
Copyright
Copyright © Cambridge University Press 2012

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Baines, W. D., Turner, J. S. & Campbell, I. H. 1990 Turbulent fountains in an open chamber. J. Fluid Mech. 212, 557–592.CrossRefGoogle Scholar
2. Bloomfield, L. J. & Kerr, R. C. 2000 A theoretical model of a turbulent fountain. J. Fluid Mech. 424, 197–216.Google Scholar
3. Burridge, H. C. & Hunt, G. R. 2012 The rise heights of low- and high-Froude-number turbulent axisymmetric fountains. J. Fluid Mech. 691, 392–416.Google Scholar
4. Friedman, P. D., Vadakoot, V. D., Meyer, W. J. Jr. & Carey, S. 2007 Instability threshold of a negatively buoyant fountain. Exp. Fluids 42, 751–759.CrossRefGoogle Scholar
5. Gharib, M., Rambod, E. & Shariff, K. 1998 A universal time scale for vortex ring formation. J. Fluid Mech. 360, 121–140.Google Scholar
6. Kaye, N. B. & Hunt, G. R. 2006 Weak fountains. J. Fluid Mech. 558, 319–328.Google Scholar
7. Lin, W. & Armfield, S. W. 2000 Very weak fountains in a homogeneous fluid. Numer. Heat Transfer A 38, 377–396.Google Scholar
8. Myrtroeen, O. J. & Hunt, G. R. 2010 Negatively buoyant projectiles – from weak fountains to heavy vortices. J. Fluid Mech. 657, 227–237.Google Scholar
9. Turner, J. S. 1966 Jets and plumes with negative or reversing buoyancy. J. Fluid Mech. 26, 779–792.Google Scholar
10. Williamson, N., Srinarayana, N., Armfield, S. W., McBain, G. D. & Lin, W. 2008 Low-Reynolds-number fountain behaviour. J. Fluid Mech. 608, 297–317.Google Scholar
11. Zhang, H. & Baddour, R. E. 1998 Maximum penetration of vertical round dense jets at small and large Froude numbers. J. Hydraul. Engng 124, 550–553.Google Scholar