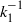Hostname: page-component-586b7cd67f-l7hp2 Total loading time: 0 Render date: 2024-11-23T04:54:05.861Z Has data issue: false hasContentIssue false
  • English
  • Français

On the ${ k}_{1}^{- 1} $ scaling in sink-flow turbulent boundary layers

Published online by Cambridge University Press:  25 November 2013

Shivsai Ajit Dixit  and
O. N. Ramesh
Shivsai Ajit Dixit
Affiliation:
Indian Institute of Tropical Meteorology, Pashan, Pune 411008, India Department of Mechanical Engineering, Maharshi Karve Stree Shikshan Samstha’s Cummins College of Engineering for Women, Karvenagar, Pune 411052, India
O. N. Ramesh*
Affiliation:
Department of Aerospace Engineering, Indian Institute of Science, Bangalore 560012, India
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Scaling of the streamwise velocity spectrum 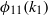${\phi }_{11} ({k}_{1} )$ in the so-called sink-flow turbulent boundary layer is investigated in this work. The present experiments show strong evidence for the ${ k}_{1}^{- 1} $ scaling i.e. 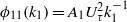${\phi }_{11} ({k}_{1} )= {A}_{1} { U}_{\tau }^{2} { k}_{1}^{- 1} $, where 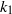${k}_{1} $ is the streamwise wavenumber and 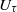${U}_{\tau } $ is the friction velocity. Interestingly, this 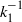${ k}_{1}^{- 1} $ scaling is observed much farther from the wall and at much lower flow Reynolds number (both differing by almost an order of magnitude) than what the expectations from experiments on a zero-pressure-gradient turbulent boundary layer flow would suggest. Furthermore, the coefficient 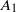${A}_{1} $ in the present sink-flow data is seen to be non-universal, i.e. ${A}_{1} $ varies with height from the wall; the scaling exponent −1 remains universal. Logarithmic variation of the so-called longitudinal structure function, which is the physical-space counterpart of spectral 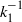${ k}_{1}^{- 1} $ scaling, is also seen to be non-universal, consistent with the non-universality of ${A}_{1} $. These observations are to be contrasted with the universal value of 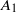${A}_{1} $ (along with the universal scaling exponent of −1) reported in the literature on zero-pressure-gradient turbulent boundary layers. Theoretical arguments based on dimensional analysis indicate that the presence of a streamwise pressure gradient in sink-flow turbulent boundary layers makes the coefficient 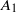${A}_{1} $ non-universal while leaving the scaling exponent −1 unaffected. This effect of the pressure gradient on the streamwise spectra, as discussed in the present study (experiments as well as theory), is consistent with other recent studies in the literature that are focused on the structural aspects of turbulent boundary layer flows in pressure gradients (Harun et al., J. Fluid Mech., vol. 715, 2013, pp. 477–498); the present paper establishes the link between these two. The variability of 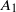${A}_{1} $ accommodated in the present framework serves to clarify the ideas of universality of the 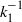${ k}_{1}^{- 1} $ scaling.

JFM classification

Boundary Layers: Boundary layer structure Turbulent Flows: Turbulent boundary layers
Type
Papers
Information
Journal of Fluid Mechanics , Volume 737 , 25 December 2013 , pp. 329 - 348
Copyright
©2013 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Davidson, P. A. & Krogstad, P. Å. 2009 A simple model for the streamwise fluctuations in the log-law region of a boundary layer. Phys. Fluids 21, 055105.CrossRefGoogle Scholar
Davidson, P. A., Nickels, T. B. & Krogstad, P. Å. 2006 The logarithmic structure function law in wall-layer turbulence. J. Fluid Mech. 550, 51–60.Google Scholar
Dixit, S. A. & Ramesh, O. N. 2008 Pressure-gradient-dependent logarithmic laws in sink flow turbulent boundary layers. J. Fluid Mech. 615, 445–475.Google Scholar
Dixit, S. A. & Ramesh, O. N. 2010 Large-scale structures in turbulent and reverse-transitional sink flow boundary layers. J. Fluid Mech. 649, 233–273.Google Scholar
Harun, Z., Monty, J. P., Mathis, R. & Marusic, I. 2013 Pressure gradient effects on the large-scale structure of turbulent boundary layers. J. Fluid Mech. 715, 477–498.Google Scholar
Högström, U., Hunt, J. C. R. & Smedman, A. S. 2002 Theory and measurements for turbulence spectra and variances in the atmospheric neutral surface layer. Boundary-Layer Meteorol. 103, 101–124.Google Scholar
Hutchins, N. & Marusic, I. 2007a Large-scale influences in near-wall turbulence. Phil. Trans. R. Soc. A 365, 647–664.Google Scholar
Hutchins, N. & Marusic, I. 2007b Evidence of very long meandering features in the logarithmic region of turbulent boundary layers. J. Fluid Mech. 579, 1–28.CrossRefGoogle Scholar
Hutchins, N., Nickels, T. B., Marusic, I. & Chong, M. S. 2009 Hot-wire spatial resolution issues in wall-bounded turbulence. J. Fluid Mech. 635, 103–136.Google Scholar
Jones, M. B. 1998 Evolution and structure of sink flow turbulent boundary layers. PhD thesis, University of Melbourne, Australia.Google Scholar
Jones, M. B., Marusic, I. & Perry, A. E. 2001 Evolution and structure of sink-flow turbulent boundary layers. J. Fluid Mech. 428, 1–27.CrossRefGoogle Scholar
Katul, G. & Chu, C. R. 1998 A theoretical and experimental investigation of energy-containing scales in the dynamic sublayer of boundary-layer flows. Boundary-Layer Meteorol. 86, 279–312.Google Scholar
Lauren, M. K., Menabde, M., Seed, A. W. & Austin, G. L. 1999 Characterisation and simulation of the multiscaling properties of the energy-containing scales of horizontal surface-layer winds. Boundary-Layer Meteorol. 90, 21–46.Google Scholar
Marusic, I., Monty, J. P., Hultmark, M. & Smits, A. J. 2013 On the logarithmic region in wall turbulence. J. Fluid Mech. 716, R3.Google Scholar
Metzger, M., Lyons, A. & Fife, P. 2008 Mean momentum balance in moderately favourable pressure gradient turbulent boundary layers. J. Fluid Mech. 617, 107–140.Google Scholar
Nickels, T. B., Marusic, I., Hafez, S. & Chong, M. S. 2005 Evidence of the ${ k}_{1}^{- 1} $ law in a high-Reynolds-number turbulent boundary layer. Phys. Rev. Lett. 95, 074501.Google Scholar
Nickels, T. B., Marusic, I., Hafez, S., Hutchins, N. & Chong, M. S. 2007 Some predictions of the attached eddy model for a high Reynolds number boundary layer. Phil. Trans. R. Soc. A 365, 807–822.Google Scholar
Nikora, V. 1999 Origin of the ‘−1’ spectral law in wall-bounded turbulence. Phys. Rev. Lett. 83 (4), 734–736.Google Scholar
Perry, A. E. & Chong, M. S. 1982 On the mechanism of wall turbulence. J. Fluid Mech. 119, 173–217.Google Scholar
Perry, A. E., Henbest, S. & Chong, M. S. 1986 A theoretical and experimental study of wall turbulence. J. Fluid Mech. 165, 163–199.Google Scholar
Perry, A. E. & Marusic, I. 1995 A wall-wake model for the turbulence structure of boundary layers. Part 1. Extension of the attached eddy hypo thesis. J. Fluid Mech. 298, 361–388.Google Scholar
Smits, A. J., Monty, J., Hultmark, M., Bailey, S. C. C., Hutchins, N. & Marusic, I. 2011 Spatial resolution correction for wall-bounded turbulence measurements. J. Fluid Mech. 676, 41–53.Google Scholar
Sreenivasan, K. R. 1989 The turbulent boundary layer. In Frontiers in Experimental Fluid Mechanics (ed. Gad-El-Hak, M.), Springer.Google Scholar
Townsend, A. A. 1976 The Structure of Turbulent Shear Flow, 2nd edn. Cambridge University Press.Google Scholar
Uddin, A. K. M., Perry, A. E. & Marusic, I. 1997 Application of the wavelet transform in turbulence. In Second International Seminar on Fluid Mechanics and Heat Transfer, 17–18 December, Dhaka, Bangladesh, pp. 25–34.Google Scholar
Wei, T., Fife, P., Klewicki, J. & Mcurtry, P. 2005 Properties of the mean momentum balance in turbulent boundary layer, pipe and channel flows. J. Fluid Mech. 522, 303–327.Google Scholar