Published online by Cambridge University Press: 12 July 2012
We study the dispersal of a plume of incompressible buoyant fluid injected into a confined inclined aquifer in which there is a background flow. We assume that, to prevent pressure buildup in the system, there is an outflow from the aquifer, with flux equal to the injection flux, through a producing well. Using the method of characteristics, we identify that the trajectory of the plume of injected fluid depends on the magnitudes of both the injection flux 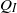 and the background aquifer flux
and the background aquifer flux 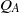 relative to the buoyancy-driven exchange flow of injected and original fluid within the aquifer
relative to the buoyancy-driven exchange flow of injected and original fluid within the aquifer 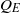 , on the direction of the background aquifer flow, and on whether the producing well lies upslope or downslope from the injecting well. We find the values of the controlling parameters
, on the direction of the background aquifer flow, and on whether the producing well lies upslope or downslope from the injecting well. We find the values of the controlling parameters 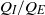 and
and 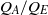 for which all injected fluid flows up-dip, for which the injected fluid partitions into a component moving up-dip and a component moving down-dip, and for which all injected fluid flows down-dip. A key learning from the analysis is that there may be very different plume trajectories when a buoyant fluid is injected into a confined, inclined aquifer, and prediction of the trajectory depends on knowledge of the background flow as well as the injection rate and location of the producing wells. In the process of
for which all injected fluid flows up-dip, for which the injected fluid partitions into a component moving up-dip and a component moving down-dip, and for which all injected fluid flows down-dip. A key learning from the analysis is that there may be very different plume trajectories when a buoyant fluid is injected into a confined, inclined aquifer, and prediction of the trajectory depends on knowledge of the background flow as well as the injection rate and location of the producing wells. In the process of 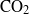 sequestration, this range of initial plume geometries can inform analysis of longer-term geological storage and assessment of the risk of activating different possible leakage pathways to the surface.
sequestration, this range of initial plume geometries can inform analysis of longer-term geological storage and assessment of the risk of activating different possible leakage pathways to the surface.