Hostname: page-component-78c5997874-j824f Total loading time: 0 Render date: 2024-11-17T05:19:34.677Z Has data issue: false hasContentIssue false
  • English
  • Français

On singularity formation via viscous vortex reconnection

Published online by Cambridge University Press:  06 February 2020

Jie Yao Open the ORCID record for Jie Yao [Opens in a new window]  and
Fazle Hussain Open the ORCID record for Fazle Hussain [Opens in a new window]
Jie Yao
Affiliation:
Department of Mechanical Engineering, Texas Tech University, Lubbock, TX79409, USA
Fazle Hussain*
Affiliation:
Department of Mechanical Engineering, Texas Tech University, Lubbock, TX79409, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Recognizing the fact that the finite-time singularity of the Navier–Stokes equations is widely accepted as a key issue in fundamental fluid mechanics, and motivated by the recent model of Moffatt & Kimura (J. Fluid Mech., vol. 861, 2019a, pp. 930–967; J. Fluid Mech., vol. 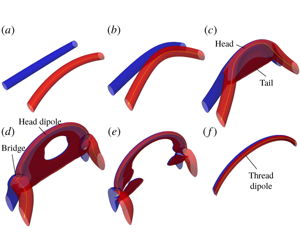870, 2019b, R1) on this issue, we have performed direct numerical simulation (DNS) for two colliding slender vortex rings of radius $R$. The separation between the two tipping points $2s_{0}$ and the scale of the core cross-section $\unicode[STIX]{x1D6FF}_{0}$ are chosen as $\unicode[STIX]{x1D6FF}_{0}=0.1s_{0}=0.01R$; the vortex Reynolds number ($Re=\text{circulation/viscosity}$) ranges from 1000 to 4000. In contrast to the claim that the core remains compact and circular, there is notable core flattening and stripping, which further increases with $Re$ – akin to our previous finding in the standard anti-parallel vortex reconnection. Furthermore, the induced motion of bridges arrests the curvature growth and vortex stretching at the tipping points; consequently, the maximum vorticity grows with $Re$ substantially slower than the exponential scaling predicted by the model – implying that, for this configuration, even physical singularity is unlikely. Our simulations not only shed light on the longstanding question of finite-time singularities, but also further delineate the detailed mechanisms of reconnection. In particular, we show for the first time that the separation distance $s(\unicode[STIX]{x1D70F})$ before reconnection follows 1/2 scaling exactly – a significant DNS result.

JFM classification

Vortex Flows: Vortex dynamics Vortex Flows: Vortex interactions Turbulent Flows: Turbulence theory
Type
JFM Rapids
Information
Journal of Fluid Mechanics , Volume 888 , 10 April 2020 , R2
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Baggaley, A. W., Sherwin, L. K., Barenghi, C. F. & Sergeev, Y. A. 2012 Thermally and mechanically driven quantum turbulence in helium II. Phys. Rev. B 86 (10), 104501.CrossRefGoogle Scholar
Beale, J. T., Kato, T. & Majda, A. 1984 Remarks on the breakdown of smooth solutions for the 3-D Euler equations. Commun. Math. Phys. 94 (1), 61–66.CrossRefGoogle Scholar
Bewley, G. P., Paoletti, M. S., Sreenivasan, K. R. & Lathrop, D. P. 2008 Characterization of reconnecting vortices in superfluid helium. Proc. Natl Acad. Sci. USA 105 (37), 13707–13710.CrossRefGoogle ScholarPubMed
Boratav, O. N. & Pelz, R. B. 1994 Direct numerical simulation of transition to turbulence from a high-symmetry initial condition. Phys. Fluids 6 (8), 2757–2784.CrossRefGoogle Scholar
Brenner, M. P., Hormoz, S. & Pumir, A. 2016 Potential singularity mechanism for the Euler equations. Phys. Rev. Fluids 1 (8), 084503.CrossRefGoogle Scholar
Constantin, P., Fefferman, C. & Majda, A. J. 1996 Geometric constraints on potentially singular solutions for the 3-D Euler equations. Commun. Part. Diff. Equ. 21 (3–4), 559–571.Google Scholar
De Waele, A. T. A. M. & Aarts, R. G. K. M. 1994 Route to vortex reconnection. Phys. Rev. Lett. 72 (4), 482.CrossRefGoogle ScholarPubMed
Doering, C. R. 2009 The 3D Navier–Stokes problem. Annu. Rev. Fluid Mech. 41, 109–128.CrossRefGoogle Scholar
Fonda, E., Sreenivasan, K. R. & Lathrop, D. P. 2019 Reconnection scaling in quantum fluids. Proc. Natl Acad. Sci. USA 116 (6), 1924–1928.CrossRefGoogle ScholarPubMed
Grauer, R., Marliani, C. & Germaschewski, K. 1998 Adaptive mesh refinement for singular solutions of the incompressible Euler equations. Phys. Rev. Lett. 80 (19), 4177–4180.CrossRefGoogle Scholar
Hou, T. Y. & Li, R. 2006 Dynamic depletion of vortex stretching and non-blowup of the 3-D incompressible Euler equations. J. Nonlinear Sci. 16 (6), 639–664.CrossRefGoogle Scholar
Hussain, F. & Duraisamy, K. 2011 Mechanics of viscous vortex reconnection. Phys. Fluids 23 (2), 021701.CrossRefGoogle Scholar
Jeong, J. & Hussain, F. 1995 On the identification of a vortex. J. Fluid Mech. 285, 69–94.CrossRefGoogle Scholar
Kerr, R. M. 1993 Evidence for a singularity of the three-dimensional, incompressible Euler equations. Phys. Fluids A 5 (7), 1725–1746.CrossRefGoogle Scholar
Kerr, R. M. 2013 Bounds for Euler from vorticity moments and line divergence. J. Fluid Mech. 729, R2.CrossRefGoogle Scholar
Kerr, R. M. 2018 Enstrophy and circulation scaling for Navier–Stokes reconnection. J. Fluid Mech. 839, R2.CrossRefGoogle Scholar
Kida, S. & Takaoka, M. 1994 Vortex reconnection. Annu. Rev. Fluid Mech. 26 (1), 169–177.CrossRefGoogle Scholar
Kida, S., Takaoka, M. & Hussain, F. 1991 Collision of two vortex rings. J. Fluid Mech. 230, 583–646.CrossRefGoogle Scholar
Kimura, Y. & Moffatt, H. K. 2014 Reconnection of skewed vortices. J. Fluid Mech. 751, 329–345.CrossRefGoogle Scholar
Kimura, Y. & Moffatt, H. K. 2017 Scaling properties towards vortex reconnection under Biot–Savart evolution. Fluid Dyn. Res. 50 (1), 011409.Google Scholar
Kimura, Y. & Moffatt, H. K. 2018 A tent model of vortex reconnection under Biot–Savart evolution. J. Fluid Mech. 834, R1.CrossRefGoogle Scholar
Koplik, J. & Levine, H. 1993 Vortex reconnection in superfluid helium. Phys. Rev. Lett. 71 (9), 1375.CrossRefGoogle ScholarPubMed
Leray, J. 1934 Sur le mouvement d’un liquide visqueux emplissant l’espace. Acta Mathematica 63, 193–248.CrossRefGoogle Scholar
Luo, G. & Hou, T. Y. 2014 Potentially singular solutions of the 3D axisymmetric Euler equations. Proc. Natl Acad. Sci. USA 111 (36), 12968–12973.CrossRefGoogle ScholarPubMed
Luo, G. & Hou, T. Y. 2019 Formation of finite-time singularities in the 3D axisymmetric Euler equations: a numerics guided study. SIAM Rev. 61 (4), 793–835.CrossRefGoogle Scholar
Melander, M. V. & Hussain, F. 1988 Cut-and-connect of two antiparallel vortex tubes. In Studying Turbulence Using Numerical Simulation Databases, vol. 2, pp. 257–286. Center for Turbulence Research.Google Scholar
Moffatt, H. K. 2019 Singularities in fluid mechanics. Phys. Rev. Fluids 4, 110502.CrossRefGoogle Scholar
Moffatt, H. K. & Kimura, Y. 2019a Towards a finite-time singularity of the Navier–Stokes equations. Part 1. Derivation and analysis of dynamical system. J. Fluid Mech. 861, 930–967.CrossRefGoogle Scholar
Moffatt, H. K. & Kimura, Y. 2019b Towards a finite-time singularity of the Navier–Stokes equations. Part 2. Vortex reconnection and singularity evasion. J. Fluid Mech. 870, R1.CrossRefGoogle Scholar
Siggia, E. D. 1985 Collapse and amplification of a vortex filament. Phys. Fluids 28 (3), 794–805.CrossRefGoogle Scholar
Siggia, E. D. & Pumir, A. 1985 Incipient singularities in the Navier–Stokes equations. Phys. Rev. Lett. 55 (17), 1749–1752.CrossRefGoogle ScholarPubMed
Sreenivasan, K. R. 1984 On the scaling of the turbulence energy dissipation rate. Phys. Fluids 27 (5), 1048–1051.CrossRefGoogle Scholar
Villois, A., Proment, D. & Krstulovic, G. 2017 Universal and nonuniversal aspects of vortex reconnections in superfluids. Phys. Rev. Fluids 2 (4), 044701.CrossRefGoogle Scholar
Yao, J. & Hussain, F. 2020 A physical model of turbulence cascade via vortex reconnection sequence and avalanche. J. Fluid Mech. 883, A51.CrossRefGoogle Scholar
Zuccher, S., Caliari, M., Baggaley, A. W. & Barenghi, C. F. 2012 Quantum vortex reconnections. Phys. Fluids 24 (12), 125108.CrossRefGoogle Scholar

Yao et al. supplementary movie

Simulation of two slender inclined vortex rings for Reynolds number up to 4000.

Download Yao et al. supplementary movie(Video)
Video 15.1 MB