Hostname: page-component-cd9895bd7-gbm5v Total loading time: 0 Render date: 2024-12-28T22:05:18.957Z Has data issue: false hasContentIssue false
  • English
  • Français

On freely decaying, anisotropic, axisymmetric Saffman turbulence

Published online by Cambridge University Press:  02 July 2012

P. A. Davidson ,
N. Okamoto  and
Y. Kaneda
P. A. Davidson*
Affiliation:
Department of Engineering, University of Cambridge, Trumpington Street, Cambridge CB2 1PZ, UK
N. Okamoto
Affiliation:
Centre for Computational Science, Nagoya University, Nagoya 464-8603, Japan
Y. Kaneda
Affiliation:
Department of Computational Science & Engineering, Nagoya University, Nagoya 464-8603, Japan
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

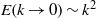We consider freely decaying, anisotropic, statistically axisymmetric, Saffman turbulence in which , where 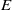is the energy spectrum and the wavenumber. We note that such turbulence possesses two statistical invariants which are related to the form of the spectral tensor 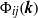at small 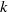. These are 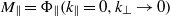and 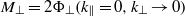, where the subscripts and indicate quantities parallel and perpendicular to the axis of symmetry. Since and , and 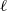being integral scales, self-similarity of the large scales (when it applies) demands and 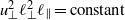. This, in turn, requires that is constant, contrary to the popular belief that freely decaying turbulence should exhibit a ‘return to isotropy’. Numerical simulations performed in large periodic domains, with different types and levels of initial anisotropy, confirm that 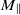and 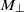are indeed invariants and that, in the fully developed state, 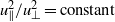. Somewhat surprisingly, the same simulations also show that 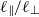is more or less constant in the fully developed state. Simple theoretical arguments are given which suggest that, when 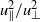and 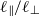are both constant, the integral scales should evolve as 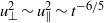and , irrespective of the level of anisotropy and of the presence of helicity. These decay laws, first proposed by Saffman (Phys. Fluids, vol. 10, 1967, p. 1349), are verified by the numerical simulations.

JFM classification

Turbulent Flows: Homogeneous turbulence Turbulent Flows: Turbulence simulation Turbulent Flows: Turbulence theory
Type
Papers
Information
Journal of Fluid Mechanics , Volume 706 , 10 September 2012 , pp. 150 - 172
Copyright
Copyright © Cambridge University Press 2012

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Batchelor, G. K. & Proudman, I. 1956 The large-scale structure of homogenous turbulence. Phil. Trans. R. Soc. Lond. A 248, 369–405.Google Scholar
2. Bennett, J. C. & Corssin, S. 1978 Small Reynolds number nearly isotropic turbulence in a straight duct and a contraction. Phys. Fluids 21 (12), 2129–2140.CrossRefGoogle Scholar
3. Chasnov, J. R. 1995 The decay of axisymmetric homogeneous turbulence. Phys. Fluids 7 (3), 600–605.CrossRefGoogle Scholar
4. Davidson, P. A. 2004 Turbulence: an Introduction for Scientists and Engineers. Oxford University Press.Google Scholar
5. Davidson, P. A. 2009 The role of angular momentum conservation in homogeneous turbulence. J. Fluid Mech. 632, 329–358.CrossRefGoogle Scholar
6. Davidson, P. A. 2010 On the decay of Saffman turbulence subject to rotation, stratification, or an imposed magnetic field. J. Fluid Mech. 663, 268–292.CrossRefGoogle Scholar
7. Davidson, P. A. 2011 The minimum energy decay rate in quasi-isotropic grid turbulence. Phys. Fluids 23, 085108.CrossRefGoogle Scholar
8. Ishida, T., Davidson, P. A. & Kaneda, Y. 2006 On the decay of isotropic turbulence. J. Fluid Mech. 564, 455.CrossRefGoogle Scholar
9. Kassinos, S. C., Reynolds, W. C. & Rogers, M. M. 2001 One-point turbulence structure tensors. J. Fluid Mech. 428, 213–248.CrossRefGoogle Scholar
10. Krogstad, P.-A. & Davidson, P. A. 2010 Is grid turbulence Saffman turbulence? J. Fluid Mech. 642, 373–394.CrossRefGoogle Scholar
11. Lavoie, P., Djenidi, L. & Antonia, R. A. 2007 Effects of initial conditions in decaying turbulence generated by passive grids. J. Fluid Mech. 585, 395–420.CrossRefGoogle Scholar
12. Saffman, P. G. 1967a The large-scale structure of homogeneous turbulence. J. Fluid Mech. 27 (3), 581–593.CrossRefGoogle Scholar
13. Saffman, P. G. 1967b Note on decay of homogeneous turbulence. Phys. Fluids 10 (6), 1349.CrossRefGoogle Scholar
14. Townsend, A. A. 1976 The Structure of Turbulence Shear Flow. Cambridge University Press.Google Scholar