Hostname: page-component-586b7cd67f-2brh9 Total loading time: 0 Render date: 2024-11-26T23:26:39.933Z Has data issue: false hasContentIssue false
  • English
  • Français

Numerical evidence of anomalous energy dissipation in incompressible Euler flows: towards grid-converged results for the inviscid Taylor–Green problem

Published online by Cambridge University Press:  15 December 2021

Niklas Fehn Open the ORCID record for Niklas Fehn [Opens in a new window] ,
Martin Kronbichler Open the ORCID record for Martin Kronbichler [Opens in a new window] ,
Peter Munch  and
Wolfgang A. Wall Open the ORCID record for Wolfgang A. Wall [Opens in a new window]
Niklas Fehn*
Affiliation:
Institute for Computational Mechanics, Technical University of Munich, Boltzmannstr. 15, 85748 Garching, Germany
Martin Kronbichler
Affiliation:
Institute for Computational Mechanics, Technical University of Munich, Boltzmannstr. 15, 85748 Garching, Germany Division of Scientific Computing, Department of Information Technology, Uppsala University, 75105 Uppsala, Sweden
Peter Munch
Affiliation:
Institute for Computational Mechanics, Technical University of Munich, Boltzmannstr. 15, 85748 Garching, Germany Institute of Material Systems Modeling, Helmholtz-Zentrum Hereon, Max-Planck-Str. 1, 21502 Geesthacht, Germany
Wolfgang A. Wall
Affiliation:
Institute for Computational Mechanics, Technical University of Munich, Boltzmannstr. 15, 85748 Garching, Germany
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

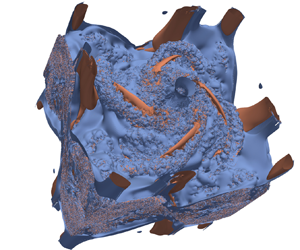
The well-known energy dissipation anomaly in the inviscid limit, related to velocity singularities according to Onsager, still needs to be demonstrated by numerical experiments. The present work contributes to this topic through high-resolution numerical simulations of the inviscid three-dimensional Taylor–Green vortex problem using a novel high-order discontinuous Galerkin discretisation approach for the incompressible Euler equations. The main methodological ingredient is the use of a discretisation scheme with inbuilt dissipation mechanisms, as opposed to discretely energy-conserving schemes, which – by construction – rule out the occurrence of anomalous dissipation. We investigate effective spatial resolution up to $8192^3$ (defined based on the $2{\rm \pi}$-periodic box) and make the interesting phenomenological observation that the kinetic energy evolution does not tend towards exact energy conservation for increasing spatial resolution of the numerical scheme, but that the sequence of discrete solutions seemingly converges to a solution with non-zero kinetic energy dissipation rate. Taking the fine-resolution simulation as a reference, we measure grid-convergence with a relative $L^2$-error of $0.27\,\%$ for the temporal evolution of the kinetic energy and $3.52\,\%$ for the kinetic energy dissipation rate against the dissipative fine-resolution simulation. The present work raises the question of whether such results can be seen as a numerical confirmation of the famous energy dissipation anomaly. Due to the relation between anomalous energy dissipation and the occurrence of singularities for the incompressible Euler equations according to Onsager's conjecture, we elaborate on an indirect approach for the identification of finite-time singularities that relies on energy arguments.

JFM classification

Mathematical Foundations: Computational methods Turbulent Flows: Turbulence theory
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 932 , 10 February 2022 , A40
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Agafontsev, D.S., Kuznetsov, E.A. & Mailybaev, A.A. 2015 Development of high vorticity structures in incompressible 3D Euler equations. Phys. Fluids 27 (8), 085102.CrossRefGoogle Scholar
Ainsworth, M. 2004 Dispersive and dissipative behaviour of high order discontinuous Galerkin finite element methods. J. Comput. Phys. 198 (1), 106–130.CrossRefGoogle Scholar
Alzetta, G., et al. 2018 The deal.II library, version 9.0. J. Numer. Maths 26 (4), 173–184.CrossRefGoogle Scholar
Arndt, D., Bangerth, W., Davydov, D., Heister, T., Heltai, L., Kronbichler, M., Maier, M., Pelteret, J.-P., Turcksin, B. & Wells, D. 2021 The deal.II finite element library: design, features, and insights. Comput. Maths. Applics. 81, 407–422.CrossRefGoogle Scholar
Arndt, D., Fehn, N., Kanschat, G., Kormann, K., Kronbichler, M., Munch, P., Wall, W.A. & Witte, J. 2020 ExaDG: high-order discontinuous Galerkin for the exa-scale. In Software for Exascale Computing - SPPEXA 2016-2019 (ed. H.-J. Bungartz, S. Reiz, B. Uekermann, P. Neumann & W.E. Nagel), pp. 189–224. Springer.CrossRefGoogle Scholar
Beale, J.T., Kato, T. & Majda, A. 1984 Remarks on the breakdown of smooth solutions for the 3-D Euler equations. Commun. Math. Phys. 94 (1), 61–66.CrossRefGoogle Scholar
Boratav, O.N. & Pelz, R.B. 1994 Direct numerical simulation of transition to turbulence from a high-symmetry initial condition. Phys. Fluids 6 (8), 2757–2784.CrossRefGoogle Scholar
Brachet, M.E. 1991 Direct simulation of three-dimensional turbulence in the Taylor–Green vortex. Fluid Dyn. Res. 8 (1–4), 1–8.CrossRefGoogle Scholar
Brachet, M.E., Meiron, D.I., Orszag, S.A., Nickel, B.G., Morf, R.H. & Frisch, U. 1983 Small-scale structure of the Taylor–Green vortex. J. Fluid Mech. 130, 411–452.CrossRefGoogle Scholar
Brachet, M.E., Meneguzzi, M., Vincent, A., Politano, H. & Sulem, P.L. 1992 Numerical evidence of smooth self-similar dynamics and possibility of subsequent collapse for three-dimensional ideal flows. Phys. Fluids A 4 (12), 2845–2854.CrossRefGoogle Scholar
Brenier, Y., De Lellis, C. & Székelyhidi, L. 2011 Weak-strong uniqueness for measure-valued solutions. Commun. Math. Phys. 305 (2), 351–361.CrossRefGoogle Scholar
Brenner, M.P., Hormoz, S. & Pumir, A. 2016 Potential singularity mechanism for the Euler equations. Phys. Rev. Fluids 1, 084503.CrossRefGoogle Scholar
Brown, D.L. 1995 Performance of under-resolved two-dimensional incompressible flow simulations. J. Comput. Phys. 122 (1), 165–183.CrossRefGoogle Scholar
Buckmaster, T., De Lellis, C. & Székelyhidi, L. Jr. 2016 Dissipative Euler flows with Onsager-critical spatial regularity. Commun. Pure Appl. Maths 69 (9), 1613–1670.CrossRefGoogle Scholar
Buckmaster, T., De Lellis, C., Székelyhidi, L. Jr. & Vicol, V. 2018 Onsager's conjecture for admissible weak solutions. Commun. Pure Appl. Maths 72 (2), 229–274.CrossRefGoogle Scholar
Buckmaster, T., Masmoudi, N., Novack, M. & Vicol, V. 2021 Non-conservative $H^1/2$-weak solutions of the incompressible 3D Euler equations. arXiv:2101.09278.Google Scholar
Buckmaster, T. & Vicol, V. 2019 Nonuniqueness of weak solutions to the Navier–Stokes equation. Ann. Maths 189 (1), 101–144.CrossRefGoogle Scholar
Buckmaster, T. & Vicol, V. 2020 Convex integration and phenomenologies in turbulence. EMS Surv. Math. Sci. 6 (1), 173–263.CrossRefGoogle Scholar
Burgers, J.M. 1948 A mathematical model illustrating the theory of turbulence. Adv. Appl. Mech. 1, 171–199.CrossRefGoogle Scholar
Bustamante, M.D. & Brachet, M. 2012 Interplay between the Beale–Kato–Majda theorem and the analyticity-strip method to investigate numerically the incompressible Euler singularity problem. Phys. Rev. E 86, 066302.CrossRefGoogle ScholarPubMed
Campolina, C.S. & Mailybaev, A.A. 2018 Chaotic blowup in the 3D incompressible Euler equations on a logarithmic lattice. Phys. Rev. Lett. 121, 064501.CrossRefGoogle ScholarPubMed
Chalmers, N., Agbaglah, G., Chrust, M. & Mavriplis, C. 2019 A parallel hp-adaptive high order discontinuous Galerkin method for the incompressible Navier–Stokes equations. J. Comput. Phys. X 2, 100023.Google Scholar
Chapelier, J.-B., De La Llave Plata, M. & Renac, F. 2012 Inviscid and viscous simulations of the Taylor–Green vortex flow using a modal discontinuous Galerkin approach. In 42nd AIAA Fluid Dynamics Conference and Exhibit, AIAA Paper 2012-3073.Google Scholar
Cheskidov, A., Constantin, P., Friedlander, S. & Shvydkoy, R. 2008 Energy conservation and Onsager's conjecture for the Euler equations. Nonlinearity 21 (6), 1233–1252.CrossRefGoogle Scholar
Cichowlas, C., Bonaïti, P., Debbasch, F. & Brachet, M. 2005 Effective dissipation and turbulence in spectrally truncated Euler flows. Phys. Rev. Lett. 95, 264502.CrossRefGoogle ScholarPubMed
Cichowlas, C. & Brachet, M.-E. 2005 Evolution of complex singularities in Kida–Pelz and Taylor–Green inviscid flows. Fluid Dyn. Res. 36 (4–6), 239–248.CrossRefGoogle Scholar
Constantin, P., Weinan, E. & Titi, E.S. 1994 Onsager's conjecture on the energy conservation for solutions of Euler's equation. Commun. Math. Phys. 165 (1), 207–209.CrossRefGoogle Scholar
Coppola, G., Capuano, F. & de Luca, L. 2019 Discrete energy-conservation properties in the numerical simulation of the Navier–Stokes equations. Appl. Mech. Rev. 71 (1), 010803.CrossRefGoogle Scholar
Daneri, S., Runa, E. & Székelyhidi, L. 2021 Non-uniqueness for the Euler equations up to Onsager's critical exponent. Ann. PDE 7 (1), 1–44.CrossRefGoogle Scholar
De Lellis, C. & Székelyhidi, L. Jr. 2013 Dissipative continuous Euler flows. Invent. Math. 193 (2), 377–407.CrossRefGoogle Scholar
De Lellis, C. & Székelyhidi, L. Jr. 2014 Dissipative Euler flows and Onsager's conjecture. J. Eur. Math. Soc. 16 (7), 1467–1505.CrossRefGoogle Scholar
DiPerna, R.J. & Majda, A.J. 1987 Oscillations and concentrations in weak solutions of the incompressible fluid equations. Commun. Math. Phys. 108 (4), 667–689.CrossRefGoogle Scholar
Drivas, T.D. & Eyink, G.L. 2019 An onsager singularity theorem for Leray solutions of incompressible Navier–Stokes. Nonlinearity 32 (11), 4465.CrossRefGoogle Scholar
Drivas, T.D. & Nguyen, H.Q. 2019 Remarks on the emergence of weak Euler solutions in the vanishing viscosity limit. J. Nonlinear Sci. 29 (2), 709–721.CrossRefGoogle Scholar
Dubrulle, B. 2019 Beyond Kolmogorov cascades. J. Fluid Mech. 867, P1.CrossRefGoogle Scholar
Duchon, J. & Robert, R. 2000 Inertial energy dissipation for weak solutions of incompressible Euler and Navier–Stokes equations. Nonlinearity 13 (1), 249–255.CrossRefGoogle Scholar
Eggers, J. 2018 Role of singularities in hydrodynamics. Phys. Rev. Fluids 3, 110503.CrossRefGoogle Scholar
Eyink, G.L. 1994 Energy dissipation without viscosity in ideal hydrodynamics I. Fourier analysis and local energy transfer. Physica D 78 (3), 222–240.CrossRefGoogle Scholar
Eyink, G.L. 2008 Dissipative anomalies in singular Euler flows. Physica D 237 (14), 1956–1968.CrossRefGoogle Scholar
Eyink, G.L. & Sreenivasan, K.R. 2006 Onsager and the theory of hydrodynamic turbulence. Rev. Mod. Phys. 78, 87–135.CrossRefGoogle Scholar
Fehn, N., Heinz, J., Wall, W.A. & Kronbichler, M. 2021 High-order arbitrary Lagrangian–Eulerian discontinuous Galerkin methods for the incompressible Navier–Stokes equations. J. Comput. Phys. 430, 110040.CrossRefGoogle Scholar
Fehn, N., Kronbichler, M., Lehrenfeld, C., Lube, G. & Schroeder, P.W. 2019 High-order DG solvers for under-resolved turbulent incompressible flows: a comparison of $L^2$ and $H$(div) methods. Intl J. Numer. Meth. Fluids 91 (11), 533–556.CrossRefGoogle Scholar
Fehn, N., Munch, P., Wall, W.A. & Kronbichler, M. 2020 Hybrid multigrid methods for high-order discontinuous Galerkin discretizations. J. Comput. Phys. 415, 109538.CrossRefGoogle Scholar
Fehn, N., Wall, W.A. & Kronbichler, M. 2017 On the stability of projection methods for the incompressible Navier–Stokes equations based on high-order discontinuous Galerkin discretizations. J. Comput. Phys. 351, 392–421.CrossRefGoogle Scholar
Fehn, N., Wall, W.A. & Kronbichler, M. 2018 a Efficiency of high-performance discontinuous Galerkin spectral element methods for under-resolved turbulent incompressible flows. Intl J. Numer. Meth. Fluids 88 (1), 32–54.CrossRefGoogle Scholar
Fehn, N., Wall, W.A. & Kronbichler, M. 2018 b Robust and efficient discontinuous Galerkin methods for under-resolved turbulent incompressible flows. J. Comput. Phys. 372, 667–693.CrossRefGoogle Scholar
Fernandez, P., Nguyen, N.C. & Peraire, J. 2018 On the ability of discontinuous Galerkin methods to simulate under-resolved turbulent flows. arXiv:1810.09435.Google Scholar
Fischer, P. & Mullen, J. 2001 Filter-based stabilization of spectral element methods. C. R. Acad. Sci. I 332 (3), 265–270.CrossRefGoogle Scholar
Flad, D. & Gassner, G. 2017 On the use of kinetic energy preserving DG-schemes for large eddy simulation. J. Comput. Phys. 350, 782–795.CrossRefGoogle Scholar
Frigo, M. & Johnson, S.G. 2005 The design and implementation of FFTW3. Proc. IEEE 93 (2), 216–231.CrossRefGoogle Scholar
Fu, G. 2019 An explicit divergence-free DG method for incompressible flow. Comput. Meth. Appl. Mech. Engng 345, 502–517.CrossRefGoogle Scholar
Gibbon, J.D. 2008 The three-dimensional Euler equations: where do we stand? Physica D 237 (14), 1894–1904.CrossRefGoogle Scholar
Grafke, T., Homann, H., Dreher, J. & Grauer, R. 2008 Numerical simulations of possible finite time singularities in the incompressible Euler equations: comparison of numerical methods. Physica D 237 (14), 1932–1936.CrossRefGoogle Scholar
Grauer, R., Marliani, C. & Germaschewski, K. 1998 Adaptive mesh refinement for singular solutions of the incompressible Euler equations. Phys. Rev. Lett. 80, 4177–4180.CrossRefGoogle Scholar
Guzmán, J., Shu, C.-W. & Sequeira, F.A. 2016 H(div) conforming and DG methods for incompressible Euler's equations. IMA J. Numer. Anal. 37 (4), 1733–1771.Google Scholar
Hou, T.Y. & Li, R. 2008 Blowup or no blowup? The interplay between theory and numerics. Physica D 237 (14), 1937–1944.CrossRefGoogle Scholar
Isett, P. 2017 Nonuniqueness and existence of continuous, globally dissipative Euler flows. arXiv:1710.11186.Google Scholar
Isett, P. 2018 A proof of Onsager's conjecture. Ann. Maths 188 (3), 871–963.CrossRefGoogle Scholar
Josserand, C., Pomeau, Y. & Rica, S. 2020 Finite-time localized singularities as a mechanism for turbulent dissipation. Phys. Rev. Fluids 5, 054607.CrossRefGoogle Scholar
Kaneda, Y., Ishihara, T., Yokokawa, M., Itakura, K. & Uno, A. 2003 Energy dissipation rate and energy spectrum in high resolution direct numerical simulations of turbulence in a periodic box. Phys. Fluids 15 (2), L21–L24.CrossRefGoogle Scholar
Karniadakis, G.E., Israeli, M. & Orszag, S.A. 1991 High-order splitting methods for the incompressible Navier–Stokes equations. J. Comput. Phys. 97 (2), 414–443.CrossRefGoogle Scholar
Karniadakis, G.E. & Sherwin, S.J. 2005 Spectral/HP Element Methods for Computational Fluid Dynamics, 2nd edn. Oxford University Press.CrossRefGoogle Scholar
Kerr, R.M. 1993 Evidence for a singularity of the three-dimensional, incompressible Euler equations. Phys. Fluids A 5 (7), 1725–1746.CrossRefGoogle Scholar
Kerr, R.M. 2013 Bounds for Euler from vorticity moments and line divergence. J. Fluid Mech. 729, R2.CrossRefGoogle Scholar
Kerr, R.M. & Hussain, F. 1989 Simulation of vortex reconnection. Physica D 37 (1), 474–484.CrossRefGoogle Scholar
Kida, S. 1985 Three-dimensional periodic flows with high-symmetry. J. Phys. Soc. Japan 54 (6), 2132–2136.CrossRefGoogle Scholar
Kolmogorov, A.N. 1991 The local structure of turbulence in incompressible viscous fluid for very large Reynolds numbers. Proc. R. Soc. Lond. A 434 (1890), 9–13.Google Scholar
Krais, N., Schnücke, G., Bolemann, T. & Gassner, G.J. 2020 Split form ALE discontinuous Galerkin methods with applications to under-resolved turbulent low-Mach number flows. J. Comput. Phys. 421, 109726.CrossRefGoogle Scholar
Krank, B., Fehn, N., Wall, W.A. & Kronbichler, M. 2017 A high-order semi-explicit discontinuous Galerkin solver for 3D incompressible flow with application to DNS and LES of turbulent channel flow. J. Comput. Phys. 348, 634–659.CrossRefGoogle Scholar
Kronbichler, M. & Kormann, K. 2019 Fast matrix-free evaluation of discontinuous Galerkin finite element operators. ACM Trans. Math. Softw. 45 (3), 29:1–29:40.CrossRefGoogle Scholar
Kuzzay, D., Saw, E.-W., Martins, F.J.W.A., Faranda, D., Foucaut, J.-M., Daviaud, F. & Dubrulle, B. 2017 New method for detecting singularities in experimental incompressible flows. Nonlinearity 30 (6), 2381.CrossRefGoogle Scholar
Lamballais, E., Dairay, T., Laizet, S. & Vassilicos, J.C. 2019 Implicit/explicit spectral viscosity and large-scale SGS effects. In Direct and Large-Eddy Simulation XI (ed. M.V. Salvetti, V. Armenio, J. Fröhlich, B.J. Geurts & H. Kuerten), pp. 107–113. Springer.CrossRefGoogle Scholar
Larios, A., Petersen, M.R., Titi, E.S. & Wingate, B. 2018 A computational investigation of the finite-time blow-up of the 3D incompressible Euler equations based on the Voigt regularization. Theor. Comput. Fluid Dyn. 32 (1), 23–34.CrossRefGoogle Scholar
Lions, P.-L. 1996 Mathematical Topics in Fluid Mechanics. Volume 1: Incompressible Models, Oxford Lecture Series in Mathematics and its Applications, vol. 3. Oxford University Press.Google Scholar
Luo, G. & Hou, T.Y. 2014 Potentially singular solutions of the 3D axisymmetric Euler equations. Proc. Natl Acad. Sci. USA 111 (36), 12968–12973.CrossRefGoogle ScholarPubMed
Manzanero, J., Ferrer, E., Rubio, G. & Valero, E. 2020 Design of a Smagorinsky spectral vanishing viscosity turbulence model for discontinuous Galerkin methods. Comput. Fluids 200, 104440.CrossRefGoogle Scholar
McKeown, R., Ostilla-Mónico, R., Pumir, A., Brenner, M.P. & Rubinstein, S.M. 2018 Cascade leading to the emergence of small structures in vortex ring collisions. Phys. Rev. Fluids 3, 124702.CrossRefGoogle Scholar
Moffatt, H.K. 2019 Singularities in fluid mechanics. Phys. Rev. Fluids 4, 110502.CrossRefGoogle Scholar
Morf, R.H., Orszag, S.A. & Frisch, U. 1980 Spontaneous singularity in three-dimensional inviscid, incompressible flow. Phys. Rev. Lett. 44, 572–575.CrossRefGoogle Scholar
Moura, R., Mengaldo, G., Peiró, J. & Sherwin, S. 2017 a An LES setting for DG-based implicit LES with insights on dissipation and robustness. In Spectral and High Order Methods for Partial Differential Equations (ed. M.L. Bittencourt, N.A. Dumont & J.S. Hesthaven), pp. 161–173. Springer.CrossRefGoogle Scholar
Moura, R.C., Mengaldo, G., Peiró, J. & Sherwin, S.J. 2017 b On the eddy-resolving capability of high-order discontinuous Galerkin approaches to implicit LES/under-resolved DNS of Euler turbulence. J. Comput. Phys. 330, 615–623.CrossRefGoogle Scholar
Murugan, S.D., Frisch, U., Nazarenko, S., Besse, N. & Ray, S.S. 2020 Suppressing thermalization and constructing weak solutions in truncated inviscid equations of hydrodynamics: lessons from the Burgers equation. Phys. Rev. Res. 2, 033202.CrossRefGoogle Scholar
Onsager, L. 1949 Statistical hydrodynamics. Il Nuovo Cimento 6, 279–287.CrossRefGoogle Scholar
Orlandi, P. 2009 Energy spectra power laws and structures. J. Fluid Mech. 623, 353–374.CrossRefGoogle Scholar
Orlandi, P., Pirozzoli, S. & Carnevale, G.F. 2012 Vortex events in Euler and Navier–Stokes simulations with smooth initial conditions. J. Fluid Mech. 690, 288–320.CrossRefGoogle Scholar
Pearson, B.R., Krogstad, P.-Å. & van de Water, W. 2002 Measurements of the turbulent energy dissipation rate. Phys. Fluids 14 (3), 1288–1290.CrossRefGoogle Scholar
Pelz, R.B. 2001 Symmetry and the hydrodynamic blow-up problem. J. Fluid Mech. 444, 299–320.CrossRefGoogle Scholar
Pelz, R.B. & Gulak, Y. 1997 Evidence for a real-time singularity in hydrodynamics from time series analysis. Phys. Rev. Lett. 79, 4998–5001.CrossRefGoogle Scholar
Piatkowski, S.-M. 2019 A spectral discontinuous Galerkin method for incompressible flow with applications to turbulence. PhD thesis, Ruprecht-Karls-Universität Heidelberg.Google Scholar
Ray, S.S., Frisch, U., Nazarenko, S. & Matsumoto, T. 2011 Resonance phenomenon for the Galerkin-truncated Burgers and Euler equations. Phys. Rev. E 84, 016301.CrossRefGoogle ScholarPubMed
Saw, E.-W., Kuzzay, D., Faranda, D., Guittonneau, A., Daviaud, F., Wiertel-Gasquet, C., Padilla, V. & Dubrulle, B. 2016 Experimental characterization of extreme events of inertial dissipation in a turbulent swirling flow. Nat. Commun. 7, 12466.CrossRefGoogle Scholar
Schroeder, P.W. 2019 Robustness of high-order divergence-free finite element methods for incompressible computational fluid dynamics. PhD thesis, Georg-August-Universität Göttingen.Google Scholar
Schroeder, P.W. & Lube, G. 2018 Divergence-free $H$(div)-FEM for time-dependent incompressible flows with applications to high Reynolds number vortex dynamics. J. Sci. Comput. 75 (2), 830–858.CrossRefGoogle Scholar
Shu, C.-W., Don, W.-S., Gottlieb, D., Schilling, O. & Jameson, L. 2005 Numerical convergence study of nearly incompressible, inviscid Taylor–Green vortex flow. J. Sci. Comput. 24 (1), 1–27.CrossRefGoogle Scholar
Sreenivasan, K.R. 1998 An update on the energy dissipation rate in isotropic turbulence. Phys. Fluids 10 (2), 528–529.CrossRefGoogle Scholar
Sulem, C., Sulem, P.L. & Frisch, H. 1983 Tracing complex singularities with spectral methods. J. Comput. Phys. 50 (1), 138–161.CrossRefGoogle Scholar
Taylor, G.I. 1935 Statistical theory of turbulence. Proc. R. Soc. Lond. A 151 (873), 421–444.CrossRefGoogle Scholar
Taylor, G.I. & Green, A.E. 1937 Mechanism of the production of small eddies from large ones. Proc. R. Soc. Lond. A 158 (895), 499–521.Google Scholar
Thalabard, S., Bec, J. & Mailybaev, A.A. 2020 From the butterfly effect to spontaneous stochasticity in singular shear flows. Commun. Phys. 3 (1), 122.CrossRefGoogle Scholar
Wiedemann, E. 2017 Weak-strong uniqueness in fluid dynamics. arXiv:1705.04220.Google Scholar
Winters, A.R., Moura, R.C., Mengaldo, G., Gassner, G.J., Walch, S., Peiro, J. & Sherwin, S.J. 2018 A comparative study on polynomial dealiasing and split form discontinuous Galerkin schemes for under-resolved turbulence computations. J. Comput. Phys. 372, 1–21.CrossRefGoogle Scholar
Supplementary material: File

Fehn et al. supplementary material

Fehn et al. supplementary material

Download Fehn et al. supplementary material(File)
File 7.7 MB