Article contents
Non-local energy transfers in rotating turbulence at intermediate Rossby number
Published online by Cambridge University Press: 23 November 2011
Abstract
Turbulent flows subject to solid-body rotation are known to generate steep energy spectra and two-dimensional columnar vortices. The localness of the dominant energy transfers responsible for the accumulation of the energy in the two-dimensional columnar vortices of large horizontal scale remains undetermined. Here, we investigate the scale-locality of the energy transfers directly contributing to the growth of the two-dimensional columnar structures observed in the intermediate Rossby number (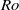 ) regime. Our approach is to investigate the dynamics of the waves and vortices separately: we ensure that the two-dimensional columnar structures are not directly forced so that the vortices can result only from association with wave to vortical energy transfers. Detailed energy transfers between waves and vortices are computed as a function of scale, allowing the direct tracking of the role and scales of the wave–vortex nonlinear interactions in the accumulation of energy in the large two-dimensional columnar structures. It is shown that the dominant energy transfers responsible for the generation of a steep two-dimensional spectrum involve direct non-local energy transfers from small-frequency small-horizontal-scale three-dimensional waves to large-horizontal-scale two-dimensional columnar vortices. Sensitivity of the results to changes in resolution and forcing scales is investigated and the non-locality of the dominant energy transfers leading to the emergence of the columnar vortices is shown to be robust. The interpretation of the scaling law observed in rotating flows in the intermediate-
) regime. Our approach is to investigate the dynamics of the waves and vortices separately: we ensure that the two-dimensional columnar structures are not directly forced so that the vortices can result only from association with wave to vortical energy transfers. Detailed energy transfers between waves and vortices are computed as a function of scale, allowing the direct tracking of the role and scales of the wave–vortex nonlinear interactions in the accumulation of energy in the large two-dimensional columnar structures. It is shown that the dominant energy transfers responsible for the generation of a steep two-dimensional spectrum involve direct non-local energy transfers from small-frequency small-horizontal-scale three-dimensional waves to large-horizontal-scale two-dimensional columnar vortices. Sensitivity of the results to changes in resolution and forcing scales is investigated and the non-locality of the dominant energy transfers leading to the emergence of the columnar vortices is shown to be robust. The interpretation of the scaling law observed in rotating flows in the intermediate-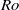 regime is revisited in the light of this new finding of dominant non-locality.
regime is revisited in the light of this new finding of dominant non-locality.
JFM classification
- Type
- Papers
- Information
- Copyright
- Copyright © Cambridge University Press 2011
References
- 28
- Cited by


