Hostname: page-component-745bb68f8f-s22k5 Total loading time: 0 Render date: 2025-01-09T22:13:32.582Z Has data issue: false hasContentIssue false
  • English
  • Français

New oscillatory instability of a confined cylinder in a flow below the vortex shedding threshold

Published online by Cambridge University Press:  24 November 2011

B. Semin ,
A. Decoene ,
J.-P. Hulin ,
M. L. M. François  and
H. Auradou
B. Semin*
Affiliation:
Laboratoire FAST, Université Paris-Sud, Université Pierre et Marie Curie–Paris 6, CNRS, Bat. 502, Campus Univ., Orsay, F-91405, France
A. Decoene
Affiliation:
Department of Mathematics, Université Paris-Sud, Bat. 452, Campus Univ., Orsay, F-91405, France
J.-P. Hulin
Affiliation:
Laboratoire FAST, Université Paris-Sud, Université Pierre et Marie Curie–Paris 6, CNRS, Bat. 502, Campus Univ., Orsay, F-91405, France
M. L. M. François
Affiliation:
Laboratoire FAST, Université Paris-Sud, Université Pierre et Marie Curie–Paris 6, CNRS, Bat. 502, Campus Univ., Orsay, F-91405, France
H. Auradou
Affiliation:
Laboratoire FAST, Université Paris-Sud, Université Pierre et Marie Curie–Paris 6, CNRS, Bat. 502, Campus Univ., Orsay, F-91405, France
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

A new type of flow-induced oscillation is reported for a tethered cylinder confined inside a Hele-Shaw cell (ratio of cylinder diameter to cell aperture, 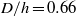) with its main axis perpendicular to the flow. This instability is studied numerically and experimentally as a function of the Reynolds number 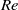and of the density 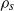of the cylinder. This confinement-induced vibration (CIV) occurs above a critical Reynolds number 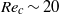much lower than for Bénard–Von Kármán vortex shedding behind a fixed cylinder in the same configuration (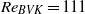). For low values, CIV persists up to the highest 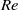value investigated (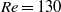). For denser cylinders, these oscillations end abruptly above a second value of larger than 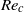and vortex-induced vibrations (VIV) of lower amplitude appear for . Close to the first threshold , the oscillation amplitude variation as 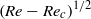and the lack of hysteresis demonstrate that the process is a supercritical Hopf bifurcation. Using forced oscillations, the transverse position of the cylinder is shown to satisfy a Van der Pol equation. The physical meaning of the stiffness, amplification and total mass coefficients of this equation are discussed from the variations of the pressure field.

JFM classification

Instability: Instability Nonlinear Dynamical Systems: Low-dimensional models
Type
Papers
Information
Journal of Fluid Mechanics , Volume 690 , 10 January 2012 , pp. 345 - 365
Copyright
Copyright © Cambridge University Press 2011

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Anagnostopoulos, P. & Bearman, P. W. 1992 Response characteristics of a vortex-excited cylinder at low Reynolds number. J. Fluids Struct. 6, 39–50.CrossRefGoogle Scholar
2. Carberry, J., Sheridan, J. & Rockwell, D. 2005 Controlled oscillations of a cylinder: forces and wake modes. J. Fluid Mech. 538, 31–69.CrossRefGoogle Scholar
3. Chen, J.-H., Pritchard, W. G. & Tavener, S. J. 1995 Bifurcation for flow past a cylinder between parallel plates. J. Fluid Mech. 284, 23–41.CrossRefGoogle Scholar
4. Cossu, C. & Morino, L. 2000 On the instability of a spring-mounted circular cylinder in a viscous flow at low Reynolds numbers. J. Fluids Struct. 14, 183–196.Google Scholar
5. Facchinetti, M. L., de Langre, E. & Biolley, F. 2004 Coupling of structure and wake oscillators in vortex induced vibrations. J. Fluids Struct. 19, 123–140.CrossRefGoogle Scholar
6. Gabbai, R. D. & Benaroya, H. 2005 An overview of modelling and experiments of vortex-induced vibration of circular cylinders. J. Sound Vib. 282, 575–616.CrossRefGoogle Scholar
7. Govardhan, R. & Williamson, C. H. K. 2002 Resonance forever: existence of a critical mass and an infinite regime of resonance in vortex-induced vibration. J. Fluid Mech. 473, 147–166.CrossRefGoogle Scholar
8. Hecht, F., Pironneau, O., le Hyaric, A. & Ohtsuka, K. 2010 FreeFem++ manual.http://www.freefem.org/ff++/.Google Scholar
9. Hirt, C. W., Amsden, A. A. & Cook, J. L. 1974 An arbitrary Lagrangian–Eulerian computing method for all flow speeds. J. Comput. Phys. 14, 227–253.CrossRefGoogle Scholar
10. Horowitz, M. & Williamson, C. H. K. 2006 Dynamics of a rising and falling cylinder. J. Fluids Struct. 22, 837–843.Google Scholar
11. Janela, J., Lefebvre, A. & Maury, B. 2005 A penalty method for the simulation of fluid–rigid body interaction. ESAIM: Proc. 14, 115–123.CrossRefGoogle Scholar
12. Lazarkov, M. & Revstedt, J. 2008 Flow-induced motion of a short circular cylinder spanning a rectangular channel. J. Fluids Struct. 24, 449–466.Google Scholar
13. Lefebvre, A. 2007 Fluid–particle simulations with Freefem++. ESAIM: Proc. 18, 120–132.CrossRefGoogle Scholar
14. Leontini, J. S., Stewart, B. E., Thompson, M. C. & Kourigan, K. 2006 Wake state and energy transitions of an oscillating cylinder at low Reynolds number. Phys. Fluids 18, 067101.Google Scholar
15. Manneville, P. 2004 Instabilities, Chaos and Turbulence: an Introduction to Nonlinear Dynamics and Complex Systems. Imperial College Press.CrossRefGoogle Scholar
16. Maury, B. 1996 Characteristics ALE method for the unsteady 3D Navier–Stokes equations with a free surface. Comput. Fluid Dyn. 6, 175–188.CrossRefGoogle Scholar
17. Meis, M., Varas, F., Velàsquez, A. & Vega, J. M. 2010 Heat transfer enhancement in micro-channels caused by vortex promoters. Intl J. Heat Mass Transfer 53, 29–40.CrossRefGoogle Scholar
18. Mittal, S. & Singh, S. 2005 Vortex-induced vibrations at subcritical Re . J. Fluid Mech. 534, 185–194.CrossRefGoogle Scholar
19. Morse, T. L. & Williamson, C. H. K. 2009 Prediction of vortex-induced vibration response by employing controlled motion. J. Fluid Mech. 634, 5–39.CrossRefGoogle Scholar
20. Nayfeh, A. H. & Mook, D. T. 1995 Nonlinear Oscillations. John Wiley.CrossRefGoogle Scholar
21. Placzek, A., Sigrist, J.-P. & Hamdouni, A. 2009 Numerical simulation of an oscillating cylinder in a cross-flow at low Reynolds number: forced and free oscillations. Comput. Fluids 38, 80–100.CrossRefGoogle Scholar
22. Prasanth, T. K., Behara, S., Singh, S. P. & Mittal, S. 2006 Effect of blockage on vortex-induced vibrations at low Reynolds numbers. J. Fluids Struct. 22, 865–876.CrossRefGoogle Scholar
23. Rehimi, F., Alaoui, F., Nasrallah, S. B., Doubliez, L. & Legrand, J. 2008 Experimental investigation of a confined flow downstream of a circular cylinder centred between two parallel walls. J. Fluids Struct. 24, 855–882.CrossRefGoogle Scholar
24. Sánchez-Sanz, M., Fernandez, B. & Velazquez, A. 2009 Energy-harvesting microresonator based on the forces generated by the Kármán street around a rectangular prism. J. Microelectromech. Syst. 18, 449–457.CrossRefGoogle Scholar
25. Sánchez-Sanz, M. & Velazquez, A. 2009 Vortex-induced vibration of a prism in internal flow. J. Fluid Mech. 641, 431–440.CrossRefGoogle Scholar
26. Sarpkaya, T. 2004 A critical review of the intrinsic nature of vortex-induced vibrations. J. Fluids Struct. 19, 389–447.CrossRefGoogle Scholar
27. Semin, B., François, M. L. M. & Auradou, H. 2011 Accurate measurement of curvilinear shapes by virtual image correlation. Eur. Phys. J. Appl. Phys. 56, 10701.CrossRefGoogle Scholar
28. Semin, B., Hulin, J. P. & Auradou, H. 2009 Influence of flow confinement on the drag force on a static cylinder. Phys. Fluids 21 (10), 103604.CrossRefGoogle Scholar
29. Shair, F. H., Grove, A. S., Petersen, E. E. & Acrivos, A. 1963 The effect of confining walls on the stability of the steady wake behind a circular cylinder. J. Fluid Mech. 17, 546–550.CrossRefGoogle Scholar
30. Shiels, D., Leonard, A. & Roshko, A. 2001 Flow-induced vibration of a circular cylinder at limiting structural parameters. J. Fluids Struct. 15, 3–21.Google Scholar
31. Weast, R. C. & Astle, M. J. 1982 Handbook of Chemistry and Physics. CRC.Google Scholar
32. Williamson, C. H. K. & Govardhan, R. 2004 Vortex-induced vibrations. Annu. Rev. Fluid Mech. 36, 413–455.CrossRefGoogle Scholar
33. Williamson, C. H. K. & Govardhan, R. 2008 A brief review of recent results in vortex-induced vibrations. J. Wind Engng Ind. Aerodyn. 96, 713–735.CrossRefGoogle Scholar
34. Zovatto, L. & Pedrizetti, G. 2001 Flow about a circular cylinder between parallel walls. J. Fluid Mech. 440, 1305–1320.CrossRefGoogle Scholar