Hostname: page-component-586b7cd67f-t7czq Total loading time: 0 Render date: 2024-11-26T00:56:42.108Z Has data issue: false hasContentIssue false
  • English
  • Français

New insights from inviscid modelling of starting flow separation with roll-up

Published online by Cambridge University Press:  28 September 2020

A. C. DeVoria Open the ORCID record for A. C. DeVoria [Opens in a new window]  and
K. Mohseni Open the ORCID record for K. Mohseni [Opens in a new window]
A. C. DeVoria
Affiliation:
Department of Mechanical & Aerospace Engineering, University of Florida, Gainesville, FL32611, USA
K. Mohseni*
Affiliation:
Department of Mechanical & Aerospace Engineering, University of Florida, Gainesville, FL32611, USA Department of Electrical & Computer Engineering, University of Florida, Gainesville, FL32611, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

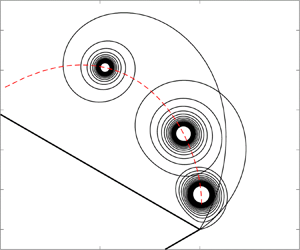
This paper investigates the use of inviscid flow theory accompanied by surfaces of discontinuity as a means of representing viscous effects. The problem studied is that of starting flow separation past a sharp wedge. We use the vortex-entrainment sheet model of DeVoria & Mohseni (J. Fluid Mech., vol. 866, 2019, pp. 660–688) to augment the set of self-similar solutions with zero entrainment as calculated by Pullin (J. Fluid Mech., vol. 88, 1978, pp. 401–430). Even under the constraints of similarity, the inclusion of entrainment allows for several new degrees of freedom, which correspond to different classes of boundary conditions, and thus solutions, that are excluded by classical potential models. Some computed examples are presented and compared to a previous experimental study. The results offer encouraging evidence that viscous or finite Reynolds number effects can, in an integrated sense, be captured by an inviscid model with singularity distributions. A normal boundary condition that contains the information pertaining to the entrainment must be supplied and represents the trade-off in surrendering the Navier–Stokes equations for the lower-order inviscid model.

JFM classification

Vortex Flows: Vortex dynamics
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 903 , 25 November 2020 , A24
Check for updates
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Batchelor, G. K. 1967 An Introduction to Fluid Dynamics. Cambridge University Press.Google Scholar
Birkhoff, G. 1962 Helmholtz and Taylor instability. In Proceedings of Symposia in Applied Mathematics, vol. 13, pp. 55–76. American Mathematical Society.Google Scholar
Brown, C. E. & Michael, W. H. 1954 Effect of leading-edge separation on the lift of a delta wing. J. Aeronaut. Sci. 21 (10), 690–694.CrossRefGoogle Scholar
DeVoria, A. C. & Mohseni, K. 2019 The vortex-entrainment sheet in an inviscid fluid: theory and separation at a sharp edge. J. Fluid Mech. 866, 660–688.CrossRefGoogle Scholar
Ellington, C. P., van den Berg, C., Willmott, A. P. & Thomas, A. L. R. 1996 Leading-edge vortices in insect flight. Nature 384 (6610), 626–630.CrossRefGoogle Scholar
Jones, M. A. 2003 The separated flow of an inviscid fluid around a moving flat plate. J. Fluid Mech. 496, 405–441.CrossRefGoogle Scholar
Krasny, R. 1987 Computation of vortex sheet roll-up in the Trefftz plane. J. Fluid Mech. 184, 123–155.CrossRefGoogle Scholar
Moore, D. W. 1979 The spontaneous appearance of a singularity in the shape of an evolving vortex sheet. Proc. R. Soc. Lond. A 365, 105–119.Google Scholar
Muskhelishvili, N. I. 1946 Singular Integral Equations, 1st edn. P. Noordhoof Ltd.Google Scholar
Pullin, D. I. 1978 The large-scale structure of unsteady self-similar rolled-up vortex sheets. J. Fluid Mech. 88 (3), 401–430.CrossRefGoogle Scholar
Pullin, D. I. & Perry, A. E. 1980 Some flow visualization experiments on the starting vortex. J. Fluid Mech. 97, 239–255.CrossRefGoogle Scholar
Rott, N. 1956 Diffraction of a weak shock with vortex generation. J. Fluid Mech. 1, 111–128.CrossRefGoogle Scholar