Hostname: page-component-745bb68f8f-mzp66 Total loading time: 0 Render date: 2025-01-08T11:23:45.431Z Has data issue: false hasContentIssue false
  • English
  • Français

Nematic–isotropic phase transition in turbulent thermal convection

Published online by Cambridge University Press:  25 November 2013

Stephan Weiss  and
Guenter Ahlers
Get access Rights & Permissions [Opens in a new window]

Abstract

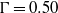We report on turbulent Rayleigh–Bénard convection of a nematic liquid crystal while it undergoes a transition from the nematic to the isotropic phase in a cylindrical convection cell with a height equal to twice the diameter (aspect ratio $\Gamma = 0. 50$). The difference between the top and bottom plate temperature $ \mathrm{\Delta} T= {T}_{b} - {T}_{t} $ was held constant, while the average temperature ${T}_{m} = ({T}_{b} + {T}_{t} )/ 2$ was varied. There was a significant increase of the transported heat when the phase transition temperature 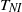${T}_{NI} $ was between 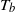${T}_{b} $ and ${T}_{t} $. Measurements of the temperatures along the sidewall of the sample as a function of 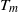${T}_{m} $ showed several ranges with qualitatively different behaviour of quantities such as the time-averaged sidewall temperature, temperature gradient, or temperature fluctuations. We interpret these different ranges in terms of properties of the thermal boundary layers close to the top and bottom plates whose stability and nature depends on the location within the sample of 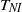${T}_{NI} $.

JFM classification

Drops and Bubbles: Drops and Bubbles Convection: Moist convection
Type
Papers
Information
Journal of Fluid Mechanics , Volume 737 , 25 December 2013 , pp. 308 - 328
Copyright
©2013 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ahlers, G. 1995 Experiments on Thermally Driven Convection. Springer.Google Scholar
Ahlers, G. 2009 Turbulent convection. Physics 2, 74.Google Scholar
Ahlers, G., Berge, L. & Cannell, D. 1993 Thermal convection in the presence of a first-order phase change. Phys. Rev. Lett. 70, 2399–2402.Google Scholar
Ahlers, G., Bodenschatz, E., Funfschilling, D., Grossmann, S., He, X., Lohse, D., Stevens, R. & Verzicco, R. 2012 Logarithmic temperature profiles in turbulent Rayleigh–Bénard convection. Phys. Rev. Lett. 109, 114501.CrossRefGoogle ScholarPubMed
Ahlers, G., Brown, E., Fontenele Araujo, F., Funfschilling, D., Grossmann, S. & Lohse, D. 2006 Non-Oberbeck–Boussinesq effects in strongly turbulent Rayleigh–Bénard convection. J. Fluid Mech. 569, 409–445.Google Scholar
Ahlers, G., Calzavarini, E., Fontenele Araujo, F., Funfschilling, D., Grossmann, S., Lohse, D. & Sugiyama, K. 2008 Non-Oberbeck-Boussinesq effects in turbulent thermal convection in ethane close to the critical point. Phys. Rev. E 77, 046302.Google Scholar
Ahlers, G., Cannell, D. S., Berge, L. I. & Sakurai, S. 1994 Thermal conductivity of the nematic liquid crystal 4- $n$ -pentyl- ${4}^{\prime } $ -cyanobiphenyl. Phys. Rev. E 49, 545–553.Google Scholar
Ahlers, G., Fontenele Araujo, F., Funfschilling, D., Grossmann, S. & Lohse, D. 2007 Non-Oberbeck–Boussinesq effects in gaseous Rayleigh–Bénard convection. Phys. Rev. Lett. 98, 054501.Google Scholar
Ahlers, G., Grossmann, S. & Lohse, D. 2009 Heat transfer and large scale dynamics in turbulent Rayleigh-Bénard convection. Rev. Mod. Phys. 81, 503–538.Google Scholar
Auernhammer, G., Vollmer, D. & Vollmer, J. 2005 Oscillatory instabilities in phase separation of binary mixtures: fixing the thermodynamic driving. J. Chem. Phys. 123 (13), 134511.Google Scholar
Bezrodna, T., Chashechnikova, I., Gavrilko, T., Puchkovska, G., Shaydyuk, Y., Tolochko, A., Baran, J. & Drozd, M. 2008 Structure formation and its influence on thermodynamic and optical properties of montmorillonite organoclay-5CB liquid crystal nanocomposites. Liquid Cryst. 35, 265–274.Google Scholar
Blasius, H. 1908 Grenzschichten in Flüssigkeiten mit kleiner Reibung. Z. Math. Phys. 56, 1–37.Google Scholar
Boussinesq, J. 1903 Theorie Analytique de la Chaleur, vol. 2, Gauthier-Villars.Google Scholar
Brent, A. D., Voller, V. R. & Reid, K. J. 1988 Enthalpy–porosity technique for modelling convection–diffusion phase change: application to the melting of a pure metal. Numer. Heat Transfer 13, 297–318.Google Scholar
Brown, E. & Ahlers, G. 2007 Temperature gradients, and search for non-Boussinesq effects, in the interior of turbulent Rayleigh-Bénard convection. Europhys. Lett. 80, 14001.Google Scholar
Brown, E., Nikolaenko, A. & Ahlers, G. 2005 Reorientation of the large scale circulation in turbulent Rayleigh–Bénard convection. Phys. Rev. Lett. 95, 084503.Google Scholar
Busse, F. H. & Schubert, G. 1971 Convection in a fluid with two phases. J. Fluid Mech. 46 (4), 801–812.CrossRefGoogle Scholar
Chillà, F. & Schumacher, J. 2012 New perspectives in turbulent Rayleigh–Bénard convection. Eur. Phys. J. E 35, 58.Google Scholar
Christensen, U. 1995 Effects of phase transitions on mantle convection. Annu. Rev. Earth Planet. Sci. 23, 65–87.Google Scholar
Dhir, V. K. 1998 Boiling heat transfer. Annu. Rev. Fluid Mech. 30, 365–401.Google Scholar
Funfschilling, D., Brown, E. & Ahlers, G. 2008 Torsional oscillations of the large scale circulation in turbulent Rayleigh–Bénard convection. J. Fluid Mech. 607, 119–139.Google Scholar
de Gennes, P. & Prost, J. 1995 The Physics of Liquid Crystals. Oxford University Press.Google Scholar
Gunton, J. D., Miguel, M. S. & Sahni, P. S. 1983 The dynamics of first-order phase transitions. In Phase Transitions and Critical Phenomena (ed. Domb, C. & Lebowitz, J. L.), p. 267. Academic Press.Google Scholar
Hartmann, D. L., Moy, L. A. & Fu, Q. 2001 Tropical convection and the energy balance at the top of the atmosphere. J. Clim. 14, 4495–4511.Google Scholar
Iezzi, R., Francolino, S. & Mucchetti, G. 2011 Natural convective cooling of cheese: predictive model and validation of heat exchange simulation. J. Food Engng 106 (1), 88–94.Google Scholar
Jacobs, M. H. & van den Berg, A. P. 2011 Complex phase distribution and seismic velocity structure of the transition zone: convection model predictions for a magnesium-endmember olivine–pyroxene mantle. Phys. Earth Planet. Inter. 186 (1/2), 36–48.Google Scholar
Kadanoff, L. P. 2001 Turbulent heat flow: structures and scaling. Phys. Today 54 (8), 34–39.Google Scholar
Kerr, R. 1992 Having it both ways in the mantle. Science 258 (5088), 1576–1578.Google Scholar
Khoo, I.-C. 2007 Liquid Crystals. Wiley.Google Scholar
Kramer, L. & Pesch, W. 1995 Convection instabilities in nematic liquid crystals. Annu. Rev. Fluid Mech. 27, 515–539.Google Scholar
Kühn, M., Bosbach, J. & Wagner, C. 2009 Experimental parametric study of forced and mixed convection in a passenger aircraft cabin mock-up. Build. Environ. 44 (5), 961–970.Google Scholar
Lohse, D. & Xia, K.-Q. 2010 Small-scale properties of turbulent Rayleigh–Bénard convection. Annu. Rev. Fluid Mech. 42, 335–364.Google Scholar
Lui, S. L. & Xia, K.-Q. 1998 Spatial structure of the thermal boundary layer in turbulent convection. Phys. Rev. E 57, 5494–5503.Google Scholar
Malkus, M. V. R. 1954 The heat transport and spectrum of thermal turbulence. Proc. R. Soc. Lond. A 225, 196–212.Google Scholar
Marshall, J. & Schott, F. 1999 Open-ocean convection: observations, theory, and models. Rev. Geophys. 37, 1–64.Google Scholar
Oberbeck, A. 1879 Über die Wärmeleitung der Flüssigkeiten bei Berücksichtigung der Strömungen infolge von Temperaturdifferenzen. Ann. Phys. Chem. 7, 271–292.CrossRefGoogle Scholar
Pauluis, O. & Schumacher, J. 2011 Self-aggregation of clouds in conditionally unstable moist convection. Proc. Natl. Acad. Sci. USA 108, 12623–12628.Google Scholar
Prandtl, L. 1905 Über Flüssigkeitsbewegung bei sehr kleiner Reibung, pp. 484–491 Teubner.Google Scholar
du Puits, R., Resagk, C. & Thess, A. 2013 Thermal boundary layers in turbulent Rayleigh–Bénard convection at aspect ratios between 1 and 9. New J. Phys. 15, 013040.Google Scholar
Rahmstorf, S. 2000 The thermohaline ocean circulation: a system with dangerous thresholds? Climate Change 46, 247–256.Google Scholar
van Roie, B., Leys, J., Denolf, K., Glorieux, C., Pitsi, G. & Thoen, J. 2005 Weakly first-order character of the nematic–isotropic phase transition in liquid crystals. Phys. Rev. E 72, 041702–1–8.Google Scholar
Sakurai, S., Tschammer, A., Pesch, W. & Ahlers, G. 1999 Convection in the presence of a first-order phase change. Phys. Rev. E 60, 539–550.Google Scholar
Schlichting, H. & Gersten, K. 2000 Boundary Layer Theory, 8th edn. Springer.Google Scholar
Schubert, G. 1992 Numerical models of mantle convection. Annu. Rev. Fluid Mech. 24, 359–394.Google Scholar
Shim, S., Duffy, T. & Shen, G. 2001 The post-spinel transformation in ${\mathrm{Mg} }_{2} {\mathrm{SIO} }_{4} $ and its relation to the 660 km discontinuity. Nature 411, 571–574.Google Scholar
Stevens, B. 2005 Atmospheric moist convection. Annu. Rev. Earth Planet. Sci. 33, 605–643.Google Scholar
Thoen, J. 1992 Calorimetric studies of liquid crystal phase transitions: steady state adiabatic techniques. In Phase Transition in Liquid Crystals. Plenum.Google Scholar
Tilgner, A., Belmonte, A. & Libchaber, A. 1993 Temperature and velocity profiles of turbulence convection in water. Phys. Rev. E 47, R2253–R2256.Google Scholar
Tong, L. & Tang, Y. 1997 Boiling Heat Transfer and Two-Phase Flow. Taylor & Francis.Google Scholar
Verhoogen, J. 1965 Phase changes and convection in the earth’s mantle. Phil. Trans. R. Soc. Lond. A 258 (1088), 276–283.Google Scholar
Weidauer, T., Pauluis, O. & Schumacher, J. 2010 Cloud patterns and mixing properties in shallow moist Rayleigh–Bénard convection. New J. Phys. 12, 105002.Google Scholar
Weinstein, S. A. 1993 Catastrophic overturn of the mantle driven by multiple phase changes and internal heat generation. Geophys. Res. Lett. 20, 101.Google Scholar
Weiss, S. & Ahlers, G. 2011 Turbulent Rayleigh–Bénard convection in a cylindrical container with aspect ratio $\Gamma = 0. 50$ and Prandtl number $Pr= 4. 38$ . J. Fluid Mech. 676, 5–40.Google Scholar
Weiss, S. & Ahlers, G. 2013 Magnetic-field effect on turbulent thermal convection of a nematic liquid crystal. J. Fluid Mech. 716, R7.Google Scholar
Zerban, A. H. & Nye, E. P. 1956 Power Plants, 2nd edn. International Textbook Company.Google Scholar
Zhong, J.-Q., Funfschilling, D. & Ahlers, G. 2009 Enhanced heat transport by turbulent two-phase Rayleigh–Bénard convection. Phys. Rev. Lett. 102, 124501.Google Scholar