Hostname: page-component-586b7cd67f-gb8f7 Total loading time: 0 Render date: 2024-11-29T16:19:33.378Z Has data issue: false hasContentIssue false
  • English
  • Français

Mutual inductance instability of the tip vortices behind a wind turbine

Published online by Cambridge University Press:  26 August 2014

Sasan Sarmast ,
Reza Dadfar ,
Robert F. Mikkelsen ,
Philipp Schlatter ,
Stefan Ivanell ,
Jens N. Sørensen  and
Dan S. Henningson
Sasan Sarmast
Affiliation:
Swedish e-Science Research Centre (SeRC), Linné FLOW Centre, KTH Mechanics, Royal Institute of Technology, SE-100 44 Stockholm, Sweden DTU Wind Energy, Denmark Technical University, 2800 Kgs. Lyngby, Denmark
Reza Dadfar
Affiliation:
Swedish e-Science Research Centre (SeRC), Linné FLOW Centre, KTH Mechanics, Royal Institute of Technology, SE-100 44 Stockholm, Sweden
Robert F. Mikkelsen
Affiliation:
DTU Wind Energy, Denmark Technical University, 2800 Kgs. Lyngby, Denmark
Philipp Schlatter
Affiliation:
Swedish e-Science Research Centre (SeRC), Linné FLOW Centre, KTH Mechanics, Royal Institute of Technology, SE-100 44 Stockholm, Sweden
Stefan Ivanell
Affiliation:
Swedish e-Science Research Centre (SeRC), Linné FLOW Centre, KTH Mechanics, Royal Institute of Technology, SE-100 44 Stockholm, Sweden Wind Energy Campus Gotland, Department of Earth Sciences, Uppsala University, 621 67 Visby, Sweden
Jens N. Sørensen
Affiliation:
DTU Wind Energy, Denmark Technical University, 2800 Kgs. Lyngby, Denmark
Dan S. Henningson*
Affiliation:
Swedish e-Science Research Centre (SeRC), Linné FLOW Centre, KTH Mechanics, Royal Institute of Technology, SE-100 44 Stockholm, Sweden
*
†Email address for correspondence: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Two modal decomposition techniques are employed to analyse the stability of wind turbine wakes. A numerical study on a single wind turbine wake is carried out focusing on the instability onset of the trailing tip vortices shed from the turbine blades. The numerical model is based on large-eddy simulations (LES) of the Navier–Stokes equations using the actuator line (ACL) method to simulate the wake behind the Tjæreborg wind turbine. The wake is perturbed by low-amplitude excitation sources located in the neighbourhood of the tip spirals. The amplification of the waves travelling along the spiral triggers instabilities, leading to breakdown of the wake. Based on the grid configurations and the type of excitations, two basic flow cases, symmetric and asymmetric, are identified. In the symmetric setup, we impose a 120° symmetry condition in the dynamics of the flow and in the asymmetric setup we calculate the full 360° wake. Different cases are subsequently analysed using dynamic mode decomposition (DMD) and proper orthogonal decomposition (POD). The results reveal that the main instability mechanism is dispersive and that the modal growth in the symmetric setup arises only for some specific frequencies and spatial structures, e.g. two dominant groups of modes with positive growth (spatial structures) are identified, while breaking the symmetry reveals that almost all the modes have positive growth rate. In both setups, the most unstable modes have a non-dimensional spatial growth rate close to 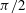$\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}\pi /2$ and they are characterized by an out-of-phase displacement of successive helix turns leading to local vortex pairing. The present results indicate that the asymmetric case is crucial to study, as the stability characteristics of the flow change significantly compared to the symmetric configurations. Based on the constant non-dimensional growth rate of disturbances, we derive a new analytical relationship between the length of the wake up to the turbulent breakdown and the operating conditions of a wind turbine.

JFM classification

Instability: Instability Wakes/Jets: Wakes
Type
Papers
Information
Journal of Fluid Mechanics , Volume 755 , 25 September 2014 , pp. 705 - 731
Creative Commons
Creative Common License - CCCreative Common License - BY
The online version of this article is published within an Open Access environment subject to the conditions of the Creative Commons Attribution licence .
Copyright
© 2014 Cambridge University Press

References

Alfredsson, P. H. & Dahlberg, J. A.1979 A preliminary wind tunnel study of windmill wake dispersion in various flow conditions. Tech. Note AU-1499, Part 7.Google Scholar
Aubry, N. 1991 On the hidden beauty of the proper orthogonal decomposition. Theor. Comput. Fluid Dyn. 2 (5), 339–352.Google Scholar
Bhagwat, M. J. & Leishman, J. G. 2001 Stability, consistency and convergence of numerical algorithms for time-marching free-vortex wake analysis. J. Am. Helicopter Soc. 46 (1), 59–70.CrossRefGoogle Scholar
Chen, K. K., Tu, J. H. & Rowley, C. W. 2012 Variants of dynamic mode decomposition: boundary condition, Koopman, and Fourier analyses. J. Nonlinear Sci. 22 (6), 887–915.Google Scholar
Chevalier, M., Schlatter, P., Lundbladh, A. & Henningson, D. S.2007 SIMSON: a pseudo-spectral solver for incompressible boundary layer flows. Tech. Rep. TRITA-MEK 2007:07. Royal Institute of Technology, Stockholm, Sweden.Google Scholar
Felli, M., Camussi, R. & Di Felice, F. 2011 Mechanisms of evolution of the propeller wake in the transition and far fields. J. Fluid Mech. 682, 5–53.Google Scholar
Frederich, O. & Luchtenburg, D. M.2011 Modal analysis of complex turbulent flow. In 7th Intl Symp. on Turbulence and Shear Flow Phenomena (TSFP-7), Ottawa, Canada.Google Scholar
Gupta, B. P. & Loewy, R. G. 1974 Theoretical analysis of the aerodynamic stability of multiple, interdigitated helical vortices. AIAA J. 12 (10), 1381–1387.CrossRefGoogle Scholar
Ivanell, S., Mikkelsen, R., Sørensen, J. N. & Henningson, D. S. 2010 Stability analysis of the tip vortices of a wind turbine. Wind Energy 13 (8), 705–715.CrossRefGoogle Scholar
Jain, R., Conlisk, A. T., Mahalingam, R. & Komerath, N. M.1998 Interaction of tip-vortices in the wake of a two-bladed rotor. In 54th Annual Forum of the Am. Helicopter Soc., Washington, DC.Google Scholar
Joukowski, N. E. 1912 Vortex theory of screw propeller. Trudy Otdeleniya Fizicheskikh Nauk Obshchestva Lubitelei Estestvoznaniya 16 (1), 1–31.Google Scholar
Lamb, H. 1932 Hydrodynamics, 6th edn Cambridge University Press.Google Scholar
Leishman, G., Bhagwat, M. J. & Ananthan, S. 2004 The vortex ring state as a spatially and temporally developing wake instability. J. Am. Helicopter Soc. 49 (2), 160–175.Google Scholar
Levy, H. & Forsdyke, A. G. 1927 The stability of an infinite system of circular vortices. Proceedings R. Soc. Lond. A 114 (768), 594–604.Google Scholar
Levy, H. & Forsdyke, A. G. 1928 The steady motion and stability of a helical vortex. Proc. R. Soc. Lond. A 120 (786), 670–690.Google Scholar
Leweke, T., Bolnot, H., Quaranta, U. & Dizès, S. Le.2013 Local and global pairing in helical vortex systems. Intl Conf. on Aerodynamics of Offshore Wind Energy Systems and Wakes, Lyngby, Denmark.Google Scholar
Lumley, J. L. 1970 Stochastic Tools in Turbulence. Academic.Google Scholar
Manhart, M. & Wengle, H. 1993 A spatiotemporal decomposition of a fully inhomogeneous turbulent flow field. Theor. Comput. Fluid Dyn. 5 (4), 223–242.Google Scholar
Michelsen, J. A.1994 Block structured multigrid solution of 2D and 3D elliptic PDE’s. Tech. Rep. AFM 94-06. Dept. of Fluid Mech., Technical University of Denmark, DTU.Google Scholar
Mikkelsen, R. F.2003 Actuator disc methods applied to wind turbines. PhD thesis, Dept. of Fluid Mech., Technical University of Denmark, DTU.Google Scholar
Okulov, V. L. & Sørensen, J. N. 2007 Stability of helical tip vortices in a rotor far wake. J. Fluid Mech. 576, 1–25.Google Scholar
Øye, S.1991 Tjæreborg wind turbine: 4. dynamic flow measurement. AFM Notat VK-204.Google Scholar
Prony, R. 1795 Essai expérimental et analytique. J. l’Ecole Polytech. 1 (2), 24–76.Google Scholar
Rao, A. R., Hamed, K. H. & Chen, H. L. 2003 Nonstationarities in Hydrologic and Environmental Time Series, Water Sci. and Tech. Library, vol. 45. Springer.Google Scholar
Rempfer, D. & Fasel, H. F. 1994 Evolution of three-dimensional coherent structures in a flat-plate boundary layer. J. Fluid Mech. 260, 351–375.CrossRefGoogle Scholar
Rowley, C. W., Mezić, I., Bagheri, S., Schlatter, P. & Henningson, D. S. 2009 Spectral analysis of nonlinear flows. J. Fluid Mech. 641, 115–127.Google Scholar
Schlatter, P. & Örlü, R. 2012 Turbulent boundary layers at moderate Reynolds numbers. Inflow length and tripping effects. J. Fluid Mech. 710, 5–34.CrossRefGoogle Scholar
Schmid, P. J. 2010 Dynamic mode decomposition of numerical and experimental data. J. Fluid Mech. 656, 5–28.Google Scholar
Sirovich, L. 1987 Turbulence and the dynamics of coherent structures. I-coherent structures. II-symmetries and transformations. III-dynamics and scaling. Q. Appl. Maths 45 (1), 561–571.Google Scholar
Smith, G., Schlez, W., Liddell, A., Neubert, A. & Peña, A.2006 Advanced wake model for very closely spaced turbines. In Conf. Procs. EWEC Athens.Google Scholar
Sørensen, N. N.1995 General purpose flow solver applied to flow over hills. PhD thesis, Risø National Laboratory, Roskilde.Google Scholar
Sørensen, J. N. 2011 Instability of helical tip vortices in rotor wakes. J. Fluid Mech. 682, 1–4.Google Scholar
Sørensen, J. N. & van Kuik, G. A. M. 2011 General momentum theory for wind turbines at low tip speed ratios. Wind Energy 14 (7), 821–839.CrossRefGoogle Scholar
Sørensen, J. N. & Shen, W. Z. 2002 Numerical modeling of wind turbine wakes. Trans. ASME: J. Fluids Engng 124 (2), 393–399.Google Scholar
Sørensen, J. N., Shen, W. Z. & Munduate, X. 1998 Analysis of wake states by a full-field actuator disc model. Wind Energy 1 (2), 73–88.Google Scholar
Tangler, J. L., Wohlfeld, R. M. & Miley, S. J.1973 An experimental investigation of vortex stability, tip shapes, compressibility, and noise for hovering model rotors. NASA 2305.Google Scholar
Ta Phuoc, L.1994 Modèles de sous maille appliqués aux ecoulements instationnaires décollés. In Proceeding of the DRET Conference: Aérodynamique Instationnaire Turbulents – Aspects Numériques et Expérimentaux.Google Scholar
Troldborg, N.2008 Actuator line modeling of wind turbine wakes. PhD thesis, Dept of Fluid Mechanics, Technical University of Denmark, DTU.Google Scholar
Walther, J. H., Guenot, M., Machefaux, E., Rasmussen, J. T., Chatelain, P., Okulov, V. L., Sørensen, J. N., Bergdorf, M. & Koumoutsakos, P. 2007 A numerical study of the stabilitiy of helical vortices using vortex methods. J. Phys.: Conf. Ser. 75 (1), 012034.Google Scholar
Welch, P. 1967 The use of fast Fourier transform for the estimation of power spectra: a method based on time averaging over short, modified periodograms. IEEE Trans. Audio Electroacoust. 15 (2), 70–73.CrossRefGoogle Scholar
Widnall, S. E. 1972 The stability of a helical vortex filament. J. Fluid Mech. 4, 641–663.CrossRefGoogle Scholar