Hostname: page-component-586b7cd67f-t7fkt Total loading time: 0 Render date: 2024-11-23T03:16:37.477Z Has data issue: false hasContentIssue false
  • English
  • Français

Model-based design of transverse wall oscillations for turbulent drag reduction

Published online by Cambridge University Press:  02 August 2012

Rashad Moarref  and
Mihailo R. Jovanović
Rashad Moarref
Affiliation:
Department of Electrical and Computer Engineering, University of Minnesota, Minneapolis, MN 55455, USA
Mihailo R. Jovanović*
Affiliation:
Department of Electrical and Computer Engineering, University of Minnesota, Minneapolis, MN 55455, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

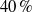Over the last two decades, both experiments and simulations have demonstrated that transverse wall oscillations with properly selected amplitude and frequency can reduce turbulent drag by as much as . In this paper, we develop a model-based approach for designing oscillations that suppress turbulence in a channel flow. We utilize eddy-viscosity-enhanced linearization of the turbulent flow with control in conjunction with turbulence modelling to determine skin-friction drag in a simulation-free manner. The Boussinesq eddy viscosity hypothesis is used to quantify the effect of fluctuations on the mean velocity in flow subject to control. In contrast to the traditional approach that relies on numerical simulations, we determine the turbulent viscosity from the second-order statistics of the linearized model driven by white-in-time stochastic forcing. The spatial power spectrum of the forcing is selected to ensure that the linearized model for uncontrolled flow reproduces the turbulent energy spectrum. The resulting correction to the turbulent mean velocity induced by small-amplitude wall movements is then used to identify the optimal frequency of drag-reducing oscillations. In addition, the control net efficiency and the turbulent flow structures that we obtain agree well with the results of numerical simulations and experiments. This demonstrates the predictive power of our model-based approach to controlling turbulent flows and is expected to pave the way for successful flow control at higher Reynolds numbers than currently possible.

JFM classification

Flow Control: Drag reduction Turbulent Flows: Turbulence control Turbulent Flows: Turbulence modelling
Type
Papers
Information
Journal of Fluid Mechanics , Volume 707 , 25 September 2012 , pp. 205 - 240
Copyright
Copyright © Cambridge University Press 2012

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Auteri, F., Baron, A., Belan, M., Campanardi, G. & Quadrio, M. 2010 Experimental assessment of drag reduction by travelling waves in a turbulent pipe flow. Phys. Fluids 22, 115103.CrossRefGoogle Scholar
2. Bamieh, B. & Dahleh, M. 2001 Energy amplification in channel flows with stochastic excitation. Phys. Fluids 13 (11), 3258–3269.CrossRefGoogle Scholar
3. Bandyopadhyay, P. R. 2006 Stokes mechanism of drag reduction. J. Appl. Mech. 73, 483.CrossRefGoogle Scholar
4. Baron, A. & Quadrio, M. 1996 Turbulent drag reduction by spanwise wall oscillations. Appl. Sci. Res. 55 (4), 311–326.CrossRefGoogle Scholar
5. Bensoussan, A., Lions, J. L. & Papanicolaou, G. 1978 Asymptotic Analysis for Periodic Structures. North-Holland.Google Scholar
6. Berger, T. W., Kim, J., Lee, C. & Lim, J. 2000 Turbulent boundary layer control utilizing the Lorentz force. Phys. Fluids 12 (3), 631–649.CrossRefGoogle Scholar
7. Bradshaw, P. & Pontikos, N. S. 1985 Measurements in the turbulent boundary layer on an infinite swept wing. J. Fluid Mech. 159, 105–130.CrossRefGoogle Scholar
8. Cess, R. D. 1958 A survey of the literature on heat transfer in turbulent tube flow. Westinghouse Research Rep. 8-0529-R24.Google Scholar
9. Chakraborty, P., Balachandar, S. & Adrian, R. J. 2005 On the relationships between local vortex identification schemes. J. Fluid Mech. 535, 189–214.CrossRefGoogle Scholar
10. Chernyshenko, S. I. & Baig, M. F. 2005 The mechanism of streak formation in near-wall turbulence. J. Fluid Mech. 544, 99–131.CrossRefGoogle Scholar
11. Choi, J. I., Xu, C. X. & Sung, H. J. 2002 Drag reduction by spanwise wall oscillation in wall-bounded turbulent flows. AIAA J. 40 (5), 842–850.CrossRefGoogle Scholar
12. Choi, K.-S. 2002 Near-wall structure of turbulent boundary layer with spanwise-wall oscillation. Phys. Fluids 14 (7), 2530–2542.CrossRefGoogle Scholar
13. Choi, K.-S., DeBisschop, J.-R. & Clayton, B. R. 1998 Turbulent boundary-layer control by means of spanwise-wall oscillation. AIAA J. 36 (7), 1157–1163.CrossRefGoogle Scholar
14. Cossu, C., Pujals, G. & Depardon, S. 2009 Optimal transient growth and very large-scale structures in turbulent boundary layers. J. Fluid Mech. 619, 79–94.CrossRefGoogle Scholar
15. Currie, I. G. 2003 Fundamental Mechanics of Fluids. CRC.Google Scholar
16. del Álamo, J. C. & Jiménez, J. 2003 Spectra of the very large anisotropic scales in turbulent channels. Phys. Fluids 15 (6), 41–44.CrossRefGoogle Scholar
17. del Álamo, J. C. & Jiménez, J. 2006 Linear energy amplification in turbulent channels. J. Fluid Mech. 559, 205–213.CrossRefGoogle Scholar
18. del Álamo, J. C., Jiménez, J., Zandonade, P. & Moser, R. D. 2004 Scaling of the energy spectra of turbulent channels. J. Fluid Mech. 500, 135–144.CrossRefGoogle Scholar
19. Dhanak, M. & Si, C. 1999 On reduction of turbulent wall friction through spanwise wall oscillations. J. Fluid Mech. 383, 175–195.CrossRefGoogle Scholar
20. Du, Y. & Karniadakis, G. E. 2000 Suppressing wall turbulence by means of a transverse travelling wave. Science 288, 1230–1234.CrossRefGoogle Scholar
21. Du, Y., Symeonidis, V. & Karniadakis, G. E. 2002 Drag reduction in wall-bounded turbulence via a transverse travelling wave. J. Fluid Mech. 457, 1–34.CrossRefGoogle Scholar
22. Durbin, P. A. & Reif, B. A. P. 2000 Theory and Modelling of Turbulent Flows. Wiley.Google Scholar
23. Farrell, B. F. & Ioannou, P. J. 1993 Stochastic forcing of the linearized Navier–Stokes equations. Phys. Fluids A 5 (11), 2600–2609.CrossRefGoogle Scholar
24. Gad-el-Hak, M. 2000 Flow Control: Passive, Active, and Reactive Flow Management. Cambridge University Press.CrossRefGoogle Scholar
25. Gardner, W. A. 1990 Introduction to Random Processes: with Applications to Signals and Systems. McGraw-Hill.Google Scholar
26. Hœpffner, J., Brandt, L. & Henningson, D. 2005 Transient growth on boundary layer streaks. J. Fluid Mech. 537, 91–100.CrossRefGoogle Scholar
27. Högberg, M., Bewley, T. R. & Henningson, D. S. 2003 Linear feedback control and estimation of transition in plane channel flow. J. Fluid Mech. 481, 149–175.CrossRefGoogle Scholar
28. Högberg, M., Bewley, T. R. & Henningson, D. S. 2003 Relaminarization of turbulence using gain scheduling and linear state-feedback control. Phys. Fluids 15 (11), 3572–3575.CrossRefGoogle Scholar
29. Jones, W. P. & Launder, B. E. 1972 The prediction of laminarization with a two-equation model of turbulence. Intl J. Heat Mass Transfer. 15 (2), 301–314.CrossRefGoogle Scholar
30. Joslin, R. D. 1998 Aircraft laminar flow control. Annu. Rev. Fluid Mech. 30, 1–29.CrossRefGoogle Scholar
31. Jovanović, M. R. 2004Modelling, analysis, and control of spatially distributed systems. PhD thesis, University of California, Santa Barbara.Google Scholar
32. Jovanović, M. R. 2008 Turbulence suppression in channel flows by small amplitude transverse wall oscillations. Phys. Fluids 20 (1), 014101.CrossRefGoogle Scholar
33. Jovanović, M. R. & Bamieh, B. 2005 Componentwise energy amplification in channel flows. J. Fluid Mech. 534, 145–183.CrossRefGoogle Scholar
34. Jovanović, M. R. & Fardad, M. 2008 norm of linear time-periodic systems: a perturbation analysis. Automatica 44 (8), 2090–2098.CrossRefGoogle Scholar
35. Jovanović, M. R. & Georgiou, T. T. 2010 Reproducing second-order statistics of turbulent flows using linearized Navier–Stokes equations with forcing. Bull. Am. Phys. Soc. 55.Google Scholar
36. Jung, W., Mangiavacchi, N. & Akhavan, R. 1992 Suppression of turbulence in wall-bounded flows by high-frequency spanwise oscillations. Phys. Fluids A 4 (8), 1605–1607.CrossRefGoogle Scholar
37. Karniadakis, G. & Choi, K.-S. 2003 Mechanisms on transverse motions in turbulent wall flows. Annu. Rev. Fluid Mech. 35, 45–62.CrossRefGoogle Scholar
38. Kim, J. & Bewley, T. R. 2007 A linear systems approach to flow control. Annu. Rev. Fluid Mech. 39, 383–417.CrossRefGoogle Scholar
39. Kim, J. & Lim, J. 2000 A linear process in wall-bounded turbulent shear flows. Phys. Fluids 12 (8), 1885–1888.CrossRefGoogle Scholar
40. Kim, J., Moin, P. & Moser, R. 1987 Turbulence statistics in fully developed channel flow at low Reynolds number. J. Fluid Mech. 177, 133–166.CrossRefGoogle Scholar
41. Laadhari, F., Skandaji, L. & Morel, R. 1994 Turbulence reduction in a boundary layer by a local spanwise oscillating surface. Phys. Fluids 6 (10), 3218–3220.CrossRefGoogle Scholar
42. Launder, B. E. & Sharma, B. I. 1974 Application of the energy-dissipation model of turbulence to the calculation of flow near a spinning disc. Lett. Heat Mass Transfer. 1, 131–137.CrossRefGoogle Scholar
43. Lee, M., Kim, J. & Moin, P. 1990 Structure of turbulence at high shear rate. J. Fluid Mech. 216, 561–583.CrossRefGoogle Scholar
44. Lieu, B., Moarref, R. & Jovanović, M. R. 2010 Controlling the onset of turbulence by streamwise travelling waves. Part 2. Direct numerical simulations. J. Fluid Mech. 663, 100–119.CrossRefGoogle Scholar
45. Luchini, P., Quadrio, M. & Zuccher, S. 2006 The phase-locked mean impulse response of a turbulent channel flow. Phys. Fluids 18, 121702.CrossRefGoogle Scholar
46. Malkus, W. V. R. 1956 Outline of a theory of turbulent shear flow. J. Fluid Mech. 1 (5), 521–539.CrossRefGoogle Scholar
47. McComb, W. D. 1991 The Physics of Fluid Turbulence. Oxford University Press.Google Scholar
48. Moarref, R. & Jovanović, M. R. 2010 Controlling the onset of turbulence by streamwise travelling waves. Part 1. Receptivity analysis. J. Fluid Mech. 663, 70–99.CrossRefGoogle Scholar
49. Moin, P. & Moser, R. 1989 Characteristic-eddy decomposition of turbulence in a channel. J. Fluid Mech. 200, 471–509.CrossRefGoogle Scholar
50. Moin, P., Shih, T. H., Driver, D. & Mansour, N. N. 1990 Direct numerical simulation of a three-dimensional turbulent boundary layer. Phys. Fluids A 2 (10), 1846–1853.CrossRefGoogle Scholar
51. Moser, R. D., Kim, J. & Mansour, N. N. 1999 DNS of turbulent channel flow up to . Phys. Fluids 11 (4), 943–945.CrossRefGoogle Scholar
52. Odeh, F. & Keller, J. B. 1964 Partial differential equations with periodic coefficients and Bloch waves in crystals. J. Math. Phys. 5, 1499–1504.CrossRefGoogle Scholar
53. Panton, R. L. 1996 Incompressible Flows. Wiley.CrossRefGoogle Scholar
54. Pope, S. B. 2000 Turbulent Flows. Cambridge University Press.CrossRefGoogle Scholar
55. Pujals, G., García-Villalba, M., Cossu, C. & Depardon, S. 2009 A note on optimal transient growth in turbulent channel flows. Phys. Fluids 21 (1), 015109.CrossRefGoogle Scholar
56. Quadrio, M. 1940 Drag reduction in turbulent boundary layers by in-plane wall motion. Phil. Trans. R. Soc. A 369, 1428–1442.CrossRefGoogle Scholar
57. Quadrio, M. & Ricco, P. 2003 Initial response of a turbulent channel flow to spanwise oscillation of the walls. J. Turbul. (4), 007.Google Scholar
58. Quadrio, M. & Ricco, P. 2004 Critical assessment of turbulent drag reduction through spanwise wall oscillations. J. Fluid Mech. 521, 251–271.CrossRefGoogle Scholar
59. Quadrio, M. & Ricco, P. 2011 The laminar generalized Stokes layer and turbulent drag reduction. J. Fluid Mech. 667, 135–157.CrossRefGoogle Scholar
60. Quadrio, M., Ricco, P. & Viotti, C. 2009 Streamwise-travelling waves of spanwise wall velocity for turbulent drag reduction. J. Fluid Mech. 627, 161–178.CrossRefGoogle Scholar
61. Quadrio, M. & Sibilla, S. 2000 Numerical simulation of turbulent flow in a pipe oscillating around its axis. J. Fluid Mech. 424, 217–241.CrossRefGoogle Scholar
62. Reynolds, W. C. & Hussain, A. K. M. F. 1972 The mechanics of an organized wave in turbulent shear flow. Part 3. Theoretical models and comparisons with experiments. J. Fluid Mech. 54 (2), 263–288.CrossRefGoogle Scholar
63. Reynolds, W. C. & Tiederman, W. G. 1967 Stability of turbulent channel flow with application to Malkus’s theory. J. Fluid Mech. 27 (2), 253–272.CrossRefGoogle Scholar
64. Ricco, P. 2004 Modification of near-wall turbulence due to spanwise wall oscillations. J. Turbul. (5).CrossRefGoogle Scholar
65. Ricco, P. 2011 Laminar streaks with spanwise wall forcing. Phys. Fluids 23, 064103.CrossRefGoogle Scholar
66. Ricco, P., Ottonelli, C., Hasegawa, Y. & Quadrio, M. 2012 Changes in turbulent dissipation in a channel flow with oscillating walls. J. Fluid Mech. 700, 77–104.CrossRefGoogle Scholar
67. Ricco, P. & Quadrio, M. 2008 Wall-oscillation conditions for drag reduction in turbulent channel flow. Intl J. Heat Mass Transfer 29 (4), 891–902.Google Scholar
68. Ricco, P. & Wu, S. 2004 On the effects of lateral wall oscillations on a turbulent boundary layer. Exp. Therm. Fluid Sci. 29 (1), 41–52.CrossRefGoogle Scholar
69. Schmid, P. J. 2007 Nonmodal stability theory. Annu. Rev. Fluid Mech. 39, 129–162.CrossRefGoogle Scholar
70. Schoppa, W. & Hussain, F. 2002 Coherent structure generation in near-wall turbulence. J. Fluid Mech. 453, 57–108.CrossRefGoogle Scholar
71. Touber, E. & Leschziner, M. A. 2012 Near-wall streak modification by spanwise oscillatory wall motion and drag-reduction mechanisms. J. Fluid Mech. 693, 150–200.CrossRefGoogle Scholar
72. Townsend, A. 1956 The Structure of Turbulent Shear Flow. Cambridge University Press.Google Scholar
73. Trefethen, L. N., Trefethen, A. E., Reddy, S. C. & Driscoll, T. A. 1993 Hydrodynamic stability without eigenvalues. Science 261, 578–584.CrossRefGoogle ScholarPubMed
74. Weideman, J. A. C. & Reddy, S. C. 2000 A MATLAB differentiation matrix suite. ACM Trans. Math. Softw. 26 (4), 465–519.CrossRefGoogle Scholar