Hostname: page-component-78c5997874-4rdpn Total loading time: 0 Render date: 2024-11-19T04:15:27.912Z Has data issue: false hasContentIssue false
  • English
  • Français

Matrix-free continuation of limit cycles and their bifurcations for a ducted premixed flame

Published online by Cambridge University Press:  17 October 2014

Iain C. Waugh ,
K. Kashinath  and
Matthew P. Juniper
Iain C. Waugh*
Affiliation:
Cambridge University, Engineering Department, Trumpington Street, Cambridge CB2 1PZ, UK
K. Kashinath
Affiliation:
Cambridge University, Engineering Department, Trumpington Street, Cambridge CB2 1PZ, UK
Matthew P. Juniper
Affiliation:
Cambridge University, Engineering Department, Trumpington Street, Cambridge CB2 1PZ, UK
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

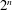Many experimental studies have demonstrated that ducted premixed flames exhibit stable limit cycles in some regions of parameter space. Recent experiments have also shown that these (period-1) limit cycles subsequently bifurcate to period-$2^{n}$, quasiperiodic, multiperiodic or chaotic behaviour. These secondary bifurcations cannot be found computationally using most existing frequency domain methods, because these methods assume that the velocity and pressure signals are harmonic. In an earlier study we have shown that matrix-free continuation methods can efficiently calculate the limit cycles of large thermoacoustic systems. This paper demonstrates that these continuation methods can also efficiently calculate the bifurcations from the limit cycles. Furthermore, once these bifurcations are found, it is then possible to isolate the coupled flame–acoustic motion that causes the qualitative change in behaviour. This information is vital for techniques that use selective damping to move bifurcations to more favourable locations in the parameter space. The matrix-free methods are demonstrated on a model of a ducted axisymmetric premixed flame, using a kinematic 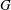$G$-equation solver. The methods find limit cycles and period-2 limit cycles, and fold, period-doubling and Neimark–Sacker bifurcations as a function of the location of the flame in the duct, and the aspect ratio of the steady flame.

JFM classification

Nonlinear Dynamical Systems: Bifurcation Mathematical Foundations: Computational methods Reacting Flows: Flames
Type
Papers
Information
Journal of Fluid Mechanics , Volume 759 , 25 November 2014 , pp. 1 - 27
Check for updates
Copyright
© 2014 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Balachandran, R., Dowling, A. P. & Mastorakos, E. 2008 Nonlinear response of turbulent premixed flames to imposed inlet velocity oscillations of two frequencies. Flow Turbul. Combust. 80 (4), 455–487.CrossRefGoogle Scholar
Balasubramanian, K. & Sujith, R. I. 2008 Thermoacoustic instability in a Rijke tube: non-normality and nonlinearity. Phys. Fluids 20, 044103.CrossRefGoogle Scholar
Birbaud, A. L., Durox, D. & Candel, S. 2006 Upstream flow dynamics of a laminar premixed conical flame submitted to acoustic modulations. Combust. Flame 146, 541–552.CrossRefGoogle Scholar
Bloxsidge, G. J., Dowling, A. P. & Langhorne, P. J. 1988 Reheat buzz – an acoustically coupled combustion instability, part II theory. J. Fluid Mech. 193, 445–473.CrossRefGoogle Scholar
Blumenthal, R. S., Subramanian, P., Sujith, R. I. & Polifke, W. 2013 Novel perspectives on the dynamics of premixed flames. Combust. Flame 160 (7), 1215–1224.CrossRefGoogle Scholar
Chandler, G. J. & Kerswell, R. R. 2013 Simple invariant solutions embedded in 2D Kolmogorov turbulence. J. Fluid Mech. 722, 554–595.CrossRefGoogle Scholar
Dowling, A. P. 1999 A kinematic model of a ducted flame. J. Fluid Mech. 394, 51–72.CrossRefGoogle Scholar
Ducruix, S., Durox, D. & Candel, S. 2000 Theoretical and experimental determinations of the transfer function of a laminar premixed flame. Proc. Combust. Inst. 28 (1), 765–773.CrossRefGoogle Scholar
Erdogan, U., Thies, J., Wubs, F. & Dijkstra, H. 2011 Determining (seasonal) periodic orbits in global ocean models using continuation methods. Bifurcations in Fluid Dynamics, Barcelona. Available at: congress.cimne.upc.es/bifd2011/Admin/Files/FileAbstract/a195.pdf.Google Scholar
Georg, K. 2001 Matrix-free numerical continuation and bifurcation. Numer. Funct. Anal. Optim. 22 (3–4), 303–320.CrossRefGoogle Scholar
Gotoda, H., Asano, Y., Chuah, K. H. & Kushida, G. 2009 Nonlinear analysis on dynamic behavior of buoyancy-induced flame oscillation under swirling flow. Intl J. Heat Mass Transfer 52 (23–24), 5423–5432.CrossRefGoogle Scholar
Gotoda, H. & Ueda, T. 2002 Transition from periodic to non-periodic motion of a bunsen-type premixed flame tip with burner rotation. Proc. Combust. Inst. 29, 1503–1509.CrossRefGoogle Scholar
Hartmann, D., Meinke, M. & Schröder, W. 2010 The constrained reinitialization equation for level set methods. J. Comput. Phys. 229 (5), 1514–1535.CrossRefGoogle Scholar
Heckl, M. A. 2013a Analytical model of nonlinear thermo-acoustic effects in a matrix burner. J. Sound Vib. 332, 4021–4036.CrossRefGoogle Scholar
Heckl, M. A.2013b Analytical model of nonlinear thermo-acoustic effects in a matrix burner. n3l - International Summer School and Workshop on Non-Normal and Nonlinear Effects in Aero- and Thermoacoustics.CrossRefGoogle Scholar
Hemchandra, S.2009 Dynamics of turbulent premixed flames in acoustic fields. PhD thesis, Georgia Institute of Technology.Google Scholar
Hemchandra, S., Preetham,   & Lieuwen, T. C. 2007 Response of turbulent premixed flames to harmonic acoustic forcing. Proc. Combust. Inst. 31 (1), 1427–1434.Google Scholar
Hemchandra, S., Shreekrishna,   & Lieuwen, T. 2010 Premixed flame response to equivalence ratio perturbations. Combust. Theor. Model. 14 (5), 681–714.Google Scholar
Jiang, G.-S. & Peng, D. 2000 Weighted ENO schemes for Hamilton–Jacobi equations. SIAM J. Sci. Comput. 21 (6), 2126–2143.CrossRefGoogle Scholar
Juniper, M. P. 2011 Triggering in the horizontal Rijke tube: non-normality, transient growth and bypass transition. J. Fluid Mech. 667, 272–308.CrossRefGoogle Scholar
Kabiraj, L., Saurabh, A., Wahi, P. & Sujith, R. I. 2012a Route to chaos for combustion instability in ducted laminar premixed flames. Chaos 22 (2), 023129.CrossRefGoogle ScholarPubMed
Kabiraj, L., Sujith, R. I. & Wahi, P. 2012b Bifurcations of self-excited ducted laminar premixed flames. Trans. ASME J. Engng Gas Turbines Power 134 (3), 031502.CrossRefGoogle Scholar
Kashinath, K., Hemchandra, S. & Juniper, M. P.2012 Nonlinear phenomena in thermoacoustic systems with premixed flames. In Proceedings of the ASME Turbo Expo, GT2012-68726.Google Scholar
Kashinath, K., Hemchandra, S. & Juniper, M. 2013a Nonlinear thermoacoustics of ducted premixed flames: the influence of perturbation convection speed. Combust. Flame 160, 2856–2865.CrossRefGoogle Scholar
Kashinath, K. & Juniper, M. P.2012 Nonlinear phenomena in thermoacoustics: a comparison between single-mode and multi-mode methods. In Proceedings of the 19th International Conference on Sound and Vibration.Google Scholar
Kashinath, K., Waugh, I. C. & Juniper, M. P. 2013b Nonlinear self-excited thermoacoustic oscillations of a ducted premixed flame: bifurcations and routes to chaos. J. Fluid Mech. (submitted).Google Scholar
Magri, L. & Juniper, M. P. 2013 Sensitivity analysis of a time-delayed thermoacoustic system via an adjoint-based approach. J. Fluid Mech. 719, 183–202.CrossRefGoogle Scholar
Miller, J.2009 Shape curve analysis using curvature. PhD thesis, University of Glasgow.Google Scholar
Moeck, J. P. & Paschereit, C. O. 2012 Nonlinear interaction of multiple linearly unstable thermoacoustic modes. Intl J. Spray Combust. Dyn. 4 (1), 1–28.CrossRefGoogle Scholar
Noiray, N., Durox, D., Schuller, T. & Candel, S. 2008 A unified framework for nonlinear combustion instability analysis based on the flame describing function. J. Fluid Mech. 615, 139–167.CrossRefGoogle Scholar
Oberlack, M. & Cheviakov, A. F. 2010 Higher-order symmetries and conservation laws of the $G$ -equation for premixed combustion and resulting numerical schemes. J. Engng Maths 66 (1–3), 121–140.CrossRefGoogle Scholar
Preetham,  2007 Modeling the response of premixed flames to flow disturbances. PhD thesis, Georgia Institute of Technology.Google Scholar
Roose, D., Lust, K., Champneys, A. & Spence, A. 1995 A Newton–Picard shooting method for computing periodic solutions of large-scale dynamical systems. Chaos Solitons Fractals 5 (10), 1913–1925.CrossRefGoogle Scholar
Saad, Y. & Schultz, M. H. 1986 GMRES: a generalized minimal residual algorithm for solving nonsymmetric linear systems. SIAM J. Sci. Stat. Comput. 7 (3), 856–869.CrossRefGoogle Scholar
Salinger, A. G., Lehoucq, R. B., Pawlowski, R. P. & Shadid, J. N. 2002 Computational bifurcation and stability studies of the 8:1 thermal cavity problem. Intl J. Numer. Meth. Fluids 1073, 1059–1073.CrossRefGoogle Scholar
Sánchez, J., Marques, F. & Lopez, J. M. 2002 A continuation and bifurcation technique for Navier–Stokes flows. J. Comput. Phys. 180 (1), 78–98.CrossRefGoogle Scholar
Sánchez, J. & Net, M. 2010 On the multiple shooting continuation of periodic orbits by Newton–Krylov methods. Intl J. Bifurcation Chaos 20 (01), 1–19.CrossRefGoogle Scholar
Sánchez, J., Net, M., García-Archilla, B. & Simó, C. 2004 Newton–Krylov continuation of periodic orbits for Navier–Stokes flows. J. Comput. Phys. 201 (1), 13–33.CrossRefGoogle Scholar
Sayadi, T., Le Chenadec, V., Schmid, P., Richecoeur, F. & Massot, M. 2014 Thermoacoustic instability – a dynamical system and time domain analysis. J. Fluid Mech. 753, 448–471.CrossRefGoogle Scholar
Schmid, P. J. 2010 Dynamic mode decomposition of numerical and experimental data. J. Fluid Mech. 656, 5–28.CrossRefGoogle Scholar
Schuller, T., Durox, D. & Candel, S. 2003 A unified model for the prediction of laminar flame transfer functions. Combust. Flame 134 (1–2), 21–34.CrossRefGoogle Scholar
Selimefendigil, F., Sujith, R. I. & Polifke, W. 2011 Identification of heat transfer dynamics for non-modal analysis of thermoacoustic stability. Appl. Maths Comput. 217, 5134–5150.CrossRefGoogle Scholar
Shin, D.-H., Plaks, D. V. & Lieuwen, T. 2011 Dynamics of a longitudinally forced, bluff body stabilized flame. J. Propul. Power 27 (1), 105–116.CrossRefGoogle Scholar
Viswanath, D. 2007 Recurrent motions within plane Couette turbulence. J. Fluid Mech. 580, 339–358.CrossRefGoogle Scholar
Waugh, I.2013 Methods for analysis of nonlinear thermoacoustic systems. PhD in Engineering, University of Cambridge.Google Scholar
Waugh, I., Illingworth, S. & Juniper, M. 2013 Matrix-free continuation for bifurcation analysis of large thermoacoustic systems. J. Comput. Phys. 240, 225–247.CrossRefGoogle Scholar
Waugh, I. C. & Juniper, M. P. 2011 Triggering in a thermoacoustic system with stochastic noise. J. Spray Combust. Dyn. 3 (3), 225–242.CrossRefGoogle Scholar