Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Haffner, Yann
Borée, Jacques
Spohn, Andreas
and
Castelain, Thomas
2020.
Unsteady Coanda effect and drag reduction for a turbulent wake.
Journal of Fluid Mechanics,
Vol. 899,
Issue. ,
Kang, Nam
Essel, Ebenezer E.
Roussinova, Vesselina
and
Balachandar, Ram
2021.
Effects of approach flow conditions on the unsteady three-dimensional wake structure of a square-back Ahmed body.
Physical Review Fluids,
Vol. 6,
Issue. 3,
Urquhart, Magnus
Varney, Max
Sebben, Simone
and
Passmore, Martin
2021.
Drag reduction mechanisms on a generic square-back vehicle using an optimised yaw-insensitive base cavity.
Experiments in Fluids,
Vol. 62,
Issue. 12,
He, Kan
Minelli, Guglielmo
Su, Xinchao
Gao, Guangjun
and
Krajnović, Siniša
2021.
Influence of the rounded rear edge on wake bi-stability of a notchback bluff body.
Physics of Fluids,
Vol. 33,
Issue. 11,
Passaggia, Pierre-Yves
Mazellier, Nicolas
and
Kourta, Azeddine
2021.
Aerodynamic drag modification induced by free-stream turbulence effects on a simplified road vehicle.
Physics of Fluids,
Vol. 33,
Issue. 10,
Haffner, Yann
Castelain, Thomas
Borée, Jacques
and
Spohn, Andreas
2021.
Manipulation of three-dimensional asymmetries of a turbulent wake for drag reduction.
Journal of Fluid Mechanics,
Vol. 912,
Issue. ,
Fan, Yajun
Parezanović, Vladimir
and
Cadot, Olivier
2022.
Wake transitions and steady -instability of an Ahmed body in varying flow conditions.
Journal of Fluid Mechanics,
Vol. 942,
Issue. ,
Khan, Tauha Irfan
Tajik, Abdul Raouf
and
Parezanovic, Vladimir
2022.
Drag reduction of a generic transport vehicle model using a fluidic oscillator.
International Journal of Thermofluids,
Vol. 15,
Issue. ,
p.
100180.
Bonnavion, G.
Borée, J.
and
Herbert, V.
2022.
On the use of kinetic energy balance for the volumetric identification of drag sources of a blunt body. Application to road vehicles.
International Journal of Heat and Fluid Flow,
Vol. 96,
Issue. ,
p.
108977.
Khan, Tauha Irfan
Parezanović, Vladimir
Pastur, Luc
and
Cadot, Olivier
2022.
Suppression of the wake steady asymmetry of an Ahmed body by central base bleed.
Physical Review Fluids,
Vol. 7,
Issue. 8,
Khan, Tauha Irfan
Tajik, Abdul Raouf
and
Parezanovic, Vladimir
2022.
Drag Reduction of a Generic Transport Vehicle Model Using a Fluidic Oscillator.
SSRN Electronic Journal ,
Haffner, Yann
Li, Ruiying
Meldi, Marcello
and
Borée, Jacques
2022.
Drag reduction of a square-back bluff body under constant cross-wind conditions using asymmetric shear layer forcing.
International Journal of Heat and Fluid Flow,
Vol. 96,
Issue. ,
p.
109003.
Bao, Di
Borée, Jacques
Haffner, Yann
and
Sicot, Christophe
2022.
Near wake interactions and drag increase regimes for a square-back bluff body.
Journal of Fluid Mechanics,
Vol. 936,
Issue. ,
Zhang, B.F.
Fan, D.W.
and
Zhou, Y.
2023.
Artificial intelligence control of a low-drag Ahmed body using distributed jet arrays.
Journal of Fluid Mechanics,
Vol. 963,
Issue. ,
Plumejeau, Baptiste
Keirsbulck, Laurent
Basley, Jérémy
Lippert, Marc
Delprat, Sébastien
and
Abassi, Wafik
2023.
Drag mitigation by steady blowing and Coanda effect on a square back Ahmed body.
European Journal of Mechanics - B/Fluids,
Vol. 98,
Issue. ,
p.
80.
Bao, D.
Borée, J.
Sicot, C.
and
Roebroeck, C.
2023.
Salient features of wheel-vehicle aerodynamic interactions: Consequences for drag.
Journal of Wind Engineering and Industrial Aerodynamics,
Vol. 236,
Issue. ,
p.
105366.
Darabasz, T.
Bonnavion, G.
Cadot, O.
Goraguer, Y.
and
Borée, J.
2023.
Drag reduction using longitudinal vortices on a flat-back Ahmed body.
Experiments in Fluids,
Vol. 64,
Issue. 1,
Semaan, Richard
Oswald, Philipp
Cornejo Maceda, Guy Y.
and
Noack, Bernd R.
2023.
Aerodynamic optimization of a generic light truck under unsteady conditions using gradient-enriched machine learning control.
Experiments in Fluids,
Vol. 64,
Issue. 3,
Khan, T.I.
Pastur, L.
Cadot, O.
and
Parezanović, V.
2024.
Equilibrium of fluid fluxes in the wake of a three-dimensional flat-back bluff body.
Journal of Fluid Mechanics,
Vol. 997,
Issue. ,
Cembalo, Agostino
Coirault, Patrick
Borée, Jacques
Dumand, Clément
and
Mercère, Guillaume
2024.
Active control of road vehicle’s drag for varying upstream flow conditions using a recursive subspace based predictive control methodology.
Control Engineering Practice,
Vol. 152,
Issue. ,
p.
106071.
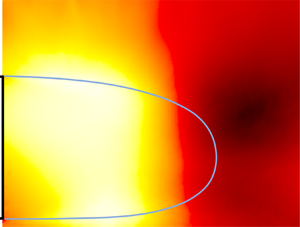
 $Re_H = 5 \times 10^5$ based on the height
$Re_H = 5 \times 10^5$ based on the height  $H$ of the body. By using passive perturbations around the model, the large-scale asymmetry and dynamics of the unforced recirculation region are modified. Depending on the unforced wake equilibrium, additional high-frequency pulsed blowing, coupled with small curved deflecting surfaces along selected edges of the base, produces a very different impact on the drag. On the one hand, forcing the wake along all edges results in important drag reduction of up to 12 % through a wake-shaping mechanism with weak influence on the large-scale asymmetry. On the other hand, the reorganization of the recirculation region equilibrium plays a key role in the observed drag changes when the wake is only forced along some edges of the base. In particular, the symmetrization of the mean wake and the influence of forcing on the interaction mechanism between facing shear layers described by Haffner et al. (J. Fluid Mech., vol. 894, 2020, A14) appears to be one of the main mechanisms involved in drag reduction. Even if asymmetric forcing strategies resulting in symmetrization of the mean wake provide more modest drag reductions of up to 7 % compared with forcing around the whole base, they are more efficient from an energetic point of view. This study provides key ingredients to adapt forcing strategies for drag reduction in the presence of various wake asymmetries typically imposed in real flow conditions around ground vehicles.
$H$ of the body. By using passive perturbations around the model, the large-scale asymmetry and dynamics of the unforced recirculation region are modified. Depending on the unforced wake equilibrium, additional high-frequency pulsed blowing, coupled with small curved deflecting surfaces along selected edges of the base, produces a very different impact on the drag. On the one hand, forcing the wake along all edges results in important drag reduction of up to 12 % through a wake-shaping mechanism with weak influence on the large-scale asymmetry. On the other hand, the reorganization of the recirculation region equilibrium plays a key role in the observed drag changes when the wake is only forced along some edges of the base. In particular, the symmetrization of the mean wake and the influence of forcing on the interaction mechanism between facing shear layers described by Haffner et al. (J. Fluid Mech., vol. 894, 2020, A14) appears to be one of the main mechanisms involved in drag reduction. Even if asymmetric forcing strategies resulting in symmetrization of the mean wake provide more modest drag reductions of up to 7 % compared with forcing around the whole base, they are more efficient from an energetic point of view. This study provides key ingredients to adapt forcing strategies for drag reduction in the presence of various wake asymmetries typically imposed in real flow conditions around ground vehicles.