Article contents
Lock-exchange gravity currents propagating in a channel containing an array of obstacles
Published online by Cambridge University Press: 26 January 2015
Abstract
Large eddy simulation (LES) is used to investigate the evolution of Boussinesq gravity currents propagating through a channel of height 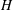 $H$ containing a staggered array of identical cylinders of square cross-section and edge length
$H$ containing a staggered array of identical cylinders of square cross-section and edge length 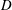 $D$. The cylinders are positioned with their axes horizontal and perpendicular to the (streamwise) direction along which the lock-exchange flow develops. The effects of the volume fraction of solids,
$D$. The cylinders are positioned with their axes horizontal and perpendicular to the (streamwise) direction along which the lock-exchange flow develops. The effects of the volume fraction of solids, 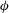 ${\it\phi}$, the Reynolds number and geometrical parameters describing the array of obstacles on the structure of the lock-exchange flow, total drag force acting on the gravity current, front velocity and global energy budget are analysed. Simulation results show that the currents rapidly transition to a state in which the extra resistance provided by the cylinders strongly retards the motion and dominates the dissipative processes. A shallow layer model is also formulated and similarity solutions for the motion are found in the regime where the driving buoyancy forces are balanced by the drag arising from the interaction with the cylinders. The numerical simulations and this shallow layer model show that low-Reynolds-number currents transition to a drag-dominated regime in which the resistance is linearly proportional to the flow speed and, consequently, the front velocity,
${\it\phi}$, the Reynolds number and geometrical parameters describing the array of obstacles on the structure of the lock-exchange flow, total drag force acting on the gravity current, front velocity and global energy budget are analysed. Simulation results show that the currents rapidly transition to a state in which the extra resistance provided by the cylinders strongly retards the motion and dominates the dissipative processes. A shallow layer model is also formulated and similarity solutions for the motion are found in the regime where the driving buoyancy forces are balanced by the drag arising from the interaction with the cylinders. The numerical simulations and this shallow layer model show that low-Reynolds-number currents transition to a drag-dominated regime in which the resistance is linearly proportional to the flow speed and, consequently, the front velocity, 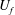 $U_{f}$, is proportional to
$U_{f}$, is proportional to 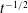 $t^{-1/2}$, where
$t^{-1/2}$, where  $t$ is the time measured starting at the gate release time. By contrast, high-Reynolds-number currents, for which the cylinder Reynolds number is sufficiently high that the drag coefficient for most of the cylinders can be considered constant, transition first to a quadratic drag-dominated regime in which the front speed determined from the simulations is given by
$t$ is the time measured starting at the gate release time. By contrast, high-Reynolds-number currents, for which the cylinder Reynolds number is sufficiently high that the drag coefficient for most of the cylinders can be considered constant, transition first to a quadratic drag-dominated regime in which the front speed determined from the simulations is given by 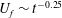 $U_{f}\sim t^{-0.25}$, before undergoing a subsequent transition to the aforementioned linear drag regime in which
$U_{f}\sim t^{-0.25}$, before undergoing a subsequent transition to the aforementioned linear drag regime in which 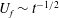 $U_{f}\sim t^{-1/2}$. Meanwhile, away from the front, the depth-averaged gravity current velocity is proportional to
$U_{f}\sim t^{-1/2}$. Meanwhile, away from the front, the depth-averaged gravity current velocity is proportional to 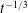 $t^{-1/3}$, a result that is in agreement with the shallow water model. It is suggested that the difference between these two is due to mixing processes, which are shown to be significant in the numerical simulations, especially close to the front of the motion. Direct estimation of the drag coefficient
$t^{-1/3}$, a result that is in agreement with the shallow water model. It is suggested that the difference between these two is due to mixing processes, which are shown to be significant in the numerical simulations, especially close to the front of the motion. Direct estimation of the drag coefficient 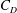 $C_{D}$ from the numerical simulations shows that the combined drag parameter for the porous medium,
$C_{D}$ from the numerical simulations shows that the combined drag parameter for the porous medium, 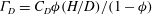 ${\it\Gamma}_{D}=C_{D}{\it\phi}(H/D)/(1-{\it\phi})$, is the key dimensionless grouping of variables that determines the speed of propagation of the current within arrays with different
${\it\Gamma}_{D}=C_{D}{\it\phi}(H/D)/(1-{\it\phi})$, is the key dimensionless grouping of variables that determines the speed of propagation of the current within arrays with different  $C_{D},{\it\phi}$ and
$C_{D},{\it\phi}$ and 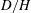 $D/H$.
$D/H$.
- Type
- Papers
- Information
- Copyright
- © 2015 Cambridge University Press
References
- 20
- Cited by



