Hostname: page-component-669899f699-qzcqf Total loading time: 0 Render date: 2025-04-24T16:48:31.912Z Has data issue: false hasContentIssue false
  • English
  • Français

Liquid plugs in narrow tubes: application to airway occlusion

Published online by Cambridge University Press:  04 November 2024

Georg F. Dietze Open the ORCID record for Georg F. Dietze [Opens in a new window]
Georg F. Dietze*
Affiliation:
Université Paris-Saclay, CNRS, FAST, 91405 Orsay, France
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

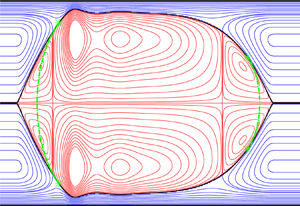
We study liquid plugs in the pulmonary airways based on the two-phase axisymmetric weighted residual integral boundary-layer model of Dietze et al. (J. Fluid Mech., vol. 894, 2020, A17), which was originally developed to study liquid films coating the inner surface of a cylindrical tube in interaction with a core gas flow. The augmented form of this model, which was never applied beyond a proof of concept, allows for the representation of liquid pseudo-plugs. Here, we demonstrate its predictive power vs experiments and direct numerical simulations, in terms of the dynamics of plug formation and the characteristics of developed liquid plugs, such as their shape, flow field, speed and length, as well as the associated wall stresses and their spatial derivatives. In particular, we show that the augmented model allows us to establish a direct continuation path from travelling-wave solutions (TWS) to travelling-plug solutions (TPS). We then apply the model to predict mucus plugs in the conducting zone of the tracheobronchial tree, based on the lung architecture model of Weibel. We proceed by numerical continuation of travelling-state solutions in terms of the airway generation, whereby we impose the wavelength of the linearly most-amplified convective instability (CI) mode or that of the absolute instability (AI) mode. We identify the critical airway generation for liquid-plug formation (TWS/TPS transition), maximum potential for wall-stress-induced epithelial cell damage and CI/AI transition, and investigate how these phenomena are affected by the main control parameters, i.e. airway orientation vs gravity, air flow rate, mucus properties and airway size.

JFM classification

Interfacial Flows (free surface): Thin films Biological Fluid Dynamics: Pulmonary fluid mechanics
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 998 , 10 November 2024 , A50
Check for updates
Copyright
© The Author(s), 2024. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

Atasi, O., Khodaparast, S., Scheid, B. & Stone, H.A. 2017 Effect of buoyancy on the motion of long bubbles in horizontal tubes. Phys. Rev. Fluids 2, 094304.CrossRefGoogle Scholar
Aussillous, P. & Quéré, D. 2000 Quick deposition of a fluid on the wall of a tube. Phys. Fluids 12, 2367–2371.CrossRefGoogle Scholar
Bahrani, S.A., Hamidouche, S., Moazzen, M., Seck, K., Duc, C., Muradoglu, M., Grotberg, J.B. & Romano, F. 2022 Propagation and rupture of elastoviscoplastic liquid plugs in airway reopening model. J. Non-Newtonian Fluid Mech. 300, 104718.CrossRefGoogle Scholar
Baudoin, M., Song, Y., Manneville, P. & Baroud, C.N. 2013 Airway reopening through catastrophic events in a hierarchical network. Proc. Natl Acad. Sci. USA 110, 859–864.CrossRefGoogle Scholar
Bico, J. & Quéré, D. 2001 Falling slugs. J. Colloid Interface Sci. 243 (1), 262–264.CrossRefGoogle Scholar
Bilek, A.M., Dee, K.C. & Gaver III, D.P. 2003 Mechanisms of surface-tension-induced epithelial cell damage in a model of pulmonary airway reopening. J. Appl. Physiol. 94, 770–783.CrossRefGoogle Scholar
Bottier, M., Peña Fernãndez, M., Pelle, G., Isabey, D., Louis, B., Grotberg, J.B. & Filoche, M. 2017 A new index for characterizing micro-bead motion in a flow induced by ciliary beating. Part II. Modeling. PLoS Comput. Biol. 13 (7), e1005552.CrossRefGoogle Scholar
Bretherton, F.P. 1961 The motion of long bubbles in tubes. J. Fluid Mech. 10, 166–188.CrossRefGoogle Scholar
Brevdo, L., Laure, P., Dias, F. & Bridges, T.J. 1999 Linear pulse structure and signalling in a film flow on an inclined plane. J. Fluid Mech. 396, 37–71.CrossRefGoogle Scholar
Camassa, R., Marzuola, J.L., Ogrosky, H.R. & Swygert, S. 2021 On the stability of traveling wave solutions to thin-film and long-wave models for film flows inside a tube. Physica D 415, 132750.CrossRefGoogle Scholar
Camassa, R., Marzuola, J.L, Ogrosky, H.R. & Vaughn, N. 2016 Traveling waves for a model of gravity-driven film flows in cylindrical domains. Physica D 333, 254–265.CrossRefGoogle Scholar
Camassa, R., Ogrosky, H.R. & Olander, J. 2014 Viscous film-flow coating the interior of a vertical tube. Part 1. Gravity-driven flow. J. Fluid Mech. 745, 682–715.CrossRefGoogle Scholar
Camassa, R., Ogrosky, H.R. & Olander, J. 2017 Viscous film-flow coating the interior of a vertical tube. Part 2. Air-driven flow. J. Fluid Mech. 825, 1056–1090.CrossRefGoogle Scholar
Chang, H.C., Demekhin, E.A. & Kalaidin, E. 1996 Simulation of noise-driven wave dynamics on a falling film. AIChE J. 42 (6), 1553–1568.CrossRefGoogle Scholar
Chatelin, R., Anne-Archard, D., Murris-Espin, M., Thiriet, M. & Poncet, P. 2017 Numerical and experimental investigation of mucociliary clearance breakdown in cystic fibrosis. J. Biomech. 53, 56–63.CrossRefGoogle ScholarPubMed
Choudhury, A., Filoche, M., Ribe, N.M., Grenier, N. & Dietze, G.F. 2023 On the role of viscoelasticity in mucociliary clearance: a hydrodynamic continuum approach. J. Fluid Mech. 971, A33.CrossRefGoogle Scholar
Corrin, B. & Nicholson, A.G. 2011 Pathology of the lungs E-book: expert consult: Online and Print. Elsevier Health Sciences.Google Scholar
Dao, E.K. & Balakotaiah, V. 2000 Experimental study of wave occlusion on falling films in a vertical pipe. AIChE J. 46 (7), 1300–1306.CrossRefGoogle Scholar
Delaunay, C. 1841 Sur la surface de révolution dont la courbure moyenne est constante. J. Math. Pures Appl. 6, 309–320.Google Scholar
Dietze, G.F. 2022 Falling Liquid Films in narrow geometries and other Thin Film Flows. Habilitation thesis, Université Paris-Saclay, HAL Id tel-04437816.Google Scholar
Dietze, G.F., Lavalle, G. & Ruyer-Quil, C. 2020 Falling liquid films in narrow tubes: occlusion scenarios. J. Fluid Mech. 894, A17.CrossRefGoogle Scholar
Dietze, G.F. & Ruyer-Quil, C. 2015 Films in narrow tubes. J. Fluid Mech. 762, 68–109.CrossRefGoogle Scholar
Ding, Z., Liu, Z., Liu, R. & Yang, C. 2019 Thermocapillary effect on the dynamics of liquid films coating the interior surface of a tube. Intl J. Heat Mass Transfer 138, 524–533.CrossRefGoogle Scholar
Doedel, E.J. 2008 AUTO07p: Continuation and Bifurcation Software for Ordinary Differential Equations. Montreal Concordia University.Google Scholar
Everett, D.H. & Haynes, J.M. 1972 Model studies of capillary condensation. J. Colloid Interface Sci. 38 (1), 125–137.CrossRefGoogle Scholar
Fahy, J.V. & Dickey, B.F. 2010 Airway mucus function and dysfunction. New England J. Med. 363 (23), 2233–2247.CrossRefGoogle Scholar
Filoche, M., Tai, C.-F. & Grotberg, J.B. 2015 Three-dimensional model of surfactant replacement therapy. Proc. Natl Acad. Sci. USA 112 (30), 9287–9292.CrossRefGoogle ScholarPubMed
Fujioka, H. & Grotberg, J.B. 2004 Steady propagation of a liquid plug in a two-dimensional channel. J. Biomech. Engng 126 (5), 567–577.CrossRefGoogle Scholar
Fujioka, H., Halpern, D., Ryans, J. & Gaver III, D.P. 2016 Reduced-dimension model of liquid plug propagation in tubes. Phys. Rev. Fluids 1, 053201.CrossRefGoogle Scholar
Fujioka, H., Takayama, S. & Grotberg, J.B. 2008 Unsteady propagation of a liquid plug in a liquid-lined straight tube. Phys. Fluids 20 (6), 062104.CrossRefGoogle Scholar
Grotberg, J. 1994 Pulmonary flow and transport phenomena. J. Fluid Mech. 26, 529–571.CrossRefGoogle Scholar
Grotberg, J. 2011 Respiratory fluid mechanics. Phys. Fluids 23, 021301.CrossRefGoogle ScholarPubMed
Halpern, D., Jensen, O.E. & Grotberg, J.B. 1998 A theoretical study of surfactant and liquid delivery into the lung. J. Appl. Physiol. 85, 333–352.CrossRefGoogle ScholarPubMed
Hassan, E.A., Uzgoren, E., Fujioka, H., Grotberg, J.B. & Shyy, W. 2011 Adaptive Lagrangian–Eulerian computation of propagation and rupture of a liquid plug in a tube. Intl J. Numer. Meth. Fluids 67 (11), 1373–1392.CrossRefGoogle Scholar
Heil, M. 2001 Finite Reynolds number effects in the Bretherton problem. Phys. Fluids 13 (9), 2517–2521.CrossRefGoogle Scholar
Hickox, C.E. 1971 Instability due to viscosity and density stratification in axisymmetric pipe flow. Phys. Fluids 14 (2), 251–262.CrossRefGoogle Scholar
Hogg, J.C. 2006 State of the art. Bronchiolitis in chronic obstructive pulmonary disease. Proc. Am. Thor. Soc. 3 (6), 489–493.CrossRefGoogle ScholarPubMed
Howell, P.D., Water, S.L. & Grotberg, J.B. 2000 The propagation of a liquid bolus along a liquid-lined flexible tube. J. Fluid Mech. 406, 309–335.CrossRefGoogle Scholar
Hu, Y., Bian, S., Grotberg, J., Filoche, M., White, M., Takayama, J. & Grotberg, J.B. 2015 A microfluidic model to study fluid dynamics of mucus plug rupture in small lung airways. Biomicrofluidics 9 (4), 044119.CrossRefGoogle ScholarPubMed
Hu, Y., Romano, F. & Grotberg, J.B. 2020 Effects of surface tension and yield stress on mucus plug rupture: a numerical study. J. Biomech. Engng 142 (6), 061007.CrossRefGoogle ScholarPubMed
Huh, D., Fujioka, H., Tung, Y.-C., Futai, N., Paine, R., Grotberg, J.B. & Takayama, S. 2007 Acoustically detectable cellular-level lung injury induced by fluid mechanical stresses in microfluidic airway systems. Proc. Natl Acad. Sci. USA 104 (48), 18886–18891.CrossRefGoogle ScholarPubMed
Jensen, M.H., Libchaber, A., Pelce, P. & Zocchi, G. 1987 Effect of gravity on the Saffman–Taylor meniscus: theory and experiment. Phys. Rev. A 35, 2221.CrossRefGoogle ScholarPubMed
Kalliadasis, S. & Chang, H.-C. 1994 Apparent dynamic contact angle of an advancing gas-liquid meniscus. Phys. Fluids 6 (1), 12–23.CrossRefGoogle Scholar
Kalliadasis, S., Ruyer-Quil, C., Scheid, B. & Velarde, M.G. 2012 Falling Liquid Films. Applied Mathematical Sciences, vol. 176. Springer.CrossRefGoogle Scholar
Kay, S.S., Bilek, A.M., Dee, K.C. & Gaver III, D.P. 2004 Pressure gradient, not exposure duration, determines the extent of epithelialcell damage in a model of pulmonary airway reopening. J. Appl. Physiol. 97, 269–352.CrossRefGoogle ScholarPubMed
Khodaparast, S., Kim, M.K., Silpe, J.E. & Stone, H.A. 2017 Bubble-driven detachment of bacteria from confined microgeometries. Environ. Sci. Technol. 51 (3), 1340–1347.CrossRefGoogle ScholarPubMed
Klaseboer, E., Gupta, R. & Manica, R. 2014 An extended Bretherton model for long Taylor bubbles at moderate capillary numbers. Phys. Fluids 26, 032107.CrossRefGoogle Scholar
Lamstaes, C. & Eggers, J. 2017 Arrested bubble rise in a narrow tube. J. Stat. Phys. 167, 656–682.CrossRefGoogle Scholar
Lasseux, D. 1995 Drainage in a capillary: a complete approximated description of the interface. C. R. Acad. Sci. Ser. IIb 321, 125–131.Google Scholar
Magniez, J.C., Baudoin, M., Liu, C. & Zoueshtiagh, F. 2016 Dynamics of liquid plugs in prewetted capillary tubes: from acceleration and rupture to deceleration and airway obstruction. Soft Matt. 12, 8710–8717.CrossRefGoogle ScholarPubMed
Mamba, S.S., Magniez, J.C., Zoueshtiagh, F. & Baudoin, M. 2018 Dynamics of a liquid plug in a capillary tube under cyclic forcing: memory effects and airway reopening. J. Fluid Mech. 838, 165–191.CrossRefGoogle Scholar
Mamba, S.S., Zoueshtiagh, F. & Baudoin, M. 2019 Pressure-driven dynamics of liquid plugs in rectangular microchannels: influence of the transition between quasi-static and dynamic film deposition regimes. Intl J. Multiphase Flow 113, 343–357.CrossRefGoogle Scholar
Mathematica 2014 Version 10.0.2.0. Wolfram Research.Google Scholar
Muradoglu, M., Romano, F., Fujioka, H. & Grotberg, J.B. 2019 Effects of surfactant on propagation and rupture of a liquid plug in a tube. J. Fluid Mech. 872, 407–437.CrossRefGoogle ScholarPubMed
Navon, I.M. 1987 PENT: a periodic pentadiagonal systems solver. Commun. Appl. Numer. Meth. 3, 63–69.CrossRefGoogle Scholar
Nikolayev, V.S. & Marengo, M. 2018 Pulsating heat pipes: basics of functioning and modeling. In ENCYCLOPEDIA OF TWO-PHASE HEAT TRANSFER AND FLOW IV: Modeling Methodologies, Boiling of CO2, and Micro-Two-Phase Cooling Volume 1: Modeling of Two-Phase Flows and Heat Transfer (ed. J.R. Thome), pp. 63–139. World Scientific.CrossRefGoogle Scholar
Ogrosky, H.R. 2021 a Impact of viscosity ratio on falling two-layer viscous film flow inside a tube. Phys. Rev. Fluids 6, 104005.CrossRefGoogle Scholar
Ogrosky, H.R. 2021 b Linear stability and nonlinear dynamics in a long-wave model of film flows inside a tube in the presence of surfactant. J. Fluid Mech. 908, A23.CrossRefGoogle Scholar
Olgac, U. & Muradoglu, M. 2013 Computational modeling of unsteady surfactant-laden liquid plug propagation in neonatal airways. Phys. Fluids 25 (7), 071901.CrossRefGoogle Scholar
Park, C.-W. & Homsy, G.M. 1984 Two-phase displacement in hele shaw cells: theory. J. Fluid Mech. 139, 291–308.CrossRefGoogle Scholar
Patankar, S.V. 1980 Numerical Heat Transfer and Fluid Flow. Taylor & Francis.Google Scholar
Piroird, K., Clanet, C. & Quéré, D. 2011 Detergency in a tube. Soft Matt. 7, 7498–7503.CrossRefGoogle Scholar
Popinet, S. 2009 An accurate adaptive solver for surface-tension-driven interfacial flows. J. Comput. Phys. 228, 5838–5866.CrossRefGoogle Scholar
Richard, G., Ruyer-Quil, C. & Vila, J.P. 2016 A three-equation model for thin films down an inclined plane. J. Fluid Mech. 804, 162–200.CrossRefGoogle Scholar
Romano, F., Muradoglu, M., Fujioka, H. & Grotberg, J.B. 2021 The effect of viscoelasticity in an airway closure model. J. Fluid Mech. 913, A31.CrossRefGoogle Scholar
Ryans, J., Fujioka, H., Halpern, D. & Gaver III, D.P. 2016 Reduced-dimension modeling approach for simulating recruitment/de-recruitment dynamics in the lung. Ann. Biomed. Engng 44, 3619–3631.CrossRefGoogle ScholarPubMed
Spagnolie, S.E. (Ed.) 2015 Complex Fluids in Biological Systems – Experiment, Theory, and Computation. Springer.CrossRefGoogle Scholar
Srinivasan, V., Rahatgaonkar, A.M. & Khandekar, S. 2021 Hydrodynamics of a completely wetting isolated liquid plug oscillating inside a square capillary tube. Intl J. Multiphase Flow 135, 103534.CrossRefGoogle Scholar
Suresh, V. & Grotberg, J.B. 2005 The effect of gravity on liquid plug propagation in a two-dimensional channel. Phys. Fluids 17 (3), 031507.CrossRefGoogle Scholar
Tavana, H., Kuo, C.-H., Lee, Q.Y., Mosadegh, B., Huh, D., Christensen, P.J., Grotberg, J.B. & Takayama, S. 2010 Dynamics of liquid plugs of buffer and surfactant solutions in a micro-engineered pulmonary airway model. Langmuir 26 (5), 3744–3752.CrossRefGoogle Scholar
Tavana, H., Zamankhan, P., Christensen, P.J., Grotberg, J.B. & Takayama, S. 2011 Epithelium damage and protection during reopening of occluded airways in a physiologic microfluidic pulmonary airway model. Biomed. Microdevices 13 (4), 731–742.CrossRefGoogle Scholar
Taylor, G.I. 1961 Deposition of a viscous fluid on the wall of a tube. J. Fluid Mech. 10 (2), 161–165.CrossRefGoogle Scholar
Thiele, U., Velarde, M.G., Neuffer, K & Pomeau, Y. 2001 Sliding drops in the diffuse interface model coupled to hydrodynamics. Phys. Rev. E 64 (6), 061601.CrossRefGoogle ScholarPubMed
Ubal, S., Campana, D.M., Giavedoni, M.D. & Saita, F.A. 2008 Stability of the steady-state displacement of a liquid plug driven by a constant pressure difference along a prewetted capillary tube. Ind. Engng Chem. Res. 47, 6307–6315.CrossRefGoogle Scholar
Vasquez, P.A., Jin, Y., Palmer, E., Hill, D. & Forest, M.G. 2016 Modeling and simulation of mucus flow in human bronchial epithelial cell cultures. Part I. Idealized axisymmetric swirling flow. PLoS Comput. Biol. 12 (8), e1004872.CrossRefGoogle ScholarPubMed
Weber, J., Straka, L., Borgmann, S., Schmidt, J., Wirth, S. & Schumann, S. 2020 Flow-controlled ventilation (fcv) improves regional ventilation in obese patients–a randomized controlled crossover trial. BMC Anesthesiol. 20, 1–10.CrossRefGoogle ScholarPubMed
Weibel, E.R. & Gomez, D.M. 1962 Architecture of the human lung: use of quantitative methods establishes fundamental relations between size and number of lung structures. Science 137 (3530), 577–585.CrossRefGoogle ScholarPubMed
Yu, Y.E., Khodaparast, S. & Stone, H.A. 2018 Separation of particles by size from a suspension using the motion of a confined bubble. Appl. Phys. Lett. 112, 181604.CrossRefGoogle Scholar
Zheng, Y., Fujioka, H. & Grotberg, J.B. 2007 Effects of gravity, inertia, and surfactant on steady plug propagation in a two-dimensional channel. Phys. Fluids 19 (8), 082107.CrossRefGoogle Scholar
Zoueshtiagh, F., Baudoin, M. & Guerrin, D. 2014 Capillary tube wetting induced by particles: towards armoured bubbles tailoring. Soft Matt. 10, 9403–9412.CrossRefGoogle ScholarPubMed