Article contents
Length scales and the turbulent/non-turbulent interface of a temporally developing turbulent jet
Published online by Cambridge University Press: 04 September 2023
Abstract
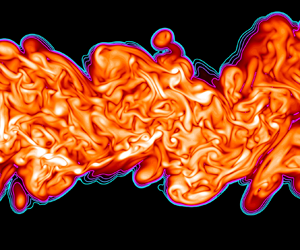
The temporally developing self-similar turbulent jet is fundamentally different from its spatially developing namesake because the former conserves volume flux and has zero cross-stream mean flow velocity whereas the latter conserves momentum flux and does not have zero cross-stream mean flow velocity. It follows that, irrespective of the turbulent dissipation's power-law scalings, the time-local Reynolds number remains constant, and the jet half-width  $\delta$, the Kolmogorov length
$\delta$, the Kolmogorov length  $\eta$ and the Taylor length
$\eta$ and the Taylor length  $\lambda$ grow identically as the square root of time during the temporally developing self-similar planar jet's evolution. We predict theoretically and confirm numerically by direct numerical simulations that the mean centreline velocity, the Kolmogorov velocity and the mean propagation speed of the turbulent/non-turbulent interface (TNTI) of this planar jet decay identically as the inverse square root of time. The TNTI has an inner structure over a wide range of closely spatially packed iso-enstrophy surfaces with fractal dimensions that are well defined over a range of scales between
$\lambda$ grow identically as the square root of time during the temporally developing self-similar planar jet's evolution. We predict theoretically and confirm numerically by direct numerical simulations that the mean centreline velocity, the Kolmogorov velocity and the mean propagation speed of the turbulent/non-turbulent interface (TNTI) of this planar jet decay identically as the inverse square root of time. The TNTI has an inner structure over a wide range of closely spatially packed iso-enstrophy surfaces with fractal dimensions that are well defined over a range of scales between  $\lambda$ and
$\lambda$ and  $\delta$, and that decrease with decreasing iso-enstrophy towards values close to
$\delta$, and that decrease with decreasing iso-enstrophy towards values close to  $2$ at the viscous superlayer. The smallest scale on these isosurfaces is approximately
$2$ at the viscous superlayer. The smallest scale on these isosurfaces is approximately  $\eta$, and the length scales between
$\eta$, and the length scales between  $\eta$ and
$\eta$ and  $\lambda$ contribute significantly to the surface area of the iso-enstrophy surfaces without being characterised by a well-defined fractal dimension. A simple model is sketched for the mean propagation speeds of the iso-enstrophy surfaces within the TNTI of temporally developing self-similar turbulent planar jets. This model is based on a generalised Corrsin length, on the multiscale geometrical properties of the TNTI, and on a proportionality between the turbulent jet volume's growth rate and the growth rate of
$\lambda$ contribute significantly to the surface area of the iso-enstrophy surfaces without being characterised by a well-defined fractal dimension. A simple model is sketched for the mean propagation speeds of the iso-enstrophy surfaces within the TNTI of temporally developing self-similar turbulent planar jets. This model is based on a generalised Corrsin length, on the multiscale geometrical properties of the TNTI, and on a proportionality between the turbulent jet volume's growth rate and the growth rate of  $\delta$. A prediction of this model is that the mean propagation speed at the outer edge of the viscous superlayer is proportional to the Kolmogorov velocity multiplied by the
$\delta$. A prediction of this model is that the mean propagation speed at the outer edge of the viscous superlayer is proportional to the Kolmogorov velocity multiplied by the  $1/4$th power of the global Reynolds number.
$1/4$th power of the global Reynolds number.
JFM classification
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2023. Published by Cambridge University Press
References
- 5
- Cited by



