Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Kamali, M.R.
Sundaresan, S.
Van den Akker, H.E.A.
and
Gillissen, J.J.J.
2012.
A multi-component two-phase lattice Boltzmann method applied to a 1-D Fischer–Tropsch reactor.
Chemical Engineering Journal,
Vol. 207-208,
Issue. ,
p.
587.
Gillissen, J. J. J.
2013.
Turbulent drag reduction using fluid spheres.
Journal of Fluid Mechanics,
Vol. 716,
Issue. ,
p.
83.
Kamali, M. R.
and
Van den Akker, H. E. A.
2013.
Simulating Gas–Liquid Flows by Means of a Pseudopotential Lattice Boltzmann Method.
Industrial & Engineering Chemistry Research,
Vol. 52,
Issue. 33,
p.
11365.
Gruber, Michael C.
Radl, Stefan
and
Khinast, Johannes G.
2013.
Coalescence and Break‐Up in Bubble Columns: Euler‐Lagrange Simulations Using a Stochastic Approach.
Chemie Ingenieur Technik,
Vol. 85,
Issue. 7,
p.
1118.
Ziegenhein, Thomas
Rzehak, Roland
Krepper, Eckhard
and
Lucas, Dirk
2013.
Numerical Simulation of Polydispersed Flow in Bubble Columns with the Inhomogeneous Multi‐Size‐Group Model.
Chemie Ingenieur Technik,
Vol. 85,
Issue. 7,
p.
1080.
Van den Akker, Harry E.A.
2015.
Mesoscale Modeling in Chemical Engineering Part I.
Vol. 46,
Issue. ,
p.
281.
Rantanen, Jukka
and
Khinast, Johannes
2015.
The Future of Pharmaceutical Manufacturing Sciences.
Journal of Pharmaceutical Sciences,
Vol. 104,
Issue. 11,
p.
3612.
Charpentier, Jean-Claude
2016.
What Kind of Modern “Green” Chemical Engineering is Required for the Design of the “Factory of Future”?.
Procedia Engineering,
Vol. 138,
Issue. ,
p.
445.
Loisy, Aurore
Naso, Aurore
and
Spelt, Peter D. M.
2017.
Buoyancy-driven bubbly flows: ordered and free rise at small and intermediate volume fraction.
Journal of Fluid Mechanics,
Vol. 816,
Issue. ,
p.
94.
Tavanashad, Vahid
Passalacqua, Alberto
Fox, Rodney O.
and
Subramaniam, Shankar
2019.
Effect of density ratio on velocity fluctuations in dispersed multiphase flow from simulations of finite-size particles.
Acta Mechanica,
Vol. 230,
Issue. 2,
p.
469.
Uhlemann, Jens
Costa, Raquel
and
Charpentier, Jean-Claude
2019.
Product Design and Engineering in Chemical Engineering: Past, Present State, and Future.
Chemical Engineering & Technology,
Vol. 42,
Issue. 11,
p.
2258.
Jean-Claude Charpentier
2019.
In the Framework of Global Trade, Sustainability and Industry Demand for Innovative Process and Technologies, what kind of Modern “Green” Chemical Engineering is Required for the Design of “the Factory of the Future”?.
International Journal of Petroleum Technology,
Vol. 6,
Issue. ,
p.
7.
Yin, Zegao
Wang, Yanxu
and
Jia, Qianqian
2019.
Hydrodynamic Characteristics of a Pneumatic Breakwater with Combined Wave–Current Actions: A Numerical Investigation.
Journal of Coastal Research,
Vol. 36,
Issue. 1,
p.
196.
Zhang, Lingxin
Zhou, Zecai
Deng, Jian
and
Shao, Xueming
2021.
A numerical study on the drag law of a gas bubble using dynamic body force method.
Physics of Fluids,
Vol. 33,
Issue. 6,
Tavanashad, Vahid
Passalacqua, Alberto
and
Subramaniam, Shankar
2021.
Particle-resolved simulation of freely evolving particle suspensions: Flow physics and modeling.
International Journal of Multiphase Flow,
Vol. 135,
Issue. ,
p.
103533.
Feng, Yi
Sun, Licheng
Mo, Zhengyu
Du, Min
Zhu, Chunxiao
Yang, Wei
and
Xu, Xin
2024.
An evaluation of predictive correlations for the terminal rising velocity of a single bubble in quiescent clean liquid.
International Journal of Multiphase Flow,
Vol. 173,
Issue. ,
p.
104736.
Balcázar-Arciniega, Néstor
Rigola, Joaquim
Pérez-Segarra, Carlos D.
and
Oliva, Assensi
2024.
Numerical study of the drag force, interfacial area and mass transfer in bubbles in a vertical pipe.
Chemical Engineering Journal,
Vol. 495,
Issue. ,
p.
153124.
Pan, Peng
Li, Shi-Jiao
Wei, Hui-Long
Zhang, Xi-Bao
and
Luo, Zheng-Hong
2025.
Bubble columns with internals: A review on research methodology and process intensification.
Chemical Engineering and Processing - Process Intensification,
Vol. 209,
Issue. ,
p.
110156.
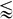 φ
φ 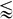 0.5 and 5
0.5 and 5 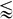 Re
Re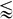 2000. Here R, Uslip and ν denote the bubble radius, the slip velocity between the liquid and the gas phases and the kinematic viscosity of the liquid phase, respectively. The results are rationalized by assuming a similarity between the CD(Reeff)-relation of the suspension and the CD(Re)-relation of an individual bubble, where the effective Reynolds number Reeff = 2RUslip/νeff is based on the effective viscosity νeff which depends on the properties of the suspension. For Re ≲ 100, we find νeff ≈ ν/(1−0.6φ1/3), which is in qualitative agreement with previous proposed correlations for CD in bubble suspensions. For Re ≳ 100, on the other hand, we find νeff ≈ RUslipφ, which is explained by considering the turbulent kinetic energy levels in the liquid phase. Based on these findings, a correlation is constructed for CD(Re, φ). A modification of the drag correlation is proposed to account for effects of bubble deformation, by the inclusion of a correction factor based on the theory of Moore (J. Fluid Mech., vol. 23, 1995, p. 749).
2000. Here R, Uslip and ν denote the bubble radius, the slip velocity between the liquid and the gas phases and the kinematic viscosity of the liquid phase, respectively. The results are rationalized by assuming a similarity between the CD(Reeff)-relation of the suspension and the CD(Re)-relation of an individual bubble, where the effective Reynolds number Reeff = 2RUslip/νeff is based on the effective viscosity νeff which depends on the properties of the suspension. For Re ≲ 100, we find νeff ≈ ν/(1−0.6φ1/3), which is in qualitative agreement with previous proposed correlations for CD in bubble suspensions. For Re ≳ 100, on the other hand, we find νeff ≈ RUslipφ, which is explained by considering the turbulent kinetic energy levels in the liquid phase. Based on these findings, a correlation is constructed for CD(Re, φ). A modification of the drag correlation is proposed to account for effects of bubble deformation, by the inclusion of a correction factor based on the theory of Moore (J. Fluid Mech., vol. 23, 1995, p. 749).

