1. Introduction
To leading order, and assuming a quasi-stationary ocean circulation, the work on the ocean by wind stress is balanced by the negative work at the seafloor due to pressure build-up by bottom topography (Naveira Garabato et al. Reference Naveira Garabato, Nurser, Scott and Goff2013). As a specific example, the strength of the current system circumnavigating the globe in the Southern Ocean, where lack of continental zonal blocking allows for the formation of zonal jets analogous to the atmosphere, may be calculated as the net zonal momentum input via wind stress at the surface and the topographic form stress across ocean ridges (Masich, Chereskin & Mazloff Reference Masich, Chereskin and Mazloff2015). Earth systems models (ESMs), typically having horizontal resolutions of  ${O}$(100 km), are capable of capturing the pressure gradient (and thus the associated form drag) across major topographic features, such as continental slopes and prominent ridges and plateaus with typical horizontal scales of 500–1000 km. Operational and regional ocean models, with typical horizontal grid spacing of
${O}$(100 km), are capable of capturing the pressure gradient (and thus the associated form drag) across major topographic features, such as continental slopes and prominent ridges and plateaus with typical horizontal scales of 500–1000 km. Operational and regional ocean models, with typical horizontal grid spacing of  ${O}$(10–100 km), further resolve flow steering and blocking by smaller topographic features such as minor ridges, seamounts, constrictions, fracture zones, etc. For horizontal scales <10 km, the interaction of tides, eddies and currents with rough topography generates both radiating waves (such as internal tides or lee waves) and non-propagating processes (e.g. hydraulically controlled overturns, trapped waves, boundary layer turbulence; see Meredith & Garabato (Reference Meredith and Garabato2021)). This latter category is almost entirely of subgrid scale in ESMs and regional ocean models. Nevertheless, such processes can have important implications for the dynamical balance of the large-scale flow. For example, while the wave drag and frictional drag associated with small-scale topography are insignificant as compared with the local wind stress momentum input over large areas of the ocean, in certain regions, such as the Southern Ocean, equatorial ocean and over the ridge systems of the Indian and Atlantic basins, they can play a significant role in the momentum budget (Naveira Garabato et al. Reference Naveira Garabato, Nurser, Scott and Goff2013). Of particular importance is the leading-order impact of wave drag induced by the interaction of strong circumpolar currents with rough topography in the Southern Ocean over the deep density stratification in the global ocean as a result of the inter-connectedness of all ocean basins through the Southern Ocean (Marshall et al. Reference Marshall, Ambaum, Maddison, Munday and Novak2017).
${O}$(10–100 km), further resolve flow steering and blocking by smaller topographic features such as minor ridges, seamounts, constrictions, fracture zones, etc. For horizontal scales <10 km, the interaction of tides, eddies and currents with rough topography generates both radiating waves (such as internal tides or lee waves) and non-propagating processes (e.g. hydraulically controlled overturns, trapped waves, boundary layer turbulence; see Meredith & Garabato (Reference Meredith and Garabato2021)). This latter category is almost entirely of subgrid scale in ESMs and regional ocean models. Nevertheless, such processes can have important implications for the dynamical balance of the large-scale flow. For example, while the wave drag and frictional drag associated with small-scale topography are insignificant as compared with the local wind stress momentum input over large areas of the ocean, in certain regions, such as the Southern Ocean, equatorial ocean and over the ridge systems of the Indian and Atlantic basins, they can play a significant role in the momentum budget (Naveira Garabato et al. Reference Naveira Garabato, Nurser, Scott and Goff2013). Of particular importance is the leading-order impact of wave drag induced by the interaction of strong circumpolar currents with rough topography in the Southern Ocean over the deep density stratification in the global ocean as a result of the inter-connectedness of all ocean basins through the Southern Ocean (Marshall et al. Reference Marshall, Ambaum, Maddison, Munday and Novak2017).
In addition to the momentum balance of the ocean, small-scale topography plays an essential role in ocean circulation through the density budget. The deep branch of ocean circulation is fed by dense waters that form in the North Atlantic and around Antarctica, sinking to the abyssal ocean, carrying anthropogenic heat and carbon (among other tracers) away from the atmosphere (Talley Reference Talley2013). Turbulence, induced by the interaction of tides, currents and eddies with rough topography, helps mix dense waters with overlying lighter waters, facilitating their upwelling and thus the ocean's meridional overturning circulation. Such turbulence consists of bottom-enhanced boundary turbulence (Polzin & McDougall Reference Polzin and McDougall2022) and interior mixing generated by topographically induced internal waves that can transfer energy and momentum upward all the way to the surface mixed layer (Shakespeare & Hogg Reference Shakespeare and Hogg2019; de Lavergne et al. Reference de Lavergne, Vic, Madec, Roquet, Waterhouse, Whalen, Cuypers, Bouruet-Aubertot, Ferron and Hibiya2020; Baker & Mashayek Reference Baker and Mashayek2021). While the energy associated with mixing is three orders of magnitude smaller than the total energy associated with the ocean meridional overturning circulation, mixing is essential for the overturning circulation as, in its absence, the deep ocean would remain filled (and stuck) with old dense waters of polar origin (Wunsch & Ferrari Reference Wunsch and Ferrari2004; Ferrari Reference Ferrari2014).
Due to the practical limitations of deep ocean observations, our understanding of the topographic processes that impact the ocean's large-scale momentum and density budgets is limited but rapidly growing (Meredith & Garabato Reference Meredith and Garabato2021). Parameterizations of such subgrid-scale processes in ESMs are in their infancy, and hence reliable turbulence closure models for flow–topography interactions are much needed. Radko (Reference Radko2023) provides a useful step forward in this direction.
2. Overview
Building on earlier works (Radko Reference Radko2022a,Reference Radkob), Radko (Reference Radko2023) extends his sandpaper theory for flow–topography interaction, which previously only included the high-Reynolds-number (fast-flow) regime, to also account for slow flows. He then provides an analytical form that bridges the two asymptotic limits, resulting in a holistic model spanning slow-to fast-flow regimes. Implementing the model in coarse-resolution simulations remarkably reproduces detailed features of flow–topography interactions. The theory is based on three key elements: multiscale analysis, spectral representation of rough topography and quasi-geostrophic rigid-lid framework in single-layer and multilayer configurations.
Within the quasi-geostrophic framework, which allows for balanced dynamics, internal waves are excluded, the impact of ageostrophic submesoscale dynamics is mimicked via an eddy viscosity and frictional bottom Ekman flow is represented through a drag coefficient (although it is not explored as the coefficient is set to zero throughout the analysis). Thus, the model accounts for horizontal scales  ${>}{O}$(10 km). The examples discussed are also aptly aligned with parameters relevant to the Southern Ocean, where flow–rough topography interactions have the most far-reaching global influence (Naveira Garabato et al. Reference Naveira Garabato, Nurser, Scott and Goff2013; Marshall et al. Reference Marshall, Ambaum, Maddison, Munday and Novak2017).
${>}{O}$(10 km). The examples discussed are also aptly aligned with parameters relevant to the Southern Ocean, where flow–rough topography interactions have the most far-reaching global influence (Naveira Garabato et al. Reference Naveira Garabato, Nurser, Scott and Goff2013; Marshall et al. Reference Marshall, Ambaum, Maddison, Munday and Novak2017).
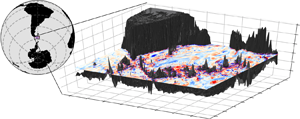
Figure 1(a) shows the topography at the model's heart (hence the term ‘sandpaper’). Such bathymetric representation, built upon the observationally derived spectrum of seafloor roughness (Goff & Jordan Reference Goff and Jordan1988), mimics the statistics of abyssal hills and has been widely used to study topographically induced internal waves and boundary turbulence (Baker & Mashayek Reference Baker and Mashayek2022; Polzin & McDougall Reference Polzin and McDougall2022). Such statistical representation allows for describing the topography in terms of Fourier modes. It thus facilitates the separation of the bathymetric wavenumbers into slowly varying bathymetry (low wavenumbers) and small-scale topography (high wavenumbers). The splitting of topographic height,  $\eta$, into large-scale (
$\eta$, into large-scale ( $\eta _L$) and small-scale (
$\eta _L$) and small-scale ( $\eta _s$) regimes naturally lends to multiscale mechanics analysis. The key parameter for such analysis in Radko's work is
$\eta _s$) regimes naturally lends to multiscale mechanics analysis. The key parameter for such analysis in Radko's work is  $\varepsilon =L_C/L_{LS}$, where
$\varepsilon =L_C/L_{LS}$, where  $L_C$ represents the cut-off wavelength formally separating small and large scales and
$L_C$ represents the cut-off wavelength formally separating small and large scales and  $L_{LS}$ represents the scale of the background flow on which the influence of topography is investigated (i.e. mesoscales and larger).
$L_{LS}$ represents the scale of the background flow on which the influence of topography is investigated (i.e. mesoscales and larger).
Figure 1. (a) A schematic diagram, representing the topography employed in the sandpaper model of Radko (Reference Radko2023). (b) An enlarged view of the solution over a small part of the domain, showing the dominant impacts of topography on the flow in the fast-flow (top row) and slow-flow (bottom row) regimes. Adapted from Radko (Reference Radko2023).
In the fast-flow regime, where  $Re\sim {O}(\varepsilon ^{-1})\gg 1$, Radko (Reference Radko2022a,Reference Radkob) had shown that the dynamics of the perturbations (due to flow–topography interactions) was controlled by the homogenization of small-scale vorticity through the diffusive influence of rough topography. Such diffusion, mimicking the steering of flow by topographic features (see figure 1b, top row), is inferred using the Fourier image of the statistically isotropic small-scale topography component. Importantly, in this regime, the topographic form drag is not consequential for the large-scale flow (more on this in the next section). The shortcoming of the fast-flow regime of Radko (Reference Radko2022a,Reference Radkob) was that once the flow speeds were reduced (
$Re\sim {O}(\varepsilon ^{-1})\gg 1$, Radko (Reference Radko2022a,Reference Radkob) had shown that the dynamics of the perturbations (due to flow–topography interactions) was controlled by the homogenization of small-scale vorticity through the diffusive influence of rough topography. Such diffusion, mimicking the steering of flow by topographic features (see figure 1b, top row), is inferred using the Fourier image of the statistically isotropic small-scale topography component. Importantly, in this regime, the topographic form drag is not consequential for the large-scale flow (more on this in the next section). The shortcoming of the fast-flow regime of Radko (Reference Radko2022a,Reference Radkob) was that once the flow speeds were reduced ( $Re\lesssim 1$), the topographic forcing would blow up. While this was dealt with by an ad hoc constraining of the forcing in the earlier works, in Radko (Reference Radko2023) this issue is addressed by introducing a slow-flow limit and a bridge between the two. In the slow-flow regime corresponding to
$Re\lesssim 1$), the topographic forcing would blow up. While this was dealt with by an ad hoc constraining of the forcing in the earlier works, in Radko (Reference Radko2023) this issue is addressed by introducing a slow-flow limit and a bridge between the two. In the slow-flow regime corresponding to  $\eta _L$, where
$\eta _L$, where  $Re\sim {O}(\varepsilon )\ll 1$, Radko shows that the leading-order balance lies between vortex stretching/squeezing due to advection of large-scale flow into deeper/shallower regions and the frictional spin-down of vortical motions (see figure 1b, bottom row). Radko manages to bridge the two limits by considering the crossing point between the two asymptotic models, represented by the transitional velocity
$Re\sim {O}(\varepsilon )\ll 1$, Radko shows that the leading-order balance lies between vortex stretching/squeezing due to advection of large-scale flow into deeper/shallower regions and the frictional spin-down of vortical motions (see figure 1b, bottom row). Radko manages to bridge the two limits by considering the crossing point between the two asymptotic models, represented by the transitional velocity  $V_C\sim \nu \kappa _{\eta }$, where
$V_C\sim \nu \kappa _{\eta }$, where  $\nu$ is the eddy viscosity representing the unresolved smaller scales and
$\nu$ is the eddy viscosity representing the unresolved smaller scales and  $\kappa _{\eta }$ is the dominant wavenumber of rough topography. Using this crossing point, Radko successfully introduces a simple functional form that bridges the two limits, is well-defined across the slow-to-fast scales and is in remarkable agreement with high-resolution numerical simulations performed for verification.
$\kappa _{\eta }$ is the dominant wavenumber of rough topography. Using this crossing point, Radko successfully introduces a simple functional form that bridges the two limits, is well-defined across the slow-to-fast scales and is in remarkable agreement with high-resolution numerical simulations performed for verification.
The strength of Radko's approach is that (a) the two limits of the parameterization are derived directly from governing equations without any of the empirical assumptions common in other closure models, and (b) the bridge between the two limits works so well that the final composite model agrees with simulations in which no explicit assumption was made about the existence of separation of scales. From a physical standpoint, Radko's model predictions have important implications: in fast flows, topographic drag decreases with increasing speed (somewhat counter-intuitive but with potentially important global implications; see also Marshall et al. (Reference Marshall, Ambaum, Maddison, Munday and Novak2017)), whereas in slow flows, drag increases with increasing speed. This non-monotonic momentum forcing could have observable consequences as it suggests a preferential suppression of flows with speeds close to  $V_C$.
$V_C$.
3. Future
The model of Radko (Reference Radko2023) provides a promising prospect for representing the unresolved complex topography in ESMs. Several extensions are nevertheless required beforehand. The following considerations are essential as theories of flow–topography interaction advance:
1. The spectral representations of seafloor roughness (e.g. the widely used one of Goff & Jordan (Reference Goff and Jordan1988), also the choice in Radko (Reference Radko2023)) have significant shortcomings. While the abyssal hills, consistent with a fractal process (Goff & Jordan Reference Goff and Jordan1988), host the rate-controlling dynamics that facilitate upwelling (Polzin & McDougall Reference Polzin and McDougall2022), they lack larger-scale topographic features that (a) can host nonlinear non-propagating dynamics and (b) are essential for prescription of correct boundary conditions for lee wave generation in models. These issues impact the drag and mixing representation adversely (Klymak et al. Reference Klymak, Balwada, Garabato and Abernathey2021; Baker & Mashayek Reference Baker and Mashayek2022; Polzin & McDougall Reference Polzin and McDougall2022).
2. The quasi-geostrophic framework employed in Radko (Reference Radko2023) systematically prohibits accounting for many ageostrophic submesoscale and radiating processes. Propagating internal tides and lee waves can significantly impact the vertical momentum distribution in the water column. They can also have a non-local impact on momentum and density budgets across the globe (Shakespeare & Hogg Reference Shakespeare and Hogg2019; de Lavergne et al. Reference de Lavergne, Vic, Madec, Roquet, Waterhouse, Whalen, Cuypers, Bouruet-Aubertot, Ferron and Hibiya2020; Baker & Mashayek Reference Baker and Mashayek2021). A host of other processes at the bottom boundary, such as a zoo of submesoscale instabilities and hydraulically controlled flow through narrow constrictions and passages, also have important implications for drag and mixing (Polzin & McDougall Reference Polzin and McDougall2022). While the internal gravity waves are not accounted for by Radko (Reference Radko2023), the eddy viscosity mimics the impact of other bottom-dwelling processes. Extension of the model to primitive equations, as suggested by the author, will go a long way towards allowing for these processes by extending the range of scales permitted and accounting for the density stratification.
3. Finally, flow–topography interactions can also prove significant for budgets of tracers such as carbon, nutrients and heat. For example, tracers have been found to concentrate around rough topography in the Southern Ocean in patterns similar to those in figure 1 (Mashayek et al. Reference Mashayek, Ferrari, Merrifield, Ledwell, St Laurent and Garabato2017; Prend et al. Reference Prend, Gille, Talley, Mitchell, Rosso and Mazloff2019). Including tracer budgets in the theory seems straightforward and can prove quite insightful.
In summary, Radko's work provides a solid base upon which the representation of rough ocean topography in ESMs can evolve.

1. Introduction
To leading order, and assuming a quasi-stationary ocean circulation, the work on the ocean by wind stress is balanced by the negative work at the seafloor due to pressure build-up by bottom topography (Naveira Garabato et al. Reference Naveira Garabato, Nurser, Scott and Goff2013). As a specific example, the strength of the current system circumnavigating the globe in the Southern Ocean, where lack of continental zonal blocking allows for the formation of zonal jets analogous to the atmosphere, may be calculated as the net zonal momentum input via wind stress at the surface and the topographic form stress across ocean ridges (Masich, Chereskin & Mazloff Reference Masich, Chereskin and Mazloff2015). Earth systems models (ESMs), typically having horizontal resolutions of ${O}$(100 km), are capable of capturing the pressure gradient (and thus the associated form drag) across major topographic features, such as continental slopes and prominent ridges and plateaus with typical horizontal scales of 500–1000 km. Operational and regional ocean models, with typical horizontal grid spacing of
${O}$(100 km), are capable of capturing the pressure gradient (and thus the associated form drag) across major topographic features, such as continental slopes and prominent ridges and plateaus with typical horizontal scales of 500–1000 km. Operational and regional ocean models, with typical horizontal grid spacing of  ${O}$(10–100 km), further resolve flow steering and blocking by smaller topographic features such as minor ridges, seamounts, constrictions, fracture zones, etc. For horizontal scales <10 km, the interaction of tides, eddies and currents with rough topography generates both radiating waves (such as internal tides or lee waves) and non-propagating processes (e.g. hydraulically controlled overturns, trapped waves, boundary layer turbulence; see Meredith & Garabato (Reference Meredith and Garabato2021)). This latter category is almost entirely of subgrid scale in ESMs and regional ocean models. Nevertheless, such processes can have important implications for the dynamical balance of the large-scale flow. For example, while the wave drag and frictional drag associated with small-scale topography are insignificant as compared with the local wind stress momentum input over large areas of the ocean, in certain regions, such as the Southern Ocean, equatorial ocean and over the ridge systems of the Indian and Atlantic basins, they can play a significant role in the momentum budget (Naveira Garabato et al. Reference Naveira Garabato, Nurser, Scott and Goff2013). Of particular importance is the leading-order impact of wave drag induced by the interaction of strong circumpolar currents with rough topography in the Southern Ocean over the deep density stratification in the global ocean as a result of the inter-connectedness of all ocean basins through the Southern Ocean (Marshall et al. Reference Marshall, Ambaum, Maddison, Munday and Novak2017).
${O}$(10–100 km), further resolve flow steering and blocking by smaller topographic features such as minor ridges, seamounts, constrictions, fracture zones, etc. For horizontal scales <10 km, the interaction of tides, eddies and currents with rough topography generates both radiating waves (such as internal tides or lee waves) and non-propagating processes (e.g. hydraulically controlled overturns, trapped waves, boundary layer turbulence; see Meredith & Garabato (Reference Meredith and Garabato2021)). This latter category is almost entirely of subgrid scale in ESMs and regional ocean models. Nevertheless, such processes can have important implications for the dynamical balance of the large-scale flow. For example, while the wave drag and frictional drag associated with small-scale topography are insignificant as compared with the local wind stress momentum input over large areas of the ocean, in certain regions, such as the Southern Ocean, equatorial ocean and over the ridge systems of the Indian and Atlantic basins, they can play a significant role in the momentum budget (Naveira Garabato et al. Reference Naveira Garabato, Nurser, Scott and Goff2013). Of particular importance is the leading-order impact of wave drag induced by the interaction of strong circumpolar currents with rough topography in the Southern Ocean over the deep density stratification in the global ocean as a result of the inter-connectedness of all ocean basins through the Southern Ocean (Marshall et al. Reference Marshall, Ambaum, Maddison, Munday and Novak2017).
In addition to the momentum balance of the ocean, small-scale topography plays an essential role in ocean circulation through the density budget. The deep branch of ocean circulation is fed by dense waters that form in the North Atlantic and around Antarctica, sinking to the abyssal ocean, carrying anthropogenic heat and carbon (among other tracers) away from the atmosphere (Talley Reference Talley2013). Turbulence, induced by the interaction of tides, currents and eddies with rough topography, helps mix dense waters with overlying lighter waters, facilitating their upwelling and thus the ocean's meridional overturning circulation. Such turbulence consists of bottom-enhanced boundary turbulence (Polzin & McDougall Reference Polzin and McDougall2022) and interior mixing generated by topographically induced internal waves that can transfer energy and momentum upward all the way to the surface mixed layer (Shakespeare & Hogg Reference Shakespeare and Hogg2019; de Lavergne et al. Reference de Lavergne, Vic, Madec, Roquet, Waterhouse, Whalen, Cuypers, Bouruet-Aubertot, Ferron and Hibiya2020; Baker & Mashayek Reference Baker and Mashayek2021). While the energy associated with mixing is three orders of magnitude smaller than the total energy associated with the ocean meridional overturning circulation, mixing is essential for the overturning circulation as, in its absence, the deep ocean would remain filled (and stuck) with old dense waters of polar origin (Wunsch & Ferrari Reference Wunsch and Ferrari2004; Ferrari Reference Ferrari2014).
Due to the practical limitations of deep ocean observations, our understanding of the topographic processes that impact the ocean's large-scale momentum and density budgets is limited but rapidly growing (Meredith & Garabato Reference Meredith and Garabato2021). Parameterizations of such subgrid-scale processes in ESMs are in their infancy, and hence reliable turbulence closure models for flow–topography interactions are much needed. Radko (Reference Radko2023) provides a useful step forward in this direction.
2. Overview
Building on earlier works (Radko Reference Radko2022a,Reference Radkob), Radko (Reference Radko2023) extends his sandpaper theory for flow–topography interaction, which previously only included the high-Reynolds-number (fast-flow) regime, to also account for slow flows. He then provides an analytical form that bridges the two asymptotic limits, resulting in a holistic model spanning slow-to fast-flow regimes. Implementing the model in coarse-resolution simulations remarkably reproduces detailed features of flow–topography interactions. The theory is based on three key elements: multiscale analysis, spectral representation of rough topography and quasi-geostrophic rigid-lid framework in single-layer and multilayer configurations.
Within the quasi-geostrophic framework, which allows for balanced dynamics, internal waves are excluded, the impact of ageostrophic submesoscale dynamics is mimicked via an eddy viscosity and frictional bottom Ekman flow is represented through a drag coefficient (although it is not explored as the coefficient is set to zero throughout the analysis). Thus, the model accounts for horizontal scales ${>}{O}$(10 km). The examples discussed are also aptly aligned with parameters relevant to the Southern Ocean, where flow–rough topography interactions have the most far-reaching global influence (Naveira Garabato et al. Reference Naveira Garabato, Nurser, Scott and Goff2013; Marshall et al. Reference Marshall, Ambaum, Maddison, Munday and Novak2017).
${>}{O}$(10 km). The examples discussed are also aptly aligned with parameters relevant to the Southern Ocean, where flow–rough topography interactions have the most far-reaching global influence (Naveira Garabato et al. Reference Naveira Garabato, Nurser, Scott and Goff2013; Marshall et al. Reference Marshall, Ambaum, Maddison, Munday and Novak2017).
Figure 1(a) shows the topography at the model's heart (hence the term ‘sandpaper’). Such bathymetric representation, built upon the observationally derived spectrum of seafloor roughness (Goff & Jordan Reference Goff and Jordan1988), mimics the statistics of abyssal hills and has been widely used to study topographically induced internal waves and boundary turbulence (Baker & Mashayek Reference Baker and Mashayek2022; Polzin & McDougall Reference Polzin and McDougall2022). Such statistical representation allows for describing the topography in terms of Fourier modes. It thus facilitates the separation of the bathymetric wavenumbers into slowly varying bathymetry (low wavenumbers) and small-scale topography (high wavenumbers). The splitting of topographic height, $\eta$, into large-scale (
$\eta$, into large-scale ( $\eta _L$) and small-scale (
$\eta _L$) and small-scale ( $\eta _s$) regimes naturally lends to multiscale mechanics analysis. The key parameter for such analysis in Radko's work is
$\eta _s$) regimes naturally lends to multiscale mechanics analysis. The key parameter for such analysis in Radko's work is  $\varepsilon =L_C/L_{LS}$, where
$\varepsilon =L_C/L_{LS}$, where  $L_C$ represents the cut-off wavelength formally separating small and large scales and
$L_C$ represents the cut-off wavelength formally separating small and large scales and  $L_{LS}$ represents the scale of the background flow on which the influence of topography is investigated (i.e. mesoscales and larger).
$L_{LS}$ represents the scale of the background flow on which the influence of topography is investigated (i.e. mesoscales and larger).
Figure 1. (a) A schematic diagram, representing the topography employed in the sandpaper model of Radko (Reference Radko2023). (b) An enlarged view of the solution over a small part of the domain, showing the dominant impacts of topography on the flow in the fast-flow (top row) and slow-flow (bottom row) regimes. Adapted from Radko (Reference Radko2023).
In the fast-flow regime, where $Re\sim {O}(\varepsilon ^{-1})\gg 1$, Radko (Reference Radko2022a,Reference Radkob) had shown that the dynamics of the perturbations (due to flow–topography interactions) was controlled by the homogenization of small-scale vorticity through the diffusive influence of rough topography. Such diffusion, mimicking the steering of flow by topographic features (see figure 1b, top row), is inferred using the Fourier image of the statistically isotropic small-scale topography component. Importantly, in this regime, the topographic form drag is not consequential for the large-scale flow (more on this in the next section). The shortcoming of the fast-flow regime of Radko (Reference Radko2022a,Reference Radkob) was that once the flow speeds were reduced (
$Re\sim {O}(\varepsilon ^{-1})\gg 1$, Radko (Reference Radko2022a,Reference Radkob) had shown that the dynamics of the perturbations (due to flow–topography interactions) was controlled by the homogenization of small-scale vorticity through the diffusive influence of rough topography. Such diffusion, mimicking the steering of flow by topographic features (see figure 1b, top row), is inferred using the Fourier image of the statistically isotropic small-scale topography component. Importantly, in this regime, the topographic form drag is not consequential for the large-scale flow (more on this in the next section). The shortcoming of the fast-flow regime of Radko (Reference Radko2022a,Reference Radkob) was that once the flow speeds were reduced ( $Re\lesssim 1$), the topographic forcing would blow up. While this was dealt with by an ad hoc constraining of the forcing in the earlier works, in Radko (Reference Radko2023) this issue is addressed by introducing a slow-flow limit and a bridge between the two. In the slow-flow regime corresponding to
$Re\lesssim 1$), the topographic forcing would blow up. While this was dealt with by an ad hoc constraining of the forcing in the earlier works, in Radko (Reference Radko2023) this issue is addressed by introducing a slow-flow limit and a bridge between the two. In the slow-flow regime corresponding to  $\eta _L$, where
$\eta _L$, where  $Re\sim {O}(\varepsilon )\ll 1$, Radko shows that the leading-order balance lies between vortex stretching/squeezing due to advection of large-scale flow into deeper/shallower regions and the frictional spin-down of vortical motions (see figure 1b, bottom row). Radko manages to bridge the two limits by considering the crossing point between the two asymptotic models, represented by the transitional velocity
$Re\sim {O}(\varepsilon )\ll 1$, Radko shows that the leading-order balance lies between vortex stretching/squeezing due to advection of large-scale flow into deeper/shallower regions and the frictional spin-down of vortical motions (see figure 1b, bottom row). Radko manages to bridge the two limits by considering the crossing point between the two asymptotic models, represented by the transitional velocity  $V_C\sim \nu \kappa _{\eta }$, where
$V_C\sim \nu \kappa _{\eta }$, where  $\nu$ is the eddy viscosity representing the unresolved smaller scales and
$\nu$ is the eddy viscosity representing the unresolved smaller scales and  $\kappa _{\eta }$ is the dominant wavenumber of rough topography. Using this crossing point, Radko successfully introduces a simple functional form that bridges the two limits, is well-defined across the slow-to-fast scales and is in remarkable agreement with high-resolution numerical simulations performed for verification.
$\kappa _{\eta }$ is the dominant wavenumber of rough topography. Using this crossing point, Radko successfully introduces a simple functional form that bridges the two limits, is well-defined across the slow-to-fast scales and is in remarkable agreement with high-resolution numerical simulations performed for verification.
The strength of Radko's approach is that (a) the two limits of the parameterization are derived directly from governing equations without any of the empirical assumptions common in other closure models, and (b) the bridge between the two limits works so well that the final composite model agrees with simulations in which no explicit assumption was made about the existence of separation of scales. From a physical standpoint, Radko's model predictions have important implications: in fast flows, topographic drag decreases with increasing speed (somewhat counter-intuitive but with potentially important global implications; see also Marshall et al. (Reference Marshall, Ambaum, Maddison, Munday and Novak2017)), whereas in slow flows, drag increases with increasing speed. This non-monotonic momentum forcing could have observable consequences as it suggests a preferential suppression of flows with speeds close to $V_C$.
$V_C$.
3. Future
The model of Radko (Reference Radko2023) provides a promising prospect for representing the unresolved complex topography in ESMs. Several extensions are nevertheless required beforehand. The following considerations are essential as theories of flow–topography interaction advance:
1. The spectral representations of seafloor roughness (e.g. the widely used one of Goff & Jordan (Reference Goff and Jordan1988), also the choice in Radko (Reference Radko2023)) have significant shortcomings. While the abyssal hills, consistent with a fractal process (Goff & Jordan Reference Goff and Jordan1988), host the rate-controlling dynamics that facilitate upwelling (Polzin & McDougall Reference Polzin and McDougall2022), they lack larger-scale topographic features that (a) can host nonlinear non-propagating dynamics and (b) are essential for prescription of correct boundary conditions for lee wave generation in models. These issues impact the drag and mixing representation adversely (Klymak et al. Reference Klymak, Balwada, Garabato and Abernathey2021; Baker & Mashayek Reference Baker and Mashayek2022; Polzin & McDougall Reference Polzin and McDougall2022).
2. The quasi-geostrophic framework employed in Radko (Reference Radko2023) systematically prohibits accounting for many ageostrophic submesoscale and radiating processes. Propagating internal tides and lee waves can significantly impact the vertical momentum distribution in the water column. They can also have a non-local impact on momentum and density budgets across the globe (Shakespeare & Hogg Reference Shakespeare and Hogg2019; de Lavergne et al. Reference de Lavergne, Vic, Madec, Roquet, Waterhouse, Whalen, Cuypers, Bouruet-Aubertot, Ferron and Hibiya2020; Baker & Mashayek Reference Baker and Mashayek2021). A host of other processes at the bottom boundary, such as a zoo of submesoscale instabilities and hydraulically controlled flow through narrow constrictions and passages, also have important implications for drag and mixing (Polzin & McDougall Reference Polzin and McDougall2022). While the internal gravity waves are not accounted for by Radko (Reference Radko2023), the eddy viscosity mimics the impact of other bottom-dwelling processes. Extension of the model to primitive equations, as suggested by the author, will go a long way towards allowing for these processes by extending the range of scales permitted and accounting for the density stratification.
3. Finally, flow–topography interactions can also prove significant for budgets of tracers such as carbon, nutrients and heat. For example, tracers have been found to concentrate around rough topography in the Southern Ocean in patterns similar to those in figure 1 (Mashayek et al. Reference Mashayek, Ferrari, Merrifield, Ledwell, St Laurent and Garabato2017; Prend et al. Reference Prend, Gille, Talley, Mitchell, Rosso and Mazloff2019). Including tracer budgets in the theory seems straightforward and can prove quite insightful.
In summary, Radko's work provides a solid base upon which the representation of rough ocean topography in ESMs can evolve.
Acknowledgements
A.M. thanks L. Cimoli for constructive comments.
Funding
The author acknowledges NERC grant NE/P018319/1 and ONR grant N00014-22-1-2082.
Declaration of interest
The author reports no conflict of interest.