Published online by Cambridge University Press: 31 August 2018
The pressure–strain-rate correlation and pressure fluctuations in convective and near neutral atmospheric surface layers are investigated. Their scaling properties, spectral characteristics, the contributions from the different source terms in the pressure Poisson equation and the effects of the wall are investigated using high-resolution (up to 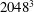 $2048^{3}$) large-eddy simulation fields and through spectral predictions. The pressure–strain-rate correlation was found to have the mixed-layer and surface-layer scaling in the strongly convective and near neutral atmospheric surface layers, respectively. Its apparent surface-layer scaling in the moderately convective surface layer is due to the slow variations of the mixed-layer contribution, and is an inherent problem for single-point statistics in a multi-scale surface layer. In the strongly convective surface layer the pressure spectrum has an approximate
$2048^{3}$) large-eddy simulation fields and through spectral predictions. The pressure–strain-rate correlation was found to have the mixed-layer and surface-layer scaling in the strongly convective and near neutral atmospheric surface layers, respectively. Its apparent surface-layer scaling in the moderately convective surface layer is due to the slow variations of the mixed-layer contribution, and is an inherent problem for single-point statistics in a multi-scale surface layer. In the strongly convective surface layer the pressure spectrum has an approximate 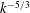 $k^{-5/3}$ scaling range for small wavenumbers (
$k^{-5/3}$ scaling range for small wavenumbers (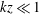 $kz\ll 1$) due to the turbulent–turbulent contribution, and does not follow the surface-layer scaling, where
$kz\ll 1$) due to the turbulent–turbulent contribution, and does not follow the surface-layer scaling, where 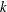 $k$ and
$k$ and  $z$ are the horizontal wavenumber and the distance from the surface respectively. The pressure–strain-rate cospectrum components have a
$z$ are the horizontal wavenumber and the distance from the surface respectively. The pressure–strain-rate cospectrum components have a 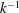 $k^{-1}$ scaling range, consistent with our prediction using the surface layer parameters. It is dominated by the buoyancy contribution. Thus the anisotropy in the surface layer is due to the energy redistribution caused by the density fluctuations of the large eddies, rather than the turbulent–turbulent (inertial) effects. In the near neutral surface layer, the turbulent–turbulent and rapid contributions are primarily responsible for redistribution of energy from the streamwise velocity component to the vertical and spanwise components, respectively. The pressure–strain-rate cospectra peak near
$k^{-1}$ scaling range, consistent with our prediction using the surface layer parameters. It is dominated by the buoyancy contribution. Thus the anisotropy in the surface layer is due to the energy redistribution caused by the density fluctuations of the large eddies, rather than the turbulent–turbulent (inertial) effects. In the near neutral surface layer, the turbulent–turbulent and rapid contributions are primarily responsible for redistribution of energy from the streamwise velocity component to the vertical and spanwise components, respectively. The pressure–strain-rate cospectra peak near  $kz\sim 1$, and have some similarities to those in the strongly convective surface layer for
$kz\sim 1$, and have some similarities to those in the strongly convective surface layer for  $kz\ll 1$. For the moderately convective surface layer, the pressure–strain-rate cospectra change signs at scales of the order of the Obukhov length, thereby imposing it as a horizontal length scale in the surface layer. This result provides strong support to the multipoint Monin–Obukhov similarity recently proposed by Tong & Nguyen (J. Atmos. Sci., vol. 72, 2015, pp. 4337–4348). We further decompose the pressure into the free-space (infinite domain), the wall reflection and the harmonic contributions. In the strongly convective surface layer, the free-space contribution to the pressure–strain-rate correlation is dominated by the buoyancy part, and is the main cause of the surface-layer anisotropy. The wall reflection enhances the anisotropy for most of the surface layer, suggesting that the pressure source has a large coherence length. In the near neutral surface layer, the wall reflection is small, suggesting a much smaller source coherence length. The present study also clarifies the understanding of the role of the turbulent–turbulent pressure, and has implications for understanding the dynamics and structure as well as modelling the atmospheric surface layer.
$kz\ll 1$. For the moderately convective surface layer, the pressure–strain-rate cospectra change signs at scales of the order of the Obukhov length, thereby imposing it as a horizontal length scale in the surface layer. This result provides strong support to the multipoint Monin–Obukhov similarity recently proposed by Tong & Nguyen (J. Atmos. Sci., vol. 72, 2015, pp. 4337–4348). We further decompose the pressure into the free-space (infinite domain), the wall reflection and the harmonic contributions. In the strongly convective surface layer, the free-space contribution to the pressure–strain-rate correlation is dominated by the buoyancy part, and is the main cause of the surface-layer anisotropy. The wall reflection enhances the anisotropy for most of the surface layer, suggesting that the pressure source has a large coherence length. In the near neutral surface layer, the wall reflection is small, suggesting a much smaller source coherence length. The present study also clarifies the understanding of the role of the turbulent–turbulent pressure, and has implications for understanding the dynamics and structure as well as modelling the atmospheric surface layer.