Hostname: page-component-78c5997874-8bhkd Total loading time: 0 Render date: 2024-11-03T04:01:27.326Z Has data issue: false hasContentIssue false
  • English
  • Français

Internally heated convection beneath a poor conductor

Published online by Cambridge University Press:  14 April 2015

David Goluskin
David Goluskin*
Affiliation:
Mathematics Department, University of Michigan, Ann Arbor, MI 48109, USA Center for the Study of Complex Systems, University of Michigan, Ann Arbor, MI 48109, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We consider convection in an internally heated (IH) layer of fluid that is bounded below by a perfect insulator and above by a poor conductor. The poorly conducting boundary is modelled by a fixed heat flux. Using solely analytical methods, we find linear and energy stability thresholds for the static state, and we construct a lower bound on the mean temperature that applies to all flows. 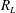The linear stability analysis yields a Rayleigh number above which the static state is linearly unstable ($R_{L}$), and the energy analysis yields a Rayleigh number below which it is globally stable (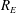$R_{E}$). For various boundary conditions on the velocity, exact expressions for 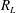$R_{L}$ and 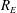$R_{E}$ are found using long-wavelength asymptotics. Each $R_{E}$ is strictly smaller than the corresponding 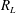$R_{L}$ but is within 1 %. The lower bound on the mean temperature is proven for no-slip velocity boundary conditions using the background method. The bound guarantees that the mean temperature of the fluid, relative to that of the top boundary, grows with the heating rate (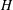$H$) no slower than $H^{2/3}$.

JFM classification

Convection: Convection Instability: Instability Mathematical Foundations: Variational methods
Type
Papers
Information
Journal of Fluid Mechanics , Volume 771 , 25 May 2015 , pp. 36 - 56
Check for updates
Copyright
© 2015 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ahlers, G., Grossmann, S. & Lohse, D. 2009 Heat transfer and large scale dynamics in turbulent Rayleigh–Bénard convection. Rev. Mod. Phys. 81 (2), 503–537.Google Scholar
Asfia, F. J. & Dhir, V. K. 1996 An experimental study of natural convection in a volumetrically heated spherical pool bounded on top with a rigid wall. Nucl. Engng Des. 163 (3), 333–348.CrossRefGoogle Scholar
Berlengiero, M., Emanuel, K. A., von Hardenberg, J., Provenzale, A. & Spiegel, E. A. 2012 Internally cooled convection: a fillip for Philip. Commun. Nonlinear Sci. Numer. Simul. 17 (5), 1998–2007.Google Scholar
Busse, F. H. 2014 Remarks on the critical value $P_{c}=0.25$ of the Prandtl number for internally heated convection found by Tveitereid and Palm. Eur. J. Mech. (B/Fluids) 47, 32–34.Google Scholar
Cartland Glover, G., Fujimura, K. & Generalis, S. 2013 Pattern formation in volumetrically heated fluids. Chaotic Model. Simul. 1, 19–30.Google Scholar
Cartland Glover, G. M. & Generalis, S. C. 2009 Pattern competition in homogeneously heated fluid layers. Engng Appl. Comput. Fluid Mech. 3 (2), 164–174.Google Scholar
Chandrasekhar, S. 1981 Hydrodynamic and Hydromagnetic Stability. Dover Publications.Google Scholar
Chapman, C. J., Childress, S. & Proctor, M. R. E. 1980 Long wavelength thermal convection between non-conducting boundaries. Earth Planet. Sci. Lett. 51, 362–369.Google Scholar
Chapman, C. J. & Proctor, M. R. E. 1980 Nonlinear Rayleigh–Bénard convection between poorly conducting boundaries. J. Fluid Mech. 101 (04), 759–782.Google Scholar
Chernyshenko, S. I., Goulart, P., Huang, D. & Papachristodoulou, A. 2014 Polynomial sum of squares in fluid dynamics: a review with a look ahead. Phil. Trans. R. Soc. Lond. A 372 (2020), doi:10.1098/rsta.2013.0350.Google ScholarPubMed
Childress, S. & Spiegel, E. A. 2004 Pattern formation in a suspension of swimming microorganisms: nonlinear aspects. In A Celebr. Math. Model. (ed. Givoli, D., Grote, M. J. & Papanicolaou, G. C.). Kluwer Academic Publishers.Google Scholar
Constantin, P. & Doering, C. R. 1996 Variational bounds on energy dissipation in incompressible flows. III. Convection. Phys. Rev. E 53 (6), 5957–5981.Google Scholar
Depassier, M. C. & Spiegel, E. A. 1982 Convection with heat flux prescribed on the boundaries of the system. I. The effect of temperature dependence of material properties. Geophys. Astrophys. Fluid Dyn. 21, 167–188.Google Scholar
Doering, C. R. & Constantin, P. 1992 Energy dissipation in shear driven turbulence. Phys. Rev. Lett. 69 (11), 1648–1651.Google Scholar
Fisher, P. F., Lottes, J. W. & Kerkemeier, S. G.2013 nek5000 Web page,http://nek5000.mcs.anl.gov.Google Scholar
Getling, A. V. 1998 Rayleigh–Bénard Convection: Structures and Dynamics. World Scientific Publishing Co.Google Scholar
Goluskin, D. 2015 Internally heated convection and Rayleigh–Bénard convection. In Springer Briefs in Thermal Engineering and Applied Science. Springer (in press).Google Scholar
Goluskin, D., Johnston, H., Flierl, G. R. & Spiegel, E. A. 2014 Convectively driven shear and decreased heat flux. J. Fluid Mech. 759, 360–385.Google Scholar
Grötzbach, G. & Wörner, M. 1999 Direct numerical and large eddy simulations in nuclear applications. Intl J. Heat Fluid Flow 20 (3), 222–240.Google Scholar
Herron, I. H. 2001 On the principle of exchange of stabilities in Rayleigh–Bénard convection. SIAM J. Appl. Maths 61 (4), 1362–1368.Google Scholar
Herron, I. H. 2003 On the principle of exchange of stabilities in Rayleigh–Bénard convection, II – no-slip boundary conditions. Ann. Univ. Ferrara IL, 169–182.Google Scholar
Hewitt, J. M., McKenzie, D. P. & Weiss, N. O. 1980 Large aspect ratio cells in two-dimensional thermal convection. Earth Planet. Sci. Lett. 51, 370–380.CrossRefGoogle Scholar
Houseman, G. 1988 The dependence of convection planform on mode of heating. Nature 332, 346–349.Google Scholar
Howard, L. N. 1963 Heat transport by turbulent convection. J. Fluid Mech. 17 (3), 405–432.Google Scholar
Hurle, D. T. J., Jakeman, E. & Pike, E. R. 1967 On the solution of the Bénard problem with boundaries of finite conductivity. Proc. R. Soc. Lond. A 296 (1447), 469–475.Google Scholar
Ichikawa, H., Kurita, K., Yamagishi, Y. & Yanagisawa, T. 2006 Cell pattern of thermal convection induced by internal heating. Phys. Fluids 18 (3), 038101.Google Scholar
Ingersoll, A. P. & Porco, C. C. 1978 Solar heating and internal heat flow on Jupiter. Icarus 35, 27–43.Google Scholar
Ishiwatari, M., Takehiro, S.-I. & Hayashi, Y.-Y. 1994 The effects of thermal conditions on the cell sizes of two-dimensional convection. J. Fluid Mech. 281, 33–50.Google Scholar
Johnston, H. & Doering, C. R. 2009 Comparison of turbulent thermal convection between conditions of constant temperature and constant flux. Phys. Rev. Lett. 102 (6), 064501.Google Scholar
Joseph, D. D. 1965 On the stability of the Boussinesq equations. Arch. Rat. Mech. Anal. 20 (1), 59–71.Google Scholar
Joseph, D. D. 1976 Stability of Fluid Motions I–II. Springer.Google Scholar
Kaiser, R., Tilgner, A. & Von Wahl, W. 2005 A generalized energy functional for plane Couette flow. SIAM J. Math. Anal. 37 (2), 438–454.Google Scholar
Kaspi, Y., Flierl, G. R. & Showman, A. P. 2009 The deep wind structure of the giant planets: results from an anelastic general circulation model. Icarus 202 (2), 525–542.Google Scholar
Kippenhahn, R. & Weigert, A. 1994 Stellar Structure and Evolution. Springer.Google Scholar
Kulacki, F. A. & Goldstein, R. J. 1975 Hydrodynamic instability in fluid layers with uniform volumetric energy sources. Appl. Sci. Res. 31 (2), 81–109.Google Scholar
Kulacki, F. A. & Richards, D. E. 1985 Natural convection in plane layers and cavities with volumetric energy sources. In Nat. Convect. Fundam. Appl., pp. 179–254. Hemisphere.Google Scholar
Lord Rayleigh 1916 On convection currents in a horizontal layer of fluid, when the higher temperature is on the under side. Phil. Mag. 32 (192), 529–546.Google Scholar
Lu, L., Doering, C. R. & Busse, F. H. 2004 Bounds on convection driven by internal heating. J. Math. Phys. 45 (7), 2967–2986.Google Scholar
Malkus, W. V. R. 1954 Discrete transitions in turbulent convection. Proc. R. Soc. Lond. A 225 (1161), 185–195.Google Scholar
Nourgaliev, R. R., Dinh, T. N. & Sehgal, B. R. 1997 Effect of fluid Prandtl number on heat transfer characteristics in internally heated liquid pools with Rayleigh numbers up to $10^{12}$ . Nucl. Engng Des. 169, 165–184.Google Scholar
Otero, J., Wittenberg, R. W., Worthing, R. A. & Doering, C. R. 2002 Bounds on Rayleigh–Bénard convection with an imposed heat flux. J. Fluid Mech. 473, 191–199.Google Scholar
Parmentier, E. M., Sotin, C. & Travis, B. J. 1994 Turbulent 3-D thermal convection in an infinite Prandtl number, volumetrically heated fluid: implications for mantle dynamics. Geophys. J. Intl 116 (2), 241–251.Google Scholar
Pellew, A. & Southwell, R. V. 1940 On maintained convective motion in a fluid heated from below. Proc. R. Soc. Lond. A 176, 312–343.Google Scholar
Plasting, S. C. & Kerswell, R. R. 2003 Improved upper bound on the energy dissipation rate in plane Couette flow: the full solution to Busse’s problem and the Constantin–Doering–Hopf problem with one-dimensional background field. J. Fluid Mech. 477, 363–379.Google Scholar
van der Poel, E. P., Ostilla-Mónico, R., Verzicco, R. & Lohse, D. 2014 Effect of velocity boundary conditions on the heat transfer and flow topology in two-dimensional Rayleigh–Bénard convection. Phys. Rev. E 90 (1), 013017.Google Scholar
Roberts, P. H. 1967 Convection in horizontal layers with internal heat generation. Theory. J. Fluid Mech. 30 (1), 33–49.Google Scholar
Schubert, G., Glatzmaier, G. A. & Travis, B. 1993 Steady, three-dimensional, internally heated convection. Phys. Fluids A 5 (8), 1928–1932.Google Scholar
Schubert, G., Turcotte, D. L. & Olson, P. 2001 Mantle Convection in the Earth and Planets. Cambridge University Press.Google Scholar
Serrin, J. 1959 On the stability of viscous fluid motions. Arch. Rat. Mech. Anal. 3 (1), 1–13.Google Scholar
Siggia, E. D. 1994 High Rayleigh number convection. Annu. Rev. Fluid Mech. 26, 137–168.Google Scholar
Sparrow, E. M., Goldstein, R. J. & Jonsson, V. K. 1963 Thermal instability in a horizontal fluid layer: effect of boundary conditions and non-linear temperature profile. J. Fluid Mech. 18 (04), 513–528.Google Scholar
Spiegel, E. A. & Veronis, G. 1960 On the Boussinesq approximation for a compressible fluid. Astrophys. J. 131, 442–447.Google Scholar
Straughan, B. 1990 Continuous dependence on the heat source and non-linear stability for convection with internal heat generation. Math. Meth. Appl. Sci. 13, 373–383.Google Scholar
Straughan, B. 2004 The Energy Method, Stability, and Nonlinear Convection, 2nd edn. Springer.CrossRefGoogle Scholar
Takahashi, J., Tasaka, Y., Murai, Y., Takeda, Y. & Yanagisawa, T. 2010 Experimental study of cell pattern formation induced by internal heat sources in a horizontal fluid layer. Intl J. Heat Mass Transfer 53 (7–8), 1483–1490.CrossRefGoogle Scholar
Thirlby, R. 1970 Convection in an internally heated layer. J. Fluid Mech. 44 (4), 673–693.Google Scholar
Tritton, D. J. & Zarraga, M. N. 1967 Convection in horizontal layers with internal heat generation. Experiments. J. Fluid Mech. 30 (1), 21–31.Google Scholar
Tveitereid, M. & Palm, E. 1976 Convection due to internal heat sources. J. Fluid Mech. 76, 499.Google Scholar
Verzicco, R. & Sreenivasan, K. R. 2008 A comparison of turbulent thermal convection between conditions of constant temperature and constant heat flux. J. Fluid Mech. 595, 203–219.Google Scholar
Wittenberg, R. W. 2010 Bounds on Rayleigh–Bénard convection with imperfectly conducting plates. J. Fluid Mech. 665, 158–198.Google Scholar